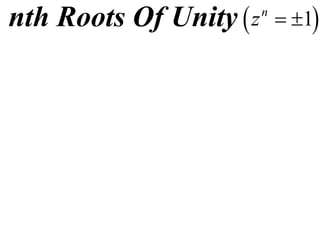
X2 T01 07 nth roots of unity
- 1. nth Roots Of Unity z n 1
- 2. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity
- 3. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle.
- 4. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1
- 5. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5
- 6. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis 5 5 5 5
- 7. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis y 5 5 5 5 x
- 8. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis y 5 5 5 5 cis0 x
- 9. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis y 2 5 5 5 5 cis 5 cis0 x
- 10. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis y 2 5 5 5 5 cis 5 cis0 x 2 cis 5
- 11. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis 4 y 2 5 5 5 5 cis cis 5 5 cis0 x 2 cis 5
- 12. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis 4 y 2 5 5 5 5 cis cis 5 5 cis0 x 4 cis 2 5 cis 5
- 13. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis 4 y 2 5 5 5 5 cis cis 5 5 cis0 x 4 cis 2 5 cis 5
- 14. nth Roots Of Unity z n 1 The solutions of equations of the form, z n 1, are the nth roots of unity When placed on an Argand Diagram, they form a regular n sided polygon, with vertices on the unit circle. e.g . z 5 1 2 k 0 z cis k 0, 1, 2 5 2 2 4 4 z cis 0, cis , cis , cis , cis 4 y 2 5 5 5 5 cis cis 5 5 cis0 x 4 cis 2 5 cis 5
- 15. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots.
- 16. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1
- 17. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1
- 18. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1
- 19. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5
- 20. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2
- 21. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 12 1
- 22. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 12 1 2 is a solution
- 23. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 12 1 2 is a solution
- 24. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 12 13 1 1 2 is a solution
- 25. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 12 13 1 1 2 is a solution 3 is a solution
- 26. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 4 5 5 4 12 13 1 1 2 is a solution 3 is a solution
- 27. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 4 5 5 4 12 13 14 1 1 1 2 is a solution 3 is a solution
- 28. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 4 5 5 4 12 13 14 1 1 1 2 is a solution 3 is a solution 4 is a solution
- 29. b) i If is a complex root of z 5 1 0, show that 2 , 3 , 4 and 5 are the other roots. z5 1 If is a solution then 5 1 15 5 5 1 is a solution 5 2 5 5 2 3 5 5 3 4 5 5 4 12 13 14 1 1 1 2 is a solution 3 is a solution 4 is a solution Thus if is a root then 2 , 3 , 4 and 5 are also roots
- 30. ii Prove that 1 2 3 4 0
- 31. ii Prove that 1 2 3 4 0 b 2 3 4 5 a
- 32. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a
- 33. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0
- 34. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1
- 35. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1 OR 1 0 5
- 36. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1 OR 1 0 5 11 2 3 4 0
- 37. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 11 2 n1 0
- 38. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0
- 39. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0 OR 1 2 3 4
- 40. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0 OR 1 2 3 4 GP: a 1, r , n 5
- 41. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1
- 42. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 1
- 43. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 0 1
- 44. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 5 1 OR 1 0 5 NOTE : n 11 2 3 4 0 1 0 1 2 3 4 0 1 11 2 n1 0 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1
- 45. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3
- 46. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3 4 2 3
- 47. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3 4 2 3 1
- 48. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3 4 2 3 4 2 3 1
- 49. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3 4 2 3 4 2 3 1 3 4 6 7
- 50. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3 4 2 3 4 2 3 1 3 4 6 7 3 4 2 1
- 51. ii Prove that 1 2 3 4 0 b 2 3 4 5 sum of the roots a 1 2 3 4 0 1 5 OR 1 0 5 NOTE : n 11 0 2 3 4 1 0 1 2 3 4 0 11 2 n1 0 1 OR a r n 1 1 2 3 4 GP: a 1, r , n 5 r 1 1 5 1 5 1 0 0 1 iii Find the quadratic equation whose roots are 4 and 2 3 4 2 3 4 2 3 1 3 4 6 7 3 4 2 equation is x 2 x 1 0 1
- 52. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to;
- 53. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3
- 54. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 3
- 55. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 3 3 1
- 56. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 3 1
- 57. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1
- 58. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 2
- 59. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 2 2 2 1
- 60. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 (iii) Form the cubic equation with roots 1, 1 , 1 2 2 2 2 1
- 61. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 (iii) Form the cubic equation with roots 1, 1 , 1 2 2 z 1z 2 z 1 0 2 2 2 3 2 2 1
- 62. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 (iii) Form the cubic equation with roots 1, 1 , 1 2 2 z 1z 2 z 1 0 2 2 2 3 2 2 z 1z 2 z 1 0 1
- 63. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 (iii) Form the cubic equation with roots 1, 1 , 1 2 2 z 1z 2 z 1 0 2 2 2 3 2 2 z 1z 2 z 1 0 1 z z z z z 1 0 3 2 2
- 64. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 (iii) Form the cubic equation with roots 1, 1 , 1 2 2 z 1z 2 z 1 0 2 2 2 3 2 2 z 1z 2 z 1 0 1 z z z z z 1 0 3 2 2 z3 2z 2 2z 1 0
- 65. c) If is a complex cube root of unity, use the fact that 1 ω ω 2 0 to; (i ) Evaluate 1 2 3 1 1 (ii ) Evaluate 1 1 2 3 1 1 2 3 1 1 (iii) Form the cubic equation with roots 1, 1 , 1 2 2 z 1z 2 z 1 0 2 2 2 3 2 2 z 1z 2 z 1 0 1 z z z z z 1 0 3 2 2 z3 2z 2 2z 1 0 Exercise 4I; 1, 3, 5 (need 4b), 7