This document discusses conformal mapping and provides examples of how it can transform complex functions and geometries while preserving angles. Specifically: - A conformal map transforms one complex coordinate system to another using a transformation function, preserving angles between curves. - Joukowski's transformation maps a circle in one plane to an airfoil-shaped curve in another plane, and can be used to analyze fluid flow around an airfoil by mapping it to simplified flow around a circle. - Examples show circles and lines transforming to hyperbolas and parabolas under different functions, and circles transforming to circles or lines, depending on conditions. This demonstrates the angle-preserving nature of conformal mapping.

2. What is conformal mapping?
• A conformal map is the transformation of a complex
valued function from one coordinate system to
another.
• This is accomplished by means of a transformation
function that is applied to the original complex
function.
For example, consider a
complex plane z shown.
Coordinates in this plane
are defined with the
complex function z=x + iy.
This is mapped to
w=f(z)=u(x,y)+iv(x,y).

3. •A conformal mapping can be used to transform this complex plane z into a new
complex plane given by w = f(z). This figure shows is the example if w = √z.
The variables x and y in the z plane have been transformed to the new variables u
and v.
•Note that while this transformation has changed the relative shape of the
streamlines and equi-potential curves, the set of curves remain perpendicular.
This angle preserving feature is the essential component of conformal mapping.

4. Two transformations examples
 w=z2
→ u+iv = (x+iy)2 = x2 - y2 + 2ixy
→so, u= x2 - y2 & v= 2xy
Case 1/a
In w-plane, let u=a.
Then x2 - y2 = a (This is a rectangular hyperbola.)
Case 1/b
In w-plane, let v=b.
b=2xy → xy=b/2 (This is a rectangular hyperbola.)
Both are rectangular hyperbola…and they are orthogonal.
So lines u=a & v=b(parallel to the axis) in w-plane is mapped
to orthogonal hyperbolas in z-plane.

5. Case 2/a
In z-plane let x=c
x2 - y2 = u xy=v/2
→ y2 = x2 - u →y=v/2c
• Eliminating y from both these equations, we have
v2=4c2(c2-u), which is a parabola in w-plane.
• Similarly by keeping y=d, in z-plane.
We get v2=4d2(c2+u), which is also a parabola.
• Both these parabolas are again orthogonal.
So the straight line parallel to the axis in z-plane is
mapped to orthogonal parabolas in w-plane.

6. Example 2
w=1/z
→ z=1/w
→x+iy = 1/(u+iv) = {(u-iv)/(u2+v2)}
• Comparing both sides
x= u/(u2+v2) & y=-v/(u2+v2)
Now let us see how this transformation works for a
circle.
• The most general equation of a circle is
x2 + y2 + 2gx + 2fy + c = 0
Substituting x and y from above
We get
→ c(u2 +v2) + 2gu – 2fv + 1=0 in w-plane.

7. Case 1
c = 0
c(u2 +v2) + 2gu – 2fv + 1=0 is a equation of a circle in w-
plane.
• So the circle in z-plane is mapped to another circle in
w-plane.
Case 2
c=0 (i.e. the circle is passing through the origin with
center (-g,-f) in z-plane )
c(u2 +v2) + 2gu – 2fv + 1=0
→ 2gu – 2fv + 1=0 which is a straight line in w-plane
So the function w=1/z maps a circle in z-plane onto a
circle in w-plane provided that the circle in z-plane
should not pass through origin.

8. Aerodynamic in air foil
• Now we will use a conformal mapping
technique to study flow of fluid around a
airfoil.

9. • Using this technique, the fluid flow around the
geometry of an airfoil can be analyzed as the flow
around a cylinder whose symmetry simplifies the
needed computations. The name of the
transformation is Joukowski’s transformation

10. Joukowski’s transformation
• The joukowski's transformation is used because it has the
property of transforming circles in the z plane into
shapes that resemble airfoils in the w plane.
• The function in z-plane is a circle given by
Where b is the radius of the circle and ranges from 0 to 2∏.
• The joukowski's transformation is given by the function
Where w is the function in the transformed w-plane, and λ
is the transformation parameter that determines the
resulting shape of the transformed function.

11. • For λ = b, the circle is mapped into a at plate
going from -2b to 2b.
• Setting the transformation parameter larger
than b causes the circle to be mapped into an
ellipse.

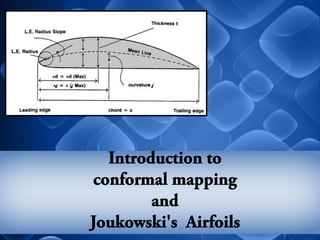
12. •The airfoil shape is realized by creating a circle in the z plane with a
centre that is offset from the origin, If the circle in the z plane is
offset slightly, the desired transformation parameter
is given by
Where s is the coordinates of the centre of the circle.
•The transformation in the w plane resembles the shape of an
airfoil symmetric about the x axis. The x coordinate of the circle
origin therefore determines the thickness distribution of
the transformed airfoil.

13. • If the centre of the circle in the z plane is also
offset on the y axis, the joukowski's
transformation yields an unsymmetrical airfoil.
This shows that the y coordinate of the circle
centre determines the curvature of the
transformed airfoil.

14. • In addition to the circle in the z plane being
transformed to air foils in w-plane, the flow
around the circle can also be transformed
because of the previously mentioned angle
preserving feature of conformal mapping
functions.
• This requires that the velocity potential and
stream function should be expressed as a
complex function. This is accomplished by
expressing the velocity potential and stream
function in a complex potential, given by
Where ɸ is velocity potential function and Ψ is
streamline function.

15. Please visit(for more presentations)