Beam deflection gere

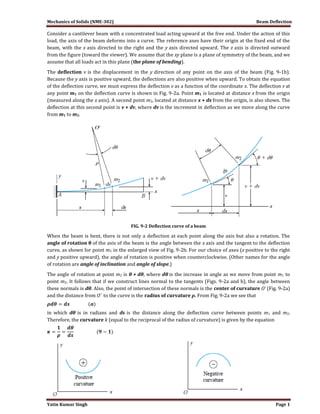
Mechanics of Solids (NME-302) Beam Deflection
Yatin Kumar Singh Page 1
Consider a cantilever beam with a concentrated load acting upward at the free end. Under the action of this
load, the axis of the beam deforms into a curve. The reference axes have their origin at the fixed end of the
beam, with the x axis directed to the right and the y axis directed upward. The z axis is directed outward
from the figure (toward the viewer). We assume that the xy plane is a plane of symmetry of the beam, and we
assume that all loads act in this plane (the plane of bending).
The deflection v is the displacement in the y direction of any point on the axis of the beam (Fig. 9-1b).
Because the y axis is positive upward, the deflections are also positive when upward. To obtain the equation
of the deflection curve, we must express the deflection v as a function of the coordinate x. The deflection v at
any point m1 on the deflection curve is shown in Fig. 9-2a. Point m1 is located at distance x from the origin
(measured along the x axis). A second point m2, located at distance x + dx from the origin, is also shown. The
deflection at this second point is v + dv, where dv is the increment in deflection as we move along the curve
from m1 to m2.
FIG. 9-2 Deflection curve of a beam
When the beam is bent, there is not only a deflection at each point along the axis but also a rotation. The
angle of rotation θ of the axis of the beam is the angle between the x axis and the tangent to the deflection
curve, as shown for point m1 in the enlarged view of Fig. 9-2b. For our choice of axes (x positive to the right
and y positive upward), the angle of rotation is positive when counterclockwise. (Other names for the angle
of rotation are angle of inclination and angle of slope.)
The angle of rotation at point m2 is θ + dθ, where dθ is the increase in angle as we move from point m1 to
point m2. It follows that if we construct lines normal to the tangents (Figs. 9-2a and b), the angle between
these normals is dθ. Also, the point of intersection of these normals is the center of curvature O’ (Fig. 9-2a)
and the distance from O’ to the curve is the radius of curvature ρ. From Fig. 9-2a we see that
in which dθ is in radians and ds is the distance along the deflection curve between points m1 and m2.
Therefore, the curvature k (equal to the reciprocal of the radius of curvature) is given by the equation

The sign convention for curvature is pictured in Fig. Note that curvature is positive when the angle of
rotation increases as we move along the beam in the positive x direction.
The slope of the deflection curve is the first derivative dv/dx of the expression for the deflection v. In
geometric terms, the slope is the increment dv in the deflection (as we go from point m1 to point m2 in Fig. 9-
2) divided by the increment dx in the distance along the x axis. Since dv and dx are infinitesimally small, the
slope dv/dx is equal to the tangent of the angle of rotation θ (Fig. 9-2b). Thus,
In a similar manner, we also obtain the following relationships:
Note that when the x and y axes have the directions shown in Fig. 9-2a, the slope dv/dx is positive when the
tangent to the curve slopes upward to the right.
Equations (9-1) through (9-3) are based only upon geometric considerations, and therefore they are valid
for beams of any material. Furthermore, there are no restrictions on the magnitudes of the slopes and
deflections.
Beams with Small Angles of Rotation:
The structures encountered in everyday life, such as buildings, automobiles, aircraft, and ships, undergo
relatively small changes in shape while in service. The changes are so small as to be unnoticed by a casual
observer. Consequently, the deflection curves of most beams and columns have very small angles of rotation,
very small deflections, and very small curvatures. Under these conditions we can make some mathematical
approximations that greatly simplify beam analysis.
Consider, for instance, the deflection curve shown in Fig. 9-2. If the angle of rotation θ is a very small
quantity (and hence the deflection curve is nearly horizontal), we see immediately that the distance ds along
the deflection curve is practically the same as the increment dx along the x axis. This same conclusion can be
obtained directly from Eq. (9-3a). Since when the angle θ is small, Eq. (9-3a) gives
With this approximation, the curvature becomes
Also, since when θ is small, we can make the following approximation
Thus, if the rotations of a beam are small, we can assume that the angle of rotation θ and the slope dv/dx are
equal. (Note that the angle of rotation must be measured in radians.) Taking the derivative of θ with respect
to x in Eq. (c), we get
Combining this equation with Eq. (9-4), we obtain a relation between the curvature of a beam and its
deflection:
This equation is valid for a beam of any material, provided the rotations are small quantities. If the material
of a beam is linearly elastic and follows Hooke’s law, the curvature is

in which M is the bending moment and EI is the flexural rigidity of the beam. Equation (9-6) shows that a
positive bending moment produces positive curvature and a negative bending moment produces negative
curvature. Combining Eq. (9-5) with Eq. (9-6) yields the basic differential equation of the deflection curve
of a beam:
This equation can be integrated in each particular case to find the deflection v, provided the bending moment
and flexural rigidity EI are known as functions of x.
As a reminder, the sign conventions to be used with the preceding equations are repeated here: (1) The x
and y axes are positive to the right and upward, respectively; (2) the deflection v is positive upward; (3) the
slope dv/dx and angle of rotation θ are positive when counterclockwise with respect to the positive x axis;
(4) the curvature k is positive when the beam is bent concave upward; and (5) the bending moment M is
positive when it produces compression in the upper part of the beam.
Additional equations can be obtained from the relations between bending moment M, shear force V, and
intensity q of distributed load.
Non-prismatic Beams:
In the case of a non-prismatic beam, the flexural rigidity EI is variable, and therefore we write Eq. (9-7) in the
form
where the subscript x is inserted as a reminder that the flexural rigidity may vary with x. Differentiating both
sides of this equation and using Eqs. (9-8a) and (9-8b), we obtain
The deflection of a non-prismatic beam can be found by solving (either analytically or numerically) any one
of the three preceding differential equations. The choice usually depends upon which equation provides the
most efficient solution.
Prismatic Beams:
In the case of a prismatic beam (constant EI), the differential equations become
To simplify the writing of these and other equations, primes are often used to denote differentiation:
Using this notation, we can express the differential equations for a prismatic beam in the following forms:
We will refer to these equations as the bending-moment equation, the shear-force equation, and the load
equation, respectively.
The general procedure consists of integrating the equations and then evaluating the constants of integration
from boundary and other conditions pertaining to the beam. When deriving the differential equations we
assumed that the material followed Hooke’s law and that the slopes of the deflection curve were very small.
We also assumed that any shear deformations were negligible; consequently, we considered only the
deformations due to pure bending. All of these assumptions are satisfied by most beams in common use.

Exact Expression for Curvature:
If the deflection curve of a beam has large slopes, we cannot use the approximations given by Eqs. (b) and
(c). Instead, we must resort to the exact expressions for curvature and angle of rotation. Combining those
expressions, we get
From Fig. 9-2 we see that
Dividing both sides of Eq. (g) by dx gives
Also, differentiation of the arctangent function gives
Substitution of expressions (i) and (j) into the equation for curvature (Eq. e) yields
Comparing this equation with Eq. (9-5), we see that the assumption of small rotations is equivalent to
disregarding (v’)2 in comparison to one. Equation (9-13) should be used for the curvature whenever the
slopes are large.
Deflections by Integration of the Bending-Moment Equation:
We are now ready to solve the differential equations of the deflection curve and obtain deflections of beams.
The first equation we will use is the bending-moment equation (Eq. 9-12a). Since this equation is of second
order, two integrations are required. The first integration produces the slope v’ = dv/dx, and the second
produces the deflection v.
We begin the analysis by writing the equation (or equations) for the bending moments in the beam. Since
only statically determinate beams are considered, we can obtain the bending moments from free-body
diagrams and equations of equilibrium. In some cases a single bending-moment expression holds for the
entire length of the beam. In other cases the bending moment changes abruptly at one or more points along
the axis of the beam. Then we must write separate bending-moment expressions for each region of the beam
between points where changes occur.
Regardless of the number of bending-moment expressions, the general procedure for solving the differential
equations is as follows. For each region of the beam, we substitute the expression for M into the differential
equation and integrate to obtain the slope v’. Each such integration produces one constant of integration.
Next, we integrate each slope equation to obtain the corresponding deflection v. Again, each integration
produces a new constant. Thus, there are two constants of integration for each region of the beam. These

constants are evaluated from known conditions pertaining to the slopes and deflections. The conditions fall
into three categories: (1) boundary conditions, (2) continuity conditions, and (3) symmetry conditions.
Boundary conditions pertain to the deflections and slopes at the supports of a beam. For example, at a
simple support (either a pin or a roller) the deflection is zero (Fig. 9-5), and at a fixed support both the
deflection and the slope are zero (Fig. 9-6). Each such boundary condition supplies one equation that can be
used to evaluate the constants of integration.
Continuity conditions occur at points where the regions of integration meet, such as at point C in the beam
of Fig. 9-7. The deflection curve of this beam is physically continuous at point C, and therefore the deflection
at point C as determined for the left-hand part of the beam must be equal to the deflection at point C as
determined for the right hand part. Similarly, the slopes found for each part of the beam must be equal at
point C. Each of these continuity conditions supplies an equation for evaluating the constants of integration.
Symmetry conditions may also be available. For instance, if a simple beam supports a uniform load
throughout its length, we know in advance that the slope of the deflection curve at the midpoint must be
zero.
Each boundary, continuity, and symmetry condition leads to an equation containing one or more of the
constants of integration. Since the number of independent conditions always matches the number of
constants of integration, we can always solve these equations for the constants. (The boundary and
continuity conditions alone are always sufficient to determine the constants. Any symmetry conditions
provide additional equations, but they are not independent of the other equations. The choice of which
conditions to use is a matter of convenience.)
Once the constants are evaluated, they can be substituted back into the expressions for slopes and
deflections, thus yielding the final equations of the deflection curve. These equations can then be used to
obtain the deflections and angles of rotation at particular points along the axis of the beam. The preceding
method for finding deflections is sometimes called the method of successive integrations. The following
examples illustrate the method in detail.
Example: Determine the equation of the deflection curve for a simple beam AB supporting a uniform load of
intensity q acting throughout the span of the beam. Also, determine the maximum deflection δmax at the
midpoint of the beam and the angles of rotation θA and θB at the supports. (Note: The beam has length L and
constant flexural rigidity EI.)
Solution:
Since the reaction at the support is qL/2, the equation for the bending moment is
Integrating each term, we obtain

in which C1 is a constant of integration. To evaluate the constant C1, we observe from the symmetry of the
beam and its load that the slope of the deflection curve at mid-span is equal to zero.
Thus, we have the following symmetry condition:
v’ = 0 when x = L/2
This condition may be expressed more succinctly as
As expected, the slope is negative (i.e., clockwise) at the left-hand end of the beam (x = 0), positive at the
right-hand end (x = L), and equal to zero at the midpoint (x = L/2). Multiplying both sides of Eq. by dx and
integrating, we obtain
The constant of integration C2 may be evaluated from the condition that the deflection of the beam at the left-
hand support is equal to zero; that is, v = 0 when x = 0, or v(0) = 0. Applying this condition to Eq. (c) yields C2
= 0;
This equation gives the deflection at any point along the axis of the beam. Note that the deflection is zero at
both ends of the beam (x = 0 and x = L) and negative elsewhere (recall that downward deflections are
negative).
From symmetry we know that the maximum deflection occurs at the midpoint of the span. Thus, setting x
equal to L/2
in which the negative sign means that the deflection is downward (as expected). Since δmax represents the
magnitude of this deflection, we obtain
The maximum angles of rotation occur at the supports of the beam. At the left-hand end of the beam, the
angle θA, which is a clockwise angle (Fig. 9-8b), is equal to the negative of the slope v’. Thus, by substituting x
= 0
In a similar manner, we can obtain the angle of rotation θB at the right-hand end of the beam. Since θB is a
counterclockwise angle, it is equal to the slope at the end:
Because the beam and loading are symmetric about the midpoint, the angles of rotation at the ends are
equal.

Deflections by Integration of the Shear-Force and Load Equations:
The equations of the deflection curve in terms of the shear force V and the load q may also be integrated to
obtain slopes and deflections. Since the loads are usually known quantities, whereas the bending moments
must be determined from free-body diagrams and equations of equilibrium, many analysts prefer to start
with the load equation. For this same reason, most computer programs for finding deflections begin with the
load equation and then perform numerical integrations to obtain the shear forces, bending moments, slopes,
and deflections.
The procedure for solving either the load equation or the shear-force equation is similar to that for solving
the bending-moment equation, except that more integrations are required. For instance, if we begin with the
load equation, four integrations are needed in order to arrive at the deflections. Thus, four constants of
integration are introduced for each load equation that is integrated. As before, these constants are found
from boundary, continuity, and symmetry conditions. However, these conditions now include conditions on
the shear forces and bending moments as well as conditions on the slopes and deflections.
Conditions on the shear forces are equivalent to conditions on the third derivative (because EIv’’’= V). In a
similar manner, conditions on the bending moments are equivalent to conditions on the second derivative
(because EIv’’ = M). When the shear-force and bending-moment conditions are added to those for the slopes
and deflections, we always have enough independent conditions to solve for the constants of integration.
Example: Determine the equation of the deflection curve for a cantilever beam AB supporting a triangularly
distributed load of maximum intensity q0. Also, determine the deflection δB and angle of rotation θB at the
free end. Use the fourth-order differential equation of the deflection curve (the load equation). (Note: The
beam has length L and constant flexural rigidity EI.)
Solution:
Differential equation of the deflection curve: The intensity of the distributed load is given by the following
equation:
Consequently, the fourth-order differential equation becomes
Shear force in the beam: The first integration of Eq. (a) gives
The right-hand side of this equation represents the shear force V. Because the shear force is zero at x = L, we
have the following boundary condition:
Using this condition with Eq. (b), we get C1 = 0. Therefore, Eq. (b) simplifies to
and the shear force in the beam is
Bending moment in the beam: Integrating a second time, we obtain the following equation from Eq. (c):
This equation is equal to the bending moment M. Since the bending moment is zero at the free end of the
beam, we have the following boundary condition:
Applying this condition to Eq. (d), we obtain C2 = 0, and therefore the bending moment is

Slope and deflection of the beam: The third and fourth integrations yield
The boundary conditions at the fixed support, where both the slope and deflection equal zero, are
Applying these conditions to Eqs. (e) and (f), respectively, we find
Substituting these expressions for the constants into Eqs. (e) and (f ), we obtain the following equations for
the slope and deflection of the beam:
Angle of rotation and deflection at the free end of the beam: The angle of rotation θB and deflection δB at
the free end of the beam are obtained from Eqs. (9-43) and (9-44), respectively, by substituting x = L. The
results are
Example: A simple beam AB with an overhang BC supports a concentrated load P at the end of the overhang
(Fig. 9-15a). The main span of the beam has length L and the overhang has length L/ 2. Determine the
equations of the deflection curve and the deflection δC at the end of the overhang (Fig. 9-15b). Use the third-
order differential equation of the deflection curve (the shear-force equation). (Note: The beam has constant
flexural rigidity EI.)
Solution:
Differential equations of the deflection curve: Because reactive forces act at supports A and B, we must
write separate differential equations for parts AB and BC of the beam. Therefore, we begin by finding the
shear forces in each part of the beam.
The downward reaction at support A is equal to P/2, and the upward reaction at support B is equal to 3P/2 .
It follows that the shear forces in parts AB and BC are
in which x is measured from end A of the beam
The third-order differential equations for the beam now become

Bending moments in the beam: Integration of the preceding two equations yields the bending-moment
equations:
The bending moments at points A and C are zero; hence we have the following boundary conditions:
Using these conditions with Eqs. (i) and (j), we get
Therefore, the bending moments are
Slopes and deflections of the beam: The next integrations yield the slopes:
The only condition on the slopes is the continuity condition at support B. According to this condition, the
slope at point B as found for part AB of the beam is equal to the slope at the same point as found for part BC
of the beam. Therefore, we substitute x = L into each of the two preceding equations for the slopes and obtain
This equation eliminates one constant of integration because we can express C4 in terms of C3:
The third and last integrations give
For part AB of the beam, we have two boundary conditions on the deflections, namely, the deflection is zero
at points A and B: v(0) = 0 ; v(L) = 0
Applying these conditions to Eq. (l), we obtain
Substituting the preceding expression for C3 in Eq. (k), we get
For part BC of the beam, the deflection is zero at point B. Therefore, the boundary condition is v(L) = 0.
Applying this condition to Eq. (m), and also substituting Eq. (p) for C4, we get
The deflection equations are obtained by substituting the constants of integration (Eqs. n, o, p, and q) into
Eqs. (l) and (m). The results are

Note that the deflection is always positive (upward) in part AB of the beam and always negative (downward)
in the overhang BC.
Deflection at the end of the overhang: We can find the deflection δC at the end of the overhang by
substituting x = 3L/ 2
Thus, we have determined the required deflections of the overhanging beam by solving the third-order
differential equation of the deflection curve.
Method of Superposition:
The method of superposition is a practical and commonly used technique for obtaining deflections and
angles of rotation of beams. The underlying concept is quite simple and may be stated as follows:
Under suitable conditions, the deflection of a beam produced by several different loads acting
simultaneously can be found by superposing the deflections produced by the same loads acting
separately.
For instance, if v1 represents the deflection at a particular point on the axis of a beam due to a load q1, and if
v2 represents the deflection at that same point due to a different load q2, then the deflection at that point due
to loads q1 and q2 acting simultaneously is v1+ v2. (The loads q1 and q2 are independent loads and each may
act anywhere along the axis of the beam.)
The justification for superposing deflections lies in the nature of the differential equations of the deflection
curve (Eqs. 9-12a, b, and c). These equations are linear differential equations, because all terms containing
the deflection v and its derivatives are raised to the first power. Therefore, the solutions of these equations
for several loading conditions may be added algebraically, or superposed.
As an illustration of the superposition method, consider the simple beam ACB shown in Fig. 9-16a. This
beam supports two loads: (1) a uniform load of intensity q acting throughout the span, and (2) a
concentrated load P acting at the midpoint. Suppose we wish to find the deflection δC at the midpoint and the
angles of rotation θA and θB at the ends (Fig. 9-16b).
Using the method of superposition, we obtain the effects of each load acting separately and then combine the
results. For the uniform load acting alone, the deflection at the midpoint and the angles of rotation are
obtained from the formulas:
in which EI is the flexural rigidity of the beam and L is its length. For the load P acting alone, the
corresponding quantities are obtained from the formulas:
The deflection and angles of rotation due to the combined loading (Fig. 9-16a) are obtained by summation:

The deflections and angles of rotation at other points on the beam axis can be found by this same procedure.
However, the method of superposition is not limited to finding deflections and angles of rotation at single
points. The method may also be used to obtain general equations for the slopes and deflections of beams
subjected to more than one load.
Distributed Loads:
We can consider an element of the distributed load as though it were a concentrated load, and then we can
find the required deflection by integrating throughout the region of the beam where the load is applied.
To illustrate this process of integration, consider a simple beam ACB with a triangular load acting on the left-
hand half. We wish to obtain the deflection δC at the midpoint C and the angle of rotation θA at the left-hand
support.
We begin by visualizing an element qdx of the distributed load as a concentrated load. Note that the load acts
to the left of the midpoint of the beam. The deflection at the midpoint due to this concentrated load (for the
case in which is
we substitute qdx for P and x for a:
This expression gives the deflection at point C due to the element qdx of the load. Next, we note that the
intensity of the uniform load is
where q0 is the maximum intensity of the load. With this substitution for q, the formula for the deflection (Eq.
c) becomes
Finally, we integrate throughout the region of the load to obtain the deflection δC at the midpoint of the beam
due to the entire triangular load:
By a similar procedure, we can calculate the angle of rotation θA at the left-hand end of the beam. The
expression for this angle due to a concentrated load P is
Replacing P with 2q0 x dx/L, a with x, and b with L - x, we obtain

Finally, we integrate throughout the region of the load:
This is the angle of rotation produced by the triangular load. This example illustrates how we can use
superposition and integration to find deflections and angles of rotation produced by distributed loads of
almost any kind. If the integration cannot be performed easily by analytical means, numerical methods can
be used.
Moment-Area Method:
In this section we will describe another method for finding deflections and angles of rotation of beams.
Because the method is based upon two theorems related to the area of the bending-moment diagram, it is
called the moment-area method.
The assumptions used in deriving the two theorems are the same as those used in deriving the differential
equations of the deflection curve. Therefore, the moment-area method is valid only for linearly elastic beams
with small slopes.
First Moment-Area Theorem:
To derive the first theorem, consider a segment AB of the deflection curve of a beam in a region where the
curvature is positive (Fig. 9-22). Of course, the deflections and slopes shown in the figure are highly
exaggerated for clarity. At point A the tangent AA’ to the deflection curve is at an angle θA to the x axis, and at
point B the tangent BB’ is at an angle θB These two tangents meet at point C.
The angle between the tangents, denoted θB/A is equal to the difference between θB and θA:
Thus, the angle θB/A may be described as the angle to the tangent at B measured relative to, or with respect
to, the tangent at A. Note that the angles θA and θB which are the angles of rotation of the beam axis at points
A and B, respectively, are also equal to the slopes at those points, because in reality the slopes and angles are
very small quantities.
Next, consider two points m1 and m2 on the deflected axis of the beam. These points are a small distance ds
apart. The tangents to the deflection curve at these points are shown in the figure as lines mlpl and m2p2. The
normals to these tangents intersect at the center of curvature (not shown in the figure). The angle dθ
between the normals is given by the following equation:

in which ρ is the radius of curvature and dθ is measured in radians. Because the normals and the tangents
(mlpl and m2p2) are perpendicular, it follows that the angle between the tangents is also equal to dθ. For a
beam with small angles of rotation, we can replace ds with dx. Thus,
in which M is the bending moment and EI is the flexural rigidity of the beam.
The quantity Mdx/EI has a simple geometric interpretation. To see this, refer to Fig. where we have drawn
the M/EI diagram directly below the beam. At any point along the x axis, the height of this diagram is equal to
the bending moment M at that point divided by the flexural rigidity EI at that point. Thus, the M/EI diagram
has the same shape as the bending-moment diagram whenever EI is constant. The term M dx/EI is the area
of the shaded strip of width dx within the M/EI diagram.
(Note that since the curvature of the deflection curve in Fig. is positive, the bending moment M and the area
of the M/EI diagram are also positive.)
Let us now integrate dθ (Eq. 9-61) between points A and B of the deflection curve:
When evaluated, the integral on the left-hand side becomes θB - θA, which is equal to the angle θB/A between
the tangents at B and A. The integral on the right-hand side of Eq. (d) is equal to the area of the M/EI diagram
between points A and B. (Note that the area of the M/EI diagram is an algebraic quantity and may be positive
or negative, depending upon whether the bending moment is positive or negative.) Now we can write Eq. (d)
as follows:
This equation may be stated as a theorem:
First moment-area theorem: The angle θB/A between the tangents to the deflection curve at two points A
and B is equal to the area of the M/EI diagram between those points.
The sign conventions used in deriving the preceding theorem are as follows:
1. The angles θA and θB are positive when counterclockwise.
2. The angle θB/A between the tangents is positive when the angle θB is algebraically larger than the angle θA
Also, note that point B must be to the right of point A; that is, it must be further along the axis of the beam as
we move in the x direction.
3. The bending moment M is positive according to our usual sign convention; that is, M is positive when it
produces compression in the upper part of the beam.
4. The area of the M/EI diagram is given a positive or negative sign according to whether the bending
moment is positive or negative. If part of the bending-moment diagram is positive and part is negative, then
the corresponding parts of the M/EI diagram are given those same signs.
The preceding sign conventions for θA, θB, and θB/A are often ignored in practice because the directions of the
angles of rotation are usually obvious from an inspection of the beam and its loading. When this is the case,
we can simplify the calculations by ignoring signs and using only absolute values when applying the first
moment area theorem.

Second Moment-Area Theorem:
Now we turn to the second theorem, which is related primarily to deflections rather than to angles of
rotation. Consider again the deflection curve between points A and B. We draw the tangent at point A and
note that its intersection with a vertical line through point B is at point B1. The vertical distance between
points B and B1 is denoted tB/A in the figure. This distance is referred to as the tangential deviation of B with
respect to A. More precisely, the distance tB/A is the vertical deviation of point B on the deflection curve from
the tangent at point A. The tangential deviation is positive when point B is above the tangent at A.
To determine the tangential deviation, we again select two points m1 and m2 a small distance apart on the
deflection curve. The angle between the tangents at these two points is dθ, and the segment on line BB1
between these tangents is dt. Since the angles between the tangents and the x axis are actually very small, we
see that the vertical distance dt is equal to x1 dθ, where x1 is the horizontal distance from point B to the small
element m1m2. Since dθ = Mdx/EI , we obtain
The distance dt represents the contribution made by the bending of element m1m2 to the tangential
deviation tB/A. The expression x1Mdx/EI may be interpreted geometrically as the first moment of the area of
the shaded strip of width dx within the M/EI diagram. This first moment is evaluated with respect to a
vertical line through point B.
Integrating Eq. (e) between points A and B, we get
The integral on the left-hand side is equal to tB/A, that is, it is equal to the deviation of point B from the
tangent at A. The integral on the right-hand side represents the first moment with respect to point B of the
area of the M/EI diagram between A and B. Therefore, we can write Eq. (f) as follows:
= First Moment of Area of the M/EI diagram between points A and B, evaluated with respect to B.
This equation represents the second theorem:
Second moment-area theorem: The tangential deviation tB/A of point B from the tangent at point A is equal
to the first moment of the area of the M/EI diagram between A and B, evaluated with respect to B.
If the bending moment is positive, then the first moment of the M/EI diagram is also positive, provided point
B is to the right of point A. Under these conditions the tangential deviation tB/A is positive and point B is

above the tangent at A. If, as we move from A to B in the x direction, the area of the M/EI diagram is negative,
then the first moment is also negative and the tangential deviation is negative, which means that point B is
below the tangent at A.
The first moment of the area of the M/EI diagram can be obtained by taking the product of the area of the
diagram and the distance x from point B to the centroid C of the area. This procedure is usually more
convenient than integrating, because the M/EI diagram usually consists of familiar geometric figures such as
rectangles, triangles, and parabolic segments.
As a method of analysis, the moment-area method is feasible only for relatively simple kinds of beams.
Therefore, it is usually obvious whether the beam deflects upward or downward and whether an angle of
rotation is clockwise or counterclockwise. Consequently, it is seldom necessary to follow the formal (and
somewhat awkward) sign conventions described previously for the tangential deviation. Instead, we can
determine the directions by inspection and use only absolute values when applying the moment-area
theorems.
Strain Energy of Bending
Let us begin with a simple beam AB in pure bending under the action of two couples, each having a moment
M. The deflection curve is a nearly flat circular arc of constant curvature k = M/EI. The angle θ subtended by
this arc equals L/ρ, where L is the length of the beam and ρ is the radius of curvature.
Therefore,
This linear relationship between the moments M and the angle θ is shown graphically by line OA in Fig. As
the bending couples gradually increase in magnitude from zero to their maximum values, they perform work
W represented by the shaded area below line OA. This work, equal to the strain energy U stored in the beam,
is
By combining Eqs. (9-77) and (9-78), we can express the strain energy stored in a beam in pure bending in
either of the following forms:
The first of these equations expresses the strain energy in terms of the applied moments M, and the second
equation expresses it in terms of the angle θ. If the bending moment in a beam varies along its length
(nonuniform bending), then we may obtain the strain energy by applying Eqs. (9-79a) and (9-79b) to an
element of the beam and integrating along the length of the beam. The length of the element itself is dx and
the angle dθ between its side faces can be obtained from Eqs. (9-4) and (9-5), as follows:
Therefore, the strain energy dU of the element is given by either of the following equations:

Side view of an element of a beam subjected to bending moments M
By integrating the preceding equations throughout the length of a beam, we can express the strain energy
stored in a beam in either of the following forms:
Note that M is the bending moment in the beam and may vary as a function of x. We use the first equation
when the bending moment is known, and the second equation when the equation of the deflection curve is
known.
In the derivation of Eqs. (9-80a) and (9-80b), we considered only the effects of the bending moments. If
shear forces are also present, additional strain energy will be stored in the beam. However, the strain energy
of shear is relatively small (in comparison with the strain energy of bending) for beams in which the lengths
are much greater than the depths (say, L/d > 8). Therefore, in most beams the strain energy of shear may
safely be disregarded.
Castigliano’s Theorem:
Castigliano’s theorem provides a means for finding the deflections of a structure from the strain energy of
the structure. Consider a cantilever beam with a concentrated load P acting at the free end. The strain energy
of this beam is
Now take the derivative of this expression with respect to the load P:
We immediately recognize this result as the deflection δA at the free end A of the beam. Note especially that
the deflection δA corresponds to the load P itself. (Recall that a deflection corresponding to a concentrated
load is the deflection at the point where the concentrated load is applied. Furthermore, the deflection is in
the direction of the load.)
Thus, Eq. (b) shows that the derivative of the strain energy with respect to the load is equal to the
deflection corresponding to the load. Castigliano’s theorem is a generalized statement of this observation.
The important requirements are that the structure be linearly elastic and that the principle of superposition
be applicable. Also, note that the strain energy must be expressed as a function of the loads (and not as a
function of the displacements), a condition which is implied in the theorem itself, since the partial derivative
is taken with respect to a load. With these limitations in mind, we can state Castigliano’s theorem in general
terms as follows:

The partial derivative of the strain energy of a structure with respect to any load is equal to the
displacement corresponding to that load.
The strain energy of a linearly elastic structure is a quadratic function of the loads, and therefore the partial
derivatives and the displacements are linear functions of the loads. When using the terms load and
corresponding displacement in connection with Castigliano’s theorem, it is understood that these terms are
used in a generalized sense. The load Pi and corresponding displacement di may be a force and a
corresponding translation, or a couple and a corresponding rotation, or some other set of corresponding
quantities.
Application of Castigliano’s Theorem:
As an application of Castigliano’s theorem, let us consider a cantilever beam AB carrying a concentrated load
P and a couple of moment M0 acting at the free end. We wish to determine the vertical deflection δA and angle
of rotation θA at the end of the beam. Note that δA is the deflection corresponding to the load P, and θA is the
angle of rotation corresponding to the moment M0.
The first step in the analysis is to determine the strain energy of the beam. For that purpose, we write the
equation for the bending moment as follows:
in which x is the distance from the free end. The strain energy is found by substituting this expression for M
into Eq. (9-80a):
in which L is the length of the beam and EI is its flexural rigidity. Note that the strain energy is a quadratic
function of the loads P and M0. To obtain the vertical deflection δA at the end of the beam, we use
Castigliano’s theorem (Eq. 9-87) and take the partial derivative of the strain energy with respect to P:
In a similar manner, we can find the angle of rotation δA at the end of the beam by taking the partial
derivative with respect to M0:
Use of a Fictitious Load:
The only displacements that can be found from Castigliano’s theorem are those that correspond to loads
acting on the structure. If we wish to calculate a displacement at a point on a structure where there is no
load, then a fictitious load corresponding to the desired displacement must be applied to the structure. We
can then determine the displacement by evaluating the strain energy and taking the partial derivative with
respect to the fictitious load. The result is the displacement produced by the actual loads and the fictitious
load acting simultaneously. By setting the fictitious load equal to zero, we obtain the displacement produced
only by the actual loads.
To illustrate this concept, suppose we wish to find the vertical deflection δC at the midpoint C of the
cantilever beam shown.

Since the deflection δC is downward, the load corresponding to that deflection is downward vertical force
acting at the same point. Therefore, we must supply a fictitious load Q acting at point C in the downward
direction. Then we can use Castigliano’s theorem to determine the deflection (δC)0 at the midpoint of this
beam. From that deflection, we can obtain the deflection δC in the beam by setting Q equal to zero.
For the left-hand half of the beam (from point A to point C), the strain energy is
For the right-hand half, the strain energy is
which requires a very lengthy process of integration. Adding the strain energies for the two parts of the
beam, we obtain the strain energy for the entire beam:
The deflection at the midpoint of the beam shown in Fig. can now be obtained from Castigliano’s theorem:
This equation gives the deflection at point C produced by all three loads acting on the beam. To obtain the
deflection produced by the loads P and M0 only, we set the load Q equal to zero in the preceding equation.
The result is the deflection at the midpoint C for the beam with two loads:
Thus, the deflection in the original beam has been obtained. This method is sometimes called the dummy-
load method, because of the introduction of a fictitious, or dummy, load.

Differentiation under the Integral Sign:
Castigliano’s theorem used for determining beam deflections may lead to lengthy integrations, especially
when more than two loads act on the beam. The reason is clear—finding the strain energy requires the
integration of the square of the bending moment. For instance, if the bending moment expression has three
terms, its square may have as many as six terms, each of which must be integrated.
After the integrations are completed and the strain energy has been determined, we differentiate the strain
energy to obtain the deflections. However, we can bypass the step of finding the strain energy by
differentiating before integrating. This procedure does not eliminate the integrations, but it does make them
much simpler. To derive this method, we begin with the equation for the strain energy and apply
Castigliano’s theorem:
We will refer to this equation as the modified Castigliano’s theorem.
Let us begin with the beam shown in Fig. and recall that we wish to find the deflection and angle of rotation
at the free end. The bending moment and its derivatives are:
From Eq. (9-88) we obtain the deflection δA and angle of rotation θA:
These equations agree with the earlier results. However, the calculations are shorter than those performed
earlier, because we did not have to integrate the square of the bending moment. The advantages of
differentiating under the integral sign are even more apparent when there are more than two loads acting on
the structure.
We wish to determine the deflection δC at the midpoint C of the beam due to the loads P and M0. To do so, we
added a fictitious load Q at the midpoint. We then proceeded to find the deflection (δC)0 at the midpoint of
the beam when all three loads (P, M0, and Q) were acting.
Finally, we set Q = 0 to obtain the deflection δC due to P and M0 alone. The solution was time-consuming,
because the integrations were extremely long. However, if we use the modified theorem and differentiate
first, the calculations are much shorter. With all three loads acting (Fig. 9-39), the bending moments and
their derivatives are as follows:

Since Q is a fictitious load, and since we have already taken the partial derivatives, we can set Q equal to zero
before integrating and obtain the deflection δC due to the two loads P and M0 as follows:
The partial derivative that appears under the integral sign in Eq. (9-88) has a simple physical interpretation.
It represents the rate of change of the bending moment M with respect to the load Pi, that is, it is equal to the
bending moment M produced by a load Pi of unit value. This observation leads to a method of finding
deflections known as the unit-load method. Castigliano’s theorem also leads to a method of structural
analysis known as the flexibility method. However, it should be remembered that the theorem is not limited
to finding beam deflections—it applies to any kind of linearly elastic structure for which the principle of
superposition is valid.
Discontinuity Functions:
Discontinuity functions are utilized in a variety of engineering applications, including beam analysis,
electrical circuits, and heat transfer. These functions probably are the easiest to use and understand when
applied to beams, and therefore the study of mechanics of materials offers an excellent opportunity to
become familiar with them.
The unique feature of discontinuity functions is that they permit the writing of a discontinuous function by a
single expression, whereas the more conventional approach requires that a discontinuous function be
described by a series of expressions, one for each region in which the function is distinct.
For instance, if the loading on a beam consists of a mixture of concentrated and distributed loads, we can
write with discontinuity functions a single equation that applies throughout the entire length, whereas
ordinarily we must write separate equations for each segment of the beam between changes in loading. In a
similar manner, we can express shear forces, bending moments, slopes, and deflections of a beam by one
equation each, even though there may be several changes in the loads acting along the axis of the beam.
These results are achievable because the functions themselves are discontinuous; that is, they have different
values in different regions of the independent variable. In effect, these functions can pass through
discontinuities in a manner that is not possible with ordinary continuous functions. However, because they
differ significantly from the functions we are accustomed to, discontinuity functions must be used with care
and caution.
Two kinds of functions, called Macaulay functions and singularity functions, will be discussed in this
section. Although these functions have different definitions and properties, together they form a family of
discontinuity functions.
Macaulay Functions:
Macaulay functions are used to represent quantities that “begin” at some particular point on the x axis and
have the value zero to the left of that point. For instance, one of the Macaulay functions, denoted F1, is
defined as follows:
In this equation, x is the independent variable and a is the value of x at which the function “begins.” The
pointed brackets (or angle brackets) are the mathematical symbol for a discontinuity function. In the case
of the function F1, the pointed brackets (with the superscript 1) tell us that the function has the value zero
when x is less than or equal to a (that is, when the expression within the brackets is negative or zero) and a
value equal to x - a when x is greater than or equal to a. A graph of this function, called the unit ramp
function, is given in figure.

In general terms, the Macaulay functions are defined by the following expressions:
Another way to express this definition is the following: If the quantity x - a within the pointed brackets is
negative or zero, the Macaulay function has the value zero; if the function x - a is positive or zero, the
Macaulay function has the value obtained by replacing the pointed brackets with parentheses. The preceding
definition of the Macaulay functions holds for values of n equal to positive integers and zero. When n = 0,
note that the function takes on the following special values:
(9-98)
This function has a vertical “step” at the point of discontinuity x = a; thus, at x = a it has two values: zero and
one. The function F0, called the unit step function.
The Macaulay functions of higher degree can be expressed in terms of the unit step function, as follows:
This equation is easily confirmed by comparing Eqs. (9-97) and (9-98). Some of the basic algebraic
operations, such as addition, subtraction, and multiplication by a constant, can be performed on the
Macaulay functions. Illustrations of these elementary operations are given in Fig. 9-47. The important thing
to note from these examples is that a function y having different algebraic expressions for different regions
along the x axis can be written as a single equation through the use of Macaulay functions. The reader should
verify each of the graphs in Fig. 9-47 in order to become familiar with the construction of these expressions.
Fig. 9-47 Graphs of expressions involving Macaulay functions

Beam deflection gere

Recommended

Recommended

More Related Content

What's hot

What's hot (20)

Viewers also liked

Viewers also liked (20)

Similar to Beam deflection gere

Similar to Beam deflection gere (20)

More from Yatin Singh

More from Yatin Singh (14)

Recently uploaded

Recently uploaded (20)

Beam deflection gere