Integrals of Trig-Products-i

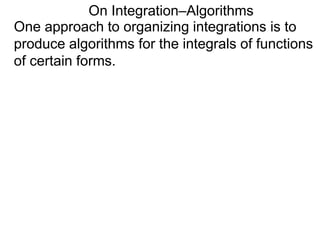
1. On Integration–Algorithms
One approach to organizing integrations is to
produce algorithms for the integrals of functions
of certain forms.

2. On Integration–Algorithms
One approach to organizing integrations is to
produce algorithms for the integrals of functions
of certain forms.
We present below examples of such an
approach by starting with the reduction formulas
for integrating trig-powers from the last section.

3. On Integration–Algorithms
One approach to organizing integrations is to
produce algorithms for the integrals of functions
of certain forms.
We present below examples of such an
approach by starting with the reduction formulas
for integrating trig-powers from the last section.
We will show the integration-algorithm for
functions of the forms:

4. On Integration–Algorithms
One approach to organizing integrations is to
produce algorithms for the integrals of functions
of certain forms.
We present below examples of such an
approach by starting with the reduction formulas
for integrating trig-powers from the last section.
We will show the integration-algorithm for
functions of the forms:
A. all products of trig–functions
B. all rational functions
C. algebraic functions that may be converted to
trig–products

5. Integrals of Trig. Products i

6. Integrals of Trig. Products i
In this section we organize the integrals of
products of trig-functions.

7. Integrals of Trig. Products i
In this section we organize the integrals of
products of trig-functions. Writing s, c, t and e
for sin(x), cos(x), tan(x) and sec(x), here are the
reduction formulas, obtained from integration by
parts from the last section:
∫sndx = + ∫sn–2dxn
–sn–1c
n
n–1
∫cndx = + ∫cn–2dxn
cn–1s
n
n–1

8. Integrals of Trig. Products i
In this section we organize the integrals of
products of trig-functions. Writing s, c, t and e
for sin(x), cos(x), tan(x) and sec(x), here are the
reduction formulas, obtained from integration by
parts from the last section:
∫sndx = + ∫sn–2dxn
–sn–1c
n
n–1
∫en(x)dx = + ∫en–2(x)dxn – 1
en–2(x)t(x)
n–1
n–2
∫cndx = + ∫cn–2dxn
cn–1s
n
n–1

9. Integrals of Trig. Products i
In this section we organize the integrals of
products of trig-functions. Writing s, c, t and e
for sin(x), cos(x), tan(x) and sec(x), here are the
reduction formulas, obtained from integration by
parts from the last section:
∫sndx = + ∫sn–2dxn
–sn–1c
n
n–1
∫tndx = – ∫tn–2dxn – 1
tn–1
∫en(x)dx = + ∫en–2(x)dxn – 1
en–2(x)t(x)
n–1
n–2
∫cndx = + ∫cn–2dxn
cn–1s
n
n–1
From change of variable, we have that:

10. These reduction formulas pass the calculation
of integrals of the powers of trig-functions of
degree n to degree n – 2.
Integrals of Trig. Products i

11. These reduction formulas pass the calculation
of integrals of the powers of trig-functions of
degree n to degree n – 2.
If n is a positive integer, repeated applications of
them reduce the integrals to the integrals of
degrees 0 or 1.
Integrals of Trig. Products i

12. These reduction formulas pass the calculation
of integrals of the powers of trig-functions of
degree n to degree n – 2.
If n is a positive integer, repeated applications of
them reduce the integrals to the integrals of
degrees 0 or 1.
If n is not a positive integer the reduction
formulas would expand indefinitely.
Integrals of Trig. Products i

13. These reduction formulas pass the calculation
of integrals of the powers of trig-functions of
degree n to degree n – 2.
If n is a positive integer, repeated applications of
them reduce the integrals to the integrals of
degrees 0 or 1.
If n is not a positive integer the reduction
formulas would expand indefinitely.
For n = 1, with the integration constant as 0,
we have that:
Integrals of Trig. Products i
∫s dx => –c
∫c dx => s

14. These reduction formulas pass the calculation
of integrals of the powers of trig-functions of
degree n to degree n – 2.
If n is a positive integer, repeated applications of
them reduce the integrals to the integrals of
degrees 0 or 1.
If n is not a positive integer the reduction
formulas would expand indefinitely.
For n = 1, with the integration constant as 0,
we have that:
Integrals of Trig. Products i
∫s dx => –c
∫c dx => s
∫ t dx => In |e|
∫ e dx => In |e + t|

15. Example A.
Integrals of Trig. Products i
a. ∫ t dx =
For simplicity,
we set all the
integration
constant = 0

16. Example A.
Integrals of Trig. Products i
a. ∫ t dx = ∫ dxs
c
For simplicity,
we set all the
integration
constant = 0

17. Example A.
Integrals of Trig. Products i
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx
For simplicity,
we set all the
integration
constant = 0

18. Example A.
Integrals of Trig. Products i
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0

19. Example A.
Integrals of Trig. Products i
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)

20. Example A.
Integrals of Trig. Products i
b. We pull a rabbit out of the hat to integrate
e(x) by multiplying to e(x).
∫ e dx =
e + t
e + t
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)

21. Example A.
Integrals of Trig. Products i
b. We pull a rabbit out of the hat to integrate
e(x) by multiplying to e(x).
∫ e dx = ∫ e dx
e + t
e + t
e + t
e + t
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)

22. Example A.
Integrals of Trig. Products i
b. We pull a rabbit out of the hat to integrate
e(x) by multiplying to e(x).
∫ e dx = ∫ e dx
e + t
e + t
e + t
e + t
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
= ∫ dxe2 + et
e + t
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)

23. Example A.
Integrals of Trig. Products i
b. We pull a rabbit out of the hat to integrate
e(x) by multiplying to e(x).
∫ e dx = ∫ e dx
e + t
e + t
e + t
e + t
Noting that
(e + t)’ = et + e2
so set u = e + t
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
= ∫ dxe2 + et
e + t
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)

24. Example A.
Integrals of Trig. Products i
b. We pull a rabbit out of the hat to integrate
e(x) by multiplying to e(x).
∫ e dx = ∫ e dx
e + t
e + t
e + t
e + t
Noting that
(e + t)’ = et + e2
so set u = e + t
= ∫ du1
u
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
= ∫ dxe2 + et
e + t
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)

25. Example A.
Integrals of Trig. Products i
b. We pull a rabbit out of the hat to integrate
e(x) by multiplying to e(x).
∫ e dx = ∫ e dx
e + t
e + t
e + t
e + t
Noting that
(e + t)’ = et + e2
so set u = e + t
= ∫ du = In(lul) = In(l e + t l)1
u
a. ∫ t dx = ∫ dxs
c
set u = c so
–du/s = dx
=> – ln(lul) = ln(l 1/u l)
= ln(l 1/c l) = ln(l e(x) l)
= ∫ s
u
–du
s = ∫ u
–du
For simplicity,
we set all the
integration
constant = 0
= ∫ dxe2 + et
e + t

26. Integrals of Trig. Products i
For n = 2, the direct calculation of the integrals
∫ s2 dx, ∫ c2 dx, ∫ t2 dx and ∫ e2 dx
require the following square–trig–identities
from the cosine double angle formulas.

27. Integrals of Trig. Products i
For n = 2, the direct calculation of the integrals
∫ s2 dx, ∫ c2 dx, ∫ t2 dx and ∫ e2 dx
require the following square–trig–identities
from the cosine double angle formulas.
c(2x) = c2(x) – s2(x)
= 2c2(x) – 1
= 1 – 2s2(x)

28. Integrals of Trig. Products i
For n = 2, the direct calculation of the integrals
∫ s2 dx, ∫ c2 dx, ∫ t2 dx and ∫ e2 dx
require the following square–trig–identities
from the cosine double angle formulas.
c(2x) = c2(x) – s2(x)
= 2c2(x) – 1
= 1 – 2s2(x)
c2(x) =
1 + c(2x)
2
s2(x) = 2
1 – c(2x)
square–trig–identities

29. Integrals of Trig. Products i
For n = 2, the direct calculation of the integrals
∫ s2 dx, ∫ c2 dx, ∫ t2 dx and ∫ e2 dx
require the following square–trig–identities
from the cosine double angle formulas.
c(2x) = c2(x) – s2(x)
= 2c2(x) – 1
= 1 – 2s2(x)
c2(x) =
1 + c(2x)
2
s2(x) = 2
1 – c(2x)
square–trig–identities
s2(x) + c2(x) = 1
t2(x) + 1 = e2(x)
1 + cot2(x) = csc2(x)
square–sum–identities
+ +
+

30. Integrals of Trig. Products i
b. ∫ s2 (x) dx
c. ∫ e2 (x) dx
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx
For simplicity,
we set all the
integration
constant = 0

31. Integrals of Trig. Products i
b. ∫ s2 (x) dx
c. ∫ e2 (x) dx
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
For simplicity,
we set all the
integration
constant = 0

32. Integrals of Trig. Products i
b. ∫ s2 (x) dx
c. ∫ e2 (x) dx
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
For simplicity,
we set all the
integration
constant = 0

33. Integrals of Trig. Products i
b. ∫ s2 (x) dx
c. ∫ e2 (x) dx
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
= x/2 + s(2x)/4
For simplicity,
we set all the
integration
constant = 0

34. Integrals of Trig. Products i
b. ∫ s2 (x) dx
= ∫ 1 – c2(x) dx
c. ∫ e2 (x) dx
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
= x/2 + s(2x)/4
For simplicity,
we set all the
integration
constant = 0

35. Integrals of Trig. Products i
b. ∫ s2 (x) dx
= ∫ 1 – c2(x) dx
=> x – [x/2 + s(2x)/4]
= x/2 –s(2x)/4
c. ∫ e2 (x) dx
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
= x/2 + s(2x)/4
For simplicity,
we set all the
integration
constant = 0

36. Integrals of Trig. Products i
b. ∫ s2 (x) dx
= ∫ 1 – c2(x) dx
=> x – [x/2 + s(2x)/4]
= x/2 –s(2x)/4
c. ∫ e2 (x) dx = t
d. ∫ t2(x) dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
= x/2 + s(2x)/4
For simplicity,
we set all the
integration
constant = 0

37. Integrals of Trig. Products i
b. ∫ s2 (x) dx
= ∫ 1 – c2(x) dx
=> x – [x/2 + s(2x)/4]
= x/2 –s(2x)/4
c. ∫ e2 (x) dx = t
d. ∫ t2(x) dx
= ∫ e2(x) – 1 dx
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
= x/2 + s(2x)/4
For simplicity,
we set all the
integration
constant = 0

38. Integrals of Trig. Products i
b. ∫ s2 (x) dx
= ∫ 1 – c2(x) dx
=> x – [x/2 + s(2x)/4]
= x/2 –s(2x)/4
c. ∫ e2 (x) dx = t
d. ∫ t2(x) dx
= ∫ e2(x) – 1 dx
=> t – x
Example B.
a. ∫ c2 (x) dx = ½ ∫ 1 + c(2x) dx
=> ½ (x + s(2x)/2)
= x/2 + s(2x)/4
For simplicity,
we set all the
integration
constant = 0

39. Integrals of Trig. Products i
We summarize the results here.
∫ c2 (x) dx => ½ x + ¼ s(2x)
∫ s2 (x) dx => ½ x – ¼ s(2x)
∫ e2 (x) dx => t
∫ t2(x) dx => t – x
∫ c(x) dx => – s(x)
∫ s(x) dx => c(x)
∫ e (x) dx => In |t(x) + e(x)|
∫ t (x) dx => In |e(x)|
HW. Integrate cot(x), cot2(x), csc(x) and csc2(x).

40. Integrals of Trig. Products i
Since all products of trig–functions may be
expressed as sMcN with M and N integers,
we summarize the calculation of ∫ sMcN dx here.

41. Integrals of Trig. Products i
Since all products of trig–functions may be
expressed as sMcN with M and N integers,
we summarize the calculation of ∫ sMcN dx here.
The basic idea is to use the trig–identities
s2 = 1 – c2 or c2 = 1 – s2 to change the
integrands into powers of sine or cosine as
much as possible.

42. Integrals of Trig. Products i
Since all products of trig–functions may be
expressed as sMcN with M and N integers,
we summarize the calculation of ∫ sMcN dx here.
The basic idea is to use the trig–identities
s2 = 1 – c2 or c2 = 1 – s2 to change the
integrands into powers of sine or cosine as
much as possible. There are three groups:

43. Integrals of Trig. Products i
Since all products of trig–functions may be
expressed as sMcN with M and N integers,
we summarize the calculation of ∫ sMcN dx here.
I. ∫ sMcN dx
II. ∫ dx or ∫ dxsM
cN
cM
sN
lII. ∫ dxsMcN
1
Letting M and N be
positive integers,
we want to integrate:
The basic idea is to use the trig–identities
s2 = 1 – c2 or c2 = 1 – s2 to change the
integrands into powers of sine or cosine as
much as possible. There are three groups:

44. Integrals of Trig. Products i
Since all products of trig–functions may be
expressed as sMcN with M and N integers,
we summarize the calculation of ∫ sMcN dx here.
I. ∫ sMcN dx
II. ∫ dx or ∫ dxsM
cN
cM
sN
lII. ∫ dxsMcN
1
letting M and N be
positive integers,
we want to integrate:
Let’s look at each
case below.
The basic idea is to use the trig–identities
s2 = 1 – c2 or c2 = 1 – s2 to change the
integrands into powers of sine or cosine as
much as possible. There are three groups:

45. Let M and N be two positive integers,
we are to integrate:
I. ∫ sMcN dx
Integrals of Trig. Products i

46. Let M and N be two positive integers,
we are to integrate:
I. ∫ sMcN dx
Integrals of Trig. Products i
a. ∫s3 c3 dx
Example C. (M is odd.)

47. Let M and N be two positive integers,
we are to integrate:
I. ∫ sMcN dx
Integrals of Trig. Products i
Because of the symmetry of the sine and cosine
and their derivatives, we would base our
decisions for the examples on the factor sM,
specifically on whether M is odd or even.
a. ∫s3 c3 dx
Example C. (M is odd.)

48. Let M and N be two positive integers,
we are to integrate:
I. ∫ sMcN dx
Integrals of Trig. Products i
a. ∫s3 c3 dx
Example C. (M is odd.)
Because of the symmetry of the sine and cosine
and their derivatives, we would base our
decisions for the examples on the factor sM,
specifically on whether M is odd or even.

49. Let M and N be two positive integers,
we are to integrate:
I. ∫ sMcN dx
Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the odd power function to the other
function as much as possible. Then use the
substitution method.
Example C. (M is odd.)
Because of the symmetry of the sine and cosine
and their derivatives, we would base our
decisions for the examples on the factor sM,
specifically on whether M is odd or even.

50. Integrals of Trig. Products i
a. ∫s3 c3 dx
Example C.(M is odd.) We set all integration
constants to be 0.

51. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
Example C.(M is odd.) We set all integration
constants to be 0.

52. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
= ∫s(c3 – c5)dx
Example C.(M is odd.) We set all integration
constants to be 0.

53. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
= ∫s(c3 – c5)dx using the sub-method
set u = c(x)
so –du/s(x) = dx
Example C.(M is odd.) We set all integration
constants to be 0.

54. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
= ∫s(c3 – c5)dx
= ∫u5 – u3du
using the sub-method
set u = c(x)
so –du/s(x) = dx
Example C.(M is odd.) We set all integration
constants to be 0.

55. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
= ∫s(c3 – c5)dx
= ∫u5 – u3du
u6/6 – u4/4
= c6/6 – c4/4
using the sub-method
set u = c(x)
so –du/s(x) = dx
Example C.(M is odd.) We set all integration
constants to be 0.

56. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
= ∫s(c3 – c5)dx
= ∫u5 – u3du
u6/6 – u4/4
= c6/6 – c4/4
using the sub-method
set u = c(x)
so –du/s(x) = dx
Example C.(M is odd.)
b. ∫s2 c3 dx (M is even.)
We set all integration
constants to be 0.

57. Integrals of Trig. Products i
a. ∫s3 c3 dx
Convert the s3 to c as much as possible.
= ∫s(1 – c2) c3dx
= ∫s(c3 – c5)dx
= ∫u5 – u3du
u6/6 – u4/4
= c6/6 – c4/4
using the sub-method
set u = c(x)
so –du/s(x) = dx
Example C.(M is odd.)
b. ∫s2 c3 dx (M is even.)
Convert the even power function to the other
function completely, continue with the reduction
formula or using the sub-method if possible.
We set all integration
constants to be 0.

58. Example D. (M is even.) b. ∫s2 c3 dx
Integrals of Trig. Products i

59. Example D. (M is even.) b. ∫s2 c3 dx
Convert the even power s2 to c.
= ∫(1 – c2) c3dx = ∫ c3 – c5 dx
Integrals of Trig. Products i

60. Example D. (M is even.) b. ∫s2 c3 dx
Convert the even power s2 to c.
= ∫(1 – c2) c3dx = ∫ c3 – c5 dx
we may use the reduction formula, or use the
sub-method in this problem.
Integrals of Trig. Products i

61. Example D. (M is even.) b. ∫s2 c3 dx
Convert the even power s2 to c.
= ∫(1 – c2) c3dx = ∫ c3 – c5 dx
we may use the reduction formula, or use the
sub-method in this problem.
∫ c3 – c5 dx
= ∫ c(c2 – c4)dx
= ∫ c[(1 – s2) – (1 – s2)2]dx
We may use the easier
sub–method here
because all cosine
powers are odd.
Integrals of Trig. Products i

62. Example D. (M is even.) b. ∫s2 c3 dx
Convert the even power s2 to c.
= ∫(1 – c2) c3dx = ∫ c3 – c5 dx
we may use the reduction formula, or use the
sub-method in this problem.
∫ c3 – c5 dx
= ∫ c(c2 – c4)dx
= ∫ c[(1 – s2) – (1 – s2)2]dx
= ∫ c[s2 – s4]dx using the sub–method
set u = s(x) so du/c(x) = dx
We may use the easier
sub–method here
because all cosine
powers are odd.
Integrals of Trig. Products i

63. Example D. (M is even.) b. ∫s2 c3 dx
Convert the even power s2 to c.
= ∫(1 – c2) c3dx = ∫ c3 – c5 dx
we may use the reduction formula, or use the
sub-method in this problem.
∫ c3 – c5 dx
= ∫ c(c2 – c4)dx
= ∫ c[(1 – s2) – (1 – s2)2]dx
= ∫ c[s2 – s4]dx
= ∫ u2 – u4du
using the sub–method
set u = s(x) so du/c(x) = dx
We may use the easier
sub–method here
because all cosine
powers are odd.
Integrals of Trig. Products i

64. Example D. (M is even.) b. ∫s2 c3 dx
Convert the even power s2 to c.
= ∫(1 – c2) c3dx = ∫ c3 – c5 dx
we may use the reduction formula, or use the
sub-method in this problem.
∫ c3 – c5 dx
= ∫ c(c2 – c4)dx
= ∫ c[(1 – s2) – (1 – s2)2]dx
= ∫ c[s2 – s4]dx
= ∫ u2 – u4du
=> u3/3 – u5/5
= s3/3 – s5/5
using the sub–method
set u = s(x) so du/c(x) = dx
We may use the easier
sub–method here
because all cosine
powers are odd.
Integrals of Trig. Products i

65. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i

66. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i
a. (M is even)
b. (M is odd)

67. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i
a. (M is even)
If M = 2K, then sMcN = (1 – c2)KcN = P(c) where
P(c) is a polynomial in cosine.
b. (M is odd)

68. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i
a. (M is even)
If M = 2K, then sMcN = (1 – c2)KcN = P(c) where
P(c) is a polynomial in cosine. We may calculate
∫sMcN dx = ∫ P(c) dx with the reduction-formula.
b. (M is odd)

69. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i
a. (M is even)
If M = 2K, then sMcN = (1 – c2)KcN = P(c) where
P(c) is a polynomial in cosine. We may calculate
∫sMcN dx = ∫ P(c) dx with the reduction-formula.
b. (M is odd)
If M is odd and M = 2K + 1,
then sMcN = s(1 – c2)KcN = sP(c)

70. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i
a. (M is even)
If M = 2K, then sMcN = (1 – c2)KcN = P(c) where
P(c) is a polynomial in cosine. We may calculate
∫sMcN dx = ∫ P(c) dx with the reduction-formula.
b. (M is odd)
If M is odd and that M = 2K + 1,
then sMcN = s(1 – c2)KcN = sP(c) so
∫sMcN dx = ∫sP(c) dx.

71. We summarize the method for finding ∫sMcN dx.
The method may also apply to the power N.
Integrals of Trig. Products i
a. (M is even)
If M = 2K, then sMcN = (1 – c2)KcN = P(c) where
P(c) is a polynomial in cosine. We may calculate
∫sMcN dx = ∫ P(c) dx with the reduction-formula.
b. (M is odd)
If M is odd and that M = 2K + 1,
then sMcN = s(1 – c2)KcN = sP(c) so
∫sMcN dx = ∫sP(c) dx. Using the sub-method,
set u = c(x), then ∫sP(c) dx = ∫P(u) du,
an integral of a polynomial in u with respect to u.

72. Integrals of Trig. Products i
∫ dx orsM
cN
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN

73. Integrals of Trig. Products i
∫ dx orsM
cN
a. (M is even)
b. (M is odd)
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN

74. Integrals of Trig. Products i
∫ dx orsM
cN
a. (M is even) If M is even with M = 2K,
then sM/cN = s2K/cN
b. (M is odd)
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN

75. Integrals of Trig. Products i
∫ dx orsM
cN
a. (M is even) If M is even with M = 2K,
then sM/cN = s2K/cN = (1 – c2)K/cN = P(c)/cN,
which is a polynomial in cosine and secant that
may be integrated using the reduction-formula.
b. (M is odd)
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN

76. Integrals of Trig. Products i
∫ dx orsM
cN
a. (M is even) If M is even with M = 2K,
then sM/cN = s2K/cN = (1 – c2)K/cN = P(c)/cN,
which is a polynomial in cosine and secant that
may be integrated using the reduction-formula.
b. (M is odd) If M is odd and M = 2K + 1,
then sM/cN = s2K+1/cN = s(1 – c2)K/cN = sP(c)/cN.
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN

77. Integrals of Trig. Products i
∫ dx orsM
cN
a. (M is even) If M is even with M = 2K,
then sM/cN = s2K/cN = (1 – c2)K/cN = P(c)/cN,
which is a polynomial in cosine and secant that
may be integrated using the reduction-formula.
b. (M is odd) If M is odd and that M = 2K + 1,
then sM/cN = s2K+1/cN = s(1 – c2)K/cN = sP(c)/cN.
Using the substitution method, set u = c(x),
then ∫ sP(c)/cN dx = ∫P(u)/uN du which is just
the integral of a polynomial in u and 1/u.
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN

78. Integrals of Trig. Products i
∫ dx orsM
cN
a. (M is even) If M is even with M = 2K,
then sM/cN = s2K/cN = (1 – c2)K/cN = P(c)/cN,
which is a polynomial in cosine and secant that
may be integrated using the reduction-formula.
b. (M is odd) If M is odd and that M = 2K + 1,
then sM/cN = s2K+1/cN = s(1 – c2)K/cN = sP(c)/cN.
Using the substitution method, set u = c(x),
then ∫ sP(c)/cN dx = ∫P(u)/uN du which is just
the integral of a polynomial in u and 1/u.
By observing the power of the numerator the
same procedure also works for ∫ dx.sM
cN
(This method also works if the numerator is cN.)

79. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Example E.
b. (M is even.) ∫s2/c3 dx
We set all integration
constants to be 0.

80. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
Example E.
b. (M is even.) ∫s2/c3 dx
We set all integration
constants to be 0.

81. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
∫s3/c3 dx
= ∫s(1 – c2)/c3dx
Example E.
b. (M is even.) ∫s2/c3 dx
We set all integration
constants to be 0.
using the sub–method
set u = c(x) so –du/s(x) = dx

82. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
∫s3/c3 dx
= ∫s(1 – c2)/c3dx
= ∫(u2 – 1)/u3du
Example E.
b. (M is even.) ∫s2/c3 dx
We set all integration
constants to be 0.
using the sub–method
set u = c(x) so –du/s(x) = dx

83. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
∫s3/c3 dx
= ∫s(1 – c2)/c3dx
= ∫(u2 – 1)/u3du
In(lul) + u–2/2 = In(lcl)+ c–2/2
Example E.
b. (M is even.) ∫s2/c3 dx
We set all integration
constants to be 0.
using the sub–method
set u = c(x) so –du/s(x) = dx

84. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
∫s3/c3 dx
= ∫s(1 – c2)/c3dx
= ∫(u2 – 1)/u3du
In(lul) + u–2/2 = In(lcl)+ c–2/2
Example E.
b. (M is even.) ∫s2/c3 dx
Convert s2 to c.
We set all integration
constants to be 0.
using the sub–method
set u = c(x) so –du/s(x) = dx

85. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
∫s3/c3 dx
= ∫s(1 – c2)/c3dx
= ∫(u2 – 1)/u3du
In(lul) + u–2/2 = In(lcl)+ c–2/2
Example E.
b. (M is even.) ∫s2/c3 dx
Convert s2 to c.
∫s2/c3 dx = ∫(1 – c2)/c3dx
We set all integration
constants to be 0.
using the sub–method
set u = c(x) so –du/s(x) = dx

86. Integrals of Trig. Products i
a. (M is odd.) ∫s3/c3 dx
Convert the s3 to c as much as possible.
∫s3/c3 dx
= ∫s(1 – c2)/c3dx
= ∫(u2 – 1)/u3du
In(lul) + u–2/2 = In(lcl)+ c–2/2
Example E.
b. (M is even.) ∫s2/c3 dx
Convert s2 to c.
∫s2/c3 dx = ∫(1 – c2)/c3dx
= ∫1/c3 – 1/c dx = ∫e3 – e dx which may be
computed with the reduction formula.
We set all integration
constants to be 0.
using the sub–method
set u = c(x) so –du/s(x) = dx

87. Integrals of Trig. Products i
For the integral of fractions of trig–powers
of the type:
lII. ∫ dxsMcN
1
we need to know how to integrate rational
functions which is the next topic.