![Prolog
What you’re looking at is the first direct observation of an atom’s electron orbital — an atom’s actual wave
function! Up until this point, scientists have never been able to actually observe the wave function. Trying
to catch a glimpse of an atom’s exact position or the momentum of its lone electron has been like trying to
catch a swarm of flies with one hand. [c.f. Phys. Rev. Lett. 110, 213001 (2017)]
2](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-2-320.jpg)
![Photoionization microscopy can directly obtain the nodal structure of the electronic orbital of a Hydrogen
atom placed in a static electric field. In the experiment, the Hydrogen atom is placed in the electric field E
and is excited by laser pulses. The ionized electron escapes from the atom and follows a particular tra-
jectory to the detector that is perpendicular to the field itself. Given that there are many such trajectories
that reach the same point on the detector, interference patterns can be observed […]. The interference
pattern directly reflects the nodal structure of the wavefunction. [c.f. Phys. Rev. Lett. 110, 213001 (2017)]
Calculated
Measured
3](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-3-320.jpg)
![with mo≡me the rest mass of the electron. By replacing E′ by E (non-relativistic energy);
eφ by −V,and p by −ih∇∇∇∇, the above equation becomes the static Schrödinger equation:
For an electron in a static field whose potential is ϕ, the Dirac equation (i.e., (E′+eϕ)ψ
={(1/2mo)[p+(e/c)A]2 +SMM[µS] Interaction−RE[p]−DT[p2]−SO[L••••S] Interaction}ψ – c.f.,
PART IV) without the higher-order relativistic corrections, simplifies and reduces to:
2017
MRT
ψψφ
o
2
2
)(
m
eE
p
=+′
in which the square of the angular momentum vector L is:
or, in spherical coordinates:
+=
+∇−= 22
e
22
e
2
)()()(
2
)( cmcEV
m
E prr ψψ
h
),,(),,()(
2
),,(
2 2
2
2
e
2
2
2
ϕθψϕθψϕθψ rrVE
rm
r
rrr
r L=−+
∂
∂
+
∂
∂
h
2
2
2
2
sin
1
sin
sin
1
ϕθθ
θ
θθ ∂
∂
+
∂
∂
∂
∂
=L
We recognize here the L2 operator from our discussion on spherical harmonics.
We will now consider a Hydrogen atom consisting of a single proton (Z=1) at its center
and an electron surrounding it. For hydrogen-like atoms (with Z>1), the reduced mass µ
=memp/(me +mp) should be used but can be neglected for this purpose of trivial illustration.
9](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-9-320.jpg)
(
2
22
e
2
2
ϕθλϕθ
λ
YY
rP
r
rPrVE
m
rd
rPd
=
=−+
L
h
lllll ,1,...,1, ++−−≡m
Substituting these into the equation above for P(r), we obtain the Radial Equation:
0)(
)1(
)]([
2
22
e
2
2
=
+
+−+ rP
r
rVE
m
rd
d ll
h
The equation r2[∂2/∂r2 +(2/r)∂/∂r]ψ +(2me r2/h2)(E−V )ψ =L2ψ above separates into:
),()(
1
)()()(),()(),,( ϕθϕθϕθϕθψ l
ll
l
l lllllll
m
nmmn
m
nmn YrP
r
rRYrRr =ΦΘ==
10](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-10-320.jpg)
![has solutions P(r)=exp(±ar) where a=√[−(2me/h2)E].
Solutions to this equation may be obtained by first considering the behavior at large r.
In the asymptotic region r →∞:
2017
MRT
)(e)(e)( o2
rfrfrP arra −−
==
where f (r) is a function to be determined by the Radial Equation and the boundary
conditions.
0)(
2)(
2
e
2
2
=+ rPE
m
rd
rPd
h
If E<0, exp(+ar)→∞ as r →∞. Since this violates (i.e., the ‘mathematical’ fact that the
exponential blows up at infinity!) the conditions (i.e., that it does not!) that the wave
function must be finite everywhere, it is not an acceptable solution (i.e.,itis not physically
possible!) On the other hand, exp(−ar)→0 as r →∞; it is therefore a possible solution.
If E >0, either sign in the exponent will satisfy the boundary conditions. We concentrate
on the case E<0, that is, the bound states of the atom.
The asymptotic behavior suggests that solutions to the Radial Equation be sought in
the form:
In the following slides, we will make use of the Bohr Radius (N.B., α =e2/hc=1/137):
11
( ) ( )MKSmorCGScm 11
2
e
2
o
o
8
e
2
e
2
o 1029.5
επ4
1052.0 −−
×==×===
em
a
cmem
a
hhh
α](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-11-320.jpg)
![0
5
10
ElectronVolt(eV)
13.60
n
1
2
3
4
5
∞ s p d f
LymanSeries
0
−5
−10
−13.60
The first few energy levels of the Hydrogen
atom – without fine structure (i.e. corrections
due to nuclear spin angular momentum I ) or
using the reduced mass µ instead of me.
The energy levels of the Hydrogen atom are given by the
Balmer formula:
The Rydberg constant is given by:
and it is related to the ‘Ry’ energy unit by:
eV605.137.3169,7310
2
2
2
e2
1
==
= −
∞
1
cm
c
e
cmR
h
Ry1eV(12)13.6056923 ≡
Ry
−−= ∞ 2
1
2
2
2 11
nn
ZREn
( )...,4,3,2,1
1
2
)(
2
2
22
22
e
=−=−= n
n
Z
n
Zem
En Ry
h
If an electron changes from one state to another, there will be a
corresponding change in energy of the system:
These transitions will in general be accompanied by an emission
or absorption of electromagnetic radiation (c.f., Appendix: Higher
Order Electromagnetic Interactions). For instance, if n1 =1, then one
gets the Lyman series, in which n2 can take on values of 2, 3, 4, ….
Recall also that the electron volt [eV] is a unit of energy equal to approximately
1.602×10−19 J [Joule] and by definition it is equal to the amount of kinetic energy gained
by a single unbound electron when it accelerates through an electric potential difference
of one volt. Thus it is 1 Volt (1 Joule per Coulomb) multiplied by the electron charge (1e,
or 1.602×10−19 C [Coulomb]).Therefore,one electron volt is equal to: 1 eV =1.602×10−19 J.
2017
MRT
19](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-19-320.jpg)
,(),( 2* lll
lll =
Finally, the probability of finding an electron between ϕ and ϕ +dϕ is simply
proportional to dϕ.
20](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-20-320.jpg)
![Plot forY1
0,R10,and the probability densityψ100
∗ψ100=|ψ100|2 of the orbital [ yellow(+)/ blue
(−) on black] which is the probability of finding an electron around the atom’s nucleus.
2017
MRT
Plots for Y1
0 , R20, & |ψ200|2, Y1
0 , R21, & |ψ210|2, and for Y1
1 , R21, & |ψ211|2.
+½
−½
+½ −½
The maximum number
Nn of electrons in a
shell characterized by
principle number n is
given by twice (i.e. due
to spin) the number of
orbital stateswiththatn:
...,18,8,22
)12(2
2
1
0
==
+= ∑
−
=
n
N
n
n
l
l
21
+
−](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-21-320.jpg)
![Average values of various powers of r are often needed in computation; these are
defined by the expectation value:
2017
MRT
Expectation values 〈rk 〉 of rk for several values of k are given in the Table below.
∫∫
∞
+
∞
==
0
22
0
2
)()( drrRrdrrPrr n
k
n
kk
ll
〈rk〉
1
2
3
4
−1
−2
−3
−4
)]1(315[
2
2
2
2
2
o
+−+ lln
n
Z
a
k
)]1(3[
2
2o
+− lln
Z
a
)]1()1)(2(3)1)(2(30)1(35[
8
222
2
3
2
o
−+++−+−− llllllnnn
n
Z
a
]12)1033)(1(5)322(3563[
8
2224
4
4
4
o
+−+++−+− llllllnn
n
Z
a
2
o
1
na
Z
)½(
1
32
o
2
+lna
Z
lll )½)(1(
1
33
o
3
++na
Z
)½)(½)(1)((2
)1(3
2
35
2
4
o
4
−+++
+−
llll
ll
n
n
a
Z
24](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-24-320.jpg)
![For the Hamiltonian H=p2/2me −(1/4πεo)Ze2/r the complete solution to the Schrödinger
equation Hψ =Eψ for the bound states consists of the orbitals ψnlml
(r,θ,ϕ) together with
the energy eigenvalues En =−Z2/n2. However, the Schrödinger equation:
2017
MRT
with the Hamiltonian (see the Appendix – Higher Order Electromagnetic Interactions):
pAAp ××××∇∇∇∇ΣΣΣΣ∇∇∇∇∇∇∇∇××××∇∇∇∇ΣΣΣΣ ϕϕ •−•−−•+
+= 22
e
22
e
2
23
e
4
e
2
e 48822
1
cm
e
cm
e
cm
p
cm
e
c
e
m
H
hhh
ψψψϕ )()( Orbit-SpinDarwinicRelativistnInteractioo HHHHHHeE ++++==+′
contains additional terms (e.g., Unperturbed, Interaction, Relativistic, Darwin and Spin-
Orbit) which all have an effect on the eigenfunctions and eigenvalues of the system.
which identify the system as a particle with spin s=½.
±=±+=±
±±=±
222
¾)1½(½
½
hh
h
S
zS
Examination of the Hamiltonian above reveals the presence of terms containing the
operator ΣΣΣΣ whose components are the Pauli matrices, σσσσ =[σ1,σ2,σ3] (where σ1
REAL/SYM =
+1, σ2
COMPLEX/ANTI = mi, and σ3
REAL/DIAG
PARITY =±1). According to our previous discussion,
S =½hΣΣΣΣ where the rectangular components of S are angular momentum operators and:
Thus, the appearance of ΣΣΣΣ in the Hamiltonian indicates that the wave function of
the system must include a spin eigenfunction.
25](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-25-320.jpg)
![In the spin-½ [in units of h] case, we saw earlier that:
2017
MRT
=
=−=−
=
=+=+
=
−
+
χ
χ
1
0
½,½
0
1
½,½
, sms
The one-electron spin function is:
−=
+=
==
−
+
½
½
)(
s
s
sm
m
m
ms
for
for
χ
χ
ξξ
whereas the (one-electron) spin orbital is:
)()(),,,(),,( ssmmn mmmnr s
ξrξ ψψϕθψ == ll ll
It shall be understood that an integral involving spin orbitals implies a spatial integration
as well as a summation over spin coordinates. Also, we now have a degeneracy of 2n2
associated with the addition of spin eigenfunctionψnlmlms
(r,θ,ϕ,±½) andweuse |l,s;ml ,ms 〉
to identify the angular and spin parts of the wave function. Degenerate eigenfunctions
may be combined linearly to form other sets in a coupled representation of degenerate
eigenfunctions using the Clebsch-Gordan coefficients Cj
mlms
=〈l,s;ml,ms |l,s; j,mj 〉:
∑∑ ==
s
s
s mm
s
j
mm
mm
jssj mmsmjsmmsmmsmjs
l
l
l
lll lllll ,;,,;,,;,,;,,;, C
26](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-26-320.jpg)
![To see how this works out, let:
2017
MRT
or:
)( 222
2
1
SLJ −−=•SL
∫∫
∞∞
====
0
2
0
2
)()()()(,)(,)( rdrPrrdrrRrnrnr nnn lll ll ξξξξξ
3322
e
22
3322
e
22
1
2
,
1
,
2
)(
rrcm
eZ
n
r
n
rcm
eZ
r
h
ll
h
==ξ
Using the coupled representation:
The Hamiltonian HSO =ξ(r)L•S also contain the radial function ξ(r). So, to obtain the
energy corrections it is also necessary to evaluate the expectation value of ξ(r):
From the Table for 〈rk 〉 (with k=−3):
SLSLJSLJ •++== 2222
and++++
jjss
jjjj
ssjj
mjsSLJmjsmjsmjs
′′′+−+−+=
−−′′′′=•′′′′
δδδ llll
llll
)]1()1()1([
,;,,;,,;,,;,
2
1
222
2
1SL
In hydrogen, ξ(r) is given by ξ(r)=Ze2h2/2me
2c2r3 in which case:
lll )½)(1(
11
23
o
3
3
++
=
na
Z
r
31](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-31-320.jpg)
![From the relation 〈l′,s′; j′,m′j|L•S|l,s;j,mj 〉=½[ j( j+1)−l(l+1)−s(s+1)]δl′lδs′sδj′j it is seen
that the matrix elements vanish unless:
These are the selection rules for spin-orbit coupling. Also, these are also valid:
0)(0 =+∆=∆=∆=∆ sj mmmj ll and
The Table below lists values of the 〈1,½; m′l,m′s|L•S|1,½;ml,ms 〉 matrix elements for p
states of ξnl= 〈n,l|ξ(r)|n,l〉 (and shortened to |ml ,ms 〉 since l=1 and s =½ for all states).
½,1−
2017
MRT
½,1
½,1 ½,0 ½,0 − ½,1− ½,1−−
½,1 −
½,0
½,0 −
½,1−
½,1 −−
2
1
2
1
−
2
1
2
1
0
0
2
1
2
1
2
1
−
2
1
1,01,0 ±=∆±=∆ smm andl
33](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-33-320.jpg)
![The most important interaction between a nucleus of charge Ze and an electron of
charge e is, of course, the Coulomb interaction −Ze2/r. All other interactions between a
nucleus and the electrons of the same atom are classified as hyperfine interactions, and
among these the most important are the ones that arise as a result of a nucleus
possessing a magnetic dipole moment (i.e., associated with the nuclear spin) and an
electric quadrupole moment (i.e., associated with a departure from a spherical charge
distribution in the nucleus). In the former case the nuclear magnetic dipole moment
interacts with the electronic magnetic dipole moments which are associated with the
electronic orbital and/or spin angular momenta. In the latter, the interaction occurs when,
at the position of the nucleus, the electronic charge distribution produced an electric field
gradient with which the nuclear electric quadrupole moment can interact.
Magnetic field created by the proton. Outside
the proton, the field B is that of a dipole – µµµµI;
inside, the field Bi depends on the exact
partition of the magnetism of the proton.
5
22
o
5
o
5
o 3
π4
µ
3
π4
µ
3
π4
µˆˆˆ
r
rz
B
r
zy
B
r
zx
BBBB zyxzyx
−
===⇔++= IIIkjiB µµµ and,
The magnetic field inside the proton, Bi, is given by (SI system):
Consider a proton of radius ro. The partition of magnetism
inside the proton creates a field B outside which can be
calculated by attributing to the proton a magnetic moment µµµµI
(which is taken to be parallel to the z-axis – see Figure).
Hyperfine Interactions
z
y
x
B
µµµµI
Bi
3
o
o 2
π4
µ
r
Bi Iµ=
2017
MRT
where µo is the magnetic permeability of free space.
ro
The external field is thus purely dipolar.
For r >> ro, we obtain the components of B (by calculating the
rotational of AI = (µo/4π)[(µµµµI ×××× r)/r3]) (SI system):
51](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-51-320.jpg)
![To derive the form of this interaction we return to the Dirac equation(mo ≡me and q≡−e):
with:
2017
MRT
It is advantageous to separate the Hamiltonian into two parts:
where:
u
e
u )()(
2
1
)( ψψφ ππ ••=+′ ΣΣΣΣΣΣΣΣ K
m
eE
φecmE
cm
KcmEE
++′
=−=′ 2
e
2
e2
e
2
2
and
HFo
e2
1
HHe
c
e
K
c
e
m
H +=−
+•
+•= φApAp ΣΣΣΣΣΣΣΣ
φeKH −••= )()(o pp ΣΣΣΣΣΣΣΣ
HHF is the hyperfine Hamiltonian and it is the quantity we will be deriving.
u
e2
1
ψφψ
−
+•
+•=′ e
c
e
K
c
e
m
E ApAp ΣΣΣΣΣΣΣΣ
which becomes (with ππππ=p++++(e/c)A):
52
and:
)]()[(
2
)]()()()[(
2 2
e
2
e
HF AApAAp ••+••+••= ΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣ K
cm
e
KK
cm
e
H](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-52-320.jpg)
![We concentrate on HHF which contains the entire dependence on the vector potential
A and the last term on the right is quadratic in A; it may therefore be neglected in a first
approximation.
2017
MRT
Also, since:
we have:
and HHF becomes:
)()(])()([)(()( r
r
rprrrrp f
rrd
dK
ifKKffKifKifΚ hhh −=+−=−= ∇∇∇∇∇∇∇∇∇)∇)∇)∇)
rrd
dK
K
r
=∇∇∇∇
rdr
dK
iKΚ
r
pp h−=
••+••−••= ))(())((
1
))((
2 e
HF pAArAp ΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣ K
rdr
dK
iK
cm
e
H h
The potential φ is assumed to be a function of r only (i.e., φ(r)) which means that K
depends only on r (i.e., K(r)) so that:
Using the identity (ΣΣΣΣ•A)(ΣΣΣΣ•B)=A•B+iΣΣΣΣ•(A××××B), HHF is converted to:
)]([
1
)()(
2 e
HF ArArpAAppAAp ××××ΣΣΣΣ××××××××ΣΣΣΣ •+•−+•+•+•= i
rdr
dK
iKiK
cm
e
H h
53](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-53-320.jpg)
![With these relations, we get:
Finally:
2017
MRT
When these relations are included into our last development for HHF one obtains:
in which:
cm
e
B
e22
hh
== µandΣΣΣΣS
)(π4
3
)( 35
2
rµ
µr
rµ
µµµ
A δΙΙΙΙ
ΙΙΙΙ
ΙΙΙΙ
ΙΙΙΙΙΙΙΙΙΙΙΙ
++++−−−−∇∇∇∇∇∇∇∇××××∇∇∇∇××××∇∇∇∇××××∇∇∇∇
rrrrr
•=
∇−
•=
=
333
)(
])[(
1
][
1
rrrr
rµrµ
rµrµrrrµrAr ΙΙΙΙΙΙΙΙ
ΙΙΙΙΙΙΙΙΙΙΙΙ −−−−))))))))((((−−−−))))××××((((××××××××
•
=••==
••
+
•
+
•+
••
+
•
= 4253HF
))((
2)(π4
))((3)(
2
rrrd
dK
rr
KH BB
rSrµSµ
Sµr
rSrµSLµ II
I
II
µδµ
−−−−
with ΣΣΣΣ whose componentsare Pauli matricesσσσσ=[σ1,σ2,σ3]. We now examine K and dK/dr.
rZecmE
cm
K 22
e
2
e
2
2
++′
=
If ϕ is replaced by Ze/r, we have:
56](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-56-320.jpg)
![An alternative expression for the magnetic hyperfine Hamiltonian is obtained by
transforming the dipole-dipole part by means of the Landé formula. Let
Then:
2017
MRT
and:
But since (L –S)•J=(L –S)•(L+S)=L2 −S2 and recalling that | j,mj〉=|l,s; j,mj 〉 is an
eigenfunction of L2 and S2 (as well as J2 and Jz ), we get 〈 j;mj|(L–S)•J| j;mj〉=
〈 j,mj|L2 −S2 | j,mj〉=l(l+1)−s(s+1). Also r•J=r•(L+S)=r•(r××××p)/h+r•S=r•S=S•r.
Therefore, as we obtained earlier (r•J)(r•S)=(r•S)(r•S)=(1/4)(ΣΣΣΣ•r)(ΣΣΣΣ•r)=r2/4.
Integrating over the radial part of the wave function we obtain 〈 j,mj |B′•J| j,mj〉=
−2µB[l(l+1)−s(s+1)+¾]〈1/r3〉=−2µBl(l+1)〈1/r3〉 (for s=½). so that:
•
+−=′ 53
)(3
2
rr
B
rSrSL
B
−−−−
µ
( )½
1
)1(
)1(
2 3
=
+
+
−=′ s
rjj
B for
ll
µB
sjj
jj
jj mjmj
jj
mjmj
mjmj ′
+
•′
=′′ ,,
)1(
,,
,, J
JB
B
jjBjj mj
rr
mjmjmj ,
))((3
,2,, 53
rSJrSL
SB
••
+−=•′
−−−−
µ
63](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-63-320.jpg)
![For Hydrogen in an s state only the contact term is effective. Since l=0, J may be
replaced by S so that:
and, since the spin of the proton is I=½, we have also s=½ thus F=0 & 1. Hence:
which indicates that the only diagonal elements are non-zero. For the two possible
values of F, the energies are:
( )
( )
=−=−
==
=
0
4
3
π4
1
4
1
3
π4
2
100
2
100
FA
FA
E
FB
FB
ψγµ
ψγµ
h
h
)(
2
1 222
ISFSI −−=•
−+=
+−+−+=−−=•
2
3
)1(
2
1
)]1()1()1([
2
1
,)(½,,, 222
FF
IIssFFmFmFmFmF FFFF ISFSI
2S1/2
0.047 cm−1 (or 21 cm)
F = 1
F = 0
n = 1
Magnetic hyperfine splitting of the ground
state of hydrogen. The entire splitting is due
to the Fermi contact term geµBgNµN (i.e., a
quantum effect.).
o
2
100
3
61
3
6π1
a
AE B
FB
h
h
γµ
ψγµ ===∆
For hydrogen, |ψ 100|2 =1/πao
3 (ao =0.529×10−8 cm), γh =µN gN =
5.05×10−23 erg/GHz and with µB =0.927×10−20 erg/GHz, the
difference in energy between the two levels for E is:
2017
MRT
as show in the Figure. If we set ∆E= hν, the frequency ν is
1420.4058 MHz, which corresponds to a wavelength of 21 cm.
65](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-65-320.jpg)
![Next, we consider the dipole-dipole term in our last expression for HHF.
Also, we have I•J=½(F2 −I2 −J2) and 〈F,mF|I•J|F,mF〉=½[F(F+1)−I(I+1)− j( j+1].
Again, only the diagonal matrix elements are non-zero. Therefore the energies are given
by:
lll )½)(1(
11
33
o
3
3
++
=
na
Z
r
For hydrogen, we use from the Table for 〈rk〉:
)]1()1()1([
)½)(1(33
o
3
+−+−+
++
= IIjjFF
jjna
Z
E B
l
hγµ
2017
MRT
66](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-66-320.jpg)
Yl
ml*(θ1,ϕ1)Yl
ml (θ2,ϕ2) – we get:
in which re is the position vector of the electron, rp is the position vector of the p-th
proton, and the sum is taken over all Z protons.
∑−=
=•=•
l
l
ll
llll
l
ll
m
mm
YY ),(),(),(),( eepp
*
ee
)(
pp
)()(
e
)(
p ϕθϕθϕθϕθ YYYY
∑
∞
=
+
>
<
•
+
=
0
1
)(
p
)(
e
pe
12
1
π4
1
l
l
l
ll
l r
r
YY
rr −−−−
Substitution in 1/|r1 −−−− r2|above yields:
z
y
x
rp re
|re − rp | e
Z eO
68](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-68-320.jpg)
![In such tables we find (e.g., with s=a+b+c and X=a(a+1)−b(b+1)−c(c+1)):
2017
MRT
where X=I(I+1)+ j( j+1)−F(F+1).
2/1
)]32)(22)(12(2)12)(32)(22)(12(2)12[(
)]1()1(4)1(3[2
)1(
2 +++−+++−
++−+
−=
cccccbbbbb
ccbbXX
bc
cba s
Themainproblemthen is to evaluatethe reduced matrix elements 〈I||Q(2)||I 〉〈 j ||U(2) || j〉.
These 6j-symbols are invariant under:
1) an interchange of columns and;
2) an interchange of any two numbers in the bottom row with the corresponding two
numbers in the top row.
)32)(22)(12(2)12)(32)(22)(12(2)12(
)]1()1(4)1(3[2
)1(
2 +++−+++−
++−−
−=
++
jjjjjIIIII
jjIIXX
Ij
FjI jIF
So, by just interchanging the first and third columns, we find ours:
71](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-71-320.jpg)
![The computation of 〈 j ||U(2) || j 〉 proceeds in analogous fashion. This time we define a
quantity eQ/2 as:
2017
MRT
where, from (i.e., for l=2) U(2) =−e√(4π/2⋅2+1)Ye
(2) /re
2+1, we get:
−
−=−= 5
e
2
e
2
e
3
e
ee
0
2
)2( 3
2
11
),(
5
π4
r
rz
e
r
YeUO ϕθ
We note that ∂2(−e/r)/∂z2 =−e(3z2 −r2)/r5 is the zz (diadic) component of the electric field
gradient tensor produced by an electron at a point whose coordinated with respect to the
electron are [x,y,z]. Since the origin of the coordinate system (see previous Figure) has
been positioned at the nucleus 2UO
(2) in the equation above is the zz component of the
electric field gradient tensor at the nucleus produced by an electron at re, or UO
(2) =
½(∂2V/∂z2)O =½Vzz where V is the potential due to the electron and the second derivative
is evaluated at the origin O (i.e.,at nucleus).We then have eq=〈 j,mj =j|Vzz | j,mj =j〉=〈Vzz〉
which is the average (or expectation value) of Vzz taken over the electronic state | j, j〉.
jmjUjmjQe jOj === ,,
2
1 )2(
qe
jj
jjjj
jj
)12(
)32)(32)(1)(12(
2
1)2(
−
++++
=U
Again, the use of the Wigner-Eckart theorem leads to the result:
73](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-73-320.jpg)
![Now (without proof – c.f. M. Weissbluth) assuming axial symmetry, Vxx =Vyy, we have:
2017
MRT
where Vzz =eq. For this case there are only diagonal elements with twofold degenerates
in mI, that is, states with ±mI have the same energy.
)]1(3[
)12(4
,,
)]3([
)12(4
2
2
QQ
22
Q
+−
−
==
−
−
=
IIm
II
qQe
mIHmIE
IIV
II
eQ
H
III
zzz
i. When I=1, the energies are:
( )
( )
=−
±=+
=−=+−
−
=
0
2
1
1
4
1
)23(
4
1
)]11(13[
)11.2(1.4 2
2
222
2
Q
I
I
II
mqQe
mqQe
mqQem
qQe
E
I = 1
mI EQ
(1/4)e2qQ±1
0 −(1/2)e2qQ
and the Figure below shows the quadrupole splitting (when I=1 and e2 qQ>0).
As has already been noted, the quadrupole interaction vanishes when I=0 or ½.
75](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-75-320.jpg)
![ii. For I=3/2, the energies are:
2017
MRT
( )
( )
±=−
±=+
=
−=+−
−
=
2/1
4
1
2/3
4
1
4
15
3
12
1
)]12/3(2/33[
)12/3.2(2/3.4 2
2
222
2
Q
I
I
II
mqQe
mqQe
mqQem
qQe
E
and the Figure below shows the quadrupole splitting (when I=3/2 and e2 qQ>0).
and the Figure below shows the quadrupole splitting (when I=2 and e2 qQ<0).
I = 3/2
mI EQ
(1/4)e2qQ±3/2
−(1/4)e2qQ±1/2
I = 2
mI EQ
−(1/8)e2qQ±1
−(1/4)e2qQ0
(1/4)e2qQ±2
iii. Finally, for I=2, the energies are:
( )
( )
( )
=−
±=−
±=+
=−=+−
−
=
0
4
1
1
8
1
2
4
1
)63(
24
1
)]12(23[
)12.2(2.4
2
2
2
222
2
Q
I
I
I
II
mqQe
mqQe
mqQe
mqQem
qQe
E
76](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-76-320.jpg)
![This is the energy associated with the scalar potential energy, ϕ (105 cm−1).
And the significance of the various terms and their energies, is indicated to within an
order of magnitude (N.B., the ‘cm−1’ scale is given by the wave number 1/λ ≅ 8000 cm−1):
This contains the kinetic energy (i.e., p2 /2me) and interaction term (i.e., (e/2mec)(p • A+ A • p)
+ e2A2/2mec2) with a field represented by a potential vector A (105 cm−1). The interaction terms
are responsible or contribute to numerous physical processes among which are absorption,
emission and scattering of electromagnetic waves, diamagnetism, and the Zeeman effect.
2017
MRT
ϕe
The spin-orbit interaction (10-103 cm−1). More precisely, itis (eh/8mec2){σσσσ •[p −−−− (e/c)A]×××× E −−−−
σσσσ • E ×××× [p −−−− (e/c)A]} and it arises from the fact that the motion of the magnetic moment gives
rise to an electric moment for the particle which then interacts with the electric field.
This term appears in the expression of the relativistic energy:
and is therefore a relativistic correction to the kinetic energy (i.e., p2/2me) (0.1 cm−1).
The interaction of the spin magnetic moment (i.e., µS = 2⋅e/2me⋅h/2) with a magnetic field B=
∇∇∇∇ ×××× A (1 cm−1). Thus, it is the magnetic moment of one Bohr magneton, eh/2mec
(i.e., µB = 9.2741×10−24 A⋅m2 or J/T) with the magnetic field.
2
e2
1
+ Ap
c
e
m
A××××∇∇∇∇σσσσ •
cm
e
e2
h
23
e
4
8 cm
p
L+−+≅+ 23
ee
2
2
e
2222
e
82
)(
cm
p
m
p
cmcpcm
4
ϕ∇∇∇∇∇∇∇∇ •− 22
e
2
8 cm
eh
This term produces an energy shift in s-states and is known as the Darwin (1887-1962) term
(< 0.1 cm−1). It is thus a correction to the direct point charge interaction due to the fact that in the
representation (Foldy-Wouthuysen), the particle is not concentrated at a point but is spread out
over a volume with radius whose magnitude is roughly that of a Compton wavelength, h/mec.
p××××∇∇∇∇σσσσ ϕ•− 22
e4 cm
eh
ϕe
c
e
m
−
+
2
e2
1
Ap
As a combination, this term represents the interaction of a point charge with the
electromagnetic field.
78](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-78-320.jpg)
![Eigenfunctions of the wave equation having different energy eigenvalues are
necessarily orthogonal, as we will now show. Let ψi and ψj be solutions of:
2017
MRT
If the integration is extended over all space, we obtain:
0)(
2 *
2
2
*2
2
*2
2
*2
2
2
2
2
2
2
*
=−+
∂
∂
+
∂
∂
+
∂
∂
−
∂
∂
+
∂
∂
+
∂
∂
∫ ∫ ∫
∫ ∫ ∫
∞
∞−
∞
∞−
∞
∞−
∞
∞−
∞
∞−
∞
∞−
zdydxdEE
m
zdydxd
zyxzyx
ijji
i
jjjiii
j
ψψ
ψ
ψψψψψψ
ψ
h
82
0)(
2
0)(
2 *
2
*2
2
2
=−+∇=−+∇ jjjiii VE
m
VE
m
ψψψψ
hh
and
Multiplying the terms in the first equation by ψ j
* and the terms in the second by ψi on the
right, and subtracting the second equation from the first, we obtain;
0)(
2
)( *
2
*22*
=−+∇−∇ ijjiijij EE
m
ψψψψψψ
h
or integrating over the particle coordinates after representing the system in Cartesian
coordinates, we obtain:
0)(
2
])([ *
2
*22*
=−+∇−∇ ∫∫ τψψτψψψψ dEE
m
d ijjiijij
h](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-82-320.jpg)
![Substituting V =V 0 +V ′, Ei =E0
i +E′i and ψi =ψ 0
i +ψ ′i into the Schrödinger equation
above and grouping the result in ascending order of approximation, we have:
2017
MRT
This first term is zero by comparing to the unperturbed Schrödinger equation, leaving us
with only the second term for a first-order approximation:
0])(-)()[()( 000000
=′′−′+′−+′−+− iiiiiiii EVEVEHEH ψψψψ assuchtermsordersecond
85
0)()( 000
=′−′+′− iiii EVEH ψψ
We now expand ψ ′i in terms of the complete orthonormal set of solutions to the
unperturbed Schrödinger equation, ψ 0
j. That is, we let:
∑
∞
=
=′
0
0
j
jjii a ψψ
which, with first-order approximation above, gives:
0)()( 0000
=′−′+−∑ ii
j
jjii EVaEH ψψ
Note that from H0ψ 0
i =E0
iψ 0
i, this last equation can be rewritten:
0)()( 0000
=′−′+−∑ ii
j
jjiij EVaEE ψψ
Multiplying this last equation by ψ 0
k
* on the left and integrating over all space, we get:
0)( 0*00*00*000
=′−′+− ∫∫∑ ∫ τψψτψψτψψ dEdVdaEE ikiik
j
jkjiij](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-85-320.jpg)
![The integrand in the last monster integral is essentially the electrostatic interaction
energy between two shells of charge density exp(−ρ1) and exp(−ρ2). To begin evaluating
it let us consider just the integral over ρ1. Let us consider further the potential at a point ρ
due to a shell of thickness dρ1 at ρ1; it is given by:
2017
MRT
and
)(eπ4
e
π4 111
1
12
1
1
1
ρρρρ
ρ
ρ
ρ ρ
ρ
<= −
−
ford
d
90
)(e
π4e
π4 11
2
1
12
1
1
1
ρρρρ
ρρ
ρ
ρ ρ
ρ
>= −
−
ford
d
)]2(e2[
π4
e
π4
eπ4)( 1
2
111
11
+−=+= −
∞
−
∞
−
∫∫ ρ
ρ
ρρ
ρ
ρρρφ ρ
ρ
ρ
ρ
ρ
dd
The total potential at ρ due to the infinite sphere of charge density exp(−ρ1) is given by:
This is the potential at a point ρ due to the entire distribution of charge density exp(−ρ1).](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-90-320.jpg)
![We can now evaluate the potential energy of interaction between the distribution of
charge density exp(−ρ1) and exp(−ρ2). The potential energy per unit volume of a charge
density exp(−ρ2) at a point ρ2 is given by (4πρ2)[2−(ρ2 +2)exp(−ρ2)]exp(−ρ2). Hence, the
second shell of charge 4πρ2
2exp(−ρ2)dρ2 has a potential energy of:
2017
MRT
91
])2(e2[
eπ)4(
2
2
2
2
2
2
2
2
+−
−
−
ρ
ρ
ρρ ρ
ρ
d
The total electrostatic potential of the sphere of charge density exp(−ρ2) due to the
sphere with charge density exp(−ρ1) is the integral over ρ2 of this last expression:
2
0
222
2
π)4(
4
5
])2(e2[eπ)4( 22
=+−∫
∞
−−
ρρρ ρρ
d
Therefore our monster integral yields a result of:
2
4
e
0
2
1
24
5
24
5
h
eZm
a
eZ
E ==′
and from Ei =−(meZ2e4/2h2)(1/n1
2 +1/n2
2)+ ∫ψ 1
0*
(1,0)ψ 2
0*
(1,0)(e2/r12)ψ 1
0
(1,0)ψ 2
0
(1,0)dτ above:
−−= ZZ
em
E
4
5
2
2
2
2
4
e
1
h
where mee4/2h2 is the ground state energy of the Hydrogen atom and 2Z 2 times this is
the unperturbed ground state energy of the two Helium electrons.](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-91-320.jpg)
![Consider a system of two identical particles such that one particle is in a state labeled
α and the other in another state labelled by β where α(1) represents particle 1 in state α,
&c. If particles 1 and 2 are interchangeable in either the α(1)α(2) or β(1)β(2) state, then
eigenfunction is unchanged and therefore these states are said to be symmetric. The
other states α(1)β(2) and β(1)α(2) are neither entirely symmetric nor entirely
antisymmetric, however, and it is convenient to treat them as a superposition of two
other states one of which is entirely symmetric and the other antisymmetric. We
therefore use linear combinations of the α(1)β(2) and β(1)α(2) states to represent the
two remaining wave functions of the system:
2017
MRT
where the factor 1/√2 is used for normalization. Since the α(1), β(2), α(2), and β(1) are
all solutions of the Schrödinger equation, the ψS and ψA will also be solutions. Now, in
our universe, systems of identical particles with integer spin must be represented by
wave functions which are symmetric with respect to the exchange of any two particles.
Similarly, all systems of identical particles with half-integer spin must be represented by
wave functions which are antisymmetric after exchange of any two particles. For multi-
electron system, this result was first postulated by W. Pauli in 1924 even before the
advent of quantum mechanics. The Pauli exclusion principle states that in a multi-elec-
tron atom there can never be more than one electron in the same quantum state. This
statement can be seen to be a consequence of saying that the electron wave function
must be antisymmetric since electrons belong to the half-integer class of particles.
)]2()1()2()1([
2
1
)]2()1()2()1([
2
1
αββαψαββαψ −=+= AS or
94](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-94-320.jpg)
![Towards the end of the The Hydrogen Atom chapter we saw that the wave function for
an electron with spin could be written as follows:
2017
MRT
so that for the Helium atom with two electrons we will have to take the appropriate
combination of the two wave functions ψa(1)=ψa(1)ξξξξa and ψb(2)=ψb(2)ξξξξb, where ψa(1)
represents the first electron at position 1 with a spin z-component represented by ξξξξa (i.e.,
either +½h or −½h) and similarly for ψb(2). The total wave function for the two electrons
will have to be the appropriate combination of space and spin states that makes it
antisymmetric. This total wave function can of course be written as the product of a
space part times a spin part; if the space part is symmetric, the spin part must be
antisymmetric and vice versa. As we have seen, the space part can be written:
95
smmn ξξ llψψψ == SpinSpace
_ _ _
_
)]1()2()2()1([
2
1
)]1()2()2()1([
2
1
babaAbabaS ψψψψψψψψψψ −=+= or](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-95-320.jpg)
![The spin wave function will be a bit more complicated because the separate spin
angular momenta can add vectorially just as the orbital and spin angular momenta do in
the case of the two-electron atom. Instead of having J=L+S, here S=s1 ++++s2 so that S=0
or 1. The magnitude of S will of course be √[S(S+1)]h.
2017
MRT
For S=0, ms =0 and we have just a single state while for S=1, ms =+1, 0, −1 and a triplet
spin state results. This corresponds to the three possible orientations with respect to
some preferred (i.e., z) direction in space and is equivalent to saying that we can start
with the following four spin combinations: ξξξξa(½)ξξξξb(½), ξξξξa(−½)ξξξξb(−½), ξξξξa(½)ξξξξb(−½), and
ξξξξa(−½)ξξξξb(½) to construct four symmetric and antisymmetric combinations:
tripletsymmetric
singletricantisymmet
-
)½()½(
)]½()½()½()½([
2
1
)½()½(
1
0
1
1
1
1
-)]½()½()½()½([
2
1
00
−−
−+−
−
+
−−−
ba
baba
ba
baba
smS
ξξ
ξξξξ
ξξ
ξξξξ
96](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-96-320.jpg)
![The total eigenfunction must be antisymmetric and thus we can form the following
combination from ψS , ψA and the above four spin combinations:
2017
MRT
and
97
]-[]-[ tripletsymmetricandsingletricantisymmet ⊗⊗ AS ψψ
or:
)]½()½()½()½([)]1()2()2()1([
2
1
babababa ξξξξ −−−⊗+ ψψψψ
−−
−+−⊗−
)½()½(
)]½()½()½()½([
2
1
)½()½(
)]1()2()2()1([
2
1
ba
baba
ba
baba
ξξ
ξξξξ
ξξ
ψψψψ
Notice that if the spin part of the wave function is symmetric, corresponding to parallel
spins, the space part must be antisymmetric. This has the interesting and important
consequence that the electrons will have small probability of being found close together
if they have parallel spins and a maximum probability of being found close together if
they have antiparallel spins. One can say that in effect parallel spins repel and
antiparallel spins attract.](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-97-320.jpg)
![By use of these matrix elements between the various hydrogen-like wave functions,
the secular determinant is written as:
2017
MRT
since all the Js and Ks with the same subscript are equal.
0
)(000000
)(000000
00)(0000
00)(0000
0000)(00
0000)(00
000000)(
000000)(
pp
pp
pp
pp
pp
pp
ss
ss
=
∆−
∆−
∆−
∆−
∆−
∆−
∆−
∆−
EJK
KEJ
EJK
KEJ
EJK
KEJ
EJK
KEJ
105
where ∆E is the small energy shift due to the perturbation. This determinant can be
rewritten as:
0])][()[( 32
p
2
p
2
s
2
s =−−∆−−∆ KJEKJE](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-105-320.jpg)
![x
y
x
y
r
ψ0
S
ψ0
A
ψ0
S
ψ0
A
r
When r is large the electron can be thought of as belonging to one proton or the other,
but as r becomes small this distinction is not possible and we must consider the effects
of exchange degeneracy. In this case, we ought then to take the total wave function as
being either symmetric or antisymmetric. For convenience we take both protons to be
lying on the x-axis so that under an exchange it is only the x coordinate that varies. Then
we write:
2017
MRT
Further, for the ground state U100 we choose the x-axis so that we have U100(x,0,0). Both
ψ0
S and ψ0
A are shown in the Figure for different values of r. Looking at these plots, we
see that as r→0 (at x=0), ψ0
S →maximum and ψ0
A →0.
)],,(),,([
2
1
)],,(),,([
2
1
2121 zyxUzyxUzyxUzyxU nn
A
nnn
S
n −=+= ψψ or
120
Illustrating the form of the symmetric and antisymmetric wave functions of the H2
++++ molecule for two
different proton separation distances (Left vs Right) .](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-120-320.jpg)
![Then the degenerate perturbation integrals are:
2017
MRT
and
∫∫ ′= 212B1A2
*
B1
*
A )()()()( ττψψψψ ddHJ rrrr
127
where dτ1 and dτ2 are the differential spherical volume elements (e.g., dτ1 =
r1
2sinθ1dr1dθ1dϕ1). The second term in the exchange integral K with H0, which we will
label K0, must be included because our initial wave functions are not orthonormal. Since:
∫∫∫∫ +′= 211B2A
0
2
*
B1
*
A211B2A2
*
B1
*
A )()()()()()()()( ττψψψψττψψψψ ddHddHK rrrrrrrr
)()(2)()()()( 1B2A11B2A
0
1B2A
0
rrrrrr ψψψψψψ EEH ==
we have:
∫∫= 211B2A2
*
B1
*
A10 )()()()(2 ττψψψψ ddEK rrrr
The contribution of e2/rAB term to J is simply e2/rAB, since the wave functions are
normalized and e2/rAB may be taken outside the integration over the electron
coordinates. Similarly, the contribution of the e2/rAB term to K is simply [(e2/rAB)/2E1]K0.
The contribution of e2/r1B to J would be ∫ψA
*(r1)(−e2/r1B)ψA(r1)dτ1 since ψB(r2) is
normalized and r1B is not a function of the position of the second electron. The
contribution of e2/r1B to K would be similar.](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-127-320.jpg)
![It is found that the symmetric wave function:
2017
MRT
is the correct zero-order wave function which with its antisymmetric twin causes the
perturbation matrix to be diagonal. The wave function above is also the symmetric wave
function whose eigenvalue is approximately E0 −K, the lower of the two energy
eigenvalues including resonances. This is in contrast to the case of Helium where both
electrons are in the same atom, and the antisymmetric wave function was found to be
the lower of the two energy eigenvalues including resonances. The attractive bonding
force of the Hydrogen molecule has been shown to be due to the possibility of two
electrons being in a symmetric state (i.e., being interchangeable in the space wave
function representation). Since no more than two electrons can be in one symmetric
state at the same time, the degeneracy in this case can be only twofold.
)]()()()([
2
1
1B2A2B1A
1
0
rrrr ψψψψψ +
+
=
E
K
S
129](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-129-320.jpg)
![The eigenfunctions of the harmonic oscillator version of the Schrödinger equation:
in which Hn(x) is a Hermit polynomial* of degree n and the eigenvalues are:
2017
MRT
A more convenient form to the ψn(q) solution above is achieved by changing the
variables to:
We then have:
which has the properties:
nn
m
uq
m
q ψξ
ω
ω
)(
h
h
== and
)()1()(
22
1
1
2
1
2
1
11
11
ξξξ
ξ
ξ
ξ
ξδξ
n
n
nnnn
nnnnnmnm
uuu
n
u
n
u
unu
d
d
unu
d
d
duu
−=−+
+
=
+=
−=
+=
−+
+−
∞+
∞−∫
and
,,,
=
−
q
m
H
m
n
q n
q
m
nn
hh
h ω
e
π
ω
!2
1
)(
2
2
ω4/1
2/
ψ
( ),...,,nnEn 210ω)½()ω( =+= h
* The Hermit polynomials are solutions to the differential equation d2Hn/dx2 – 2xdHn/dx − 2nHn = 0 and have a solution of the form
Hn(x) = (−1)n exp(x2)dn[exp(−x2)]/dxn. The first four Hermit polynomials are H0(x) = 1, H1(x) = 2x, H2(x) = −2 + 4x2 and H3(x)= −12x + 8x3.
)(e
)!2π(
1
)( 2
2/12/
2
ξξ ξ
nnn H
n
u −
=
142](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-142-320.jpg)
![We shall now investigate the harmonic oscillator be means of a matrix formulation. For
convenience, let:
so that the Hamiltonian H=p2/2m+½mω2q2 becomes
2017
MRT
The transition to quantum mechanics is made by reinterpreting P and Q as Hermitian
operators which obey the commutation relation:
which is equivalent to the replacement of P by −ih∂/∂Q. It is important to note that the
quantum mechanical operators Q and P are independent of time.
We now construct the linear combinations:
hiQPQPPQ =≡− ],[
)ω(
ω2
1
)ω(
ω2
1 †
PiQaPiQa −=+=
hh
and
22
2
2
)()( qmqQ
m
p
pP == and
)ω(
2
1 222
QPH +=
or:
)(
2
ω
)(
ω2
††
aaiPaaQ −=+=
hh
and
143](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-143-320.jpg)
![In terms of a and a†, the commutator [Q,P]=ih becomes:
and the Hamiltonian H=½(P2 + ω2Q2) takes the form:
where:
2017
MRT
is called the number operator and it is Hermitian. Also from [a,a†]=1, we have:
)1()1()1( †††
−=−=−== NaaaaaaaaaaNa
1],[ †
=aa
+=
+=+=
2
1
ω
2
1
ω)(ω
2
1 †††
NaaaaaaH hhh
aaN †
=
144
)1()1( ††††††
+=+== NaaaaaaaNa
and:](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-144-320.jpg)
![We now express the vector potential A(r,t) as a linear superposition of plane waves:
2017
MRT
∑∑
∑∑
=
−•−−•
−•−−•−•−−•
+=
+++=
k
rkrk
k
rkrk
k
rkrk
kk
kkkk
kkke
kkkekkkerA
2,1
)ω(*)ω(
)ω(*
2
)ω(
22
)ω(*
1
)ω(
11
]e)(e)()[(ˆ
]e)(e)()[(ˆ]e)(e)()[(ˆ),(
r
ti
r
ti
rr
titititi
AA
AAAAt
where êr(k) is a real unit vector which indicates the linear polarization; êr(k) depends on
the propagation direction k and has two independent components ê1(k) and ê2(k) which
satisfy êr(k)•ês(k) =δrs (r,s=1,2). Ar(k) is a constant amplitude for the mode (k,r).
The Coulomb gauge ∇∇∇∇•A=0 ensures the transversality of the electromagnetic fields
so that êr(k)•k=0. Thus ê1(k), ê2(k), and k form a right-handed set of mutually orthogonal
unit vectors.
ˆ ˆ
The vector potential A(r,t) above then consists of plane wave each one labeled by the
propagation vector k and the real polarization vector êr(k). Furthermore, A(r,t) is real.
We may also replace the linear polarization vectors ê1(k) and ê2(k) by unit vectors
which indicate circular polarizations. This is accomplished by defining ê+(k)=−(1/√2)[ê1(k)
++++iê2(k)] and ê−(k)=(1/√2)[ê1(k)−−−−iê2(k)]. These vectors satisfy êr(k) ××××ês(k)=iδrs (r,s=±1).
With r=+1 the cross product gives a vector parallel to the direction of propagation
whereas with r=−1 the cross product is antiparallel. For this reason one refers to ê+(k)
and ê−(k) as positive and negative helicity (unit) vectors. ê+(k) and ê−(k) represent left
and right circular polarization, respectively.
152](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-152-320.jpg)
![The electric and magnetic fields are obtained directly from the vector potential.
For the electric field:
For the magnetic field, since it is defined by B(r,t)=∇∇∇∇××××A(r,t), it is necessary to
evaluate ∇∇∇∇××××A. Noting that ∇∇∇∇××××(ϕA)=∇∇∇∇ϕ ××××A++++ϕ∇∇∇∇××××A we have:
2017
MRT
Since both the amplitude Ar(k) and the polarization vector êr(k) are constant the second
term vanishes and we get:
Hence the magnetic field is:
∑∑ −•−−•
−=
∂
∂
−=
k
rkrk
k
kk
kkke
rA
rE
r
ti
r
ti
rr AA
c
i
t
t
c
t ])e()e([)(ˆω
),(1
),( )ω(*)ω(
)(ˆ)e()(ˆe)()(ˆ)e( kekkekkek rkrkrk
r
i
rr
i
rr
i
r AAA ××××∇∇∇∇××××∇∇∇∇××××∇∇∇∇ •••
+=
)(ˆˆ)e(
ω
)(ˆe)()(ˆ)e( kekkkekkek rkkrkrk
r
i
rr
i
rr
i
r A
c
iAA ××××××××∇∇∇∇××××∇∇∇∇ •••
+==
∑∑ −•−−•
−==
k
rkrk
k
kk
kkkekrArB
r
ti
r
ti
rr AA
c
i
tt ])e()e(][)(ˆˆ[ω),(),( )ω(*)ω(
××××××××∇∇∇∇
153](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-153-320.jpg)
![To obtain the Hamiltonian for the electromagnetic field in the cavity it is necessary to
express the field energy W in terms of canonical variable.
From electromagnetic theory:
where V is the volume of the cavity and the fields E and B are given by the expressions
derived earlier. Because of the boundary conditions of the type exp(ikx x)=exp[ikx (x+L)],
we get:
2017
MRT
Therefore:
If we write:
( )
( )
=
≠
=∫
•±
0
00
e
k
krk
for
for
V
dV
V
i
ti
rr AtA k
kk ω
e)(),( −
=
∫∫ +=•+•=
VV
dVdVW )(
π8
1
)(
π8
1 22
BEBBEE
VdVVdV
V
i
V
i
kk
rkk
kk
rkk
′
•′−±
−′
•′+±
== ∫∫ δδ )()(
ee and
in our previous expression for E(r,t) and use the orthogonality condition êr(k)•ês(k)=δrs
on the polarization vectors and the relation ωk =ω−k, we get:
∑∑∑∑∑∫ −+−−•−=•
k
k
k
k kkkkkekekkEE
r s
srsr
r
rr
V
tAtAtAtA
c
V
tAtA
c
V
dV )],(),(),(),()][(ˆ)(ˆ[ω),(),(ω
2 **2
2
*2
2
154](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-154-320.jpg)
![To compute the field energy associated with B we employ the vector identity
(A××××B)•(C××××D)=(A•C)(B•D)−(A•D)(B•C) to show that [k××××êr(k)]•[−k××××ês(k)]=δrs in
which the orthogonality property êr(k)•k=0 has been used. We also have
[k××××êr(k)]•[−k××××ês(k)]=−êr(k)•ês(−k).
2017
MRT
Actually, W is dependent of time because of the exponential time dependence of Ar(k,t)
as given previously. Hence:
It is observed that the total energy is merely the sum of the energies in the individual
modes as a consequence of the orthogonality conditions ∫V exp(±i(k+k′)•r)dV =δk′−kV
and ∫V exp(±i(k−k′)•r)dV =δk′kV; furthermore the energy is shared equally by the electric
and magnetic fields.
∑∑=
k
k kk
r
rr tAtA
c
V
W ),(),(ω
π2
*2
2
ˆ
ˆ ˆ
Therefore in computing the integral of B•B using the expression B(r,t) derived
previously one finds two terms identical to the two terms on the right side of the
∫V E•EdV except for the sign of the second term. The field energy then becomes:
ˆ ˆ
∑∑=
k
k kk
r
rr AA
c
V
W )()(ω
π2
*2
2
155](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-155-320.jpg)
![We now introduce a new set of variables:
which when substituted into our previous expression for W, gives:
2017
MRT
)]()(ω[
π
ω
)()]()(ω[
π
ω
)( *
kkkkkk k
k
k
k
rrrrrr PiQ
V
c
APiQ
V
c
A −=+= and
∑∑ +=
k
k kk
r
rr QPW )](ω)([
2
1 222
Upon inverting the Qr(k) and Pr(k) variables above, we get:
)]()([
2
ω
π
)()]()([
2
1
π
)( **
kkkkkk k
rrrrrr AA
c
V
iPAA
c
V
Q −−=+= and
Finally, it is noted that the Hamiltonian W=½ΣkΣr[Pr
2(k)+ωk
2Qr
2(k)] is precisely of the
same form as that for an assembly of simple harmonic oscillators whose Hamiltonians
are given by H=½(P2 +ω2Q2).
Each mode of radiation field is therefore formally equivalent to a single harmonic
oscillator when we let P2 =p2/m and Q2 =mq2 so that the Hamiltonian H=p2/2m+(m/2)ω2q2
became:
)ω(
2
1 222
QPH +=
156](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-156-320.jpg)
and a† =[1/√(2hω)](ωQ−iP)) we define:
which then must obey, the commutation rules:
In term of these operators the Hamiltonian W=½Σkr[Pr
2(k)+ωk
2Qr
2(k)] becomes:
0])(,)([])(,)([])(,)([ =′=′=′ ′ kkkkkk kk srsrsrsr PPQQiPQ andδδh
Quantization of the Radiation Field
])()(ω[
ω2
1
)(])()(ω[
ω2
1
)( †
kkkkkk k
k
k
k
rrrrrr PiQaPiQa −=+=
hh
and
0)](),([)](),([)](),([ ††
=′=′=′ ′ kkkkkk kk srsrsrsr aaaaaa andδδ
∑∑∑∑∑∑
+=
+==
k
k
k
k
k
kkkk
r
r
r
rr
r
r NaaHH
2
1
)(ω
2
1
)()(ω)( †
hh
in which Nr(k) is known as the number operator for the mode k and polarization r and is
given by:
)()()( †
kkk rrr aaN =
157](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-157-320.jpg)
![A complete description of the radiation field consists of an enumeration of the
occupation numbers nr(k). Since each mode is independent of the product of all
eigenstates, |nr(k)〉 is an eigenstate of the total field. A many-photon state is therefore
described by:
The notation is becoming quite cumbersome; we shall therefore make the replacement
nri
(ki) =ni so that the many-photon state is written as:
2017
MRT
With the orthogonality requirement:
for operators, we also replace the indices ri by the index i. The many-photon analog of
ar(k)|nr(k)〉=√ nr(k)|nr(k)−1〉, a†
r(k)|nr(k)〉=√[nr(k)+1]|nr(k)+1〉 and ar(k)|0〉=0 is:
with:
LLLL ),(,),(),()()()( 2121 2121 irrrirrr ii
nnnnnn kkkkkk =
∑
+=
i
ii nE
2
1
ωh
( ))(,,,, 21 irii i
nnnnn k≡LL
LLLLLL ii nnnnnnii nnnnnn ′′′=′′′ δδδ 2211
,,,,,,,, 2121
as well as ai|n1,n1,…,0,…〉=0, and the analog of Nr(k)|nr(k) 〉=nr(k) |nr(k)〉 is:
LLLL ,,,,,,,, 2121 iiii nnnnnnnN =
LLLLLLLL ,1,,,1,,,,,1,,,,,,, 2121
†
2121 ++=−= iiiiiiii nnnnnnnannnnnnna &
160](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-160-320.jpg)
![When nr(k)=0, there are no photons in the mode (k,r); nevertheless the energy in the
mode, according to E=ΣkΣr Er(k) =ΣkΣr hωk[nr(k)+½], is ½hωk. This value, which is
characteristic of the harmonic oscillator, is known as the zero point energy.
2017
MRT
Since observables associated with non-commutating operators are subject to the
uncertainty principle, an increase in precision in the number of photons means an
increase in the uncertainty in the fields.
For the radiation field as a whole with an infinite number of modes, the zero point
energy becomes infinite. This is one of the particularities of the quantum-mechanical
description of the radiation field. A formal explanation is based on the non-commutativity
of the number operator Nr(k) with the annihilation and creation operators ar(k) and a†
r(k)
as a result of which ΣkΣrNr(k) does not commute with the fields E and B.
When no photons are present, the fluctuations in the field strengths are responsible for
the infinite zero point energy. Fortunately, a consistent description of physical processes
in practically all cases is obtained by simply ignoring the infinite zero point energy of the
radiation field.
161](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-161-320.jpg)
![The transition from classical to quantum mechanics seen in [Qr(k),Ps(k′)]=ihδkk′δrs
and [Qr(k),Qs(k′)]=[Pr(k),Ps(k′)]=0 which implies that the classical vector potential
A(r,t)=ΣkΣr êr(k){Ar(k) exp[i(k•r−ωkt)]+Ar
* (k)exp[−i(k•r−ωkt)]} becomes a quantum-
mechanical operator.
2017
MRT
We now write the quantum-mechanical vector potential by substituting Ar(k) and Ar
*(k)
into A(r,t)=ΣkΣrêr(k){Ar(k) exp[i(k•r−ωkt)]+Ar
*(k)exp[−i(k•r−ωkt)]} and we get:
)(
ω
2π
)()(
ω
2π
)( †
2
*
2
kkkk
kk
rrrr a
V
c
Aa
V
c
A
hh
→→ and
∑∑ −•−−•
+=
k
rkrk
k
kk
kkkerA
r
ti
r
ti
rr aa
V
c
t ]e)(e)()[(ˆ
ω
2π
),( )ω(†)ω(
2
H
h
This transition may be accomplished by means of the transformations
Qr(k)=√(V/π)(1/2c)[Ar(k)+Ar
*(k)] and Pr(k)=−i√(V/π)(ωk/2c )[Ar(k)−Ar
*(k)] which relate
Ar(k) and Ar
*(k) to Qr(k) and Pr(k); the latter in turn are related to the annihilation and
creation operators ar(k) and ar
†(k), via ar(k)=[1/√(2hωk)][ωk Qr(k)+iPr(k)] and
ar
†(k)=[1/√(2hωk)][ωk Qr(k) −iPr(k)]. Hence the required replacement is:
which is spelled out in the Heisenberg representation. In the Schrödinger representation:
∑∑ •−•
+=
k
rkrk
k
kkkerA
r
i
r
i
rr aa
V
c
]e)(e)()[(ˆ
ω
2π
)( †
2
h
162](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-162-320.jpg)
![The electric and magnetic field operators may also be expressed in terms of ar(k) and
ar
†(k). Using our results for A(r) only, as well as Ar(k) and Ar
*(k) just obtained, we get:
Similarly, with this result for E(r) above, as well as Ar(k) and Ar
*(k) again, we get:
2017
MRT
∑∑ •−•
−=
k
rkrkk
kkkerE
r
i
r
i
rr aa
V
i ]e)(e)()[(ˆ
ω2π
)( †h
∑∑ •−•
−=
k
rkrkk
kkkekrB
r
i
r
i
rr aa
V
i ]e)(e)()][(ˆˆ[
ω2π
)( †
××××
h
163](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-163-320.jpg)
![The total Hamiltonian for a radiation field interacting with an atomic system may be
written as a sum of the three following terms:
Here:
2017
MRT
is the Hamiltonian for the free field. The Atomic Hamiltonian is:
where V contains whatever terms are necessary to define the atomic state, e.g.,
Coulomb interaction with the nucleus, Coulomb repulsion among electrons, spin-orbit
interaction external fields, etc. For HInteraction we refer to the Schrödinger equation
and pick out all terms which contain the vector potential.
nInteractioAtomicRadiation HHHH ++=
∑∑ +=
k
k kk
r
rr aahH ]½)()([ω †
Radiation
V
m
p
H
i
i
+
= ∑ 2
2
Atomic
ψϕϕψϕ
•−•−−•+
+=+′ pAAp ××××∇∇∇∇ΣΣΣΣ∇∇∇∇∇∇∇∇××××∇∇∇∇ΣΣΣΣ 2222
2
23
42
48822
1
)(
cm
e
cm
e
cm
p
mc
e
c
e
m
eE
hhh
164](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-164-320.jpg)
![The total Hamiltonian H=HRadiation +HAtomic +HInteraction may now be written as:
where:
∑∑∑∑ •−+•−
•=•=
k
rk
kk
rk
k
kpkekpke
r
i
rr
r
i
rr a
Vm
e
Ha
Vm
e
H e)(])(ˆ[
ω
2π
e)(])(ˆ[
ω
2π †)(
1
)(
1
hh
and
)(
1
)(
1
†
1 ]e)(e)(][)(ˆ[
ω
2π
+−
•−•
+=
+•= ∑∑
HH
aa
Vm
e
H
r
i
r
i
rr
k
rkrk
k
kkpke
h
With the use of the vector potential obtain A(r)=ΣkΣr êr (k){Ar (k)exp[i(k•r−ωkt)]+
Ar
*(k)exp[−i(k•r−ωkt)]} earlier:
in which H0 contains HRadiation and HAtomic and HInteraction is given by the expression derived
above.
We now assume that HInteraction can be regarded as a perturbation on H0 although, as in
previous cases, the justification is not apparent until the calculations have been
completed. We shall concentrate on the term, H1, of the perturbed Hamiltonian:
nInteractio0 HHH +=
Ap•=
cm
e
H1
which is most often the dominant term in electromagnetic interactions.
2017
MRT
166](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-166-320.jpg)
![For a single mode characterized by (k,r) or what amounts to the same thing, for
photons of momentum hk and polarization r, we have:
2017
MRT
in which |ψa〉 represents the initial atomic state and |ψb〉 represents the final atomic
state. In the same fashion, we have:
a
r
i
rb
r
ra
r
i
rrrbrarb
V
n
m
e
nan
Vm
e
nHn
ψψ
ψψψψ
∑∑
∑∑
•
•−
•=
•−=−
k
rk
k
k
rk
k
pke
k
kkpkekkk
e])(ˆ[
ω
)(2π
)(;e)(])(ˆ[1)(;
ω
2π
)(;1)(; )(
1
h
h
a
r
i
rb
r
ra
r
i
rrrbrarb
V
n
m
e
nan
Vm
e
nHn
ψψ
ψψψψ
∑∑
∑∑
•−
•−+
•
+
=
•+=+
k
rk
k
k
rk
k
pke
k
kkpkekkk
e])(ˆ[
ω
]1)([2π
)(;e)(])(ˆ[1)(;
ω
2π
)(;1)(; †)(
1
h
h
167](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-167-320.jpg)
![Matrix elements of the type:
maybesimplifiedif k•r<<1so that eik•r ≈1. Using the Atomic Hamiltonian HAtomic=Σi(pi
2/2m)
+V and the commutation law [ri,p2]=2ihpi (ri =x, y,z), we have [r,HAtomic] =(ih/m)p. Thus:
2017
MRT
For an atom, r is the position vector of an electron referred, most conveniently, to the
nucleus; Ea and Eb are the eigenvalues for the Atomic Hamiltonian corresponding to the
eigenstates |ψa〉 and |ψb〉, respectively; and ωab =ωk by energy conservation.
The approximation eik•r ≈1 in the vector potential corresponds to the approximation in
which all the terms in the multipole expansion of the field are neglected except for the
electric dipole (E1) term. Therefore within the electric dipole approximation:
and:
a
i
bra
i
rb ψψψψ rkrk
pkepke ••
•≡• e)(ˆe])(ˆ[
ab
abbaabababa
i
b
mi
miEE
mi
H
i
m
ψψ
ψψψψψψψψ
r
rrrp
k
rk
ω
ω)(],[e Atomic
=
=−==•
hh
abrabra
i
br im ψψψψψψ rkepkepke k
rk
•=•• •
)(ˆω)(ˆe)(ˆ and
abr
r
rarb
abr
r
rarb
V
n
ienHn
V
n
ienHn
ψψψψ
ψψψψ
rke
k
kk
rke
k
kk
•
+
=+
•=−
+
−
)(ˆ
]1)([2π
)(;1)(;
)(ˆ
)(2π
)(;1)(;
E1
)(
1
E1
)(
1
h
h
168](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-168-320.jpg)
](ˆˆ[
ω
)](ˆˆ[
2
ω
)](ˆ[
)()](ˆ[)]()(ˆ[)]()(ˆ[)()(ˆ
2
1
2
1
2
1
2
1
A
rrBrr
rrrr
e
m
i
e
m
i
c
i
i
iiiO
××××××××××××××××
××××
µ
h
SA
2
1
2
1
)()(ˆ)()(ˆ)(ˆ)]()(ˆ[
OO
iiii rrrr
+≡
•+•+•−•=••≡•• kprrpkekprrpkekrpkerkpke
LL
k
k
µkBµkekkk •=•−== −−
)()](ˆˆ)[(
ω2π
)(
ω
2π )(
A
)(
1 rrrr a
V
iOa
Vm
e
H ××××
hh
µB is the Bohr magneton and µµµµL (=µBL) the magnetic moment operator associated with
the orbital angular momentum L. The vector k××××êr (k) lies in the direction of the magnetic
field as is evidentfrom B(r)=iΣkΣr√(2πhωk/V )[k××××êr (k)][ar (k)exp(ik•r)−ar
†(k)exp(−ik•r)].
The connection with the magnetic field can be made more explicit by writing the
complete interaction asociated with OA. From our previous definition for H1
(−), we get:
ˆ
ˆ
where Br
(−)(k)is the term containingar(k) inB(r)=iΣkΣr√(...)[k××××êr(k)][ar(k)exp(ik•r)−...].ˆ
169](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-169-320.jpg)
(
)ˆˆˆ()ˆˆ()(
2
1
2
1
M1
)2(
zx
zyxzxx
pxpzki
pppxzki
−=
++•−=•=
kjieepA l
)()( 2
1
M1
)2(
zyy pypzki −=•=
pA l
which corresponds to the polarization vector êr(k) pointing in the direction of the y-axis
since then k××××êr(k) is in the direction of the negative x-axis giving:ˆ
)()()()](ˆˆ[ 2
1
2
1
2
1
A zyxr pypzkikikiO −=×−=ו= prprkek ××××
170](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-170-320.jpg)
![2017
MRT
in which µµµµJ is the total magnetic moment operator associated with both orbital and
spin angular momenta.
There is really no need to limit the magnetic moment operators to those which are
associated with the orbital angular momentum. We may as well include the spin which
will now take into amount the term proportional to ΣΣΣΣ•∇∇∇∇××××A in HInteraction. This amounts to
adding 2S to L in OA =−i(mωk/e)[k××××êr(k)]•µµµµL. Hence the matrix elementsformagnetic
(M1) transitions may now be written as:
ˆ
aBbr
r
abr
r
rarrbrarb
V
n
i
V
n
i
nnnHn
ψµψ
ψψ
ψψψψ
)2()](ˆˆ[
)(ω2π
)](ˆˆ[
)(2π
)(;)(1)(;)(;1)(; )(
M1
)(
1
SLkek
k
µkek
k
kkBµkkk
k
J
J
+•=
•−=
•−−=− −−
××××
××××
h
h
aBbr
r
abr
r
rarrbrarb
V
n
i
V
n
i
nnnHn
ψµψ
ψψ
ψψψψ
)2()](ˆˆ[
]1)([ω2π
)](ˆˆ[
]1)([2π
)(;)(1)(;)(;1)(; )(
M1
)(
1
SLkek
k
µkek
k
kkBµkkk
k
J
J
+•
+
−=
•
+
=
•−+=+ ++
××××
××××
h
h
and:
171](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-171-320.jpg)
![For the symmetric operator OS, we have:
The replacement of p according to [r,HAtomic] = (ih/m)p gives:
2017
MRT
and:
which is identified as an operator as being the electric quadrupole (E2).
krrkekrrke ••−=••−−= abr
ba
abrabab
m
EE
m
O ψψψψψψ )(ˆ
2
ω
)(ˆ)(
2
S
h
kprrpke •+•= )()(ˆ2
1
S riO
krrkekrrrrke ••=•+•−= ]),[)(ˆ
2
]),[],([)(ˆ
2
AtomicAtomicAtomicS H
m
HH
m
O rr
hh
jirQ δ2
2
1−= rr
which is an irreducible tensor of rank 2. We now have:
)(2
1
S zx pxpzkiO +=
kke
kkekke
k
k
ˆ)(ˆ
2
ω
)(ˆ
2
ω
)(ˆ
2
ω
2
S
••−=
••−=••−=
abr
abrabr
ba
ab
Q
c
m
Q
m
Q
m
O
ψψ
ψψψψψψ
The operator rr is a symmetric Cartesian tensor of rank 2. It is observed that
êr(k)•〈ψb|r2δij |ψa〉•k=〈ψb|r2δij |ψa〉êr(k)•k=0 as a consequence of the transversality
condition (i.e., ∇∇∇∇••••A=0 ⇒ êr (k)•k=0). Therefore rr may be replaced by:
ˆ ˆ
ˆ
The multipole character of OS =(m/2h)êr(k)•[rr,HAtomic] may be investigated:
172](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-172-320.jpg)
![The matrix elements for electric quadrupole (E2) transitions may now be written as:
2017
MRT
kke
k
kk k ˆ)(ˆ
)(ω2π
2
)(;1)(;
3
E2
)(
1 ••−=− −
abr
r
rarb Q
V
n
c
e
nHn ψψψψ
h
and:
kke
k
kk k ˆ)(ˆ
]1)([ω2π
2
)(;1)(;
3
E2
)(
1 ••
+
=+ +
abr
r
rarb Q
V
n
c
e
nHn ψψψψ
h
The discussion up to this point involved the interaction of a radiation field with a single
electron. We shall now summarize the important expressions and put them in the form
applicable to a multi-electron system. However, we shall continue to use a single mode
characterized by (k,r). For a system containing N electrons let:
where rj is the position vector of the j-th electron. For an atom the natural reference is
the nucleus; more generally, as in molecules, rj is referred to the center of gravity of the
positive charge.
∑=
=
N
j
j
1
rR
173](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-173-320.jpg)
![The interaction matrix elements are then the following:
2017
MRT
kke
k
kk
kke
k
kk
k
k
ˆ)(ˆ
]1)([ω2π
2
)(;1)(;
ˆ)(ˆ
)(ω2π
2
)(;1)(;
E2
3
E2
)(
1
3
E2
)(
1
••
+
=+
••−=−
∑
∑
+
−
a
j
jbr
r
rarb
a
j
jbr
r
rarb
Q
V
n
c
e
nHn
Q
V
n
c
e
nHn
ψψψψ
ψψψψ
h
h
:)(QuadrupoleElectric
a
j
j
br
r
rarb
a
j
j
br
r
rarb
V
n
inHn
V
n
inHn
ψψψψ
ψψψψ
∑
∑
•
+
=+
•−=−
+
−
J
J
µkek
k
kk
µkek
k
kk
)](ˆˆ[
]1)([2π
)(;1)(;
)](ˆˆ[
)(2π
)(;1)(;
M1
M1
)(
1
M1
)(
1
××××
××××
h
h
:)(DipoleMagnetic
abr
r
rarb
abr
r
rarb
V
n
ienHn
V
n
ienHn
ψψψψ
ψψψψ
Rke
k
kk
Rke
k
kk
•
+
=+
•=−
+
−
)(ˆ
]1)([2π
)(;1)(;
)(ˆ
)(2π
)(;1)(;
E1
E1
)(
1
E1
)(
1
h
h
:)(DipoleElectric
174](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-174-320.jpg)
![In the description of absoption and emission processes in atoms it is often permissible to
replace the totality of atomic states by just two discrete states between which the
transition occurs (see Figure – zero point energy has been omitted).
2017
MRT
Such a simplification is possible if the energy separation between two states of an
atom correspond to the photon energy hω whereas all other levels are spaced so that
there are no energy differences close to hω. Assuming this to be the case we consider
an atom in the state |ψa〉 interacting with a radiation field described by |nr (k)〉. The initial
state of the entire system, i.e., atom plus field, is |ψA〉=|ψa;nr (k)〉. If absorption takes
place, the atom makes a transition to the state |ψb〉 and there is one photon fewer in the
field. Hence the final state of the system is |ψB〉=|ψb;nr (k)−1〉.* Thus:
Transition Probabilities
|ψb 〉
ABSORPTION EMISSION
|ψa 〉
|ψb 〉
|ψa 〉
|ψa 〉
|ψb 〉
|ψa 〉
|ψb 〉
|ψA〉 = |ψa ; n〉
EA = Ea + nhω
|ψB〉 = |ψb ; n – 1〉
EB = Eb + (n – 1)hω
|ψB〉 = |ψb ; n + 1〉
EB = Eb + (n + 1)hω
|ψA〉 = |ψa ; n〉
EA = Ea + nhω
* In this notation reference to other atomic states or other photons is suppressed since they are not involved in the physical
process.
( )
( )
)ωω(ω
ω]½)([1)(;
ω]½)([)(;
kk
k
k
kk
kk
−=−−=−
−+=−=
++==
baabAB
rbBrbB
raAraA
EEEE
nEEn
nEEn
hh
h
h
ψψ
ψψ
in which Ea and Eb are the energies of the initial and final atomic states, respectively,and:
abba EE −=ωh
175](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-175-320.jpg)
![If it is stipulated that the Hamiltonian is independent of time, as will henceforth be
assumed, a formal solution to the Schrödinger equation ih∂tΨΨΨΨ(r,t)=HΨΨΨΨ(r,t) is:
in which the exponential operator is interpreted as:
2017
MRT
Comparing ψ(t)=U(t,to)ψ(to) with ΨΨΨΨ(r,t)=exp[iHo(t−to)/h]ΨΨΨΨ(r,to), it is seen that an
explicit form for the evolution operator is:
Another formulation for the evolution operator is obtained by converting ih∂tU(t,to)=
HU(t,to) and condition U(to,to)=1 into the integral equation:
)(
o
o
e),(
ttH
i
ttU
−−
= h
),(e),( o
)( o
tt
ttH
i
rr ΨΨΨΨΨΨΨΨ
−−
= h
∫ ′′−=
t
t
tdttUH
i
ttU
o
),(1),( oo
h
K
hh
h +−
+−+=
−−
2
o
2
2
o
)(
)(
1
!2
1
)(
1
1e
o
ttH
i
ttH
i
ttH
i
177](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-177-320.jpg)
![We now consider the formal interaction representation which is particularly appropriate
for certain formulation of time-dependent perturbation theory.
2017
MRT
and an operator AI(t) in the same representation is given by:
The time derivative of ψI(t) is:
This is an equation of motion for the wave function in the interaction representation
while the other equation of motion is for the operators AI(t):
)(e)(
o
I tt
tH
i
ψψ h=
tH
i
tH
i
tAtA
oo
e)(e)(I
hh
−
=
t
t
tH
i
t
t tH
i
tH
i
∂
∂
+=
∂
∂ )(
e)(e
)( oo
o
I ψ
ψ
ψ hh
h
and upon substituting ih∂ψ/∂t=Hψ =(Ho +V)ψ, we find:
)()()(eee)(e
)(
II
I oooo
ttVtVtV
t
t
i
tH
i
tH
i
tH
i
tH
i
ψψψ
ψ
===
∂
∂ −
hhhhh
]),([
)(
oI
I
HtA
t
tA
i =
∂
∂
h
Let the time-independent Hamiltonian H in the Schrödinger representation be written
as the sum of two parts Ho +V and a wave function ψI(t) in the interaction representation
is defined by:
182](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-182-320.jpg)
![We now define an operator UI(t,to) by:
with:
Upon combining ψ(t)=U(t,to)ψ(to) with ψI(t)=exp(iHot/h)ψ(t) one finds:
2017
MRT
from which it is concluded that:
with the second equality based on U(t,to)=exp[−iH(t−to)/h].
The unitarity property of U(t,to) confers unitarity of UI(t,to) so that:
)(),()( oIoII tttUt ψψ =
ooooooo
eeee),(e),(
)(
ooI
tH
i
ttH
i
tH
i
tH
i
tH
i
ttUttU hhhhh
−−−−
==
1),( ooI =ttU
)(e),(e)(),(e)(e)( oIoooI
ooooo
tttUtttUtt
tH
i
tH
i
tH
i
tH
i
ψψψψ hhhh
−
===
),(),( o
1
Io
†
I ttUttU −
=
We may now also find the differential equation obeyed by UI(t,to) by substituting ψI(t)=
UI(t,to)ψI(to) into ih∂ψI(t)/∂t=VI(t)ψI(t). Since ψI(to) is constant:
),()(
),(
oII
oI
ttUtV
t
ttU
i =
∂
∂
h
183](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-183-320.jpg)
![We shall now let:
and if we specialize to the case of a transition between the stationary states ϕa and ϕb,
the transition probability is:
The equality between the two forms in the above for wba arises as a result of UI(t,to)=
exp(iHot/h)U(t,to)exp(−iHoto/h) which merely introduces a phase factor into the matrix
elements when U(t,to) is replaced by UI(t,to). Since the probability is determined by the
absolute square of the matrix elements, such phase factors are of no consequence. We
shall use the form |〈ϕb|UI(t,to)|ϕa〉|2 to evaluate the probability per unit time:
2017
MRT
Since the time dependence of 〈ϕb|UI(t,to)|ϕa〉 is contained entirely in the operator UI(t,to)
which satisfies ih∂UI(t,to)/∂t=VI(t)UI(t,to), we have:
bbbaaa EHEHVHH ϕϕϕϕ ==+= ooo and,
2
oI
2
o ),(),( ababba ttUttUW ϕϕϕϕ ==
]),(),([),(
*
oIoI
2
oI abababba ttUttU
td
d
ttU
td
d
W ϕϕϕϕϕϕ ==
]),(),()(Im[
2
]),(),()([
]),()(),(),(),()([
*
oIoII
oIoII
*
oIIoI
*
oIoII
abab
abab
ababababba
ttUttUtV
ttUttUtV
i
ttUtVttUttUttUtV
i
W
ϕϕϕϕ
ϕϕϕϕ
ϕϕϕϕϕϕϕϕ
h
h
h
=
−−=
−=
conjugatecomplex
184](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-184-320.jpg)
![We shall now insert(i.e.,using AI(t)=exp(iHot/h)A(t)exp(−iHot/h) and UI(t,to)=exp(iHot/h)
×exp[−iH(t–to)/h]exp(−iHoto/h)) VI(t)=exp(iHot/h)Vexp(−iHot/h) andUI(t,to)=UI(t,0)UI(0,to)=
exp(iHot/h)exp(−iHt/h)UI(0,to) into Wba =(2/h)Im[〈ϕb|VI(t)UI(t,to)|ϕa〉〈ϕb|UI(t,to)|ϕa〉*] to
obtain:
Up to this point to was arbitrary; it will now be assumed that to →−∞. This will allow us
to apply the perturbation V in adiabatically by which it is meant that V is multiplied by the
convergence factor exp(−ε|t|/h) (ε >0). This is a convenient mathematical device and has
the effect of causing the perturbation to vanish at very early and very late times but to
remain unaffected at t=0. Therefore, with ψa=UI(0,−∞)ϕa an eigenfunction of H with
eigenvalue Ea we get:
2017
MRT
which is the reaction matrix.
with:
abba VR ψϕ=
=
−−
*
oIoI ),0(ee),0(eeIm
2 oo
a
tH
i
tH
i
ba
tH
i
tH
i
bba tUtUVW ϕϕϕϕ hhhh
h
]Im[
2
]Im[
2
eeeeIm
2
*
**
abba
abab
tE
i
ab
tE
i
tE
i
ab
tE
i
ba
R
VVW
aaaa
ψϕ
ψϕψϕψϕψϕ
h
hh
hhhh
=
=
=
−−
185](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-185-320.jpg)
![We shall now replace ψa in Wba =(2/h)Im[Rba〈ϕb|ψa〉*] by the Lippmann-Schwinger
equation (stated here without proof):
where we use the notation ψI(−∞)=ϕa and ψI(0)=ψa with the assumption that VI(−∞)=0
and Hoϕa =Eaϕa. By the way, this permits us to identifyUI(0,−∞)=1+limε →0[1/(Ea−H+iε)]V.
We then get:
2017
MRT
so that the final result is the Fermi Golden Rule:
Now:
)(
π2 2
bababa EERW −= δ
h
a
a
aa V
iHE
ψ
ε
ϕψ
ε ++
+=
→ o0
1
lim
++
+==
→
*
o0
** 1
limIm
2
]Im[
2
a
a
bbaabbaabbaba V
iHE
RRRW ψ
ε
ϕϕϕψϕ
εhh
Assuming ϕa ≠ϕb, the first term in the brackets vanishes and:
++
=
++
=
→→ εε
ψϕ
εε iEE
R
iEE
V
RW
ba
ba
ba
ab
baba
2
0
*
0
limIm
2
limIm
2
hh
)(π
)(
lim
)(
1
Im 22022 ba
bababa
EE
EEEEiEE
−=
+−+−
=
++ →
δ
ε
ε
ε
ε
ε ε
and
186](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-186-320.jpg)
![Physically it is more meaningful to assume a distribution of modes within a narrow
range of frequencies. For a radiation field in a cubical enclosure, according to dN=
[V/(2π)3]k2dkdΩ, the number of modes per unit energy interval is:
2017
MRT
We shall therefore replace the infinitely sharp density distribution δ(Eb −Ea −hωk) in
WAbsorption by (1/h)dN/dωk above to obtain:
Here α =e2/hc is the fine structure constant and quantities such as ωk and nr(k) must be
understood as averages within the interval.
For emission, the calculation goes through in exactly the same way but with the matrix
elements:
Ω= d
c
V
d
dN 2
33
ω
)π2(ω
1
k
k hh
Ω•= d
c
n
dW abr
r 2
2
3
Absorption )(ˆ
π2
)(ω
ψψ
α
Rke
kk
abr
r
rarb
V
n
ienHn ψψψψ Rke
k
kk •
+
=+ +
)(ˆ
]1)([2π
)(;1)(; E1
)(
1
h
The final result, analogous to that in dWAbsorption above is:
Ω•
+
= d
c
n
dW abr
r 2
2
3
Emission )(ˆ
π2
]1)([ω
ψψ
α
Rke
kk
188](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-188-320.jpg)
![If W(b,a) represents the transition probability per unit time from the state |αa;Ja,Ma〉
with degeneracy ga to the state with degeneracy gb where αa and αb represent the
quantum numbers required to complete the specification of the states |ψa 〉 and |ψb〉,
respectively, we shall have, in place of WAbsorption =(4αω3n/3c2)|〈ψb|R|ψa〉|2 and WEmission =
[4αω3(n+1)/3c2)]|〈ψb|R|ψa〉|2, we have:
2017
MRT
In these expressions the probabilities for all possible transitions |αa;Ja,Ma〉→|αb;Jb,Mb〉
have been added, but since the probability of an electron being in any one of the initial
states |αa;Ja,Ma〉 is 1/ga, the sum of the probabilities must be multiplied by the factor.
WInduced and WSpontaneous are correspondingly modified. Note that:
where W(b,a) stands for either WAbsorption(b,a) or WEmission(b,a) and the same for W(a,b).
),(),( abW
g
g
baW
b
a
=
∑∑
∑∑
+
=
=
a b
a b
M M
aaabbb
a
M M
aaabbb
a
MJMJ
c
n
g
abW
MJMJ
c
n
g
abW
2
2
3
Emission
2
2
3
Absorption
,;,;
3
)1(ω41
),(
,;,;
3
ω41
),(
αα
α
αα
α
R
R
191](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-191-320.jpg)
![Let us now suppose that the population (atoms per cm3) in |ψa 〉 and |ψb〉 are Na and Nb,
respectively. When the system is in equilibrium, the number of transitions per unit time
|ψa 〉→|ψb〉 will be equal to the number of transitions per unit time |ψb〉→|ψa〉 or:
where a refers to the lower state and b to the upper state (see Figure – where the
radiative transitions between two levels are shown).
2017
MRT
In terms of the Einstein coefficients:
)],(),([),( StimulatedInducedAbsorption baWbaWNabWN ba +=
Planck’s Law
|ψ a〉
|ψ b〉
WSponteneous(a,b) WInduced(a,b) WAbsorption(b,a)
)],()ω(),([)ω(),( baAIbaBNIabBN ba +=
But:
),(
ω
π
),(),(
ω
π
),( 3
32
3
32
baA
g
gc
abBbaA
c
baB
a
b
hh
== and
195](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-195-320.jpg)
![Also, the equilibrium population satisfies the Boltzmann distribution*:
where Ei is the separation in energy between the states – in this case |ψa〉 and |ψb〉:
2017
MRT
This is known as Planck’s distribution law, where I(ω)dω is the energy per unit volume
(under conditions of thermodynamic equilibrium) for photons in the interval ω to ω+dω.
Tk
E
b
a
b
a B
i
g
g
N
N
e=
1e
1
π
ω
)ω( ω32
3
−
= TkB
c
I h
h
Note that Planck’s law is independent of degeneracies (i.e., ga or gb).
* In chemistry, physics, and mathematics, the Boltzmann distribution is a certain distribution function (or probability measure) for the
distribution of the states of a system. It underpins the concept of the canonical ensemble, providing its underlying distribution. A
special case of the Boltzmann distribution, used for describing the velocities of particles of a gas, is the Maxwell–Boltzmann (M-B)
distribution. So, the Boltzmann distribution for the fractional number of particles Ni /N occupying a set of states i possessing energy Ei
is Ni /N = [gi exp(−Ei /kB T )]/Z(T) where kB is the Boltzmann constant, T is the temperature, gi is the degeneracy (i.e., the number of
levels having energy Ei), N = ΣiNi is the total number of particles and Z(T) = Σigi exp [−Ei/kB T ] is the partition function which encodes
the statistical properties of a system in thermodynamic equilibrium. Alternatively, for a single system at a well-defined temperature, it
gives the probability that the system is in the specified state. The Boltzmann distribution applies only to particles at a high enough
temperature and low enough density that quantum effects can be ignored, and the particles are obeying M-B statistics. When the
energy is simply the kinetic energy of the particle Ei = ½mv2 then the distribution correctly gives the M-B distribution of gas
molecule speeds, previously predicted by James C. Maxwell (1831-1879) in 1859. [Source: Wikipedia] To study this subject
(e.g., canonical ensembles, partition functions, etc.) in its entirety see Feynman, R., Statistical Mechanics (Perseus Books).
Inserting these relations into NaB(b,a)I(ω)=Nb[B(a,b)I(ω)+A(a,b)], we obtain:
ωh=−⇒ abi EEE
196](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-196-320.jpg)
![For photon absorption it is necessary to evaluate the matrix elements:
o
o
eee)(ˆ
π
eee)(ˆ
π
1
)(ˆe
2/3
o
2/3
o
s1Free
arZii
r
arZii
rir
i
f
Va
Z
Va
Z
−••
−•••
•
=
•
=•
rkrq
rkrqrk
qke
pkepke
h
ψψ
Coordinate system used in the
calculations.
qkK −−−−=
Defining:
The relationship among the various vectors is shown in the Figure; it is noted
that:
in spherical coordinates. If the coordinate system is oriented so that the z-axis
is along the direction of K and the polarization vector ê along the x-axis:
we have:
∫∫∫ ′′== •
∞
−−•−••
π
00
2
sineeπ2eeeee ooo
θθ drdrd iarZarZiarZii rKrKrkrq
r
222
o
2
3
o
0
π
0 )(
π4
sine
π4
eeesin
2
sine oo
KaZ
aZ
drKrr
K
Kr
rK
d arZarZiii
+
===′′
∫∫
∞
−−••• rkrqrK
andθθ
θ
ϕθ
cos2)(
cossin)(ˆ
2222
qk
qr
−+==
=•
qkqkK
qke
−−−−
2017
MRT
Thus:
])(ˆ[
)(
)(π8
e 222
o
2
2/3
o
qkerk
•
+
=•
ri
i
f
KaZV
ZZa h
ψψ
z
y
x
ê
θ
k
ϕ q
K
e
z
y
x
r
e−
Ze+
o
e
π
1
2/3
o
arZ
i
a
Z −
=ψ
rq•
= i
f
V
e
1
ψ
1s
200](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-200-320.jpg)
![It will be assumed that the photon energy is large compared with the binding energy of
the electron in the atom but small compared to the rest mass of the electron. The first
assumption ensures that the final state is that of a free electron and the second avoids
the necessity of a relativistic treatment. Fortunately both conditions are satisfied when:
The first factor on the right is of the order of the binding energy I and the second factor is
2⋅137/Z; hence E is at least several times larger than I. With the assumption that E=I,
the photons energy becomes comparable to thekineticenergyof the electron hck=mov2/2
from which itfollowsthat k/q=v/2c=Z/qao orqao=Z and Z2 +ao
2K2 =Z2 +ao
2(k2 +q2 −2qkcosθ)
≈q2ao
2(1−β cosθ) (with β =v/c). Therefore the matrix element is:
where k=|k| is the photon wave number, ao the Bohr radius, and Z the atomic number of
the atom. To see the justification of this criterion we first calculate the photon energy
corresponding to kao ≈ Z:
and the complete interaction matrix element is:
22
o
2
2/3
o
)]cos1([
cossin)(π8
)(ˆe
θβ
ϕθ
ψψ
−
=••
aqV
qZZa
ir
i
f
h
pkerk
2017
MRT
Zak ≈o
=== 22
4
o
2
o
2
2 eZ
cemZ
a
Zc
kcE
h
h
h
h
ir
i
f
V
n
m
e
ψψ pkerk
••
)(ˆe
ω
π2
o
h
201](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-201-320.jpg)
![Higher Order Electromagnetic Interactions
The process of absorption and emission (e.g., the photoelectric absorption Wn(k,q;θ,ϕ))
described earlier are one-photon processes since in every transition the field either loses
or gains a photon while the atom undergoes a corresponding change of state to keep
the total energy (atom plus field) constant.
In the absence of an external magnetic field the Hamiltonian describing the interaction
of an electron with a radiation field is:
There are other important interactions between an atom and a radiation field which
involve a change of more than one photon. In a general scattering event, for example,
the field loses an incident photon characterized by kr (e.g.,aspart of the sums Σk and Σr
seen earlier) and gains another photon – the scattered one with momentum k′ –
characterized by k′s. With laser sources, numerous multiphoton processes have been
observed although the present discussion will be restricted to several aspects of the
two-photon interaction.
where A is given by A(r)=ΣkΣr√(2πhc2/V ωk)êr(k)[ar(k)exp(ik•r)−ar
†(k)exp(−ik•r)] and
the H3 term is neglected.
321
2
2
2
nInteractio
22
HHH
mc
e
cm
e
cm
e
H
++=
•++•= AσAAp ××××∇∇∇∇
h
2017
MRT
203](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-203-320.jpg)
![As we’ve done before, we let:
For H2, we have:
in which Hkr
(+)Hk′s
(+) refers to the term containing a†
kr a†
k′s; Hkr
(−)Hk′s
(−) to the term with
ar (k)as(k′), etc.
where:
2017
MRT
{
}
)()()()()()()()(
)(†)(†
)()(††
22
††
22
2
e)()(e)()(
e)()(e)()(
ωω
)(ˆ)(ˆπ
]e)(e)(][e)(e)()][(ˆ)(ˆ[
ωω
1π
+
′
−−
′
+−
′
−+
′
+
•′−•′−−
•′+•′+−
′
•′−•′•−•
′
+++=
′+′+
′+′
′•
=
′+′+′•=
∑∑
∑∑
srsrsrsr
i
sr
i
sr
r
i
sr
i
sr
sr
r
i
s
i
s
i
r
i
rsr
HHHHHHHH
aaaa
aaaa
mV
e
aaaa
mV
e
H
kkkkkkkk
rkkrkk
k
rkkrkk
kk
k
rkrkrkrk
kk
kkkk
kkkk
keke
kkkkkeke
h
h
∑∑∑∑ •−+•−
•=•=
k
rk
kk
rk
k
kpkekpke
r
i
rr
r
i
rr a
Vm
e
Ha
Vm
e
H e)(])(ˆ[
ω
2π
e)(])(ˆ[
ω
2π †)(
1
)(
1
hh
&
)(
1
)(
1
†
1 ]e)(e)(][)(ˆ[
ω
2π
+−
•−•
+=
+•= ∑∑
HH
aa
Vm
e
H
r
i
r
i
rr
k
rkrk
k
kkpke
h
204](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-204-320.jpg)
![Matrix elements of ar (k) and ar
†(k) may be obtained from ar (k)|nr (k)〉=√ nr (k)|nr (k)−1〉
and ar
†(k)|nr (k)〉=√[nr (k)+1]|nr (k)+1〉:
which leads directly to:
Since the photon modes are independent we also have:
The only non-vanishing matrix elements of ar
†(k) and ar (k) are those which involve a
change of one photon. For all bilinear combinations of ar
†(k) and ar (k) the change in the
number of photons must be zero or two.
)()()(1)(1)()()(1)( †
kkkkkkkk rrrrrrrr nnannnan =−+=+ and
)()()(),()()(1)(,1)(
]1)()[()(),()()(1)(,1)(
)(]1)([)(),()()(1)(,1)(
]1)(][1)([)(),()()(1)(,1)(
†
†
††
kkkkkkkk
kkkkkkkk
kkkkkkkk
kkkkkkkk
′=′′−′−
+′=′′+′−
′+=′′−′+
+′+=′′+′+
srsrsrsr
srsrsrsr
srsrsrsr
srsrsrsr
nnnnaann
nnnnaann
nnnnaann
nnnnaann
0)](),([)](),([)](),([ †††
=′=′=′ kkkkkk srsrsr aaaaaa
2017
MRT
205](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-205-320.jpg)
![We consider a two-level system (see Figure).
)(),(;I kk ′= sri nnψ
Also scattering has occurred, ns (k′) is increased by one photon and nr (k) is decreased
by one photon so that the total system is now in the state |F 〉:
with energy (N.B., not including the zero point energy):
ssrri nnEE kk kk ′′++= ω)(ω)(I hh
with energy:
2017
MRT
1)(,1)(;F +′−= kk srf nnψ
ssrrf nnEE kk kk ′+′+−+= ω]1)([ω]1)([F hh
The energy Ei relative to Ef is arbitrary and the scattered photon may have more of
less energy compared with the incident photon. This represents the general case of
Raman scattering and includes elastic scattering as a special case.
Initially the atom is in the state |ψi 〉 and two photon modes with occupation numbers
nr (k) and ns (k′) (i.e., simultaneously observable – through momentums k and k′ photon
eigenvalues of the occupation numbers) are present. The total system is in the state |I 〉:
|ψf〉
SCATTERING
|ψi〉
|ψf〉
|ψi〉
| I 〉 = |ψi ; nr(k),ns(k′)〉 | F 〉 = |ψf ; nr(k)−1,ns(k′) +1〉
206](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-206-320.jpg)
![From 〈nr (k)+1,ns (k′)+1|ar
†(k)as
†(k′)|nr (k),ns (k′) 〉=√{[nr (k)+1][ns (k′)+1]},
〈nr (k)+1,ns (k′)−1|ar
†(k)as
†(k′)|nr (k),ns (k′)〉=√{[nr (k)+ 1]ns (k′)}, and also
〈nr (k)−1,ns (k′)+1|ar(k)as
†(k′)|nr (k),ns (k′)〉=√{nr (k)[ns (k′)+1]} and finally with
〈nr (k)−1,ns (k′)−1|ar(k)as(k′)|nr (k),ns (k′)〉=√[nr (k)ns (k′)], it follows that:
i
i
fsr
sr
srsr
srsr
nn
mV
e
HHHH
HHHH
ψψ rkk
kk
keke
kk
kkkk
kkkk
•′−
′
+−−+
−−++
′•
+′
=′=′
=′=′
)(
2
)()()()(
)()()()(
e)](ˆ)(ˆ[
ωω
]1)()[(π
I)()(FI)()(F
0I)()(FI)()(F
h
The scattering process is regarded as a two-photon process in the sense that an inci-
dent photon of specified energy, polarization, and propagation direction symbolized by
the indices kr (i.e., a discrete sum for each index) is scattered so that the emerging pho-
ton may have a different energy, polarization, and direction of propagation symbolized by
the indices k′s. Hence, a kr photon has been lost and a k′s photon has been gained!
Therefore:
2017
MRT
i
i
fsr
sr nn
mV
e
H ψψ rkk
kk
keke
kk •′−
′
′•
+′
= )(
2
2 e)](ˆ)(ˆ[
ωω
]1)()[(2π
IF
h
Matrix elements of H1 will be of type ar(k)|nr(k)〉=√nr(k)|nr(k)−1〉 and ar
†(k)|nr(k)〉=
√[nr(k)+1]|nr(k)+1〉 in which where is a chance of one photon. Therefore H1 cannot
contribute to scattering in the first order of perturbation theory but may contribute to
higher order. The matrix elements of H2 are non-vanishing when there is a change
of two photons; first order contributions from H2 are therefore expected.
207](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-207-320.jpg)
![We saw earlier that H1
(−) was associated with the absorption of a single photon and
H1
(+) with the emission of a single photon. Therefore the matrix elements in the two paths
are the following:
2017
MRT
ir
i
lls
i
f
sr
srisrl
srlsrf
nn
Vm
e
nnHnn
nnHnnHLLH
L
ψψψψ
ψψ
ψψ
])(ˆ[e])(ˆ[e
ωω
]1)()[(2π
)(),(;)(,1)(;
)(,1)(;1)(,1)(;IF
FI
11
1
1
2
2
)(
1
)(
1
)(
111
)(
1
1
pkepke
kk
kkkk
kkkk
rkrk
kk
••′
+′
=
′′−×
′−+′−=
→→
••′−
′
+
+−+
h
For
is
i
llr
i
f
sr
srisrl
srlsrf
nn
Vm
e
nnHnn
nnHnnHLLH
L
ψψψψ
ψψ
ψψ
])(ˆ[e])(ˆ[e
ωω
]1)()[(2π
)(),(;1)(),(;
1)(),(;1)(,1)(;IF
FI
22
2
2
2
2
)(
1
)(
1
)(
122
)(
1
2
pkepke
kk
kkkk
kkkk
rkrk
kk
•′•
+′
=
′+′×
+′+′−=
→→
•′−•
′
+
−+−
h
For
210](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-210-320.jpg)
![Both paths contribute to the second-order term in 〈ψk| R|ψm〉=〈ψk |V |ψm〉
+Σl[〈ψk|V |ψl〉〈ψl|V |ψm〉/(Em−El)]. On setting:
the second-order term becomes:
kk ′−−=−+−=− ωω 2211 II hh liLliL EEEEEEEE and
in which the general summation index l indicates the sum over all intermediate states
accessible to one-photon transitions.
2017
MRT
−−
•′•
+
+−
••′+′
′
•′−•
••′−
′
∑
k
rkrk
k
rkrk
kk
pkepke
pkepkekk
ω
])(ˆ[e])(ˆ[e
ω
])(ˆ[e])(ˆ[e
ωω
]1)()[(2π
2
22
1
11
2
2
h
h
h
li
is
i
llr
i
f
l li
ir
i
lls
i
fsr
EE
EE
nn
Vm
e
ψψψψ
ψψψψ
211](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-211-320.jpg)
(ˆ[
ωω
]1)()[(2π
)(),(;1)(,1)(;
2
nInteractio
hh
h
li
isllrf
l li
irllsf
ifsr
sr
srisrf
EEEEm
nn
mV
e
nnHnn
ψψψψψψψψ
δψψ
−−
•′•
+
+−
••′+′
′
•′−•
••′−
′
∑
k
rkrk
k
rkrk
kk
pkepke
pkepkekk
ω
])(ˆ[e])(ˆ[e
ω
])(ˆ[e])(ˆ[e
ωω
]1)()[(2π
2
22
1
11
2
2
h
h
h
li
is
i
llr
i
f
l li
ir
i
lls
i
fsr
EE
EE
nn
Vm
e
ψψψψ
ψψψψ
i
i
fsr
sr nn
mV
e
H ψψ rkk
kk
keke
kk •′−
′
′•
+′
= )(
2
2 e)](ˆ)(ˆ[
ωω
]1)()[(2π
IF
h
212](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-212-320.jpg)
(ˆ[]1)()[(
ω
ω
−−
•′•
+
+−
••′
+
+′•+′Ω
=
′
′
∑ kk
k
k
pkepkepkepke
kekekk
hh li
isllrf
l li
irllsf
ifsrsr
EEEEm
nnd
V
c
mc
e
W
ψψψψψψψψ
δ
The intermediate states |ψl〉 which appear in theKramers-Heisenbergdispersionformu-
la,aswellastheinitial state |ψi〉 and the final state |ψf 〉 arealleigenstates of theatom, i.e.,
solutions of the atomic Schrödinger equation. By virtue of the existence of matrix ele-
ments between |ψi〉 and |ψl〉 and |ψl〉 and |ψf〉, the two states |ψi〉 and |ψf〉 can interact
via two-photon processes. However, the transition |ψi〉→|ψl 〉 and |ψl 〉→| ψf 〉 are not
observable – the states |ψl〉 are sometimes called “virtual” because of this feature.
213](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-213-320.jpg)
(ˆ[]1)([
ω
ω
)(
−−
•′•
+
+−
••′
+
+′•+′=
Ω
=
′
=
Ω
′
′
∑ kk
k
k
pkepkepkepke
kekek
k
k
k
hh li
ilslfr
l li
ilrlfs
ifsrs
r
EEEEm
nr
Vcn
dW
r
s
d
d
ψψψψψψψψ
δ
σ
2
cm-secscattered/photonsincidentofnumber
sr-secscattered/photonsofnumber
electrontheofradiusclassical== 2
2
o
mc
e
r
The formula above for the differential cross section contains the factor [ns(k′′′′)+1];
hence the cross section is a sum of two terms one of which is proportional to ns (k′′′′), the
number of scattered photons. This term corresponds to stimulated Raman scattering;
it contributes negligibly at ordinary intensities, but produces important effects at high
intensities.
214](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-214-320.jpg)
![2017
MRT
ir
i
f
r
if
rf
ri
V
n
m
e
HHR
n
n
ψψ
ψ
ψ
])(ˆ[e
ω
)(π2
IFIF
1)(;F
)(;I
)(
11
)1(
pke
k
k
k
rk
k
•===
−=
=
•− h
Starting with the Fermi Golden Rule Wkm =(2π/h)|〈ψk|R|ψm〉|2δ(Ek −Em) in which the
general form of the matrix elements is 〈ψk |R|ψm〉≡Rkm = Rkm
(1)+ Rkm
(2) +…and it is possible
to symbolize these expressions by means of diagrams which are helpful in writing the
pertinent matrix elements associated with a particular process:
Single photon emission:
ir
i
f
r
if
rf
ri
V
n
m
e
HHR
n
n
ψψ
ψ
ψ
])(ˆ[e
ω
]1)([π2
IFIF
1)(;F
)(;I
)(
11
)1(
pke
k
k
k
rk
k
•
+
===
−=
=
•−+ h
k
| I 〉 | F 〉
k
| I 〉 | F 〉
Single photon absorption:
215](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-215-320.jpg)
![Processes involving two photons (second order processes) are scattering, two-photon
absorption and two-photon emission. Scattering has already been discussed earlier (the
pertinent diagrams were shown 7 slides ago):
2017
MRT
Two-photon absorption:
∑
∑
∑∑
′
•′•
′
••′
′
−−−−
+−
•′•′
=
+−
••′′
=
+≡
−
+
−
=
−′=−′−=
′−=′=
2 2
22
1 1
11
2 21 1
2
1
ω
])(ˆ[e])(ˆ[e
ωω
)()(π2
ω
])(ˆ[e])(ˆ[e
ωω
)()(π2
IFIF
1)(),(;1)(,1)(;F
)(,1)(;)(),(;I
2
2
4
2
2
3
43
I
)(
122
)(
1
I
)(
111
)(
1)2(
2
1
l li
is
i
llr
i
fsr
l li
ir
i
lls
i
fsr
L LL L
if
srlsrf
srlsri
EE
nn
Vm
e
M
EE
nn
Vm
e
M
MM
EE
HLLH
EE
HLLH
R
nnLnn
nnLnn
k
rkrk
kk
k
rkrk
kk
pkepkekk
pkepkekk
kkkk
kkkk
h
h
h
h
ψψψψ
ψψψψ
ψψ
ψψ
| I 〉 | F 〉| L2 〉
k′ k
where
| I 〉 | F 〉| L1 〉
k k′
216](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-216-320.jpg)
![Two-photon emission:
2017
MRT
∑
∑
∑∑
′
•′−•−
′
•−•′−
′
++++
−−
•′+′+
=
−−
••′+′+
=
+≡
−
+
−
=
+′=+′+=
′+=′=
2 2
22
1 1
11
2 21 1
2
1
ω
])(ˆ[e])(ˆ[e
ωω
]1)(][1)([π2
ω
])(ˆ[e])(ˆ[e
ωω
]1)(][1)([π2
IFIF
1)(),(;1)(,1)(;F
)(,1)(;)(),(;I
2
2
6
2
2
5
65
I
)(
122
)(
1
I
)(
111
)(
1)2(
2
1
l li
is
i
llr
i
fsr
l li
ir
i
lls
i
fsr
L LL L
if
srlsrf
srlsri
EE
nn
Vm
e
M
EE
nn
Vm
e
M
MM
EE
HLLH
EE
HLLH
R
nnLnn
nnLnn
k
rkrk
kk
k
rkrk
kk
pkepkekk
pkepkekk
kkkk
kkkk
h
h
h
h
ψψψψ
ψψψψ
ψψ
ψψ
| I 〉 | F 〉| L2 〉
k′ k
where
| I 〉 | F 〉| L1 〉
k k′
217](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-217-320.jpg)
(ˆ[
ωω
]1)()[(π2
I)()(F2I)()(F2IF
1)(,1)(;F
)(),(;I
h
k′k
| I 〉 | F 〉
218](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-218-320.jpg)
(ˆ[
ωω
)()(π2
I)()(FIF
1)(,1)(;F
)(),(;I
h
Two-photon emission:
i
i
fsr
sr
srif
srf
sri
nn
Vm
e
HHHR
nn
nn
ψψ
ψ
ψ
rkk
kk
keke
kk
kk
kk
kk
•′+−
′
++
′•
+′+
=
′==
+′+=
′=
)(
2
)()(
2
)1(
e)](ˆ)(ˆ[
ωω
]1)(][1)([π2
I)()(FIF
1)(,1)(;F
)(),(;I
h
k′k
| I 〉 | F 〉
k′
k
| I 〉 | F 〉
219](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-219-320.jpg)
![Epilogue
“Where did we get that [equation] from? Nowhere. It is not possible to derive
it from anything you know. It came out of the mind of Schrödinger!”
Richard Feynman
),(),(ˆ t
t
itH rr ΨΨΨΨΨΨΨΨ
∂
∂
= h
2017
MRT
221](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-221-320.jpg)
![Ice forms as a Hydrogen bond between water molecules by a polar H bond: δ+-δ−.
H bond
H2O (1s[1 -1s22s2p[2] -1s1])
2017
MRT
223](https://image.slidesharecdn.com/linked-inslides-hydrogen-130507131157-phpapp01/85/Part-V-The-Hydrogen-Atom-223-320.jpg)
Uploaded byMaurice R. TREMBLAY
Part V - The Hydrogen Atom
The document summarizes key details about the hydrogen atom and its electron orbital structure based on quantum mechanics. It provides: 1) A direct observation of the electron orbital of a hydrogen atom placed in an electric field, obtained through photoionization microscopy. Interference patterns observed directly reflect the nodal structure of the wavefunction. 2) Calculated and measured probability patterns for the electron in different energy levels of the hydrogen atom are shown. Bright regions correspond to high probability of finding the electron. 3) An overview of solving the radial, angular and azimuthal coordinate functions of the hydrogen atom through series expansion, as exact solutions have not been found. Approximate solutions can be obtained for more complex atoms like he
More Related Content
Similar to Part V - The Hydrogen Atom
Part V - The Hydrogen Atom
- 1. From First Principles PARTV – THE HYDROGEN ATOM June 2017 – R3.4 Maurice R. TREMBLAY
- 2. Prolog What you’re lookingat is the first direct observation of an atom’s electron orbital — an atom’s actual wave function! Up until this point, scientists have never been able to actually observe the wave function. Trying to catch a glimpse of an atom’s exact position or the momentum of its lone electron has been like trying to catch a swarm of flies with one hand. [c.f. Phys. Rev. Lett. 110, 213001 (2017)] 2
- 3. Photoionization microscopy candirectly obtain the nodal structure of the electronic orbital of a Hydrogen atom placed in a static electric field. In the experiment, the Hydrogen atom is placed in the electric field E and is excited by laser pulses. The ionized electron escapes from the atom and follows a particular tra- jectory to the detector that is perpendicular to the field itself. Given that there are many such trajectories that reach the same point on the detector, interference patterns can be observed […]. The interference pattern directly reflects the nodal structure of the wavefunction. [c.f. Phys. Rev. Lett. 110, 213001 (2017)] Calculated Measured 3
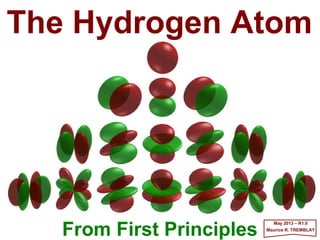
- 4. n = 1 llll= 0 mllll = 0 n = 2 llll = 0 mllll = 0 ↑↑↑↑ z n = 2 llll = 1 mllll = ±±±±1 n = 2 llll = 1 mllll = 0 n = 3 llll = 1 mllll = ±±±±1 n = 3 llll = 1 mllll = 0 n = 3 llll = 0 mllll = 0 n = 3 llll = 2 mllll = ±±±±1 n = 3 llll = 2 mllll = 0 n = 3 llll = 2 mllll = ±±±±2 Contents PART V – THE HYDROGEN ATOM What happens at 10−−−−10 m? The Hydrogen Atom Spin-Orbit Coupling Other Interactions Magnetic & Electric Fields Hyperfine Interactions Multi-Electron Atoms and Molecules Appendix – Interactions The Harmonic Oscillator Electromagnetic Interactions Quantization of the Radiation Field Transition Probabilities Einstein’s Coefficients Planck’s Law A Note on Line Broadening The Photoelectric Effect Higher Order Electromagnetic Interactions References Probability patterns for the electron in the Hydrogen atom. The computer generated pictures show slices through the atom for the lowest energy levels. The pictures are coded so that the bright regions correspond to high probability of finding the electron. 2017 MRT “The underlying physical laws necessary for the mathematical theory of a large part of physics and the whole of chemistry are thus completely known, and the difficulty lies only in the fact that the exact application of these laws leads to equations much too complicated to be soluble.” Paul Dirac, ‘Quantum Mechanics of Many-Electron Systems’ Proceedings of the Royal Society (London),123 (1929) pp. 714-733. 4
- 5. 2017 MRT “In order toexplain the results of experiments on scattering of αααα rays by matter Prof. Rutherford has given a theory of the structure of atoms. According to this theory, the atom consist of a positively charged nucleus surrounded by a system of electrons kept together by attractive forces from the nucleus; the total negative charge of the electrons is equal to the positive charge of the nucleus.” Niels Bohr, Philos. Mag. 26, 1 (1913). PART IV – QUANTUM FIELDS Review of Quantum Mechanics Galilean Invariance Lorentz Invariance The Relativity Principle Poincaré Transformations The Poincaré Algebra Lorentz Transformations Lorentz Invariant Scalar Klein-Gordon & Dirac One-Particle States Wigner’s Little Group Normalization Factor Mass Positive-Definite Boosts & Rotations Mass Zero The Klein-Gordon Equation The Dirac Equation References PART III – QUANTUM MECHANICS Introduction Symmetries and Probabilities Angular Momentum Quantum Behavior Postulates Quantum Angular Momentum Spherical Harmonics Spin Angular Momentum Total Angular Momentum Momentum Coupling General Propagator Free Particle Propagator Wave Packets Non-Relativistic Particle Appendix: Why Quantum? References 5
- 6. What happens at10−−−−10 m? 2017 MRT This is the last of a three-part series on quantum mechanics. I initially intended to finish this work with a good description of what we think an atom such as Hydrogen looks like and show you in detail its atomic orbital framework and then end there but I deemed the content void of a few important features: the Harmonic Oscillator and an introduction to Electromagnetic Interactions which leads directly to a formulation of the Quantization of the Radiation Field! Then I could not finish without wrapping it up with a development of Transition Probabilities and Einstein Coefficients which opens up the proof of the Planck distribution law, the photoelectric effect and higher order electromagnetic interactions – in essence justifying how things started in PART III. I believe this is the key contribution: making it more understandable up to, but not including, Quantum Electrodynamics (QED)! For that QED part, you can refer to PART VII which is entirely dedicated to its formulation. On the other hand, I then felt compelled to add a whole new chapter on Multi-Electron Atoms and Molecules which I have taken almost piecemeal from the book from David B. Beard and George B. Beard, Quantum Mechanics with Applications. 6 Now, its funny but all of this rough work of presenting all this machinery of quantum mechanics, angular momentum and Dirac’s equation leads us now to the exercise of formulating the Schrödinger equation with a non-vanishing radial potential V(r) which is the Coulomb potential between the Hydrogen atom’s nucleus (i.e., the proton) and an electron in ‘orbit’, &c. But we will do better by taking the v2/c2 approximation to Dirac’s equation developed earlier in PART IV and just limit ourselves here to only a few cases of academic and theoretical interest notably Spin-Orbit Coupling and Other Interactions such as Magnetic & Electric Fields and Hyperfine Interactions.
- 7. 2017 MRT As I willmention in the Multi-Electron Atoms and Molecules chapter, only reasonably accurate solutions of the Hydrogen atom are possible because it is comprised of only one electron surrounding a single proton and Bohr radius of the electron is ~0.5×10−10 m. I say ‘reasonably accurate solutions’ because the differential equations that indepen- dently represent the radial, angular and azimuthal coordinate functions are solved pretty much only by assuming a series expansion. So, in effect, for the simplest kind of atom in the universe, mankind still hasn’t figured out an exact solution to the differential equa- tions that represent its “orbitals”… Now, Helium atoms are composed of a nucleus com- posed on two protons and two neutrons (i.e., an alpha particle) that is surrounded by two electrons. In theory, this is a three-body problem; it cannot be solved explicitly in closed form as unfortunately no one has ever been able to solve in closed form any three-dimen- sional case involving more than two interacting particles. However, as with the Hydrogen atom, approximate solutions can be obtained for the energy of the ground state using perturbation theory and I will show you how to do it but only up to first-order accuracy. 7 In essence, it is so important to understand one thing: the quantum ‘mechanics’ used in this theory of the representation of the Hydrogen atom has generated vast amounts of quantitative verifications – even relativistically! So, this is the way nature is like to a certain degree since I did not include interactions with other particles or photons. Nature does act weird at small scales, i.e., those of the atom or about 10−10 m! An electron does have spin and it does fill defined orbitals which can be represented quite faithfully for a Hydrogen atom – one of the simplest, yet quite intricate solution. This is the outcome of quantum mechanics – allowing us to finally ‘see’ an atom! Well, a kinda blury one!
- 8. 2017 MRT By weight, 75%of the visible* universe is Hydrogen, a colorless gas. In space, vast quantities interact with starlight to create spectacular sights such as the Eagle Nebula (seen by the Hubble Space Telescope). The Hydrogen atom is the simplest atom… yet: Symbol: H – element number 1. Atomic Weight: 1.008. Color: Colorless. Discovery: 1766 in the United Kingdom. Electron Configuration: 1 s 1 ; Valence: 1. Electronegativity: 2.2. Electron Affinity: 72.8 kJ/mol. Ionization Energies: 1312 kJ/mol. Atomic Radius: 53 pm; Covalent Radius: 37 pm; Van der Waals Radius: 120 pm. Crystal Structure: Simple Hexagonal. Density: 0.0899 g/l. Melting Point: −259.14 °C . Boiling Point: −252.87 °C . Thermal Conductivity: 0.1805 W/(m K). Gas phase: Diatomic. Magnetic Type: Diamagnetic. Mass Magnetic Susceptibility: −2.48×10 −8 . % in Universe: 75%; % in Sun: 75%; % in Meteorites: 2.4%; % in Earth's Crust: 0.15%; % in Oceans: 11%; % in Humans: 10%. Half-Life: Stable; Quantum Numbers: 2 S1/2. Stable Isotopes: 1 H, 2 H. Isotopic Abundances: 1 H - 99.9885% 2 H - 0.0115% The Hydrogen Atom 8 * In the form of baryonic mass and note that most of the universe’s mass is not in the form of baryons or chemical elements (e.g., dark matter and dark energy.)
- 9. with mo≡me therest mass of the electron. By replacing E′ by E (non-relativistic energy); eφ by −V,and p by −ih∇∇∇∇, the above equation becomes the static Schrödinger equation: For an electron in a static field whose potential is ϕ, the Dirac equation (i.e., (E′+eϕ)ψ ={(1/2mo)[p+(e/c)A]2 +SMM[µS] Interaction−RE[p]−DT[p2]−SO[L••••S] Interaction}ψ – c.f., PART IV) without the higher-order relativistic corrections, simplifies and reduces to: 2017 MRT ψψφ o 2 2 )( m eE p =+′ in which the square of the angular momentum vector L is: or, in spherical coordinates: += +∇−= 22 e 22 e 2 )()()( 2 )( cmcEV m E prr ψψ h ),,(),,()( 2 ),,( 2 2 2 2 e 2 2 2 ϕθψϕθψϕθψ rrVE rm r rrr r L=−+ ∂ ∂ + ∂ ∂ h 2 2 2 2 sin 1 sin sin 1 ϕθθ θ θθ ∂ ∂ + ∂ ∂ ∂ ∂ =L We recognize here the L2 operator from our discussion on spherical harmonics. We will now consider a Hydrogen atom consisting of a single proton (Z=1) at its center and an electron surrounding it. For hydrogen-like atoms (with Z>1), the reduced mass µ =memp/(me +mp) should be used but can be neglected for this purpose of trivial illustration. 9
- 10. Assuming V=V(r), thegeneralized wave function for the Hydrogen Atom is: with λ as a separation constant. It is assumed that ψ =ψ (r,θ,ϕ) and its first derivatives are everywhere continuous, single-valued, and finite. The consequence of imposing these conditions are that: 2017 MRT ( )...,2,1,0)1( =+≡ lllλ The functions Y(θ,ϕ) are the spherical harmonics Yl ml(θ,ϕ) with: on which we impose the requirement (a boundary condition) that V(r)→0as r →∞. ),(),( )()()]([ 2)( 2 22 e 2 2 ϕθλϕθ λ YY rP r rPrVE m rd rPd = =−+ L h lllll ,1,...,1, ++−−≡m Substituting these into the equation above for P(r), we obtain the Radial Equation: 0)( )1( )]([ 2 22 e 2 2 = + +−+ rP r rVE m rd d ll h The equation r2[∂2/∂r2 +(2/r)∂/∂r]ψ +(2me r2/h2)(E−V )ψ =L2ψ above separates into: ),()( 1 )()()(),()(),,( ϕθϕθϕθϕθψ l ll l l lllllll m nmmn m nmn YrP r rRYrRr =ΦΘ== 10
- 11. has solutions P(r)=exp(±ar)where a=√[−(2me/h2)E]. Solutions to this equation may be obtained by first considering the behavior at large r. In the asymptotic region r →∞: 2017 MRT )(e)(e)( o2 rfrfrP arra −− == where f (r) is a function to be determined by the Radial Equation and the boundary conditions. 0)( 2)( 2 e 2 2 =+ rPE m rd rPd h If E<0, exp(+ar)→∞ as r →∞. Since this violates (i.e., the ‘mathematical’ fact that the exponential blows up at infinity!) the conditions (i.e., that it does not!) that the wave function must be finite everywhere, it is not an acceptable solution (i.e.,itis not physically possible!) On the other hand, exp(−ar)→0 as r →∞; it is therefore a possible solution. If E >0, either sign in the exponent will satisfy the boundary conditions. We concentrate on the case E<0, that is, the bound states of the atom. The asymptotic behavior suggests that solutions to the Radial Equation be sought in the form: In the following slides, we will make use of the Bohr Radius (N.B., α =e2/hc=1/137): 11 ( ) ( )MKSmorCGScm 11 2 e 2 o o 8 e 2 e 2 o 1029.5 επ4 1052.0 −− ×==×=== em a cmem a hhh α
- 12. First few radialfunctions Rnl(r) for R10, R20 and R21 as a function of the Bohr radius, ao = 4πεoh2/mee2 and has a value for Hydrogen of 5.29×10−11 m. 0 )1(2 επ4 1 2 2 o 2 2 = + −+− f rr e rd fd a rd fd ll The substitution of P(r) = f (r)exp(−a r) into this equation yields: To ensure that f remains finite as r→ 0, it is necessary for s to be positive. When this series is substituted into the differential equation for f , it is found that s=l +1 >0. Without immersing ourselves into the fairly intricate details, the final solution for Rnl is given by (if nuclear movement is not negligible: ao'=ao(1+ me /mN)): The Radial Equation now becomes: 0)( )1( επ4 12)( 2 2 o 2 e 2 2 = + −++ rP rr e E m rd rPd ll h To proceed further, it is necessary to specify the form of the potential V(r). Let us now assume that the physical system consists of an electronof rest mass me interactingwith a nucleus of charge e, viatheCoulomb interaction (notice how this is a function of r only): r e rV 2 oεπ4 1 )( = whose solutions may be expressed as a power series (i.e., we can solve it only approximately!): )( 2 210 L+++= rArAArf s 12 / o /2 1 1 2/3 o 2 1 o o e )e( 1 )!1()!( 2 )( 1 )( + +− −− −− + + −−+ == l l l l l l l ll ll ra r r rd d annn rP r rR anr nanr n n nn n = 1, l = 0 n = 2, l = 0 n = 2, l = 1 Rnl(r) r /ao 2017 MRT o/ 2/3 o 10 e2 1 )( ar a rR − = o2/ o 2/3 o 20 e2 2 1 )( ar a r a rR − − = o2/ o 2/3 o 21 e 32 1 )( ar a r a rR − = 12
- 13. Pnl(r) 1 0 2 0 21 3 0 3 1 3 2 4 0 4 1 4 2 4 3 o2 o 23 o e 2 1 2 1 arZ a rZ r a Z − − Explicit forms of Pnl(r) for several values of n and l are given in the Table below. n l o e2 23 o arZ r a Z − o2 o 223 o e 62 1 arZ a rZ a Z − o3 2 oo 23 o e 27 2 3 2 1 33 2 arZ a rZ a rZ r a Z − +− 2017 MRT o3 oo 223 o e 6 1 627 8 arZ a rZ a rZ a Z − − o3 2 o 3223 o e 3081 4 arZ a rZ a Z − o4 3 o 2 oo 23 o e 192 1 8 1 4 3 1 4 arZ a rZ a rZ a rZr a Z − − +− o4 2 ooo 223 o e 80 1 4 1 3 5 16 1 arZ a rZ a rZ a rZ a Z − +− o4 o 2 o 3223 o e 12 1 564 1 arZ a rZ a rZ a Z − − o4 3 o 4323 o e 35768 1 arZ a rZ a Z − 13
- 14. With V(r)=(1/4πεo)e2r−1 andin spherical coordinates, the Schrödinger equation reads: ϕρ ρ θ θ θ ϕθρρϕθψ l l l l l ll l ll l l l l l l l l l l l l l ll l l ll l l mi m m m nar nnar n n ar m nmnmn d d ra r r dr d am m nn YLNr e )cos( sin sin e )e( 1 )!( )!( π)!1()!( 12 !2 )1( ),()(e),,( 2 12 o 2 1 1 23 o 2 2 12 1 2 o oo + + + +− −− −− + = + −− − + − −−+ +− → ⋅= 0),,( πε4 sinπ8),,(),,( sinsin ),,( sin o 2 2 22 e 2 2 2 22 = ++ ∂ ∂ + ∂ ∂ ∂ ∂ + ∂ ∂ ∂ ∂ ϕθψ θ ϕ ϕθψ θ ϕθψ θ θ θ ϕθψ θ rE r e h rmrr r r r r )()()(),,( ϕθϕθψ ΦΘ= rRr 02 2 2 =Φ+ Φ lm d d ϕ ↓ ↓ ↓ ϕϕ ϕ ll l mimi m BA − +=Φ ee)( 0 sin )1(sin sin 1 2 2 =Θ −++ ∂ Θ∂ θθ θ θθ l ll m d d ↓ θcos= ↓ w 0 1 )1(2)1( 2 2 2 2 2 =Θ − −++ Θ − Θ − w m wd d w wd d w l ll )()( wPAw m mm l ll lll =Θ ↓ ↓ ↓ ↓ l l l l l ll l l l l l l ll l m m m m d d m m + + + + −+− =Θ )cos( sin sin )!( )!( 2 12 ! )( )( 2 12 θ θ θθ ↓ ϕ ϕ l l mi m e π2 1 )( =Φ ↓ 12 / o /2 1 1 2/3 o 2 1 o o e )e( 1 )!1()!( 2 )( + +− −− −− + + −−+ = l l l l l l l l ll ra r r rd d annn rR anr nanr n n n ↓ ↓ ↓ )(e)( 122/ ρρρ ρ + + − = l l l ll nnn LAR 0 )1( 4 22 2 = + −+++ Rn rd dR rd Rd ρ ρ ρ ll 0 )1( πε4 π81 2 o 2 2 e 2 2 2 = + + +++ R r E r e h m n rd dR r rd d r ll ↓ and the solution for the wave function becomes (which is a functionof r,θ andϕ only): 2017 MRT Time Independent Schrödinger Differential Equation Legendre Associated Differential Equation Laguerre Differential Equation Second-Order Homogeneous Differential Equation with Constant Coefficients 14 o/2 ar=↓ ρ
- 15. Notation Wave functionEnergy (×R) Degeneracy (n2) 1 0 0 1s −Z2 1 2 0 0 2s −(Z/4)2 4 1 0 2p0 1 ±1 2p±1 o2 o 23 o 200 e2 32π 1 arZ a rZ a Z − − =ψ To summarize, we have three quantum numbers n, l, and ml, where n is the principle quantum number with possible value 1,2,3,…; l is the orbital angular momentum quantum number (orbital quantum number, for short) with possible values 0,1,2,…, n−1; and, ml the magnetic (or projection) quantum number whose values are restricted to l, l–1,…,−l. In spectroscopic notation, s, p, d, f, g,… correspond to l=0,1,2,3,4,…, respectively. n l ml o e π 1 23 o 100 arZ a Z − =ψ θψ cose 32π 1 o2 o 23 o 210 arZ a rZ a Z − = φ θψ iarZ a rZ a Z ±− ± = esine 64π 1 o2 o 23 o 121 2017 MRT It is convenient to employ a natural unit of energy,calledaRydberg, R, for measuring the energy levels of hydrogen: 2 4 e 2h em R = 15
- 16. 2017 MRT Notation Wave functionEnergy (×R) Degeneracy (n2) 3 0 0 3s −(Z/9)2 9 1 0 3p0 1 ±1 3p±2 2 0 3d0 2 ±1 3d±1 2 ±2 3d±2 4 0 0 4s −(Z/16)2 16 1 0 4p0 1 ±1 3p±2 n l ml o3 2 oo 23 o 300 e21827 3π81 1 arZ a rZ a rZ a Z − +− =ψ θψ cose6 π 2 81 1 o3 2 oo 23 o 310 arZ a rZ a rZ a Z − − = ϕ θψ iarZ a rZ a rZ a Z ±− ± − = esine6 π81 1 o3 2 oo 23 o 131 )1cos3(e 6π81 1 23 2 o 23 o 320 o − = − θψ arZ a rZ a Z ϕ θθψ iarZ a rZ a Z ±− ± = ecossine π81 1 o3 2 o 23 o 132 ϕ θψ iarZ a rZ a Z 223 2 o 2/3 o 232 esine π162 1 o ±− ± = o4 3 o 2 oo 23 o 400 e24144921 π1536 1 arZ a rZ a rZ a rZ a Z − − +− =ψ θψ cose2080 π 5 2560 1 o4 3 o 2 oo 23 o 410 arZ a rZ a rZ a rZ a Z − + − = ϕ θψ iarZ a rZ a rZ a rZ a Z ±− ± + − = esine2080 2π 5 2560 1 o4 3 o 2 oo 23 o 141 16
- 17. Notation Wave function 20 4d0 2 ±1 4d±1 2 ±2 4d±2 3 0 4f0 3 ±1 4f±1 3 ±2 4f±2 3 ±3 4f±3 n l ml )1cos3(e20 π3062 1 24 3 o 2 o 23 o 420 o − − = − θψ arZ a rZ a rZ a Z ϕ θθψ iarZ a rZ a rZ a Z ±− ± − = ecossine12 2π 3 1536 1 o4 3 o 2 o 23 o 142 ϕ θψ iarZ a rZ a rZ a Z 224 3 o 2 o 2/3 o 242 esine12 2π 3 3072 1 o ±− ± − = ϕ θψ iarZ a rZ a Z ±− ± − = e)1cos5(e 5π 3 6144 1 24 3 o 23 o 143 o ϕ θθψ iarZ a rZ a Z 224 3 o 23 o 243 ecossine 2π 3 3072 1 o ±− ± = ϕ θψ iarZ a rZ a Z 334 3 o 2/3 o 343 esine π6144 1 o ±− ± = )cos3cos5(e 5π3072 1 34 3 o 23 o 430 o θθψ − = − arZ a rZ a Z 2017 MRT The fact that in a Hydrogen atom the energy level degeneracy (i.e., two or more different states give the same value of energy) is actually n2 is due to the special property of the Coulomb field. In fact, it is the classification of states with respect to the irreducible representations of the four-dimensional group – of which O+(3) is a subgroup – that leads to the n2 degeneracy. 17
- 18. A Hydrogen atomhas an electron which is distributed around a normalized radius ao. is a complex probability density of the electron in a Hydrogen atom. ϕ θ θ θϕθψ l l l l l l ll l l l l l l l l l l l l ll l l mi m m m anr nanr n n mn d d ra r r dr d am m nn r e )cos( sin sin e )e( 1 )!( )!( π)!1()!( 12 !2 )1( ),,( 2 12 o 2 1 1 23 o 2 o o + + + +− −− −− + + − −−+ +− = Here is the generalized wavefunction of a Hydrogen atom (forjustasingleelectron): where n, ml and l are quantum numbers indicating the state ψnlml (r,θ,ϕ) of the electron’s position r given by its orbital level, magnetic moment, and angular momentum, respectively. 1 nm = 10−9 m n = 2,llll = 1 n = 2,llll = 0 n = 1,llll = 0 ψ100 ψ200 ψ211 ml=0 ml = 0 mllll = 1 mllll = 0 |ψ 84±1(r,θ,ϕ)|2 2017 MRT 18
- 19. 0 5 10 ElectronVolt(eV) 13.60 n 1 2 3 4 5 ∞ s pd f LymanSeries 0 −5 −10 −13.60 The first few energy levels of the Hydrogen atom – without fine structure (i.e. corrections due to nuclear spin angular momentum I ) or using the reduced mass µ instead of me. The energy levels of the Hydrogen atom are given by the Balmer formula: The Rydberg constant is given by: and it is related to the ‘Ry’ energy unit by: eV605.137.3169,7310 2 2 2 e2 1 == = − ∞ 1 cm c e cmR h Ry1eV(12)13.6056923 ≡ Ry −−= ∞ 2 1 2 2 2 11 nn ZREn ( )...,4,3,2,1 1 2 )( 2 2 22 22 e =−=−= n n Z n Zem En Ry h If an electron changes from one state to another, there will be a corresponding change in energy of the system: These transitions will in general be accompanied by an emission or absorption of electromagnetic radiation (c.f., Appendix: Higher Order Electromagnetic Interactions). For instance, if n1 =1, then one gets the Lyman series, in which n2 can take on values of 2, 3, 4, …. Recall also that the electron volt [eV] is a unit of energy equal to approximately 1.602×10−19 J [Joule] and by definition it is equal to the amount of kinetic energy gained by a single unbound electron when it accelerates through an electric potential difference of one volt. Thus it is 1 Volt (1 Joule per Coulomb) multiplied by the electron charge (1e, or 1.602×10−19 C [Coulomb]).Therefore,one electron volt is equal to: 1 eV =1.602×10−19 J. 2017 MRT 19
- 20. The probability offinding an electron in an element of volume dτ is: 2017 MRT If this equation is integrated over the surface of a sphere, we obtain the probability of finding an electron in a shell between two spheres of radii r and r +dr. Since the spherical harmonics are normalized to unity, the result is simply Pnl 2(r)dr. In the sense that ψ ∗ψ is a charge density, Pnl 2(r) is a radial charge density. τdYYrP r τd mm n ),(),()( 1 * *2 2 ϕθϕθψψ ll lll= The probability of finding an electron between θ and θ +dθ is proportional to: θθθθθϕθϕθ dPdYY mmm sin)](cos[sin),(),( 2* lll lll = Finally, the probability of finding an electron between ϕ and ϕ +dϕ is simply proportional to dϕ. 20
- 21. Plot forY1 0,R10,and theprobability densityψ100 ∗ψ100=|ψ100|2 of the orbital [ yellow(+)/ blue (−) on black] which is the probability of finding an electron around the atom’s nucleus. 2017 MRT Plots for Y1 0 , R20, & |ψ200|2, Y1 0 , R21, & |ψ210|2, and for Y1 1 , R21, & |ψ211|2. +½ −½ +½ −½ The maximum number Nn of electrons in a shell characterized by principle number n is given by twice (i.e. due to spin) the number of orbital stateswiththatn: ...,18,8,22 )12(2 2 1 0 == += ∑ − = n N n n l l 21 + −
- 22. Plots for Y3 0, R30, & |ψ300|2, Y0 0 , R31, & |ψ310|2, Y1 1 , R31, & |ψ311|2, Y2 0 , R32, & |ψ320|2, Y2 1 , R32, & |ψ321|2, and for Y2 2 , R32, & |ψ322|2. And finally, a few more plots for Y3 ...≤3 , R4 …≤3, & |ψ4 …≤3 …≤3|2 … 2017 MRT 22
- 23. One intricate configurationfor a Hydrogen atom is for a single electron (reduced mass µ, charge e) surrounding a single proton (with proton-to-electronmass ratio mp/me ≅1836). Joule or 19- 2 32 2 2 0,2,4d4 2 22 e 2 2 2 eo 2 101.36eV85.0 4 34 4 1 4 eV6.13 24 3 ½ 1 1 2 1 2 ×=−= − +− →−≅ − + + −= ααα z n n cmn nnma E l h l 0)( επ42 420 o 2 4 2 e 2 = ++∇ r r ψ e E m h 4 222 0,2,4d42 )3( π16 52 r rzz − →ΘΦ or Schrödinger’s time-independent wave equation (i.e.,the steady state in quantum theory) in r position-vector space:* This can also be represented differently by expanding time t and the Laplacian operator ∇2 into spherical coordinates r, θ, ϕ and keeping the potential −e2/r generalized as V: ),,(),,(),,( 2 ),,( 420420 2 e 2 420 ϕθψϕθϕθψϕθψ rrVr m r t i +∇ −= ∂ ∂ h h This 4dz2 wavefunction is then for n=4, l=2 and ml=0:† † The probability distribution of the electron surrounding the protonic nucleus is – or this 4dz2 state: * The total energy required to provide the electron surrounding the protonic nucleus to reach this state is calculated as always being: )1cos3(e 1 π1536 5 )cos( sine)e(1 π04 5 32 1 24 2 o 23 o 2 42 5 42 o 62 23 o o oo − = = − θ θ θ ar arar a r ad d ra r dr rd a 4dz2 One of the many states possible for an electron to occupy in a Hydrogen atom. ||||ψ420 (r,θ,ϕ =0)||||2 2017 MRT 23
- 24. Average values ofvarious powers of r are often needed in computation; these are defined by the expectation value: 2017 MRT Expectation values 〈rk 〉 of rk for several values of k are given in the Table below. ∫∫ ∞ + ∞ == 0 22 0 2 )()( drrRrdrrPrr n k n kk ll 〈rk〉 1 2 3 4 −1 −2 −3 −4 )]1(315[ 2 2 2 2 2 o +−+ lln n Z a k )]1(3[ 2 2o +− lln Z a )]1()1)(2(3)1)(2(30)1(35[ 8 222 2 3 2 o −+++−+−− llllllnnn n Z a ]12)1033)(1(5)322(3563[ 8 2224 4 4 4 o +−+++−+− llllllnn n Z a 2 o 1 na Z )½( 1 32 o 2 +lna Z lll )½)(1( 1 33 o 3 ++na Z )½)(½)(1)((2 )1(3 2 35 2 4 o 4 −+++ +− llll ll n n a Z 24
- 25. For the HamiltonianH=p2/2me −(1/4πεo)Ze2/r the complete solution to the Schrödinger equation Hψ =Eψ for the bound states consists of the orbitals ψnlml (r,θ,ϕ) together with the energy eigenvalues En =−Z2/n2. However, the Schrödinger equation: 2017 MRT with the Hamiltonian (see the Appendix – Higher Order Electromagnetic Interactions): pAAp ××××∇∇∇∇ΣΣΣΣ∇∇∇∇∇∇∇∇××××∇∇∇∇ΣΣΣΣ ϕϕ •−•−−•+ += 22 e 22 e 2 23 e 4 e 2 e 48822 1 cm e cm e cm p cm e c e m H hhh ψψψϕ )()( Orbit-SpinDarwinicRelativistnInteractioo HHHHHHeE ++++==+′ contains additional terms (e.g., Unperturbed, Interaction, Relativistic, Darwin and Spin- Orbit) which all have an effect on the eigenfunctions and eigenvalues of the system. which identify the system as a particle with spin s=½. ±=±+=± ±±=± 222 ¾)1½(½ ½ hh h S zS Examination of the Hamiltonian above reveals the presence of terms containing the operator ΣΣΣΣ whose components are the Pauli matrices, σσσσ =[σ1,σ2,σ3] (where σ1 REAL/SYM = +1, σ2 COMPLEX/ANTI = mi, and σ3 REAL/DIAG PARITY =±1). According to our previous discussion, S =½hΣΣΣΣ where the rectangular components of S are angular momentum operators and: Thus, the appearance of ΣΣΣΣ in the Hamiltonian indicates that the wave function of the system must include a spin eigenfunction. 25
- 26. In the spin-½[in units of h] case, we saw earlier that: 2017 MRT = =−=− = =+=+ = − + χ χ 1 0 ½,½ 0 1 ½,½ , sms The one-electron spin function is: −= += == − + ½ ½ )( s s sm m m ms for for χ χ ξξ whereas the (one-electron) spin orbital is: )()(),,,(),,( ssmmn mmmnr s ξrξ ψψϕθψ == ll ll It shall be understood that an integral involving spin orbitals implies a spatial integration as well as a summation over spin coordinates. Also, we now have a degeneracy of 2n2 associated with the addition of spin eigenfunctionψnlmlms (r,θ,ϕ,±½) andweuse |l,s;ml ,ms 〉 to identify the angular and spin parts of the wave function. Degenerate eigenfunctions may be combined linearly to form other sets in a coupled representation of degenerate eigenfunctions using the Clebsch-Gordan coefficients Cj mlms =〈l,s;ml,ms |l,s; j,mj 〉: ∑∑ == s s s mm s j mm mm jssj mmsmjsmmsmmsmjs l l l lll lllll ,;,,;,,;,,;,,;, C 26
- 27. n = 1=1= 1= 1 mllll = 0= 0= 0= 0 llll = 0= 0= 0= 0 llll = 1= 1= 1= 1 llll = 2= 2= 2= 2 llll = 3= 3= 3= 3 n = 2= 2= 2= 2 mllll = 0= 0= 0= 0 n = 2= 2= 2= 2 mllll = 1= 1= 1= 1 n = 3= 3= 3= 3 mllll = 0= 0= 0= 0 n = 3= 3= 3= 3 mllll = 1= 1= 1= 1 n = 3= 3= 3= 3 mllll = 2= 2= 2= 2 n = 4= 4= 4= 4 mllll = 0= 0= 0= 0 n = 4= 4= 4= 4 mllll = 1= 1= 1= 1 ψ100 ψ200 & ψ210 ψ211 ψ300, ψ310 & ψ320 ψ311 & ψ321 ψ322 ψ400, ψ410, ψ420 & ψ430 ψ411, ψ421 & ψ431 Atomic orbitals are best represented by various probability distributions where the electron will most probably be present given the quantum numbers n and ml versus llll. LEVEL 1 – FUNDAMENTAL 1H ENERGY LEVEL LEVEL 2 – FIRST EXCITATION AVAILABLE LEVEL 2 – ADDED SECTORIAL HARMONICS LEVEL 3 – SECOND EXCITATION AVAILABLE LEVEL 3 – MORE SECTORIAL HARMONICS LEVEL 3 – ADDED TESSERAL HARMONICS 2017 MRT 27
- 28. No more than2 electrons in this single 1s orbital. No more than 6 electrons in these 2p (px, py, & pz ) orbitals. No more than 2 electrons in this 2s orbital. No more than 6 electrons in these 3p (px, py, & pz ) orbitals. No more than 2 electrons in this 3s orbital. No more than 10 electrons in these 3d (dxz, dyz ,dxy, d x2 −−−−y2 & dz2 ) orbitals. No more than 18 electrons in these 4 f (fz3 −−−−(3/5)zr2 , fy3 −−−− (3/5)yr2 , fx3 −−−−(3/ 5)xr2 , fx(z2 −−−−y2) , fy(x2 −−−−z2) , fz(x2 −−−−y2) & fxyz) orbitals. ψ1s ψ2s & ψ2pz ψ2px ψ3s, ψ3pz & ψ3dx2 ψ3px & ψ3dxz ψ3dxy ψ4s , ψ4pz , ψ4dz2 & ψ4f z3 −−−−(3/5)zr2 ψ4px , ψ4dxz & ψ4f y(x2−−−− z2) 1s 2s 2pz 2px 3dz2 3pz 3px 3dxz 3dxy 3s 4fz3−−−−(3/5)zr2 4fy(x2−−−−z2) Each electron can have a Spin Up component(Sz =+½h) and a Spin Down (Sz =−−−−½h) along the z-axis within each orbital – both not both.This is Pauli’s Exclusion Principle. llll = 0= 0= 0= 0 llll = 1= 1= 1= 1 llll = 2= 2= 2= 2 llll = 3= 3= 3= 3 2017 MRT 28
- 29. We turn nowto the spin-orbit coupling term in the Schrödinger equation. The Hamiltonian HSpin-Orbit is given by (the reduced mass µ =memp/(me +mp) is negligeable): Spin-Orbit Coupling 2017 MRT in which L (=r××××p) is the orbital angular momentum operator. When ∇∇∇∇φ ××××p=(dφ/dr)(1/r)L is substituted into HSO above and σσσσ is replaced by (2/h)S we obtain: 322 e 22 2 )( rcm eZ r h =ξ p××××∇∇∇∇ΣΣΣΣ φ•−=≡ 22 e SOOrbit-Spin 4 cm e HH h where e is the electronic charge (we also set henceforth e=e/4πεo ), me is the rest mass of the electron and φ is the electrostatic potential. If φ depends only on r, we have: r r ˆφ φ φ ′== rrd d ∇∇∇∇ and: Lprp rrrd d φφ φ ′ == h××××××××∇∇∇∇ 1 SLSL •=•−= )( 1 2 22 e 2 SO r rcm e H ξ h For Hydrogen, the potential is φ =Ze/r so that: which is the spin-orbit amplitude (or intensity). = oπε4 e e 29
- 30. The one-electron Hamiltonianwith spin-orbit coupling now becomes: 2017 MRT with: SO0 HHH += SL•=−∇−= )( 2 SO 2 2 e 2 0 rH r eZ m H ξand h 0)1(SO =− jinji EH δ )0( SO )0(SO jninji HH ψψ= We regard HSO as a perturbation (i.e., an approximation based on energy or potential series) although the justification for this may not be apparent until a calculation of the magnitude of the effect has been carried out. where: and ψ (0) ni, ψ (0) nj are degenerate eigenfunctions of Ho. Since H0 has degenerate eigenvalues, the first-order corrections to the energy with be obtained from the solution of the secular (or determinant) equation: Without proof, we state that |l,s;j,mj 〉 is a simultaneous eigenfunction of L2, S2, J2, Lz, and L•S. We therefore expect the coupled representation to be the most convenient since only diagonal matrix elements will appear in the secular determinant. 30
- 31. To see howthis works out, let: 2017 MRT or: )( 222 2 1 SLJ −−=•SL ∫∫ ∞∞ ==== 0 2 0 2 )()()()(,)(,)( rdrPrrdrrRrnrnr nnn lll ll ξξξξξ 3322 e 22 3322 e 22 1 2 , 1 , 2 )( rrcm eZ n r n rcm eZ r h ll h ==ξ Using the coupled representation: The Hamiltonian HSO =ξ(r)L•S also contain the radial function ξ(r). So, to obtain the energy corrections it is also necessary to evaluate the expectation value of ξ(r): From the Table for 〈rk 〉 (with k=−3): SLSLJSLJ •++== 2222 and++++ jjss jjjj ssjj mjsSLJmjsmjsmjs ′′′+−+−+= −−′′′′=•′′′′ δδδ llll llll )]1()1()1([ ,;,,;,,;,,;, 2 1 222 2 1SL In hydrogen, ξ(r) is given by ξ(r)=Ze2h2/2me 2c2r3 in which case: lll )½)(1( 11 23 o 3 3 ++ = na Z r 31
- 32. On combining theabove relations for 〈l′,s′; j′,m′j|L•S|l,s;j,mj 〉, ξnl, and 〈r−3 〉, the spin- orbit interaction energy is ( j=l+½): 2017 MRT or in Rydberg units: lll llh )½)(1( )1()1()1( 4 322 e 3 o 224 SO ++ +−+−+ = n ssjj cma eZ E with α =e2/hc and ao =h2/mec2. It is possible to rewrite the above equations in terms of l since j is confined to the values l±½ and s =½. We then have (for l≠0 only): For Z=1 and l=1, the splitting due to spin-orbit coupling is 0.36, 0.12, and 0.044 cm−1 for n=2, 3, and 4, respectively. Since these energies are much smaller than the energies which separates states with different values of the principle quantum number n – about 104 cm−1 – the use of perturbation theory to first order is certainly appropriate. Ry lll ll )½)(1( )1()1()1( 3 24 SO ++ +−+−+ = ssjj n Z E α +−++ == ± )1()½)(1( 1 2 2 1 2 1 33 o 22 e 224 ½SO llll h l nacm eZ EE 1 cm− + =∆ )1( 84.5 3 4 SO lln Z E 32
- 33. From the relation〈l′,s′; j′,m′j|L•S|l,s;j,mj 〉=½[ j( j+1)−l(l+1)−s(s+1)]δl′lδs′sδj′j it is seen that the matrix elements vanish unless: These are the selection rules for spin-orbit coupling. Also, these are also valid: 0)(0 =+∆=∆=∆=∆ sj mmmj ll and The Table below lists values of the 〈1,½; m′l,m′s|L•S|1,½;ml,ms 〉 matrix elements for p states of ξnl= 〈n,l|ξ(r)|n,l〉 (and shortened to |ml ,ms 〉 since l=1 and s =½ for all states). ½,1− 2017 MRT ½,1 ½,1 ½,0 ½,0 − ½,1− ½,1−− ½,1 − ½,0 ½,0 − ½,1− ½,1 −− 2 1 2 1 − 2 1 2 1 0 0 2 1 2 1 2 1 − 2 1 1,01,0 ±=∆±=∆ smm andl 33
- 34. The term inthe Schrödinger equation that corresponds to the relativistic correction to the kinetic energy is: 2017 MRT or, with H0 =p2/2me −Ze2/r: ′+ ′+′+ +′−=′ jj jjjj jjjj mjsn r mjsneZ mjsnH r mjsnmjsn r HmjsneZ mjsnHmjsn cm mjsnHmjsn ,;,, 1 ,;,,)( ,;,, 1 ,;,,,;,, 1 ,;,, ,;,,,;,, 2 1 ,;,,,;,, 2 22 00 2 2 02 e R ll llll llll Other Interactions We shall be interested in the effects produced by HR within a manifold of states specified byparticularvaluesof n, l, s, j as for example 2S1/2 or 2P3/2 (where 2s+1Xj with X=S,P,D,F,… stands for l=0,1,2,3,…) eigenstates of H0 belonging to a particular value of n. Therefore, treating HR as a perturbation, the basis set is |n,l,s; j,mj〉 and the matrix elements are: 2 e 2 2 e 23 e 4 RicRelativist 22 1 8 −=−=≡ m p cmcm p HH + ++−= +−= 2 22 00 22 02 e 2 2 02 e R 1 )( 11 2 1 2 1 r eZH rr HeZH cmr eZ H cm H 34
- 35. This last equationfor 〈n,l,s; j,m′j|HR |n,l,s; j,mj〉 can be simplified. For the first term on the right we have: 2017 MRT where En (0) is an eigenvalue of H0. In view of the non-commutativity of r and p, H0 and 1/r do not commute; nevertheless, the Hermitian property of H0 leads to the equality of the matrix elements: jj mmnjj EmjsnHmjsn ′=′ δ2)0(2 0 )(,;,,,;,, ll in which 〈r−1 〉 depends only on the radial part of the wave function and is independent of mj. Similarly: The net result is that HR has only diagonal matrix elements: 22 e 22 (0) 2 e 2 2 e 2(0) R 1 2 )(1 2 )( rcm eZ r E cm eZ cm E H n n −−−= jj mmnjjn jjjj r Emjsn r mjsnE mjsnH r mjsnmjsn r Hmjsn ′=′= ′=′ δ 1 ,;,, 1 ,;,, ,;,, 1 ,;,,,;,, 1 ,;,, )0()0( 00 ll llll jjmmjj r mjsn r mjsn ′=′ δ22 1 ,;,, 1 ,;,, ll 35
- 36. For hydrogen, wehave: Ry2 2 2 4 e 2 2 o 2 2 2 )0( 22 n Z em n Z a e n Z En −= −=−= h + +−= + +−= )½( 1 4 3 )½( 11 4 1 2 22)0( 22 o 2 e )0(22 R ll nn ZE nnnacm EeZ H n n α Also, from the Table for 〈rk 〉 (with k=−1 and k=−2): Therefore: This expression holds for all values of l including l=0. )½( 1111 32 o 2 22 o + == lna Z rna Z r and with α2 =(e2/hc)2 =e2/mec2ao and ao =h2/mec2. In Rydberg units: Ry + +−−= ½ 1 4 32 3 4 R lnn Z E α 36 2017 MRT
- 37. Next we considerthe Darwin term in the Schrödinger equation: 2017 MRT which is of the same order as HR. With E=−∇∇∇∇φ and φ =Ze/r: where we have used the relationship ∇2(1/r)=−4πδ (r). Since only the radial part of the wave function will influence the matrix elements of HD: and: Therefore: π 1 3 o 2 00 = an Z nψ E•≡•−=≡ ∇∇∇∇∇∇∇∇∇∇∇∇ 22 e 2 22 e 2 DDarwin 88 cm e cm e HH hh φ )( 8 π41 8 22 e 22 2 22 e 2 D r cm eZ rcm e H δ hh = ∇−= 2 100,)(, ψδ =ll nrn D 2 10022 e 22 D 2 π E cm eZ H == ψ h Thus, the matrix elements of HD are non-zero only for s states. In hydrogen: Ry3 24 3 o 22 e 3 224 D 2 n Z acmn eZ E α == h ( )only0=l 37
- 38. It is nowpossible to combine the expressions for ESO, ER and ED intoasingle expression which depends on n and j but not on l (or ml): 2017 MRT with j=l+½. When l=0, ESO=0, and: Ry − + −=++ njn Z EEE 4 3 ½ 1 3 24 DRSO α When l≠0, ED =0 and: Therefore, the combined effects of the spin-orbit coupling, the relativistic energy correc- tion, and the Darwin term are all included in ESO+ER +ED above. It is of interest to note that on the basis of the expression for ESO +ER +ED the energies of 2S1/2 and 2P1/2 are identical for a given n. The same result is obtained from the exact solution of the Dirac equation for hydrogen. Experimentally,a smalldifference (0.035 cm−1 or 1048.95 MHz for Z=1) between the energies of 2S1/2 and 2P1/2 has been observed. This is known as the Lamb shift and its explanation is based on higher-order radiative corrections (e.g., using Quantum Electrodynamics and considering electron self-energy, vertex correction, electron mass counter-terms, photon self-energy, &c. giving actually 1057.36 MHz). Ry −−=+ nn Z EE 4 3 13 24 DR α Ry − + −=+ njn Z EE 4 3 ½ 1 3 24 RSO α 38
- 39. A partial energylevel Figure of Hydrogen is shown below for the energy levels of the Hydrogen atom for n=1, 2, and 3. The energies are listed in reciprocal centimeters (cm−1) (note that this Figure is not to scale). 2017 MRT The splitting that arises within a manifold of states belonging to the same value of n is known as fine structure. Due to the Lamb shift, the 2S1/2 level of the Hydrogen atom lies 0.035 cm−1 above the 2P1/2 level: an external electric field induces Stark splitting, as a result of which the 2S1/2-2P1/2 transition becomes possible. n 2S1/2 2P1/2 2P3/2 2D3/2 2D5/2 3 2 1 97492.208 82258.942 0 82258.907 97492.198 82259.272 97492.306 97492.342 } 0.035 cm−−−−1 39
- 40. First, it isassumed that the electron (or Hydrogen atom) is placed in a constant magnetic field B with vector potential: 2017 MRT Magnetic & Electric Fields rBA ×××× 2 1 = When A has the form A=½B××××r, ∇∇∇∇•A is identically zero and as a result of which we get p•A=A••••p. We shall confine our attention, initially, to the effects which are linear in B= ∇∇∇∇××××A; hence, the Hamiltonian describing the interaction – HI for short – with the field is: But A••••p=½(B××××r)•p=½B•(r××××p)=½B•L in which L is the orbital angular momentum operator. With the replacement of ΣΣΣΣ by (2/h)S and substitution of A••••p into HI M we have: The positive constant µB is known as the Bohr magneton: BpArΒrΒpA •+•≅ •+ +•= ΣΣΣΣ××××××××∇∇∇∇ΣΣΣΣ×××× cm e cm e cm e cm e cm e H eee 2 2 e 2 e M I 22 1 22 1 2 hh Referring to the Schrödinger equation again, the interaction terms that depend on the vector potential are: AApAApp ××××∇∇∇∇ΣΣΣΣ •++•+•+=+= cm e cm e cm e m HHH e 2 2 e 2 e 2 e nInteractioo 22 )( 22 1 h )2()2( 2 e M I SLBSLB ++++++++ •=•= B cm e H µ h J/T24 e 1027.9 2 − ×== cm e B h µ 40
- 41. The equation HI M=µB B•(L++++2S) may also be written as: 2017 MRT BµBµ SL •−•−=M IH The resemblance of HI M =−µµµµL •B−µµµµS •B to the classical expression for the energy of a magnetic dipole in a magnetic field suggests that µµµµL and µµµµS be interpreted as magnetic moment operator associated with L and S, respectively. The minus signs in −µB L and −2µB S are due to the negative charge on the electron. Note that the factor of 2 appears in the relation between µµµµS and S and is absent in the relation between µµµµL and L. The latter has a classical analog but the former does not. Actually, the factor of 2 is slightly erroneous; higher order corrections shows that: although in most cases it is sufficient to set ge =2. where: SµLµ SL BB µµ 2−=−= and SµS Bµge−= with: 0023.2e =g 41
- 42. It is importantto distinguish between a magnetic moment operator µµµµ such as µµµµL and µµµµS defined by µµµµL=−µB L and µµµµS=−2µB S from the quantity µ known as the magnetic moment. The orbital magnetic moment µL is defined as: 2017 MRT llll ll === mm L z ,, µµL where µz L is the z-component of µµµµL. From µµµµL=−µB L: In other words, the absolute value of the spin magnetic moment of the electron is one Bohr magneton. So, in place of the relations µµµµL=−µB L and µµµµS=−2µB S we may now write: lllll ll BzB mLm µµµ −===−= ,,L Similarly, the spin magnetic moment µS is given by: BB BszsBs S zs g sgsmsSsmssmssms µµ µµµµ −≈−= −===−==== e e 2 1 ,,,,S SSµLµ S S S L L µ s µµ 2=== and l It is convenient, although not essential, to assume that the coordinate system has been chosen so that the z-axis coincides with the direction of B. In this case, the relation HI M =µB B•(L+2S) simplifies to: )2(M I zzB SLBH += µ where B=Bz. 42
- 43. We shall nowdivide the discussion of magnetic field effects into two parts: ‘weak’ fields and ‘strong’ fields. The scale is set by the spin-orbit interaction energy. If the changes in energy due to the application of a magnetic field are small compared with the spin-orbit coupling energy, the field is said to be ‘weak’; otherwise it is strong. The ‘weak’ field case is the regime of the ordinary Zeeman effect while the ‘strong’ field case corresponds to the Paschen-Back effect. 2017 MRT When the fields are ‘weak’ it is presumed that the effects of spin-orbit coupling have already been taken into account so that the eigenstates are described in the coupled representation |l,s; j,mj 〉. We shall therefore be interested in matrix elements of HI M in this basis set. To evaluate such matrix elements, we apply the Landé formula (which is a special form of the Wigner-Eckart Theorem and stems from irreducible tensors T(k) with k=1 which we have not discussed): But: and we have used (L ++++2S)•J=(J ++++S)•J=J2 ++++S•J. Now, since L=J−−−−S, L2 =J2 ++++S2 −2S•J and S•J=½(J2 +S2 −L2) we have: jj mmjjzj mmjsJmjs ′=′ δ,;,,;, ll jzj jj jzzj mjsJmjs jj mjsmjs mjsSLmjs ,;,,;, )1( ,;,)2(,;, ,;,2,;, ll ll ll ′ + • =+′ JSL ++++ )( 2 1 2 3 )2( 222 LSJ −+=•JSL ++++ 43
- 44. Therefore: 2017 MRT Substituting these keyresults into the Landé formula we obtain: which indicates that only diagonal elements are non-zero. Hence the energies in a “weak” magnetic field are given by: jj mmjJjzzj mgmjsSLmjs ′=+′ δ,;,2,;, ll factorLandé gg jj ssjj ssjj mjsLSJmjsmjsjmjs J jjj =≡ + +−+++ += +−+++= −+=• )1(2 )1()1()1( 1 )1( 2 1 )1( 2 1 )1( 2 3 ,;,)(,;,,;,)2(,;, 22 2 12 2 3 ll ll llll JSL ++++ These are known as the Zeeman levels with energies proportional to the magnetic number mj. Thus, the effect of the magnetic field has been to remove the mj-degeneracy. The Landé g factor may also be written as: jJB mBgE µ=M I )1(2 )1()1()1( )1(1 e + +−+++ −+= jj ssjj ggJ ll to permit the use of the more exact value of ge given by ge =2.0023. 44
- 45. It is nowpossible to define a total magnetic moment operator µµµµJ by: 2017 MRT Hence µµµµJ=−µB gJ J above may written as: and, in terms of µµµµJ, the magnetic Hamiltonian HI M =µB B•(L++++2S) is: JµJ JB gµ−= which contains µµµµL=−µB L and µµµµS=−2µB S as special cases. Along the train of development leading the matrix elements for µL and µS we have, for the total magnetic moment: jgjmjJjmjgjmjjmj JBjzjBJj J zj µµµµ −===−==== ,,,,J Jµ J J j µ = BµJ •−=M IH which then leads directly to the relation for EI M =µBgJBmj. Also,on comparing HI M =−µµµµJ •B above to HI M =−µµµµL •B−µµµµS •B, it is seen that: SLJ µµµ ++++= 45
- 46. For an electronin an s state (2S1/2), l=0, s=½, j =1/2, gJ =2 so that the energies from EI M =µB gJ Bmj are: as shown in the Figure below. 2017 MRT BE Bµ±=M I as shown in the Figure below. BBE BE BB B µµ µ 3 2 2/3 2M I 3 1 2/1 2M I 2)P( )P( ±±= ±= and The energy separations in 2P1/2 and 2P3/2 are: 2P1/2 mj EI M (1/3)µBB −(1/3)µBB (1/3)µBB +1/2 −1/2 gJ=2/3 2P3/2 mj EI M (2/3)µBB −(2/3)µBB +1/2 −1/2 gJ=4/3 2µBB+3/2 −2µBB−3/2 WEAK FIELD WEAK FIELD 2S1/2 mj EI M µBB −µBB 2µBB +1/2 −1/2 gJ = 2 WEAK FIELD 46
- 47. As the strengthof the field is increased to the point where the splitting is comparable to the spin-orbit coupling, it is no longer legitimate to isolate a single term with a specific value of j. 2017 MRT )2()(M I zzB SLBrH ++•=′ µξ SL We forgo the development (c.f., M. Weissbluth) and enunciate only the result: 2 1 2 4 9 2 1 2 1 4 9 2 1 2 1 2 1 2 6 2/1 2 5,4 2/1 2 3,2 1 +−= + − ± +−= + + ± −= += ll llll llll ll n B n n B n B n B n n B n B n B n n B n BE BBBE BBBE BE ξ µ ξ ξ µ ξ µ ξ µ ξ ξ µ ξ µ ξ µ ξ ξ µ ξ In place of HI M =µB B•(L++++2S), the Hamiltonian must now include both the spin-orbit interaction and the magnetic field terms: 47
- 48. +5 +4 +3 +2 +1 0 −1 −2 −3 −4 −5 E/ξnl +1 +2 +3 ml=1,ms=−½ ml=−1, ms=½ ml=1, ms=½ 2P1/2 2P3/2 µBB/ξnl 2017 MRT µBB >> ξnl: This is the Paschen-Back region. In this approximation the energies conform to the expression: )2(M I sB mmBE += lµ µBB << ξnl: If we confine ourselves to linear terms in µBB, the reduction of the {E1, E2,3, E4,5, E6}/ξnl gives: .,, ,,, BEBEBE BEBEBE BnBnBn BnBnBn µξµξµξ µξµξµξ 2 2 2 1 63 1 53 2 2 1 4 3 1 33 2 2 1 22 1 1 −=−−=−= +−=+=+= lll lll These energies are plotted in the Figure below for a few special case of interest such as the transition from weak to strong magnetic field for a 2P term. >>1 ln B B ξ µ ml=−1, ms=−½ ml=0, ms=−½ ml=0, ms=½ Paschen-Backregion 48
- 49. The correlation betweenthe “weak” field and “strong” field levels are shown in the Figure below. Note that states with the same value of mj (=ml +ms) do not cross. A further point to note is that, when an atom is subjected to a magnetic field, the Hamil- tonian is no longer invariant under all three-dimensionalrotationsbut only under rotations about an axis parallel to the magnetic field. In other words, the symmetry has been re- duced from O+(3) to a group called C∞. The consequence of this restriction in symme- try is that the Hamiltonian no longer commutes with J2, although it commutes with Jz. p ms EI M = µBB(ml + ms) µBB 0 +½ −½ 2µBB+½ +½ SPIN-ORBIT SPLITTING ml +½ 1 1 −1 −µBB−½ −2µBB−½ 0 −1 WEAK FIELD STRONG FIELD ξnl ½ξnl 2P1/2 2P3/2 mj = 3/2 mj = 1/2 mj = −1/2 mj = −3/2 mj = 1/2 mj = −1/2 BE Bn µξ 22 1 1 += l BE Bn µξ 3 2 2 1 2 += l BE Bn µξ 3 1 3 +−= l BE Bn µξ 3 2 2 1 4 −= l BE Bn µξ 3 1 5 −−= l BE Bn µξ 22 1 6 −= l J L S B L S B 2017 MRT 49
- 50. Electric fields mayalso have an effect on the states of an atom. This is known as the Stark effect. If the coordinate system is chosen so that the z-axis coincides with the direction of the electric field, the Hamiltonian for the interaction is: θcosE I rEezEeH == The situation of greatest physical interest is the one in which the splittings due to the Stark effect are large compared to the spin-orbit splittings. Matrix elements for the Stark effect in Hydrogen with n=2, s =½, ms = m′s. 1 cm− = 3.1 3 o Z aEe In hydrogen, assuming E =104 V cm−1 and Z =1:ZaEe o3 0 |0,0〉 |1,0〉 |1,1〉 |1,−1〉 which is considerably larger that the fine structure splitting. It is seen that because of the degeneracy of states with different l and the same n there is a linear Stark effect in hydrogen. At very high field strengths a quadratic effect appears, superimposed upon the linear effect, and results in an asymmetric shift of energy levels. 〈0,0| 〈1,0| 〈1,1| 〈1,−1| 0 ZaEe o3 2017 MRT The Hamiltonian matrix is the shown in the Table below with eigenvalues: Z aEe Z aEe E ooStark I 3 00 3 −= and,, The two states with ml =0 are shifted up and down symmetrically while the states with ml =±1 are not affected by the electric field. Thus, the ml degeneracy is only partially lifted. 50
- 51. The most importantinteraction between a nucleus of charge Ze and an electron of charge e is, of course, the Coulomb interaction −Ze2/r. All other interactions between a nucleus and the electrons of the same atom are classified as hyperfine interactions, and among these the most important are the ones that arise as a result of a nucleus possessing a magnetic dipole moment (i.e., associated with the nuclear spin) and an electric quadrupole moment (i.e., associated with a departure from a spherical charge distribution in the nucleus). In the former case the nuclear magnetic dipole moment interacts with the electronic magnetic dipole moments which are associated with the electronic orbital and/or spin angular momenta. In the latter, the interaction occurs when, at the position of the nucleus, the electronic charge distribution produced an electric field gradient with which the nuclear electric quadrupole moment can interact. Magnetic field created by the proton. Outside the proton, the field B is that of a dipole – µµµµI; inside, the field Bi depends on the exact partition of the magnetism of the proton. 5 22 o 5 o 5 o 3 π4 µ 3 π4 µ 3 π4 µˆˆˆ r rz B r zy B r zx BBBB zyxzyx − ===⇔++= IIIkjiB µµµ and, The magnetic field inside the proton, Bi, is given by (SI system): Consider a proton of radius ro. The partition of magnetism inside the proton creates a field B outside which can be calculated by attributing to the proton a magnetic moment µµµµI (which is taken to be parallel to the z-axis – see Figure). Hyperfine Interactions z y x B µµµµI Bi 3 o o 2 π4 µ r Bi Iµ= 2017 MRT where µo is the magnetic permeability of free space. ro The external field is thus purely dipolar. For r >> ro, we obtain the components of B (by calculating the rotational of AI = (µo/4π)[(µµµµI ×××× r)/r3]) (SI system): 51
- 52. To derive theform of this interaction we return to the Dirac equation(mo ≡me and q≡−e): with: 2017 MRT It is advantageous to separate the Hamiltonian into two parts: where: u e u )()( 2 1 )( ψψφ ππ ••=+′ ΣΣΣΣΣΣΣΣ K m eE φecmE cm KcmEE ++′ =−=′ 2 e 2 e2 e 2 2 and HFo e2 1 HHe c e K c e m H +=− +• +•= φApAp ΣΣΣΣΣΣΣΣ φeKH −••= )()(o pp ΣΣΣΣΣΣΣΣ HHF is the hyperfine Hamiltonian and it is the quantity we will be deriving. u e2 1 ψφψ − +• +•=′ e c e K c e m E ApAp ΣΣΣΣΣΣΣΣ which becomes (with ππππ=p++++(e/c)A): 52 and: )]()[( 2 )]()()()[( 2 2 e 2 e HF AApAAp ••+••+••= ΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣ K cm e KK cm e H
- 53. We concentrate onHHF which contains the entire dependence on the vector potential A and the last term on the right is quadratic in A; it may therefore be neglected in a first approximation. 2017 MRT Also, since: we have: and HHF becomes: )()(])()([)(()( r r rprrrrp f rrd dK ifKKffKifKifΚ hhh −=+−=−= ∇∇∇∇∇∇∇∇∇)∇)∇)∇) rrd dK K r =∇∇∇∇ rdr dK iKΚ r pp h−= ••+••−••= ))(())(( 1 ))(( 2 e HF pAArAp ΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣΣ K rdr dK iK cm e H h The potential φ is assumed to be a function of r only (i.e., φ(r)) which means that K depends only on r (i.e., K(r)) so that: Using the identity (ΣΣΣΣ•A)(ΣΣΣΣ•B)=A•B+iΣΣΣΣ•(A××××B), HHF is converted to: )]([ 1 )()( 2 e HF ArArpAAppAAp ××××ΣΣΣΣ××××××××ΣΣΣΣ •+•−+•+•+•= i rdr dK iKiK cm e H h 53
- 54. It is nownecessary to specify the vector potential A. 2017 MRT 23 ˆ rr rµrµ A ×××××××× ΙΙΙΙΙΙΙΙ == Using the vector identities ∇∇∇∇•(a××××b)=b•(∇∇∇∇××××a)−−−−a•(∇∇∇∇××××b) and ∇∇∇∇××××ka)=∇∇∇∇k××××a−−−−k∇∇∇∇××××a, and assuming that µµµµI=0 outside of the origin so that ∇∇∇∇××××µµµµI, it is found that ∇∇∇∇•A=0 and r •A= 0. We also have p•A=ih∇∇∇∇•A=A•p and p××××A=−ih∇∇∇∇××××A−−−−A××××p. The result is: •+•+•= )( 1 )(2 2 e HF ArApA ××××ΣΣΣΣ××××∇∇∇∇ΣΣΣΣ rrd Kd KK cm e H hh The nucleus is presumed to be a point dipole with a magnetic dipole moment µµµµI; hence the vector potential at r is: •+ •+ •= 2 I 2 I 3 e HF ˆ1ˆ 2 2 rrrd Kd r K r K cm e H rµ r rµ Lµ ×××× ××××ΣΣΣΣ ×××× ××××∇∇∇∇ΣΣΣΣΙΙΙΙ hh h and with A=µµµµI ××××r/r2: This expression may be put into another form by using A=(µµµµI××××r)/r3 so that: Lµprµp rµ pA •=•=•=• ΙΙΙΙΙΙΙΙ ΙΙΙΙ ×××× ×××× 333 )( 1 rrr h 54
- 55. 2017 MRT But: Therefore: )(π4 1 1111 3 rµµ µ A rµ µµµµ µ δΙΙΙΙ 2222 ΙΙΙΙ ΙΙΙΙ2222 ΙΙΙΙ ΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙ ΙΙΙΙ ×××× ×××× ∇∇∇∇××××××××∇∇∇∇××××∇∇∇∇++++××××∇∇∇∇××××∇∇∇∇ −= ∇= ∇ == −= = = rr rrrrrr ΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙ ++++++++++++++++∇∇∇∇ µkjikjirµ== ∂ ∂ + ∂ ∂ + ∂ ∂ =• ˆˆˆ)ˆˆˆ()( zyxzyx µµµzyx z µ y µ x µ 35 3 )( rrr ΙΙΙΙ ΙΙΙΙ ΙΙΙΙ ∇∇∇∇∇∇∇∇ µr rµ µ −•= • Since µµµµI is constant, the vector product terms break down as: rµ r rµrµrµ rµµ rµ µµµ µ )( 13 )()( 11 )( 111 35333 3 ∇∇∇∇∇∇∇∇++++∇∇∇∇∇∇∇∇∇∇∇∇∇∇∇∇ ∇∇∇∇∇∇∇∇∇∇∇∇∇∇∇∇ ΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙΙ ΙΙΙΙΙΙΙΙ ΙΙΙΙ ΙΙΙΙΙΙΙΙΙΙΙΙ ΙΙΙΙ •−•= • •−= • •−= • • −= •=•+ •= • rrrrrr rrrrr in first order of the Gradient, ∇∇∇∇, and then to second order via the Laplacian, ∇2. And the same for the scalar product terms: 55
- 56. With these relations,we get: Finally: 2017 MRT When these relations are included into our last development for HHF one obtains: in which: cm e B e22 hh == µandΣΣΣΣS )(π4 3 )( 35 2 rµ µr rµ µµµ A δΙΙΙΙ ΙΙΙΙ ΙΙΙΙ ΙΙΙΙΙΙΙΙΙΙΙΙ ++++−−−−∇∇∇∇∇∇∇∇××××∇∇∇∇××××∇∇∇∇××××∇∇∇∇ rrrrr •= ∇− •= = 333 )( ])[( 1 ][ 1 rrrr rµrµ rµrµrrrµrAr ΙΙΙΙΙΙΙΙ ΙΙΙΙΙΙΙΙΙΙΙΙ −−−−))))))))((((−−−−))))××××((((×××××××× • =••== •• + • + •+ •• + • = 4253HF ))(( 2)(π4 ))((3)( 2 rrrd dK rr KH BB rSrµSµ Sµr rSrµSLµ II I II µδµ −−−− with ΣΣΣΣ whose componentsare Pauli matricesσσσσ=[σ1,σ2,σ3]. We now examine K and dK/dr. rZecmE cm K 22 e 2 e 2 2 ++′ = If ϕ is replaced by Ze/r, we have: 56
- 57. It will nowbe assumed that E–mec2 <<2mec2, but that Ze2/r and 2mec2 may be comparable in magnitude. Hence: When Z=1, ro =1.4×10−13 cm; this is the nuclear dimension and is much smaller than the Bohr radius which is ao =0.52×10−8 cm (about 30000 times smaller in fact!) Thus: 2 2 2 1 2 e 2 2 e 2 o o 137 1 2 1 2 1 2 1 2 1 == = = − α c e emcm e a r h h )(1 1 )1)(2(1 1 o 2 e 2 rrrcmZe K + = + = Variation of K and dK/dr with r. K(r) rises from zero at r=0 to almost unity in a distance of seve- ral units of ro, i.e., in a distance very small compared to ao (see Figure). K(r) may therefore be approximated by a step function: ( ) ( )00)(00)( ss 2 100ss ≠==== rrKK atandat ψδψψψδψ rr because ψl≠0 =0 at r=0, and δ(r)= 0 at r≠0. Assume first, that we are dealing with an s state. Then, since K= 0 at r =0 and δ(r)=0 at r≠0: With the approximation for K it may now be shown that the δ - function term does not contribute. 0 1 2 3 4 5 6 7 8 9 10 1.0 0.9 0.8 0.7 0.6 0.5 0.4 0.3 0.2 0.1 0.0 2017 MRT K dK/dr r/ro ≠ = = 01 00 )( r r rK when when For a state with l≠ 0: ≠ = =≠≠ 0 0 0)( 00 r r atll ψδψ r 57
- 58. Therefore, our lastexpression for HHF may now be written without the δ function term: where: 2017 MRT ba HHH HFHFHF += •• + • = •• + • = 42HF 53HF ))(( 2 ))((3)( 2 rrrd dK H rr H B b B a rSrµSµ rSrµSLµ II II µ µ −−−− and where the factor K that multiplies HHF a has been set to unity. 58
- 59. Let us nowconsider HHF b and let: and, with T=B, J=S, j=s, we obtain: 2017 MRT with: and: 2 4 1 ))(( 4 1 ))(( r=••=•• rσrσrSrS jj jj jj mjmj jj mjmj mjmj ′ + • =′ ,, )1( ,, ,, J JT T Applying the Landé formula: • −−= 42 )( 2 rrdr dK B rSrS B µ BµI •−=b HHF with: ss ss ss msms ss msms msms ′ + • =′ ,, )1( ,, ,, S SB B ssBss ms rrdr dK msmsms , )( ,2,, 4 2 2 2 • −−=• rSS SB µ 59
- 60. Therefore: 2017 MRT where 〈(dK/dr)(1/r2)〉 isan integral taken over the radial part of the wave function. But, it is possible to express dK/dr by K′=4πr2δ(r): or: Substituting this into the Landé formula above gives: so that HHF b becomes: )(π4 1 2 rδ= rdr dK )(π4 rSB δµB−=• ( )½ 1 4 1 )1( 1 2, 4 1 ,2 ,, 2 222 2 =−= −+−= −−= •≡• s rdr dK ss rdr dK ms rrdr dK ms msms B BssB ss forµ µµ S SBSB SrBSB )( 3 π16 ,, 3 π16 ,, δµµ BssBss msmsmsms −=⇒′−=′ SµrBµ II •=•−= )( 3 π16 HF δµB b H 60
- 61. Collecting terms, themagnetic hyperfine Hamiltonian acquires the form: The nuclear magnetic moment operator µµµµI is related to the nuclear spin operator I by an expression of the same form that relates electronic magnetic moments to their respective angular momenta (i.e., from the Landé factor gJ): where: is the nuclear Bohr magneton. Here mN is the mass of the proton (i.e., the nuclear mass) and gN a factor whose magnitude and sign are characteristic of each nucleus(see Table). J/T27 N N 100505.5 2 − ×== cm eh µ •+ •• + • = Sµr rSrµSLµ I II )( 3 π8))((3)( 2 53HF δµ rr H B −−−− IµI NNµg= I gN µI γ Q I gN µI γ Q (×104 rad/SG) (×10−24 cm2) (×104 rad/SG) (×10−24 cm2) 1H ½ 5.586 2.793 2.675 14N 1 0.404 0.404 0.193 7.1×10−2 2H 1 0.857 0.857 0.411 2.77×10−3 15N ½ −0.566 −0.283 0.271 n ½ −3.826 −1.913 1.832 16O 0 12C 0 17O 5/2 −0.757 −1.893 0.363 −4×10−3 13C ½ 1.404 0.702 0.673 19F ½ 5.254 2.627 2.516 35Cl 3/2 0.548 0.821 0.262 −7.97×10−2 2017 MRT 61
- 62. It is alsocustomary to write the relation µµµµI =gN µNI as: where γ =gNµN /h is known as the gyromagnetic ratio. Following the discussion we had earlier for magnetic fields, the magnetic moment of the nucleus µI is defined as: 2017 MRT in which the coefficient in front of the square brackets can be put in several equivalent forms: The expression for HHF is the most common expression for the magnetic hyperfine interaction. The first two terms in the bracket taken together are known as the dipole- dipole interaction in analogy with the corresponding classical expression. The last term is the Fermi contact interaction; it has no classical analog. It’s totally ‘quantum’! NNeeNN22 µµγµµµγµ gggg BBBB =≈= hh IµI hγ= IIgImIIImIgImIImI IzII I zI hγµµµµ ======== NNNN ,,,,I which permits the magnetic moment operator to be written as: I I µ I I µ = Substituting the relation µµµµI =γ hI into our expression for HHF, we obtain: •+ •• + • = SIr rSrISLI )( 3 π8))((3)( 2 53HF δγµ rr H B −−−− h 62
- 63. An alternative expressionfor the magnetic hyperfine Hamiltonian is obtained by transforming the dipole-dipole part by means of the Landé formula. Let Then: 2017 MRT and: But since (L –S)•J=(L –S)•(L+S)=L2 −S2 and recalling that | j,mj〉=|l,s; j,mj 〉 is an eigenfunction of L2 and S2 (as well as J2 and Jz ), we get 〈 j;mj|(L–S)•J| j;mj〉= 〈 j,mj|L2 −S2 | j,mj〉=l(l+1)−s(s+1). Also r•J=r•(L+S)=r•(r××××p)/h+r•S=r•S=S•r. Therefore, as we obtained earlier (r•J)(r•S)=(r•S)(r•S)=(1/4)(ΣΣΣΣ•r)(ΣΣΣΣ•r)=r2/4. Integrating over the radial part of the wave function we obtain 〈 j,mj |B′•J| j,mj〉= −2µB[l(l+1)−s(s+1)+¾]〈1/r3〉=−2µBl(l+1)〈1/r3〉 (for s=½). so that: • +−=′ 53 )(3 2 rr B rSrSL B −−−− µ ( )½ 1 )1( )1( 2 3 = + + −=′ s rjj B for ll µB sjj jj jj mjmj jj mjmj mjmj ′ + •′ =′′ ,, )1( ,, ,, J JB B jjBjj mj rr mjmjmj , ))((3 ,2,, 53 rSJrSL SB •• +−=•′ −−−− µ 63
- 64. We may nowreplace B′ and substitute it in HHF. The Hamiltonian, for s=½, then take the form: where, for Hydrogen ψ 100 =(1/√π)ao 3/2, and the hyperfine coupling constant AF is given by: 2017 MRT The first (dipole) term in HHF is zero when l=0; on the other hand, the second (contact) term is zero when l≠0, which means, then, that the two parts of the magnetic hyperfine interaction do not overlap. For s states, we need only consider the contact interaction, whereas for non-s states, only the dipole part contributes. )( 3 π16 2 100 2 100 rδψψγµ == andhBFA JIJI SIJI •+• + + = •+• + + = FB B A rjj rjj H 3 2 1003HF 1 )1( )1( 2 3 π81 )1( )1( 2 ll h ll h γµ ψγµ As in the case of spin-orbit coupling, the latest form of HHF suggest that it would be useful to couple the angular momenta I and J to form a new angular momentum operator: with the notation |j;mj〉, |I;mI〉 and |F;mF〉 to designate the eigenfunctions belonging to J, I and F, respectively. SLIJIF ++=+≡ 64
- 65. For Hydrogen inan s state only the contact term is effective. Since l=0, J may be replaced by S so that: and, since the spin of the proton is I=½, we have also s=½ thus F=0 & 1. Hence: which indicates that the only diagonal elements are non-zero. For the two possible values of F, the energies are: ( ) ( ) =−=− == = 0 4 3 π4 1 4 1 3 π4 2 100 2 100 FA FA E FB FB ψγµ ψγµ h h )( 2 1 222 ISFSI −−=• −+= +−+−+=−−=• 2 3 )1( 2 1 )]1()1()1([ 2 1 ,)(½,,, 222 FF IIssFFmFmFmFmF FFFF ISFSI 2S1/2 0.047 cm−1 (or 21 cm) F = 1 F = 0 n = 1 Magnetic hyperfine splitting of the ground state of hydrogen. The entire splitting is due to the Fermi contact term geµBgNµN (i.e., a quantum effect.). o 2 100 3 61 3 6π1 a AE B FB h h γµ ψγµ ===∆ For hydrogen, |ψ 100|2 =1/πao 3 (ao =0.529×10−8 cm), γh =µN gN = 5.05×10−23 erg/GHz and with µB =0.927×10−20 erg/GHz, the difference in energy between the two levels for E is: 2017 MRT as show in the Figure. If we set ∆E= hν, the frequency ν is 1420.4058 MHz, which corresponds to a wavelength of 21 cm. 65
- 66. Next, we considerthe dipole-dipole term in our last expression for HHF. Also, we have I•J=½(F2 −I2 −J2) and 〈F,mF|I•J|F,mF〉=½[F(F+1)−I(I+1)− j( j+1]. Again, only the diagonal matrix elements are non-zero. Therefore the energies are given by: lll )½)(1( 11 33 o 3 3 ++ = na Z r For hydrogen, we use from the Table for 〈rk〉: )]1()1()1([ )½)(1(33 o 3 +−+−+ ++ = IIjjFF jjna Z E B l hγµ 2017 MRT 66
- 67. For the state22P1/2, we have Z=1, j=1/2, I=½, n=2 and l=1, which gives: 2017 MRT which is smaller, by a factor of 24, than the splitting due to the Fermi (1901-1954) contact interaction (i.e., the term geµBgNµN way above.) ( ) ( ) −=∆ = − = = 3 o 3 o 3 o 99 2 0 6 1 1 18 1 a E F a F a E B B B h h h γµ γµ γµ Similarly, for the state 22P3/2, we have Z=1, j=3/2, I=½, n=2 and l=1, which gives: ( ) ( ) =∆ = − = = 3 o 3 o 3 o 945 4 1 18 1 2 30 1 a E F a F a E B B B h h h γµ γµ γµ 67
- 68. The quadrupole momentof a nucleus is a measure of the departure of the mean distribution of nuclear charge from spherical symmetry. It is positive for a distribution which is prolate ellipsoid (e.g., like a football), negative for an oblate (e.g., like a door knob), and zero for a spherically symmetric distribution. Some nuclei that possess quadrupole moments are 2H (+), 14N (+), 17O (−), and 35Cl (−). Referring to a coordinate system (see Figure) whose origin is located within the nucleus, the electrostatic interaction, W, between a single electron (with charge e) and a nucleus containing Z protons is: ∑= = Z e W 1p pe 2 rr −−−− Coordinate system for the equation W=Σp (e2/|re – rp|) . ∑ ∑ ∞ = −= + > < + = 0 1eepp * pe ),(),( 12 1 π4 1 l l l l l ll l ll lm mm r r YY ϕθϕθ rr −−−− Another variation of this equation is obtained by writing: 2017 MRT From a relation we obtained earlier – that is 1/|r1 −−−− r2|= ΣlΣml [4π/(2l +1)](r< l/r> l+1)Yl ml*(θ1,ϕ1)Yl ml (θ2,ϕ2) – we get: in which re is the position vector of the electron, rp is the position vector of the p-th proton, and the sum is taken over all Z protons. ∑−= =•=• l l ll llll l ll m mm YY ),(),(),(),( eepp * ee )( pp )()( e )( p ϕθϕθϕθϕθ YYYY ∑ ∞ = + > < • + = 0 1 )( p )( e pe 12 1 π4 1 l l l ll l r r YY rr −−−− Substitution in 1/|r1 −−−− r2|above yields: z y x rp re |re − rp | e Z eO 68
- 69. For an electronthat does not penetrate the nucleus, re >rp, so that W above becomes: 2017 MRT such that: ∑∑ ∑= ∞ = −= ++ −= Z m mm r r YYeW 1p 0 1 e p eepp *2 ),(),( 12 1 π4 l l l l l ll l ll l ϕθϕθ When l=0, W becomes: ( )0 e 2 )0()0( Coulomb =−=•= l r eZ W UQ It is possible to express W more compactly by writing: ),(),(),(),()1(),(),( ee )( pp )( eeppeepp * ϕθϕθϕθϕθϕθϕθ ll l l ll l l ll l lll l ll YY •=−= ∑∑ −= − −= m mmm m mm YYYY 1 e ee )()( 1p ppp )()( 1 ),( 12 π4 ),( 12 π4 + = + −≡ + ≡ ∑ l lllll ll r ere Z ϕθϕθ YUYQ and We then have: )3()2()2()1()1()0()0( 0 )()( OW +•+•+•=•= ∑ ∞ = UQUQUQUQ l ll which is the ordinary Coulomb interaction with Z being the sum over the protons.* * For a nucleus of finite size, there is a correction term to be added to W (i.e., (2π/3)Ze2|ψ100 |2 〈R2〉 in which 〈R2 〉 is the mean square charge radius of the nucleus and e2|ψ 100 |2 is the electronic charge density at the nucleus.) 69
- 70. The term withl=1 in W=ΣlQ(l)•U(l) vanishes (i.e., Q(1)•U(1) =0) because it corresponds to the interaction between a nuclear electric dipole moment and the electric field established by the electrons. 2017 MRT We will now suppose that the electronic state is characterized by angular momentum quantum numbers j,mj; the nuclear state by I,mI; and when the two angular momenta are coupled, the quantum numbers are I, j, F,mF. We shall compute the interaction energy associated with HQ in the latter representation. The pertinent expression is: which are the matrix elements of the scalar product T(k)•U(k) in the coupled representation. In the present context, this expression becomes: The next term, with l=2, is the electric quadrupole interaction: )2()2( Q UQ •=W 2 )( 21 )( 1 12 21 21 )()( 21 12 )1(,;,,;, jjjj kjj jjj mjjjmjjj kk mmjj jjj j kk j jj ′′ ′′ −=′′′′• ′′ ′++ UTUT δδ jjII Ij FjI mFjImFjI FF mmFF IjF FF ′ −= ′′• ′′ ++ )2()2( )2()2( 2 )1( ,;,,;, 1 UQ UQ δδ where the quantities {: : :} are called 6j-symbols and they are usually found in spec- ialized tables(Refs) and 〈I||Q(2)||I〉〈 j||U(2) || j〉 are called the reduced matrix elements. 70
- 71. In such tableswe find (e.g., with s=a+b+c and X=a(a+1)−b(b+1)−c(c+1)): 2017 MRT where X=I(I+1)+ j( j+1)−F(F+1). 2/1 )]32)(22)(12(2)12)(32)(22)(12(2)12[( )]1()1(4)1(3[2 )1( 2 +++−+++− ++−+ −= cccccbbbbb ccbbXX bc cba s Themainproblemthen is to evaluatethe reduced matrix elements 〈I||Q(2)||I 〉〈 j ||U(2) || j〉. These 6j-symbols are invariant under: 1) an interchange of columns and; 2) an interchange of any two numbers in the bottom row with the corresponding two numbers in the top row. )32)(22)(12(2)12)(32)(22)(12(2)12( )]1()1(4)1(3[2 )1( 2 +++−+++− ++−− −= ++ jjjjjIIIII jjIIXX Ij FjI jIF So, by just interchanging the first and third columns, we find ours: 71
- 72. First consider 〈I||Q(2)||I 〉. This quantity can be related to the nuclear quadrupolar moment Q which is defined by: 2017 MRT Since: )2( 1p 2 ppp 0 2 p 2 p 2 p 2 ),( 5 π4 2)3( O Z Q e rYrz ==− ∑∑ = ϕθ or: ImIrzImIQ II =−== ∑ ,)3(, p 2 p 2 p ImIQImIQe IOI === ,, 2 1 )2( It is now possible to invoke the Wigner-Eckart theorem: II mm II ImIQImI II mI IOI I )2()2( 0 2 )1(,, Q − −=== − and, on setting mI =I and evaluating the 3j-symbols, we get: IIIIIIIImIQImI IOI )2()2( )32)(1)(12)(12(,, Q+++−=== Qe II IIII II )12( )32)(32)(1)(12( 2 1)2( − ++++ =Q where the second equality comes from(i.e.,forl=2)Q(2)=eΣp√(4π/2⋅2+1)Yp (2)rp 2,we have: 72
- 73. The computation of〈 j ||U(2) || j 〉 proceeds in analogous fashion. This time we define a quantity eQ/2 as: 2017 MRT where, from (i.e., for l=2) U(2) =−e√(4π/2⋅2+1)Ye (2) /re 2+1, we get: − −=−= 5 e 2 e 2 e 3 e ee 0 2 )2( 3 2 11 ),( 5 π4 r rz e r YeUO ϕθ We note that ∂2(−e/r)/∂z2 =−e(3z2 −r2)/r5 is the zz (diadic) component of the electric field gradient tensor produced by an electron at a point whose coordinated with respect to the electron are [x,y,z]. Since the origin of the coordinate system (see previous Figure) has been positioned at the nucleus 2UO (2) in the equation above is the zz component of the electric field gradient tensor at the nucleus produced by an electron at re, or UO (2) = ½(∂2V/∂z2)O =½Vzz where V is the potential due to the electron and the second derivative is evaluated at the origin O (i.e.,at nucleus).We then have eq=〈 j,mj =j|Vzz | j,mj =j〉=〈Vzz〉 which is the average (or expectation value) of Vzz taken over the electronic state | j, j〉. jmjUjmjQe jOj === ,, 2 1 )2( qe jj jjjj jj )12( )32)(32)(1)(12( 2 1)2( − ++++ =U Again, the use of the Wigner-Eckart theorem leads to the result: 73
- 74. On substituting thekey results into 〈I, j;F,mF |Q(2) ••••U(2) |I, j;F,mF 〉 one obtains: 2017 MRT in which e2qQ is known as the quadrupole coupling constant and X is the same quantity we identified earlier (i.e., X=I(I+1)+ j( j+1)−F(F+1)). The quadrupole coupling constant may be positive or negative. This is the electric quadrupole interaction Hamiltonian. ++−− −− =′′• )1()1()1( 4 3 )12()12(2 ,;,,;, 2 )2()2( jjIIXX jjII qQe mFjImFjI FF UQ )1()1()1( 4 3 ,;,)( 2 3 )(3,;, 222 ++−−=′′ −•+• jjIIXXmFjImFjI FF JIJIJI which, when compared to our previous result for 〈I, j;F,mF |Q(2) ••••U(2)|I, j;F,mF 〉 gives: −•+• −− =•= 222 2 )2()2( Q )( 2 3 )(3 )12()12(2 JIJIJIUQ jjII qQe H The above equation can be cast into another form.From F=I++++J and −2I•J=I2 +J2 −F2, as well as 〈I, j;F,mF |−2I••••U|I, j;F,mF 〉=I(I+1)+ j( j+1)−F(F+1)=X, we get: 74
- 75. Now (without proof– c.f. M. Weissbluth) assuming axial symmetry, Vxx =Vyy, we have: 2017 MRT where Vzz =eq. For this case there are only diagonal elements with twofold degenerates in mI, that is, states with ±mI have the same energy. )]1(3[ )12(4 ,, )]3([ )12(4 2 2 QQ 22 Q +− − == − − = IIm II qQe mIHmIE IIV II eQ H III zzz i. When I=1, the energies are: ( ) ( ) =− ±=+ =−=+− − = 0 2 1 1 4 1 )23( 4 1 )]11(13[ )11.2(1.4 2 2 222 2 Q I I II mqQe mqQe mqQem qQe E I = 1 mI EQ (1/4)e2qQ±1 0 −(1/2)e2qQ and the Figure below shows the quadrupole splitting (when I=1 and e2 qQ>0). As has already been noted, the quadrupole interaction vanishes when I=0 or ½. 75
- 76. ii. For I=3/2,the energies are: 2017 MRT ( ) ( ) ±=− ±=+ = −=+− − = 2/1 4 1 2/3 4 1 4 15 3 12 1 )]12/3(2/33[ )12/3.2(2/3.4 2 2 222 2 Q I I II mqQe mqQe mqQem qQe E and the Figure below shows the quadrupole splitting (when I=3/2 and e2 qQ>0). and the Figure below shows the quadrupole splitting (when I=2 and e2 qQ<0). I = 3/2 mI EQ (1/4)e2qQ±3/2 −(1/4)e2qQ±1/2 I = 2 mI EQ −(1/8)e2qQ±1 −(1/4)e2qQ0 (1/4)e2qQ±2 iii. Finally, for I=2, the energies are: ( ) ( ) ( ) =− ±=− ±=+ =−=+− − = 0 4 1 1 8 1 2 4 1 )63( 24 1 )]12(23[ )12.2(2.4 2 2 2 222 2 Q I I I II mqQe mqQe mqQe mqQem qQe E 76
- 77. And now weremind ourselves of the The Dirac Equation chapter at end of PART IV – QUANTUM FIELDS by recalling the Dirac equation with electromagnetic coupling. We obtained the equation for an electron to order v2/c2: 2017 MRT where E′=E−moc2 and e is the electronic charge. This equation, which may be regarded as the Schrödinger equation for an electron interacting with fields describable by the potentials A and ϕ, is the starting point for discussions of atomic and molecular properties. ψϕϕ ψϕ •−•−− −•+ +=+′ p AAp ××××∇∇∇∇σσσσ∇∇∇∇∇∇∇∇ ××××∇∇∇∇σσσσ 22 o 22 o 2 23 o 4 o 2 o 488 22 1 )( cm e cm e cm p cm e c e m eE hh h 77 In the case of hydrogen energy levels En j are described by the total angular momentum by the total quantum number j=l+s=1/2,3/2,…(N.B.,l=0,1,2,…ands=½) with: with the principle quantum number n = n′+j +½ =1,2,… (n′=0,1,2,…). The electromag- netic fine structure constant is α =e2/4πhc≅1/137 and F=I++++J (i.e.,F=I++++ L++++S) which represents the vector coupling of nuclear spin I and total angular momentumJ=L++++S. ... )½)(1( )1()1()1( 4 3 ½ 11 1 2 1 33 o 2 2 2 e 22 e + ++ +−+−+ + − + +−= l h jjna IIjjFF njnn cmcmE Bjn γµ α α
- 78. This is theenergy associated with the scalar potential energy, ϕ (105 cm−1). And the significance of the various terms and their energies, is indicated to within an order of magnitude (N.B., the ‘cm−1’ scale is given by the wave number 1/λ ≅ 8000 cm−1): This contains the kinetic energy (i.e., p2 /2me) and interaction term (i.e., (e/2mec)(p • A+ A • p) + e2A2/2mec2) with a field represented by a potential vector A (105 cm−1). The interaction terms are responsible or contribute to numerous physical processes among which are absorption, emission and scattering of electromagnetic waves, diamagnetism, and the Zeeman effect. 2017 MRT ϕe The spin-orbit interaction (10-103 cm−1). More precisely, itis (eh/8mec2){σσσσ •[p −−−− (e/c)A]×××× E −−−− σσσσ • E ×××× [p −−−− (e/c)A]} and it arises from the fact that the motion of the magnetic moment gives rise to an electric moment for the particle which then interacts with the electric field. This term appears in the expression of the relativistic energy: and is therefore a relativistic correction to the kinetic energy (i.e., p2/2me) (0.1 cm−1). The interaction of the spin magnetic moment (i.e., µS = 2⋅e/2me⋅h/2) with a magnetic field B= ∇∇∇∇ ×××× A (1 cm−1). Thus, it is the magnetic moment of one Bohr magneton, eh/2mec (i.e., µB = 9.2741×10−24 A⋅m2 or J/T) with the magnetic field. 2 e2 1 + Ap c e m A××××∇∇∇∇σσσσ • cm e e2 h 23 e 4 8 cm p L+−+≅+ 23 ee 2 2 e 2222 e 82 )( cm p m p cmcpcm 4 ϕ∇∇∇∇∇∇∇∇ •− 22 e 2 8 cm eh This term produces an energy shift in s-states and is known as the Darwin (1887-1962) term (< 0.1 cm−1). It is thus a correction to the direct point charge interaction due to the fact that in the representation (Foldy-Wouthuysen), the particle is not concentrated at a point but is spread out over a volume with radius whose magnitude is roughly that of a Compton wavelength, h/mec. p××××∇∇∇∇σσσσ ϕ•− 22 e4 cm eh ϕe c e m − + 2 e2 1 Ap As a combination, this term represents the interaction of a point charge with the electromagnetic field. 78
- 79. In the previouschapters we solved the Schrödinger equation exactly for a simple Coulomb potential energy function as it applies to the hydrogenic atom. In general, for any arbitrary potential, it is not possible to solve the Schrödinger equation exactly and we wish to take up in this last chapter approximation methods of particular utility for problems in which the potential energy function does not differ much (i.e., is only slightly perturbed) from that in a simple, previously solved problem. This is called perturbation theory and our goal in this chapter is to apply it to molecular structures. 2017 MRT Multi-Electron Atoms and Molecules 79 There are many cases that can be handled by perturbation theory; here we will be particularly interested in establishing procedures whereby various problems in atomic spectroscopy can be handled. For example, these would include: 1. The spin-orbit interaction which results from the interaction of the intrinsic magnetic moment of the electron about the nucleus; 2. The superposition of an externally applied magnetic field on the usual Coulomb interaction between an electron and the positively charged nucleus; 3. The problem of emission or absorption of electromagnetic radiation by atomic electrons; and 4. The electrons in a Helium atom whose wave functions would exactly correspond to the wave function of the electron in a Helium ion but for the slight disturbance or perturbation caused by the added repulsion of the two electrons; The first two are discussed in the text whereas the third deals with interactions and is considered in the Appendix. Onto the fourth one then! But first, let us refresh the postulates of quantum mechanics so as to use them in perturbation theory.
- 80. In developing theperturbation theory we will have occasion to express various wave functions in the following manner: 2017 MRT where the Cs are constants and where the ϕ s form a complete set of orthogonal functions. The orthogonality property, it will be recalled, is: ∑= n nnC ϕψ 80 where the integration is extended over all space, and the completeness property means that any arbitrary ψ can be put into this form. Let us agree to multiply each ϕ by a suitable constant so as to make it satisfy the condition: ( )mndmn ≠=∫ if0* τϕϕ 1 2 =∫ τϕ dn which is called the normalization condition. Then, for any ψ , we may calculate the coefficients Cn as follows: Multiply the equation for ψ given above by the conjugate ϕ m * and integrate over all space: m n nmnm CdCd == ∑ ∫∫ τϕϕτψϕ ** where use was made of the orthogonality and normalization conditions. Thus, given the function ψ , we can easily calculate Cm by evaluating the integral.
- 81. Let A beany Hermitian operator, with eigenvalues an and eigenfunctions ϕn: 2017 MRT It can be shown, using the Hermitian property, that any two eigenfunctions ϕn and ϕm are orthogonal, provided the eigenvalues an and am are distinct. If, however, ϕn and ϕm are two distinct eigenfunctions belonging to a single eigenvalue (i.e., the eigenvalue is degenerate in this case), then they need not be orthogonal, but they can be made so by a procedure to be explained next. nnn aA ϕϕ = 81 Note that the expression of the solution to a given wave equation in terms of a sum of wave functions which are solutions to a different wave equation is similar, for example, to the superposition of plane waves in simple Fourier analysis where any function may be represented by a sum of sinusoidal functions having specified amplitudes. See it this way: A single complicated electromagnetic disturbance can be represented as a sum, or superposition, of many simple plane waves of differing frequency whose amplitudes are found by doing Fourier analysis. Any attempt to measure the intensity of a single wave present in the summation representing the single complicated disturbance would yield a positive result with a relative intensity identical with the square of the absolute value of the computed amplitude. Similarly we may represent a given quantum mechanical state by a superposition of states of the complete orthonormal set of solutions to a wave equation! As such, it is most convenient to choose solutions to a wave equation closely corres- ponding to the actual wave equation we wish to solve or discuss.
- 82. Eigenfunctions of thewave equation having different energy eigenvalues are necessarily orthogonal, as we will now show. Let ψi and ψj be solutions of: 2017 MRT If the integration is extended over all space, we obtain: 0)( 2 * 2 2 *2 2 *2 2 *2 2 2 2 2 2 2 * =−+ ∂ ∂ + ∂ ∂ + ∂ ∂ − ∂ ∂ + ∂ ∂ + ∂ ∂ ∫ ∫ ∫ ∫ ∫ ∫ ∞ ∞− ∞ ∞− ∞ ∞− ∞ ∞− ∞ ∞− ∞ ∞− zdydxdEE m zdydxd zyxzyx ijji i jjjiii j ψψ ψ ψψψψψψ ψ h 82 0)( 2 0)( 2 * 2 *2 2 2 =−+∇=−+∇ jjjiii VE m VE m ψψψψ hh and Multiplying the terms in the first equation by ψ j * and the terms in the second by ψi on the right, and subtracting the second equation from the first, we obtain; 0)( 2 )( * 2 *22* =−+∇−∇ ijjiijij EE m ψψψψψψ h or integrating over the particle coordinates after representing the system in Cartesian coordinates, we obtain: 0)( 2 ])([ * 2 *22* =−+∇−∇ ∫∫ τψψτψψψψ dEE m d ijjiijij h
- 83. Since: 2017 MRT because of theboundary conditions on ψ, we get as a result: 1* =∫∫∫ τψψ dij 83 0 * * * * 2 *2 2 2 * = ∂ ∂ − ∂ ∂ = ∂ ∂ − ∂ ∂ ∂ ∂ = ∂ ∂ − ∂ ∂ ∞ ∞− ∞ ∞− ∞ ∞− ∫∫ i ji ji ji ji ji j xx xd xxx xd xx ψ ψψ ψψ ψψ ψψ ψψ ψ 0)( 2 * 2 =− ∫ ∫ ∫ ∞ ∞− ∞ ∞− ∞ ∞− zdydxdEE m ijji ψψ h Therefore the normalized wave functions are orthogonal, for either i= j and: or i≠ j in which case Ei ≠ Ej and: 0* =∫∫∫ τψψ dij
- 84. In the first-orderperturbation theory,we start with the famous Schrödinger equation: 2017 MRT which may contain a potential energy term V which is only slightly different from the potential energy V 0 of a problem already solved. Since we should expect the solutions of the Schrödinger equation above in a perturbation problem to differ very little from the solution found with V 0, we will use the wave function for V 0 in attempting to expand the solutions to the Schrödinger equation above in terms of known functions. For a given wave function ψi, let: 02 2 0000 2 V m HEEEVVV iiiiii +∇−=′+=′+=′+= h and,, ψψψ 84 0)( 2 2 2 =−+∇ ψψ VE m h where ψ 0 i and E0 i are the solutions (i.e., eigenfunctions) and permitted energy values (i.e., energy eigenvalues) of the unperturbed Schrödinger equation: 0)( 2 000 2 02 =−+∇ iii VE m ψψ h which may be written more compactly as: 0000 iii EH ψψ = The subscript i, distinguishing the i independent energy eigenvalues for the system, takes on different values with any change in the separate eigenvalues (e.g., n,l,ml) of the wave function.
- 85. Substituting V =V0 +V ′, Ei =E0 i +E′i and ψi =ψ 0 i +ψ ′i into the Schrödinger equation above and grouping the result in ascending order of approximation, we have: 2017 MRT This first term is zero by comparing to the unperturbed Schrödinger equation, leaving us with only the second term for a first-order approximation: 0])(-)()[()( 000000 =′′−′+′−+′−+− iiiiiiii EVEVEHEH ψψψψ assuchtermsordersecond 85 0)()( 000 =′−′+′− iiii EVEH ψψ We now expand ψ ′i in terms of the complete orthonormal set of solutions to the unperturbed Schrödinger equation, ψ 0 j. That is, we let: ∑ ∞ = =′ 0 0 j jjii a ψψ which, with first-order approximation above, gives: 0)()( 0000 =′−′+−∑ ii j jjii EVaEH ψψ Note that from H0ψ 0 i =E0 iψ 0 i, this last equation can be rewritten: 0)()( 0000 =′−′+−∑ ii j jjiij EVaEE ψψ Multiplying this last equation by ψ 0 k * on the left and integrating over all space, we get: 0)( 0*00*00*000 =′−′+− ∫∫∑ ∫ τψψτψψτψψ dEdVdaEE ikiik j jkjiij
- 86. Since the ψ0 i s are orthonormal, all but one term in the summation is zero, and this last equation becomes: 2017 MRT If k=i, E0 i −E0 k =0 and the shift in energy, E′i, due to the perturbation is: 00 0*0 ik ik ki EE dV a − ′ = ∫ τψψ 86 0)( 0*00*000 =′−′+− ∫∫ τψψτψψ dEdVaEE ikiikkiik iVi dVE iii ′≡ ′=′ ∫ τψψ 0*0 where 〈i |V ′|i〉 spell out the diagonal matrix elements (i.e., coefficients). If k≠i, the third term is zero and the aik are given by: aii is not given by this last result but we already know its value; aii =1 approximately since ψ i ~ψ 0 i : 11 22 =−= ∑≠ij jiii aa up to and including terms of first order in the perturbation.
- 87. Note that thefirst-order shift in energy from the unperturbed energy level, given by E′i = ∫ψ 0 i *V′ψ 0 i dτ, is just the perturbed potential energy averaged over the unperturbed wave functions: 2017 MRT where 〈k |V ′|i〉 spell out the off-diagonal matrix elements. We obtain thus the following expressions for the energy and wave functions of the perturbed system to first order: 0 0 00 00 k ik k ki ik iiiiii EE V VEE ψψψ ∑ ∞ ≠ = − ′ +=′+= and 87 iVkdVV ikik ′≡′=′ ∫ τψψ 0*0 Note also that the effect of the perturbing potential is to give the particle a small but finite probability of occupying states of the unperturbed system other than the single unperturbed state it would be in if no perturbing potential existed. At this point we will stop but not before mentioning that there also exists the possibility of developing a second-order perturbation theory. This is needed when the first-order terms are zero or in general if a better approximation is needed, the second-order terms must be kept. We can generalize the result further then by assuming an additional correction to the potential V ′′, so that our result may be applied also to nondegenerate cases in which a further, smaller physical effect may be estimated in addition to a larger perturbing potential. For this case we would let: iiiiiiii EEEEVVVV ψψψψ ′′+′+=′′+′+=′′+′+= 000 and,
- 88. A good first-orderexample is to take the total potential energy for the Helium atom as: 2017 MRT with the electronic charge e, the Helium nucleus (i.e.,Z) charge 2e (i.e., 2 protons of charge +e), r1 and r2 are the electron-nucleus separations distances for each electron, and r12 is the separation of the two electrons from each other. Note that the potential above conveniently arranges as V =V1 0 +V2 0 +V ′ where V2 0 and V2 0 are the hydrogen-like potential functions of the two electrons in the nuclear Coulomb field and their interaction energy e2/r12 is treated as a perturbation V ′. If derivatives with respect to a particular electron’s position are denoted by a subscript 1 or 2, we can write the Schrödinger equation as: 12 2 2 2 1 2 22 2 1 21 r e r e r e V +−−= = ++ electronto electron electron tonucleus electron tonucleus 88 0)( 2 0 2 0 12 2 2 2 1 =′−−−+∇+∇ iiii VVVE m ψψψ h Letting a superscript zero represent the zero-order wave function, the total wave function ψ 0 i may be written as the product ψ1 0 iψ 2 0 j of the wave functions of the two electrons taken separately, where ψ1 0 i for example is a solution of: 0)( 2 0 1 0 1 0 12 0 1 2 1 =−+∇ iii VE m ψψ h or: 0 1 0 1 0 1 0 1 iii EH ψψ = and E0 i =E1 0 i +E2 0 i. The ψ 0 i are the wave functions of prior chapters (e.g.,ψnlml (r,θ,ϕ)).
- 89. The first approximationto the energy of the Helium atom is given by substituting these wave functions into Ei =E1 0 i +E2 0 i +V′ii (e.g., E1 0 i =−(meZ2e4/2h2)(1/n1 2)): 2017 MRT with n1 and n2 are the total quantum numbers of the unperturbed hydrogen-like wave functions. For the particular case of the ground state of the Helium atom, n1 =n2 =1 and l =ml =0. Each of the one-electron wave functions is an exponential times the zero-order Laguerre polynomial (a constant), so that the normalized wave functions are: ∫+ +−= τψψψψ d r e nn eZm E nnnni 0 ),(2 0 ),(1 12 2 *0 ),(2 *0 ),(12 2 2 1 2 42 e 22112211 11 2 llll h 89 22 3 0 3 3 0 3 0 )0,1(2 0 )0,1(1 21 ee ππ ρρ ψψ −− = a Z a Z where a0 =h2/mee2, ρ1 =2Zr1/a0, and the energy E1 is given by one-half the first term in the equation for Ei above. Since in spherical coordinates, the volume element is dτ = r1 2sinθ1dr1dθ1dϕ1r2 2sinθ2dr2dθ2dϕ2 the integral in Ei above becomes: ∫ ∫ ∫ ∫ ∫ ∫ ∞ ∞ −− −=′ 0 π 0 π2 0 0 π 0 π2 0 2222 2 21111 2 1 12 22 0 2 2 1 sinsin ee )π4(2 21 ϕθρθρϕθρθρ ρ ρρ dddddd a eZ E where ρ12 =2Zr12/a0. Note that the integration over a six-dimensional space, since the overall wave function is concerned with the positions of two particles.
- 90. The integrand inthe last monster integral is essentially the electrostatic interaction energy between two shells of charge density exp(−ρ1) and exp(−ρ2). To begin evaluating it let us consider just the integral over ρ1. Let us consider further the potential at a point ρ due to a shell of thickness dρ1 at ρ1; it is given by: 2017 MRT and )(eπ4 e π4 111 1 12 1 1 1 ρρρρ ρ ρ ρ ρ ρ <= − − ford d 90 )(e π4e π4 11 2 1 12 1 1 1 ρρρρ ρρ ρ ρ ρ ρ >= − − ford d )]2(e2[ π4 e π4 eπ4)( 1 2 111 11 +−=+= − ∞ − ∞ − ∫∫ ρ ρ ρρ ρ ρρρφ ρ ρ ρ ρ ρ dd The total potential at ρ due to the infinite sphere of charge density exp(−ρ1) is given by: This is the potential at a point ρ due to the entire distribution of charge density exp(−ρ1).
- 91. We can nowevaluate the potential energy of interaction between the distribution of charge density exp(−ρ1) and exp(−ρ2). The potential energy per unit volume of a charge density exp(−ρ2) at a point ρ2 is given by (4πρ2)[2−(ρ2 +2)exp(−ρ2)]exp(−ρ2). Hence, the second shell of charge 4πρ2 2exp(−ρ2)dρ2 has a potential energy of: 2017 MRT 91 ])2(e2[ eπ)4( 2 2 2 2 2 2 2 2 +− − − ρ ρ ρρ ρ ρ d The total electrostatic potential of the sphere of charge density exp(−ρ2) due to the sphere with charge density exp(−ρ1) is the integral over ρ2 of this last expression: 2 0 222 2 π)4( 4 5 ])2(e2[eπ)4( 22 =+−∫ ∞ −− ρρρ ρρ d Therefore our monster integral yields a result of: 2 4 e 0 2 1 24 5 24 5 h eZm a eZ E ==′ and from Ei =−(meZ2e4/2h2)(1/n1 2 +1/n2 2)+ ∫ψ 1 0* (1,0)ψ 2 0* (1,0)(e2/r12)ψ 1 0 (1,0)ψ 2 0 (1,0)dτ above: −−= ZZ em E 4 5 2 2 2 2 4 e 1 h where mee4/2h2 is the ground state energy of the Hydrogen atom and 2Z 2 times this is the unperturbed ground state energy of the two Helium electrons.
- 92. The correction tothe zero-order ground state energy of the Helium atom is, as we should suspect, large, being (5/4)(Z/2Z2)=(5/8)Z ~30% of the zero-order energy, and is subtractive since the effect of the electron-electron interaction is to detract from or put up a shield reducing the electron-nucleus interaction. The approximation is found surprisingly good, however, in view of the size: The observed ground state energy of the Helium atom is 78.6 eV, the zero-order calculated energy is 108.2 eV, and the first-order calculated energy is 74.4 eV. Thus, the zero-order calculation is found to be 38% too high while the first-order calculation is within 4.2 eV of the observed value, only 5% too low. Later, using the same wave functions we will take up a slightly different method which will reduce the error to only 2% of the observed value. 2017 MRT 92 Now we are ready to consider generalize the situation where there is more than one electron in the atomic system. To do this, we first take up the problem of a system containing two identical particles and then apply the results to the two-electron Helium atom again.
- 93. For a systemcontaining two identical particles (e.g., the two electrons in the Helium atom), one cannot distinguish between two eigenfunctions of the system which differ only in that the particles are interchangeable. They are both eigenfunctions of the same eigenvalue. For example, if x denotes spin and space coordinates, then in: 2017 MRT ),(),(),(),( 12122121 xxExxHxxExxH ψψψψ == and 93 ψ (x1,x2) and ψ (x2,x1) are degenerate eigenfunctions of the H operator. The exchange operator consists in exchanging the two particles, and if ψ is an eigenfunction of the exchange operator P then: ),(),( 1221 xxkxxP ψψ = where k is the eigenvalue of the exchange operator. When this exchange operation is taken twice, one must end up with the original eigenfunction: ),(),(),( 2112 2 21 2 xxxxkxxP ψψψ == Therefore k=±1 and: ),(),(),(),( 12211221 xxxxPxxxxP ψψψψ −=+= or This first equation defines a symmetric eigenfunction and the second an antisymmetric eigenfunction. If the state is degenerate and two or more eigenfunctions are possible for a given eigenvalue, it is possible to construct combinations of these eigenfunctions which are either entirely symmetrical or entirely antisymmetrical.
- 94. Consider a systemof two identical particles such that one particle is in a state labeled α and the other in another state labelled by β where α(1) represents particle 1 in state α, &c. If particles 1 and 2 are interchangeable in either the α(1)α(2) or β(1)β(2) state, then eigenfunction is unchanged and therefore these states are said to be symmetric. The other states α(1)β(2) and β(1)α(2) are neither entirely symmetric nor entirely antisymmetric, however, and it is convenient to treat them as a superposition of two other states one of which is entirely symmetric and the other antisymmetric. We therefore use linear combinations of the α(1)β(2) and β(1)α(2) states to represent the two remaining wave functions of the system: 2017 MRT where the factor 1/√2 is used for normalization. Since the α(1), β(2), α(2), and β(1) are all solutions of the Schrödinger equation, the ψS and ψA will also be solutions. Now, in our universe, systems of identical particles with integer spin must be represented by wave functions which are symmetric with respect to the exchange of any two particles. Similarly, all systems of identical particles with half-integer spin must be represented by wave functions which are antisymmetric after exchange of any two particles. For multi- electron system, this result was first postulated by W. Pauli in 1924 even before the advent of quantum mechanics. The Pauli exclusion principle states that in a multi-elec- tron atom there can never be more than one electron in the same quantum state. This statement can be seen to be a consequence of saying that the electron wave function must be antisymmetric since electrons belong to the half-integer class of particles. )]2()1()2()1([ 2 1 )]2()1()2()1([ 2 1 αββαψαββαψ −=+= AS or 94
- 95. Towards the endof the The Hydrogen Atom chapter we saw that the wave function for an electron with spin could be written as follows: 2017 MRT so that for the Helium atom with two electrons we will have to take the appropriate combination of the two wave functions ψa(1)=ψa(1)ξξξξa and ψb(2)=ψb(2)ξξξξb, where ψa(1) represents the first electron at position 1 with a spin z-component represented by ξξξξa (i.e., either +½h or −½h) and similarly for ψb(2). The total wave function for the two electrons will have to be the appropriate combination of space and spin states that makes it antisymmetric. This total wave function can of course be written as the product of a space part times a spin part; if the space part is symmetric, the spin part must be antisymmetric and vice versa. As we have seen, the space part can be written: 95 smmn ξξ llψψψ == SpinSpace _ _ _ _ )]1()2()2()1([ 2 1 )]1()2()2()1([ 2 1 babaAbabaS ψψψψψψψψψψ −=+= or
- 96. The spin wavefunction will be a bit more complicated because the separate spin angular momenta can add vectorially just as the orbital and spin angular momenta do in the case of the two-electron atom. Instead of having J=L+S, here S=s1 ++++s2 so that S=0 or 1. The magnitude of S will of course be √[S(S+1)]h. 2017 MRT For S=0, ms =0 and we have just a single state while for S=1, ms =+1, 0, −1 and a triplet spin state results. This corresponds to the three possible orientations with respect to some preferred (i.e., z) direction in space and is equivalent to saying that we can start with the following four spin combinations: ξξξξa(½)ξξξξb(½), ξξξξa(−½)ξξξξb(−½), ξξξξa(½)ξξξξb(−½), and ξξξξa(−½)ξξξξb(½) to construct four symmetric and antisymmetric combinations: tripletsymmetric singletricantisymmet - )½()½( )]½()½()½()½([ 2 1 )½()½( 1 0 1 1 1 1 -)]½()½()½()½([ 2 1 00 −− −+− − + −−− ba baba ba baba smS ξξ ξξξξ ξξ ξξξξ 96
- 97. The total eigenfunctionmust be antisymmetric and thus we can form the following combination from ψS , ψA and the above four spin combinations: 2017 MRT and 97 ]-[]-[ tripletsymmetricandsingletricantisymmet ⊗⊗ AS ψψ or: )]½()½()½()½([)]1()2()2()1([ 2 1 babababa ξξξξ −−−⊗+ ψψψψ −− −+−⊗− )½()½( )]½()½()½()½([ 2 1 )½()½( )]1()2()2()1([ 2 1 ba baba ba baba ξξ ξξξξ ξξ ψψψψ Notice that if the spin part of the wave function is symmetric, corresponding to parallel spins, the space part must be antisymmetric. This has the interesting and important consequence that the electrons will have small probability of being found close together if they have parallel spins and a maximum probability of being found close together if they have antiparallel spins. One can say that in effect parallel spins repel and antiparallel spins attract.
- 98. We are nowready to turn to the discussion of the Helium atom again. Since this is a three-body problem, we can expect immediately that it cannot be solved explicitly in closed form as unfortunately no one has ever been able to solve in closed form any three-dimensional case involving more that two interacting particles. However, as we have already seen early on in this chapter, approximate solutions can be obtained for the energy of the ground state. We pointed out that the excited states of the He atom are degenerate and hence degenerate perturbation theory is necessary in order to calculate their energies and approximate wave functions. Fortunately, the simplified development of degenerate perturbation theory given early is adequate to treat, as an example, the first excited state of Helium. 2017 MRT For the degenerate first excited state of Helium it is useful to think of a resonance occurring in which either electron may spend some time in the higher energy level. The resonance, or exchange, energy that will be shown to result from this situation shifts the energy of the first excited p states from the energy expected if resonance is not taken into account. The energy shift due to resonance is observed in the shift of the frequency of the bright-line emitted radiation when the Helium atom is de-excited and collapses to its ground state. 98
- 99. The zero-order wavefunctions for the Helium atom, in which the interaction of the two electrons is ignored. are hydrogen-like wave functions. Because of the degeneracy between states ψnlml of different n, l, and ml there are eight states with the same energy possible to the unperturbed first excited energy level: if, say, electron number 1 is in the ground state ψ100 ≡|100〉 (i.e., called 1s) electron number 2 may be in any one of the excited states |200〉 (2s), |210〉 (2pz), |21+1〉 (2px), or |21−1〉 (2py), and conversely for electron number 2 in the ground state, the resulting four more states being physically indistinguishable from the first four. The total wave function would be written, for exam- ple, |100,200〉 if the first electron was in the |100〉 state and the second in the |200〉 state. 2017 MRT 99
- 100. The interaction energybetween the two electrons is treated by means of degenerate perturbation theory. In this case, this means that independent eigenfunctions (e.g., those with different l and ml) which have the same energy eigenvalue are said to belong to a degenerate energy level. We begin by considering only eigenfunctions belonging to the same energy level and assume that the zero-order eigenfunctions are all orthogonal. 2017 MRT Making the substitution of ψ =Σjajψ0 j above, V =V 0 +V ′, and Ei =E0 i +E′i into the Schrödinger wave equation ∇2ψ +(2me/h2)(E −V)ψ =0, we obtain: 0)()( 000000 =′−′=′−′+− ∑∑∑∑ j jj j jj j jj j jj aEVaEVaEaH ψψψψ 100 The wave function for the perturbed system in this degenerate energy level is not written as before (i.e., ψi =ψ 0 i +ψ ′i) but is expressed approximately in terms of the wave function of the same energy level: ∑= = n j jja 1 0 ψψ where ψ 0 j are the zero-order eigenfunctions for the particular energy level chosen and n is the order of the degeneracy. (N.B., We have dropped the subscript i referring to the particular energy level chosen, since all the zero-order eigenfunctions of interest have the same energy eigenvalue). since H0 Σjajψ 0 j =E0Σjajψ0 j where H0 =−(h2/2me)∇2 +V 0.
- 101. If we multiplythis last equation on the left with ψ 0 k * and integrate over all space, a set of simultaneous equations for aj can be obtained by letting k take all values from 1 to n: 2017 MRT where V ′jk =∫ψ 0 k *V ′ψ 0 j * dτ , or: 0)( 0 0)( 0)( 2211 2222211 1122111 =′−′++′+′ = =′++′−′+′ =′++′+′−′ EVaVaVa VaEVaVa VaVaEVa nnnnn nn nn K MMM K K 101 0=′−′∑ EaVa k j jkj Unless the determinant of the coefficients of the aj is zero, all the aj must be identically zero. Therefore: 0 )( )( )( 21 22221 11211 = ′−′′′ ′′−′′ ′′′−′ EVVV VEVV VVEV nnnn n n L MOMM L L where the degeneracy of the energy level is n-fold.
- 102. It frequently happensthat the perturbation potential taken between two states is zero unless the two states are the same. That is to say, V ′jm =δjmV jj . For example, this would occur if the perturbating potential were independent of angle, with the original wave functions being for a spherical symmetric potential. In this case the orthogonality of the spherical harmonics Yl ml would result in V ′jm =δjmV ′jj . Our last determinant then becomes diagonal and we will call this the secular determinant: 2017 MRT with the particularly simple form: 0)())(( 2211 =′−′′−′′−′ EVEVEV nnL 102 0 )(00 0)(0 00)( 22 11 = ′−′ ′−′ ′−′ EV EV EV nnL MOMM L L whose solutions are E ′=V ′11,V ′22,…,V ′nn for a level of a n-fold degeneracy. (N.B., With the V ′jj being given by where V ′jj =∫ψ 0 j *V ′ψ0 j * dτ , is essentially the same result as that obtained with nondegenerate perturbation theory – i.e., E′i =∫ψ 0 i *V ′ψ 0 i * dτ – with the difference that in degenerate perturbation theory the perturbation energy V ′ must be evaluated for not just one eigenfunction belonging to E 0, but between all eigenfunctions in the same energy level E 0).
- 103. The perturbation matrixelements for the energy of interaction between any two electron states are abbreviated by the letters J and K with subscripts s and p depending on whether both electrons are in an s state (i.e., with l=0) or one of them is in a p state (i.e., with l=1): 2017 MRT where (1) and (2) refer to each of the two electrons, dτ 1 is the differential volume element r 1 2sinθ 1dr 1dθ 1dϕ1 over the three degrees of freedom available to the first electron, and similarly for dτ 2. 〈100,200| and |100,200〉 are the products of two hydrogen-like wave functions derived in the The Hydrogen Atom chapter involving the Laguerre polynomials. Js is also written for 〈200,100|e2/r12|200,100〉 which has an identical numerical value. As for K, it is given by: ∫≡= 21 )2( 200 )1( 100 12 2 *)2( 200 *)1( 100 12 2 s 200,100200,100 ττψψψψ dd r e r e J 103 ∫≡= 21 )2( 100 )1( 200 12 2 *)2( 200 *)1( 100 12 2 s 100,200200,100 ττψψψψ dd r e r e K or 〈200,100|e2/r12|100,200〉 which has the same numerical value. Jp, and also Kp, is written for six different matrix elements all having the same numerical result, one of which is: 100,211211,100211,100211,100 12 2 p 12 2 p r e K r e J == and
- 104. The other Jpand Kp matrix elements differ from these only in having the two electrons interchanged or in the orientation of the total angular momentum in space (i.e., in having ml =0 or −1 instead of +1). The matrix elements other than these 16 elements are zero (e.g., consider the matrix element 〈100,200|e2/r12|100,211〉: the |211〉 wave function is an odd function; the other terms are all even, and thus, integrated over all space this matrix element must be zero). The J integrals are called Coulomb integrals since they give the potential energy contribution from the mutual repulsion of the two electrons. The K integrals are called the exchange integrals since they result from the exchange of the two electrons in the wave function on the left as compared with the wave function of the right in the matrix element. Another name for the K integrals is resonance integrals since this energy contribution arises from the resonance of the two electrons between states on the left and right of the matrix elements. 2017 MRT 104
- 105. By use ofthese matrix elements between the various hydrogen-like wave functions, the secular determinant is written as: 2017 MRT since all the Js and Ks with the same subscript are equal. 0 )(000000 )(000000 00)(0000 00)(0000 0000)(00 0000)(00 000000)( 000000)( pp pp pp pp pp pp ss ss = ∆− ∆− ∆− ∆− ∆− ∆− ∆− ∆− EJK KEJ EJK KEJ EJK KEJ EJK KEJ 105 where ∆E is the small energy shift due to the perturbation. This determinant can be rewritten as: 0])][()[( 32 p 2 p 2 s 2 s =−−∆−−∆ KJEKJE
- 106. The solutions ofthis last equation are: 2017 MRT with the last two terms still giving a triple degeneracy since the degeneracy in ml has not been broken up. Since the energy does not depend on magnetic quantum number ml, for convenience we will drop the ml from the bra and ket vectors for the rest of this chapter. ppppssss KJKJKJKJE −+−+=∆ and,, 106 The splitting up of the degeneracy between |10,20〉 and |10,21〉 (i.e., the 2s and 2p states – see Figure), corresponding to the difference between Js and Jp is due to the greater penetration of lower l electrons within the electron cloud. The further splitting of these states, however, results from the resonance energy expressed by the ground state sue to the mutual shielding of the positive nucleus by the electrons. The Figure illustrated the effect of these perturbation calculations. Illustration of the effect of the interaction energy of the two electrons in the lowest two unperturbed levels for the electrons in the Helium atom. The two lines on the left represent the ground state energy and first excited state energy if the perturbation caused by the electron repulsion is neglected. Both levels are raised by the electron shielding of the nuclear electrostatic potential, but the excited level is split into several levels as a result of differences in shielding between s (l = 0) and p (l = 1) electrons and quantum mechanical resonance. E Js Ks Kp |10,21〉 |10,20〉 |10,10〉 Ground Excited Jp
- 107. Thus, we havefound that the eightfold degenerate first excited level of the Helium atom splits into four levels. of which the lower two are entirely nondegenerate due to the addition or subtraction of the exchange energy. If we choose the new wave functions: 2017 MRT then Ks becomes: )100,200200,100( 2 1 )100,200200,100( 2 1 −=+= AS ψψ and 107 0100,200200,100200,100200,100 100,200200,100200,100200,100 2 1 12 2 12 2 12 2 12 2 12 2 * s = −+ −== ∫ r e r e r e r e d r e K AS τψψ Similarly, Kp vanishes when the wave functions above are used. Similarly, the nonzero J integrals are found to be symmetric and antisymmetric: 100,200200,100200,100200,100 12 2 12 2 s r e r e J S += 100,200200,100200,100200,100 12 2 12 2 s r e r e J A −= and
- 108. ψS is asymmetric wave function since it does not change sign if the two electrons are interchanged. ψA , however, does change sign and is this an antisymmetric wave function. ψS and ψA are seen to be the correct first-order wave functions since off- diagonal elements Ks, Kp vanish if these wave functions are used. 2017 MRT 108 Now for a few words on complex atoms. As the number of electrons in the atomic system becomes larger, a perturbation calculation such as we have discussed for the Helium atom rapidly becomes extremely difficult in practice to carry out. One way out of this difficulty was given by Hartree. In this approximation one assumes a system of the nucleus plus electrons wherein the Coulomb interactions between various electrons are accounted for by supposing that each electron moves independently in a central potential field Vi(ri). This field consists of the field of the charged nucleus and a spherically symmetric field associated with the average distribution of the remaining electrons. The Schrödinger equation for the total wave function of the electrons in the atomic system will then be of the form: TT Z i Tii Z i Ti EV m ψψψ =+∇− ∑∑ == 11 2 e 2 )( 2 r h
- 109. Since the electronsare assumed to be noninteracting, this equation splits into Z one- particle Schrödinger equations. Therefore, the solution for ψT is: 2017 MRT 109 )()()( 2211 ZZT rrr φφφψ K= where the φi(ri) are the solutions to the one-particle Schrödinger equations. The effects of the electron spin are ignored, except that the Pauli exclusion principle is invoked in the assignment of quantum numbers to the single-particle wave functions so that no two electrons in one atom can have the same values for all four quantum numbers n, l, ml, and ms. At this point, neither the Vi(ri) not the φi(ri) are known so that in general one might expect the problem to be insolvable. However, reasonably accurate solutions can be obtained in the following way. First, an educated guess for Vi(ri) is made and then the one-particle equations are solved. The resulting φi are used to calculate the total charge density arising from the electrons in the atom. The potential, as seen by the i-th electron, resulting from this charge density is calculated by means of Poisson's equation from electrostatics: iiV ρ−=′∇2 where ρi is the total charge density arising from the electrons (except the i-th one) which produce the average potential V ′i. When V ′i is added to the potential of the charged nucleus one has the average potential seen by the i-th electron. Ideally, the average potentials calculated for all the electrons should then agree with the Vi(ri) assume initially in setting up the Schrödinger equation.
- 110. In general, thesecalculated potentials will differ from the original assumed Vi(ri) and thus an improved choice for the Vi(ri) is made and new φi are calculated, from which another set of Vi(ri) can be calculated. The iteration process in continued until the Vi(ri) obtained from the final φi agree satisfactorily with Vi(ri) used to calculate the final φi; the Vi(ri) are then said to form a self-consistent field. 2017 MRT In the Hartree approximation one finds that the net potential any one electron sees is given by V(r)=−e2Z(r)/r where Z(r) is the effective charge seen by the electron. For r→0, Z(r)→Z and for r→∞, Z(r)→1. In between, Z(r) takes on intermediate values which can be calculated. 110
- 111. It should beemphasized that this has been a most elementary discussion and the actual calculation must include refinements such as spin-orbit interactions in order to get quantitative agreement with electron binding energies and other experimentally measurable quantities. Moreover, wave functions belonging to the same energy level are not orthogonal in the Hartree theory; this more precise refinement is embodied in the Hartree-Fock theory. 2017 MRT In general, a product wave function can be made orthogonal by forming a Slater determinant wave function. This is a superposition of product wave functions of the individual electrons which makes use of the fact that the interexchange of any two columns of a determinant (i.e., corresponding to the operation of exchanging two electrons) changes the sign of the determinant. With χ denoting the spin of the electron and ϕ the electron total space and spin state, the determinant is written as: ),(),(),( ),(),(),( ),(),(),( 2211 2222112 1221111 nnnnn nn nn χϕχϕχϕ χϕχϕχϕ χϕχϕχϕ ψ rrr rrr rrr L MOMM L L = 111
- 112. We are nowin a position to construct a table of the elements giving the quantum numbers appropriate to the various electrons and in particular to show ho the periodic atomic structure arises. 2017 MRT Consider the one-electron atom, hydrogen. We have already seen that in its lowest energy level, the ground state, the electron will be described by the following quantum numbers n=1, l=0, ml =0, and ms =±½. 112 If we choose ms =−½ for hydrogen, then the next electron added to give the ground state of Helium will have quantum numbers n=1, l=0, ml =0, and ms =+½ since the Pauli exclusion principle says that any given atomic state can be occupied by no more than one electron. When a third electron is added to give the ground state of lithium, it must necessarily go to the next energy level for which n=2. Then l can be 0 or ±1. From our previous discussion on the Helium atom we know that the shielding effect of the inner electrons will cause the l=0 state to lie lover than l=1. Therefore, we can say that the third electron added to make a lithium atom will be specified by n=2, l=0, ml =0, and ms = ±½.
- 113. Following this lineof reasoning, we can start to build up the periodic table of the elements as shown in the Table. The atomic shell structure is readily apparent if one examines the periodic table, and it is easy to see why families of elements should exhibit similar chemical properties and spectra. For instance, consider the alkali metals (i.e., Lithium, Sodium, Potassium, Rubidium, Cesium, and Francium). Each has one s electron outside a closed shell. Such an electron has a small binding energy and therefore all the alkali metals exhibit Hydrogen-like spectra. Another interesting family is that of the noble gases (i.e., Helium, Neon, Argon, Krypton, Xenon, and Radon). Each of these elements has a filled outer shell of electrons and are all chemically inactive. In general, it is the number and configuration of the outermost electrons which determine the chemical properties of the elements. 2017 MRT 113 n l ml ms Element Name Configurations 1 0 0 −½ H Hydrogen 1s 1 0 0 +½ He Helium 1s2 2 0 0 −½ Li Lithium 1s22s 2 0 0 +½ Be Beryllium 1s22s22p 2 1 −1 −½ B Boron 1s22s22p2 2 1 −1 +½ C Carbon 1s22s22p3 2 1 0 −½ N Nitrogen 1s22s22p4 2 1 0 +½ O Oxygen 1s22s22p5 2 1 1 −½ F Fluorine 1s22s22p6 2 1 1 +½ Ne Neon 1s22s22p6 3 0 0 −½ Na Sodium 1s22s22p63s 4 0 0 +½ Mg Magnesium 1s22s22p63s2
- 114. The electron shellsfill in sequence up through Argon which has the configuration 1s22s22p63s23p6. At this point we find a deviation from the expected ordering. Because of the various electron-electron interactions and the more penetrating orbit of l=0 electrons, the 4s electrons are more tightly bound than the 3d electrons. Consequently, after Argon one 4s electron is added in Potassium and a second in Calcium. Following this the 3d shell is filled, starting with Scandium, which has the configuration 1s22s22p63s23p64s23d. Iron, Cobalt, and Nickel belong to this transition group and their magnetic properties are due to the presence of the partially filled d shell in each case. As more and more electrons are added, the shells are formed to fill up on average in the following order: 2017 MRT The 14 elements in which the 4f electrons are added one by one to fill the 4f shell are known as the rare earths. Since the orbits of the 4f electrons lie far inside those of the outer electrons and since the configurations of the outer electrons are similar for all the rare earths, the chemical properties of the rare earths are very nearly the same. Consequently, it is difficult to separate these elements by chemical means. A similar situation exists higher up in the periodic table when the 5f shell is being filled in elements such as Protactinium and Uranium. 114 The periodic table terminates at Z~100 because these nuclei are too unstable due to fission of radioactive decay. 101426101426102610262622 6d5f7s6p5d4f6s5p4d5s4p3d4s3p3s2p2s1s
- 115. And below isthe Periodic Table of the Elements (D. Mendeleev, 1869). 115
- 116. This is amusingto show since these are the element’s applications as seen on Earth. 116
- 117. Now on withmolecules. Since molecules consist of atoms held together by relatively low binding energies (i.e., a few eV) we may well expect that a knowledge of atomic electron systems can help in understanding molecular systems. In this discussion, we shall deal mainly with the simplest of molecules, namely those containing only two atoms, diatomic molecules. The molecular binding holding the atoms together can be either of two types, ionic binding (i.e., heteropolar) or covalent binding (i.e., homoplanar). 2017 MRT As an illustration of ionic binding let us consider the NaCl molecule (e.g., rock salt). For sodium Z=11 and the electron configuration for the sodium atom is 1s22s22p63s. Furthermore, the binding energy of the 3s electron is −5.1 eV. For chlorine with Z=17 the electron configuration is 1s22s22p63s23p5. The chlorine atom lacks one electron for filling the 3p subshell. Since the filled p subshell is a particularly stable configuration, the lack of the electron acts as though these was a hole present with an effective net binding energy of +3.8 eV. If the 3s electron is transferred from the sodium atom to the 3p shell of the chlorine atom, it would take 5.1 eV−3.8 eV=1.3 eV of energy, or, in other words, the system of Na+ and Cl− would have a net gain of 1.3 eV. However, there will also be a Coulomb attraction between the two ions. The Coulomb potential energy is −e2/ro so that the net binding energy for the system will be BE=+1.3 eV−e2/ro. Experimentally, one find a binding energy for NaCl or −4.24 eV. Thus ro =2.36 Å, a value which is in good agreement with results of independent measurements using X rays. 117
- 118. The NaCl molecule(Figure - Left) cannot collapse because when the atoms get too close the wave functions overlap. At this point the electron densities get distorted, partially as a consequence of the Pauli exclusion principle, and there is a net repulsion between the electron clouds and between the positively charged nuclei. The ro is the value for which the total potential energy of the system is a minimum. This is shown schematically in the Figure - Right. 2017 MRT From the foregoing picture, we might expect the molecule to vibrate about the equilibri- um distance ro if one of the atoms should be hit in some way. These vibrations do occur and give rise to characteristic vibrational spectra. Also, because NaCl molecule consists of a positively charged Na ion (Na++++) together with a negatively charged Cl ion (Cl−−−−), we also expect, and find, that NaCl has a permanent electric dipole moment. A permanent electric dipole moment is characteristic of all diatomic molecules with ionic binding. 118 Left: Sodium Chloride (Na++++Cl−−−−) crystal; Right: Illustrating the potential energy for ionic binding of the NaCl molecule as a function of the separation distance between the ions. The value of 2.36 Å for ro at the minimum of the curve is found from the experimentally observed binding energy of 4.24 eV. r V (r) 0 ro BE = 4.24 eV 2.36 Å
- 119. In ionic bindingwe have just seen that the individual atoms either gain or lose electrons and become effectively ions. In covalent binding the electrons are shared between the atoms and one should not think in terms of gaining or losing electrons. Again, we will choose a particularly simple system to illustrate; in this case it is H2 ++++ (Z=1) two protons sharing a single electron. 2017 MRT For this system we can write down the Hamiltonian: r e r e r e m p m P m P H 2 2 2 1 2 e 2 p 2 2 p 2 1 222 +−−++= 119 where mp is the mass of a proton and me the electron mass; P1 and P2 are the momenta of protons 1 and 2 respectively, p is the electron momentum; r is the separation between protons 1 and 2 and r1 and r2 are the separations between proton 1 and the electron and proton 2 and the electron respectively. By using the Hamiltonian we could write the Schrödinger equation, although it could not be solved in closed form since this is a three-body problem. Therefore, it is helpful to use the adiabatic approximation in which the motion of the protons is neglected. This is a reasonable procedure because the protons are so much more massive than the electron that their relative motion will necessarily be much smaller than that of the electron. In the adiabatic approximation we ignore the P1 2/2mp, P2 2/2mp and e2/r and the Hamiltonian becomes: 2 2 1 2 e 2 2 r e r e m p H −−=
- 120. x y x y r ψ0 S ψ0 A ψ0 S ψ0 A r When r islarge the electron can be thought of as belonging to one proton or the other, but as r becomes small this distinction is not possible and we must consider the effects of exchange degeneracy. In this case, we ought then to take the total wave function as being either symmetric or antisymmetric. For convenience we take both protons to be lying on the x-axis so that under an exchange it is only the x coordinate that varies. Then we write: 2017 MRT Further, for the ground state U100 we choose the x-axis so that we have U100(x,0,0). Both ψ0 S and ψ0 A are shown in the Figure for different values of r. Looking at these plots, we see that as r→0 (at x=0), ψ0 S →maximum and ψ0 A →0. )],,(),,([ 2 1 )],,(),,([ 2 1 2121 zyxUzyxUzyxUzyxU nn A nnn S n −=+= ψψ or 120 Illustrating the form of the symmetric and antisymmetric wave functions of the H2 ++++ molecule for two different proton separation distances (Left vs Right) .
- 121. As the protonscome reasonably close together the electron has the greatest probability for being found between them. It acts to give a net attraction until the Coulomb repulsion between the protons overrides the attraction to give a net repulsion. Therefore, we again have a situation where there will be a minimum in the potential energy for a certain equilibrium separation of the protons as shown in the Figure. For the H2 ++++ molecule ro =2.65 Å and BE=−2.65 eV. Since the binding energy is negative the H2 ++++ system is stable; it is interesting to note that H2 ++++ molecules can readily be produced in the laboratory. 2017 MRT 121 Illustrating the potential energy for covalent binding of the H2 ++++ molecule as a function of the separation distance between the protons. The binding energy and separation at the minimum point have been obtained from experiment. r V (r) 0 ro BE = 2.65 eV 2.65 Å
- 122. The preceding discussionon the H2 ++++ molecule has been made quite qualitative. Taking the H2 molecule as an example we will show how a much more quantitative treatment can be carried out. In the diatomic Hydrogen molecule the two electrons can alternate in orbiting around one proton or the other; thus the Hydrogen molecule ground state furnishes us with another simple example of resonance. In general, the Hydrogen- Hydrogen bond is composed of two types of states, one in which both electrons surround one proton forming an ionic bond and the other type corresponding to a perturbation of the wave functions for infinitely separated Hydrogen atoms, is the one we will assume to predominate (i.e., covalent binding). 2017 MRT 122
- 123. Both electron cloudswill share an electron – i.e., one electron with spin up (Sz =+½h) and the other electron with spin down (Sz =−½h) – whereas both nuclei repel each other. In the bond 1s1-1s1, two scenarios occur causing coupling betweennucleusandorbitals: e− e− Electron cloud (1s1) Nucleus (proton) Electron (in 1s) p+ p+ e− e− p+p+ e−e− Attractive Repulsive Sz = −½h Sz = +½h e− w/ +½ e− w/ −½ Both e− w/ +½ Both e− w/ −½ 1HA 1HB Spin Up e−−−− Spin Down e−−−− 2017 MRT p+p+ Proton p1 point charge Q = +e1 attracts electron e2 pointcharge q = −e2 Proton p1 point charge Q = +e1 repels proton p2 point charge q = +e2p1 p2 e1 e2 123
- 124. Unperturbed State Perturbed GroundState Unperturbed State Perturbed Excited State Ground Energy ψ E0 V E0 + E′ E0 ψ V E0 ψ V Correct Energy E0 ψ V The Figure illustrates the change in electron potential and consequent change in wave functions as the two atoms are brought close together. A continuous wave function and derivative cannot be realized by joining the two infinite-separation wave functions when the two atoms are joined. Only by lowering the energy eigenvalue of the system (or raising it to a new first excited level) can a smooth fit for the total wave function be made. 2017 MRT 124 Illustration of the behavior of the potential energy, the energy levels, and the complete two-electron wave functions for two Hydrogen atoms (Top Left) infinitely separated, (Top Right) brought together with the separated wave functions drawn (unchanged from Top Left), (Bottom Left) brought together and showing the corrected, smoothly joining wave functions, (Bottom Right) brought together and showing the correct wave function for the first excited state. The dashed lines in Bottom Left and Bottom Right are for the correct energy levels appropriate to the wave functions shown in Bottom Left and Bottom Right. The straight solid horizontal lines on all four plots show the ground energy level of the infinitely separated atoms, for comparison.
- 125. = = ∂ ∂ ∂ ∂ =∇ ∑ g g g gg x gg xg ijij ij ji j ij i cof det 12 and where Thetotalenergy (i.e.,thesum of kinetic and potential energies – using an‘observable’ such as the Hamiltonian operator) for a Hydrogen molecule (H2) is given by the sum: r1B r2A r2B r1A r12 −e1 −e2 mp is the mass of a Hydrogen nucleus while me is the electronic mass. The Laplacian operator is: with respect to the positions of the 1H nuclei A and B and the positions of the electrons 1 and 2. 2 2 222 2 2 2 3 1 3 1 2 2 2 sin 1 sin sin 11 sin sin 1 ϕθθ θ θθ θ θ ∂ ∂ + ∂ ∂ ∂ ∂ + ∂ ∂ ∂ ∂ =∇ ∂ ∂ ∂ ∂ =∇ ∑∑= = rrr r rr x gr xr i j j ij i ABo 2 21o 2 A2o 2 B1o 2 B2o 2 A1o 2 2 2 2 1 e 2 2 B 2 A p 2 πε4πε4πε4πε4πε4πε4 )( 2 )( 2 r e r e r e r e r e r e mm H ++−−−−∇+∇−∇+∇−= hh A Hydrogen molecule – with two bound electrons (1 and 2) a distance r12 apart to two protons (1H nuclei A and B) separated by a inter-proton distance rAB. The electrons and protons are each separated from one another by the radii r1A, r1B and r2A, r2B. 1HA 1HB 2017 MRT +e1 +e2 One of the great early achievements of quantum mechanics was the description of the chemical bond by Heitler and London in 1927. Prior to this time, it was impossible to explain why two Hydrogen atoms came together to form a stable chemical bond. Using spherical coordinates, x1 =r, x2 =θ, and x3 =ϕ, we have for the Laplacian operator in spherical coordinates (the square of the gradient or ∇2 =∇∇∇∇•∇∇∇∇): 125 rAB
- 126. To begin with,we consider the two atoms well separated as compared to the distance of the electrons from the closest protons. The initial wave function will then consist of the product of the ordinary ground state Hydrogen wave functions for each atom, ψA(r1)ψB(r2), and will yield resonance or exchange energy. Let r1A represent the separation of electron 1 from proton A, and similarly r2B for electron 2 and proton B. The complete wave equation is then: 2017 MRT The unperturbed function is ψ =ψA(r1)ψB(r2) where ψA(r1) is a solution to: ψψ E r e r e r e r e r e r e m = ++−−−−∇+∇− AB 2 21 2 A2 2 B1 2 B2 2 A1 2 2 2 2 1 e 2 )( 2 h 126 )()( 2 1A11A A1 2 2 1 e 2 rr ψψ E r e m = −∇− h ψA(r1) is the associated Laguerre and spherical harmonic function of the The Hydrogen Atom chapter, and similarly for ψB(r2) (i.e., with ∇2, e2/r2B, and E2 instead). Let: AB 2 21 2 A2 2 B1 2 r e r e r e r e H ++−−=′
- 127. Then the degenerateperturbation integrals are: 2017 MRT and ∫∫ ′= 212B1A2 * B1 * A )()()()( ττψψψψ ddHJ rrrr 127 where dτ1 and dτ2 are the differential spherical volume elements (e.g., dτ1 = r1 2sinθ1dr1dθ1dϕ1). The second term in the exchange integral K with H0, which we will label K0, must be included because our initial wave functions are not orthonormal. Since: ∫∫∫∫ +′= 211B2A 0 2 * B1 * A211B2A2 * B1 * A )()()()()()()()( ττψψψψττψψψψ ddHddHK rrrrrrrr )()(2)()()()( 1B2A11B2A 0 1B2A 0 rrrrrr ψψψψψψ EEH == we have: ∫∫= 211B2A2 * B1 * A10 )()()()(2 ττψψψψ ddEK rrrr The contribution of e2/rAB term to J is simply e2/rAB, since the wave functions are normalized and e2/rAB may be taken outside the integration over the electron coordinates. Similarly, the contribution of the e2/rAB term to K is simply [(e2/rAB)/2E1]K0. The contribution of e2/r1B to J would be ∫ψA *(r1)(−e2/r1B)ψA(r1)dτ1 since ψB(r2) is normalized and r1B is not a function of the position of the second electron. The contribution of e2/r1B to K would be similar.
- 128. KJKJE −+=∆ , E+ J + K + ∆ E + J + ∆ E + J − K + ∆ Symmetric Antisymmetric No Exchange E rAB BE We thus obtain the secular determinant: 2017 MRT from which the shifts in energy level caused by the resonance energy are found to be: 0 )( )( = ∆− ∆− EJK KEJ 128 The Coulomb effects between all the charges roughly cancel out, leaving the exchange effects which cause chemical bonding, as illustrated in the Figure. Illustration of effects of exchange or resonance energy on the binding energy BE of the Hydrogen molecule. The middle curve includes all effects J+∆ other than the resonance of the two electrons where ∆ represents all interactions not specifically calculated in the text.
- 129. It is foundthat the symmetric wave function: 2017 MRT is the correct zero-order wave function which with its antisymmetric twin causes the perturbation matrix to be diagonal. The wave function above is also the symmetric wave function whose eigenvalue is approximately E0 −K, the lower of the two energy eigenvalues including resonances. This is in contrast to the case of Helium where both electrons are in the same atom, and the antisymmetric wave function was found to be the lower of the two energy eigenvalues including resonances. The attractive bonding force of the Hydrogen molecule has been shown to be due to the possibility of two electrons being in a symmetric state (i.e., being interchangeable in the space wave function representation). Since no more than two electrons can be in one symmetric state at the same time, the degeneracy in this case can be only twofold. )]()()()([ 2 1 1B2A2B1A 1 0 rrrr ψψψψψ + + = E K S 129
- 130. The symmetric wavefunction ψS above yields a probability of finding both electrons between the two protons larger than the probability of this event for the two atoms placed a distance rAB apart and no resonance effects taken into account(i.e.,|ψA(r1)ψB(r2)|2 gives a probability of this event smaller than that given by the absolute square of the symmetric wave function ψS). The antisymmetric wave function, on the other hand, gives smaller electron probability density in this region, compared to the nonresonance value. Thus, despite the mutual repulsion of the two electrons, the attraction of the two protons for the (symmetric state) negative cloud causes the Hydrogen molecule to be a stable configuration. The mutual repulsion of the less screened protons in the antisymmetric electronic state results in an unstable configuration. ∆E, J, and K are functions of internuclear distance as shown in the previous Figure. 2017 MRT From the discussion it is evident why covalent binding does not give rise to a permanent electric dipole moment as was the case for ionic binding. Further, it is also reasonable to expect that negative ions such as H2 −−−− would be producible in the laboratory. 130 Now, we have seen that the attractive potential energy curves for both ionic and covalent binding have a minimum corresponding to the equilibrium distance of separation of the atoms and we have already speculated that, if somehow the system were displaced from this equilibrium position, it might vibrate with simple harmonic motion, much like two balls held together by a spring. We might also expect that the simple harmonic diatomic molecule would appear like a dumbbell, so that it could rotate about its center of mass.
- 131. For diatomic molecule,rotational and vibrational motions cannot be completely separated as there will be interactions between the rotational and vibrational motion. This can be seen by considering the two atoms as being held together by a harmonic or spring force. Then, as the molecule rotates about an axis perpendicular to the line between the atoms, the atoms will tend to move farther apart, thereby giving a different effective vibrational potential for different rotational states. However, for low rotational states, the effect is small and reasonable agreement with experimental results can be obtained by separating the two motions. 2017 MRT 131 To be more rigorous, one could start with the Hamiltonian to be used in the Schrödinger equation for two atoms of mass M1 and M2 separated by a distance r: )( 22 2 2 2 1 2 1 rV M P M P H n++= where Vn(r) is the interaction potential for any given electronic state of the system. Now, we can simplify things by considering that so long as Vn(r) is only a function of r, one can go to center-of-mass coordinates and separate out the angular dependence. Therefore, both the total orbital angular momentum of the system and its z-axis component will be quantized and only the solution for the radial part of the motion will differ for the solution for the one-electron atom. A detailed solution of the radial equation is beyond the scope of this chapter, but it is instructive to consider how the problem can be treated. We will consider that presently.
- 132. If the angularmotion is considered as being due to the nuclear motion (i.e., ignoring angular momentum contributions from the electrons) then the radial wave equation becomes of the form: 2017 MRT where µ is the reduced mass (i.e., µ=M1M2/(M1 +M2)) and J is the rotational quantum number. If we let χ(r)=rR(r) then: 0 2 )1( )( 21 2 2 2 2 2 = + −−+ R r JJ rVE rd Rd r rd d r ns µ µ h h 132 0 2 )1( )( 2 2 2 2 22 = − + −+− χ µ χ µ sn E r JJ rV rd d hh But this is the same equation one gets for a one-dimensional system consisting of a particle moving on a line under the influence of a potential: 2 2 2 )1( )()( r JJ rVrU nn µ + += h
- 133. Consider the formof Vn(r), it is readily seen that Un(r) should have the form shown in the Figure. For a given value of J, and near its minimum point, Un(r) is approximately the same as the harmonic oscillator potential. As J increases, the minimum of the potential Un(r) becomes less pronounced and finally disappears. Physically we see that the molecule stretches as it rotates, and at high enough rotational speeds the stretching becomes so great the atoms will fly apart. 2017 MRT 133 Illustrating the effective potential for the diatomic molecule showing the dependency of the rotational quantum number J as well as the distance r between two atoms of mass M1 and M2 . r Un (r) 0 J = 25 J = 30 J = 20 J = 15 J = 0 J = 10
- 134. The radial equationcannot be solved exactly, but good results can be obtained using approximation methods. When this is done, it is found that the major feature of the nuclear motions is the harmonic vibration with the rotational effects appearing as a fine structure upon each vibrational energy level. The energy levels of the system are found to be given by: 2017 MRT Here Vn represents the energy of the electronic state and rJ is the atomic separation for the J-th rotational state. Here we have considered the harmonic oscillator approximation in which the two atoms are pictured as being held together by a spring with spring constant k, so that the potential energy is V =½kr2. The energy levels are given by: 2 2 2 )1( 2 1 ω J nnvkn r JJ vVE µ + + ++= h h 134 += 2 1 ω vEv h with v =0,1,2,3,… and where: µ k =ω
- 135. Transitions between theseelectronic states usually occur in the visible region of the spectrum. The second term corresponds to vibrational (i.e., harmonic oscillator) energy levels and gives rise to transitions in the near infrared region, while the last term (i.e., rotational states) produces spectral lines in the far infrared. Since these energy regions are far removed from one another, they can be treated separately as illustrated in the Figure. 2017 MRT 135 Energy-level diagram for a typical diatomic molecule, showing electronic, vibrational, and rotational levels. r E 0 r Excited electronic state Ground electronic state Rotational levels Vibrational levels
- 136. Now, when nuclearspins are included, the total wave function for a molecule can be written (to a good approximation) as: 2017 MRT where the product of wave functions consists of the description for electronic states, vibrational and rotational motions, and the state of the nuclear spin. SpinNuclearRotationallVibrationaElectronicTotal ψψψψψ = 136 Nuclear spins will generally have small effect on the molecular properties except for special cases when the molecules are composed of identical nuclei. In this situation the spins can indirectly exert a very great influence. To see how this comes about let us consider the case of ortho- and para-hydrogen, first explained by Dennison. Since each proton has a spin of ½ (in units of h) the H2 molecule can be formed with the proton spins parallel (i.e., ortho-hydrogen) and antiparallel (i.e., para-hydrogen). In cases such as this involving identical statistics (i.e., the total wave function is antisymmetric and the Pauli exclusion principle follows) if the nuclear spins are half-integer and Bose-Einstein statistics (i.e., the total wave function is symmetric) if the nuclear spins are integers. Consequently, for the H2 molecule the total wave function must be antisymmetric.
- 137. At reasonably lowtemperatures where there is little excitation energy available, we expect to find the H2 molecule in the electronic ground state (symmetric) and also in the vibrational ground state (symmetric). Therefore, the symmetry of ψTotal will depend on the relative symmetries of the nuclear spin and the rotational states. Now, recalling earlier discussion in this chapter, if one has two spin-½ particles, three symmetric and one antisymmetric spin functions can be formed. Also, we note that the angular momentum eigenfunctions and rotational states with odd J are antisymmetric. It follows that H2 molecules in the ground electronic and vibrational states will have symmetric spin states matched with antisymmetric rotational states and antisymmetric spin states with symmetric rotational states. At room temperatures where many rotational states of the molecules are formed one expects and find a statistical distribution of ¾ ortho- hydrogen molecules (i.e., three symmetric spin functions) and ¼ para-hydrogen molecules (i.e., one antisymmetric spin function). 2017 MRT 137 However, at very low temperatures only the J=0 rotational band will be occupied. In this case the spin function must be antisymmetric and we should expect to find just para- hydrogen present. In practice, to achieve this result one must overcome the obstacle that the sins of the nuclei interact only very slightly with each other; it is very difficult to change the nuclear spin state from triplet to singlet (e.g., just as in the case of transitions between atomic energy levels where transitions between different electrons spin states are forbidden).
- 138. At low temperatures,the vast majority of the molecules will be in the lowest electronic and vibrational states and transitions will generally take place between various rotational levels. At higher temperatures where excitations to higher vibrational states is frequent, the rotational states are also observed as fine structure in the observed vibrational spectrum. For example, if we consider a gas of diatomic molecules in thermal equilibrium, then the relative populations of the vibrational and rotational levels will be governed by the Maxwell-Boltzmann distribution law. The relative number of molecules in a particular vibrational energy state Ev =(v +½)hω will be: 2017 MRT TkvTk v Tkv Tkv v TkE TkE v BB B B Bv Bv N N ωω 0 ω½)( ω½)( 0 Total e)e1( e e e e hh h h −− ∞ = +− +− ∞ = − − −=== ∑∑ 138 Under ordinary conditions the ratio Ev /kBT is large for most diatomic molecules so that the great majority of the molecules will be found in the lowest vibrational energy state with v =0. Therefore, at room temperatures and below, particularly for light molecules, it is reasonable to assume the molecules are in the ground vibrational state. For rotational levels, the situation is quite different because the energy differences between low-lying rotational levels are much smaller than those between low-lying vibrational levels. Also, in considering the populations of the rotational levels, we must take into account the fact that each level specified by a given E is 2J +1 degenerate. Therefore, a statistical weight factor of 2J +1 must be included in specifying the relative number of molecules occupying the J-th energy level.
- 139. Finally, as wehave seen earlier in this chapter, a homonuclear diatomic molecule cannot have a permanent electric dipole moment. However, the presence of an external electric field can cause an induced dipole moment which will be proportional to the electric field. Under these conditions the varying electric field of an incident light wave should produce an induced dipole moment changing at the same frequency. Therefore, when incident light quanta of frequency νo are incident on the system we might expect to see scattered photons also of frequency νo (i.e., Raleigh scattering). In practice if the scattered photons are examined closely, photons of energy hνo ±∆E can sometimes be seen as well as those of energy hνo. Since the molecule represents a quantum mechanical system the ∆Es must correspond to energy differences between various states of the system. Scattering for which the incident photon of energy hνo goes to hνo ±∆E is called Raman scattering. When the induced transitions are between vibrational levels they give rise to vibrational Raman spectra as distinguished from induced transitions between rotational levels, which produce rotational Raman spectra. 2017 MRT 139
- 140. Appendix – Interactions Contents TheHarmonic Oscillator Electromagnetic Interactions Quantization of the Radiation Field Transition Probabilities Einstein Coefficients Planck’s Law A Note on Line Broadening Photoelectric Effect Higher Order Electromagnetic Interactions 2017 MRT “A philosopher once said: ‘It is necessary for the very existence of science that the same conditions always produce the same results’. Well, they don’t!” R.P. Feynman, The Character of Physical Law, P. 141. 140
- 141. The harmonic oscillatoris of interest in many different fields of physics since it can be often be used as a good approximation for physical systems. At this time, we shall consider the one-dimensional (or linear) harmonic oscillator both because of its interest and because the techniques used in the solution will be useful when evaluating the Hydrogen molecule where both atoms can be assumed to be held together by a spring. The Harmonic Oscillator The harmonic oscillator potential in one-dimension generalized coordinate q may be written as: 2017 MRT where k is a spring constant (N.B., with k=mω2 for an angular frequency ω of oscillation). where p is the momentum and q the displacement (both canonical variables) with: and the Schrödinger equation corresponding to the Hamiltonian H=p2/2m +½mω2q2 is: 22 2 ω 22 q m m p H += q ip ∂ ∂ −= h 0)(ω 2 12)( 22 22 2 = −+ qqmE m qd qd ψ ψ h 2 2 1 )( qkqV = Foraone-dimensionalharmonicoscillatorofmass m andfrequency ω theHamiltonian is: 141
- 142. The eigenfunctions ofthe harmonic oscillator version of the Schrödinger equation: in which Hn(x) is a Hermit polynomial* of degree n and the eigenvalues are: 2017 MRT A more convenient form to the ψn(q) solution above is achieved by changing the variables to: We then have: which has the properties: nn m uq m q ψξ ω ω )( h h == and )()1()( 22 1 1 2 1 2 1 11 11 ξξξ ξ ξ ξ ξδξ n n nnnn nnnnnmnm uuu n u n u unu d d unu d d duu −=−+ + = += −= += −+ +− ∞+ ∞−∫ and ,,, = − q m H m n q n q m nn hh h ω e π ω !2 1 )( 2 2 ω4/1 2/ ψ ( ),...,,nnEn 210ω)½()ω( =+= h * The Hermit polynomials are solutions to the differential equation d2Hn/dx2 – 2xdHn/dx − 2nHn = 0 and have a solution of the form Hn(x) = (−1)n exp(x2)dn[exp(−x2)]/dxn. The first four Hermit polynomials are H0(x) = 1, H1(x) = 2x, H2(x) = −2 + 4x2 and H3(x)= −12x + 8x3. )(e )!2π( 1 )( 2 2/12/ 2 ξξ ξ nnn H n u − = 142
- 143. We shall nowinvestigate the harmonic oscillator be means of a matrix formulation. For convenience, let: so that the Hamiltonian H=p2/2m+½mω2q2 becomes 2017 MRT The transition to quantum mechanics is made by reinterpreting P and Q as Hermitian operators which obey the commutation relation: which is equivalent to the replacement of P by −ih∂/∂Q. It is important to note that the quantum mechanical operators Q and P are independent of time. We now construct the linear combinations: hiQPQPPQ =≡− ],[ )ω( ω2 1 )ω( ω2 1 † PiQaPiQa −=+= hh and 22 2 2 )()( qmqQ m p pP == and )ω( 2 1 222 QPH += or: )( 2 ω )( ω2 †† aaiPaaQ −=+= hh and 143
- 144. In terms ofa and a†, the commutator [Q,P]=ih becomes: and the Hamiltonian H=½(P2 + ω2Q2) takes the form: where: 2017 MRT is called the number operator and it is Hermitian. Also from [a,a†]=1, we have: )1()1()1( ††† −=−=−== NaaaaaaaaaaNa 1],[ † =aa += +=+= 2 1 ω 2 1 ω)(ω 2 1 ††† NaaaaaaH hhh aaN † = 144 )1()1( †††††† +=+== NaaaaaaaNa and:
- 145. Now, let |n〉be an eigenstate of N with eigenvalues n, i.e., let: and from the equations Na=a(N−1) and Na† =a†(N+1), we get: Thus we have the result that if |n〉 is an eigenstate of N with eigenvalue n, then a|n〉 is also an eigenstate of N with eigenvalue n−1. Similarly, a†|n〉 is also an eigenstate of N with eigenvalue n+1. Since N is Hermitian the eigenvalue n is real. The Hermitian property of N also require that: nnnN = nannanannanaNnNanNa )1()1( −=−=−=−= nannannananNanNanNa ††††††† )1()1( +=+=+=+= Eigenvalues end eigenstates of the number operator N superimposed on the potential V. 2017 MRT The eigenvalues of N and the corresponding eigenstates may be displayed in the form of a ladder (see Figure), and in view of the equation H= hω(N +½), the energy levels of the harmonic oscillator must have a constant spacing with an energy hω between adjacent levels. 0† ≥== anannaannNn However, the ladder has a lower bound because: n − 2 n − 1 n n + 1 n + 2 • • • • • • Eigenvalues of N (a†)2| n 〉 a†| n 〉 | n 〉 a| n 〉 a2| n 〉 and 〈n|N|n〉 =n so that: 0≥== nanannNn V&En q 145
- 146. From N|n〉=n|n〉 andNa|n〉=(n−1)a|n〉, we have: Since there are no degeneracies: where cn is a constant of proportionality. Since: 2017 MRT and from the relation 〈n|N|n〉=〈an|an〉=n, we have cn =+√n where the positive square root is in accord with (c.f., Weissbluth’s) convention. Thus: Because of these two relations for a|n〉=√n|n−1〉 and a†|n〉=√(n+1)|n+1〉, the operators a and a† have been given the names of annihilation and creation operators, respectively. 1)1(11)1(1 −−=−−−=− nannNannnN and 1−= ncna n 22 11 nn cnncanan =−−= 1−= nnna In the same way, we find: 11† ++= nnna Is n an integer? Assume that it is not; then the relation a|n〉=√n|n−1〉 will ultimately lead to a negative value of n which would then violate the relation 〈n|N|n〉=〈an|an〉=n. On the other hand, when n is an integer, the relation a|n〉=√n|n−1〉 leads to a|0〉=0 which is the lower limit of the sequence and is consistent with a|n〉=√n|n−1〉. Annihilation operator Creation operator 146
- 147. It is nowpossible to construct matrices to represent the various operators: 2017 MRT =⇒+=+ =⇒=− OMMM L L L L OMMMM L L L 300 020 001 000 11 3000 0200 0010 1 † annanannan & =⇒+=+= ==⇒== OMMM L L L OMMMM L L L L 300 020 001 1 3000 0200 0010 0000 ††† †† aannnnaannaan aaNnnNnnaan & and: 〈row|a|col〉 |col〉 〈row| 147
- 148. 2017 MRT The eigenfunctions |n〉may also be given a matrix representation as column vectors: = = = = M M L MMM 1 0 1 0 0 2 0 1 0 1 0 0 1 0 n,, Q and P are related to a and a† by the transformations Q=√(h/2ω)(a−a†) (with Q2 =mq2) and P=i√(hω/2)(a† −a) (with P2 =p2/m). Hence: − − − − = = OMMMMM L L L L h OMMMMM L L L L h 40300 03020 00201 00010 2 ω 40300 03020 00201 00010 ω2 iPQ & Finally, the Hamiltonian H=hω(N+½) is: = + + + =+= OMMM L L L h OMMM L L L hh 2 5 2 3 2 1 2 1 2 1 2 1 2 1 00 00 00 ω 200 010 000 ω)(ω NH 148
- 149. The quantum harmonicoscillator wave functions ψn(q) are given by: 2017 MRT 0 1 2 3 4 • • • n Eo = (1/2)hω • • • E1 = (3/2)hω • • • E2 = (5/2)hω • • • E3 = (7/2)hω ψ0 = e−ξ 2 /2 ||||ψψψψ0||||2 =e−−−−ξξξξ 2 ψ1 = 2ξ e−ξ 2 /2 ||||ψψψψ1||||2 =4ξξξξ 2e−−−−ξξξξ 2 ψ2 = (4ξ 2 – 2)e−ξ 2 /2 ||||ψψψψ2||||2 =(4ξξξξ2 – 2)2e−−−−ξξξξ 2 ψ3 = (8ξ3 – 12ξ)e−ξ 2 /2 ||||ψψψψ3||||2 =(8ξξξξ3 – 12ξξξξ )2e−−−−ξξξξ 2 2 2 2 2 2 ω 3 2/34/1 2/33 2 ω 2 4/1 2 2 ω4/1 1 2 ω4/1 o e ω 8 ω 12 π ω 62 1 )( e ω 42 π ω 22 1 )( e ω 2 π ω 2 1 )( e π ω )( q m q m q m q m q m q mm q q mm q q mm q m q h h h h hhh hh hh h − − − − +− = +− = = = ψ ψ ψ ψ In diagrammatic fashion the eigenstates (unnormalized ψn(ξ) – and associated probabi- lity densities ||||ψψψψn||||2 – with the dummy variable ξ =√(mω/2h)q) and energy eigenvalues (En ) of a single harmonic oscillator may be represented as shown in the Figure below. 149 = q m h2 ω ξ
- 150. Henceforth, our ultimateobjective is to derive Planck’s distribution law and then introduce the basics of Quantum Electrodynamics (QED). As such, the interaction between atoms and radiation requires a transition from classical to quantum mechanics. 2017 MRT It is convenient to impose boundary conditions that areperiodicateachfaceof thecube. In the x direction, for example, it will be required that all plane wave satisfy eikx x =eikx (x+L) as a result of which kx =(2π/L)Nx (Nx =0,±1,±2,…).Similarly, for propagations in the y and z directions, we have ky =(2π/L)Ny (Ny =0,±1,±2,…) and kz =(2π/L)Nz (Nz =0,±1,±2,…). These components define the propagation vector k (or wave vector): which may be normalized to give the unit vector: )ˆˆˆ( π2ˆπ2ˆπ2ˆπ2ˆˆˆ kjikjikjik zyxzyxzyx NNN L N L N L N L kkk ++=++=++= Electromagnetic Interactions We shall be interested, at first, in a pure radiation field in which E(r,t) and B(r,t) are perpendicular to one another and both are perpendicular to the direction of propagation. The electromagnetic field is describable by a vector potential A(r,t); a complete description requires the specification of the three components of A(r,t) at each point in space and at each instant of time. Such a description leads to problems of normalization which can be avoided by assuming that the radiation field is enclosed in a cavity.* * For simplicity the cavity is assumed to be a cube of side L with perfectly conducting walls. Provided L is large compared with the dimensions of any physical system with which the radiation field may interact, physical results will be independent of the size and shape of the cavity. in the direction of propagation. For a plane wave we have k=ωk/c. ( )kkk == kkˆ 150
- 151. The imposition ofperiodic boundary conditions give rise to a discrete set of modes in the cavity. Although the number of modes is infinite, it is a denumberable infinity which is mathematically simpler than the continuous infinity of modes existing in free space. 2017 MRT For a box whose dimensions are large compared to the wavelength of the radiation we may regard the modes as forming a quasi-continuous distribution (i.e., a ‘pool’ of water versus a ‘bucket’ of water) in which the relation above for ∆N may be replaced by: This gives the number of propagation modes contained in an interval dk and in the direction defined by the element of solid angle dΩ. Ω=Ω = = = dkdk V ddkk L ddkdk L kdkdkd L dN zyx 2 3 2 3 2 3 3 π)2(π2 sin π2 π2 ϕθθ Each set of integers {Nx ,Ny ,Nz} defines a mode in the cavity (apart from the polarization). The number of modes ∆N contained in an interval specified by ∆Nx, ∆Ny, and ∆Nz is simply the product: zyxzyx kkk L NNNN ∆∆∆ =∆∆∆=∆ 3 π2 151
- 152. We now expressthe vector potential A(r,t) as a linear superposition of plane waves: 2017 MRT ∑∑ ∑∑ = −•−−• −•−−•−•−−• += +++= k rkrk k rkrk k rkrk kk kkkk kkke kkkekkkerA 2,1 )ω(*)ω( )ω(* 2 )ω( 22 )ω(* 1 )ω( 11 ]e)(e)()[(ˆ ]e)(e)()[(ˆ]e)(e)()[(ˆ),( r ti r ti rr titititi AA AAAAt where êr(k) is a real unit vector which indicates the linear polarization; êr(k) depends on the propagation direction k and has two independent components ê1(k) and ê2(k) which satisfy êr(k)•ês(k) =δrs (r,s=1,2). Ar(k) is a constant amplitude for the mode (k,r). The Coulomb gauge ∇∇∇∇•A=0 ensures the transversality of the electromagnetic fields so that êr(k)•k=0. Thus ê1(k), ê2(k), and k form a right-handed set of mutually orthogonal unit vectors. ˆ ˆ The vector potential A(r,t) above then consists of plane wave each one labeled by the propagation vector k and the real polarization vector êr(k). Furthermore, A(r,t) is real. We may also replace the linear polarization vectors ê1(k) and ê2(k) by unit vectors which indicate circular polarizations. This is accomplished by defining ê+(k)=−(1/√2)[ê1(k) ++++iê2(k)] and ê−(k)=(1/√2)[ê1(k)−−−−iê2(k)]. These vectors satisfy êr(k) ××××ês(k)=iδrs (r,s=±1). With r=+1 the cross product gives a vector parallel to the direction of propagation whereas with r=−1 the cross product is antiparallel. For this reason one refers to ê+(k) and ê−(k) as positive and negative helicity (unit) vectors. ê+(k) and ê−(k) represent left and right circular polarization, respectively. 152
- 153. The electric andmagnetic fields are obtained directly from the vector potential. For the electric field: For the magnetic field, since it is defined by B(r,t)=∇∇∇∇××××A(r,t), it is necessary to evaluate ∇∇∇∇××××A. Noting that ∇∇∇∇××××(ϕA)=∇∇∇∇ϕ ××××A++++ϕ∇∇∇∇××××A we have: 2017 MRT Since both the amplitude Ar(k) and the polarization vector êr(k) are constant the second term vanishes and we get: Hence the magnetic field is: ∑∑ −•−−• −= ∂ ∂ −= k rkrk k kk kkke rA rE r ti r ti rr AA c i t t c t ])e()e([)(ˆω ),(1 ),( )ω(*)ω( )(ˆ)e()(ˆe)()(ˆ)e( kekkekkek rkrkrk r i rr i rr i r AAA ××××∇∇∇∇××××∇∇∇∇××××∇∇∇∇ ••• += )(ˆˆ)e( ω )(ˆe)()(ˆ)e( kekkkekkek rkkrkrk r i rr i rr i r A c iAA ××××××××∇∇∇∇××××∇∇∇∇ ••• +== ∑∑ −•−−• −== k rkrk k kk kkkekrArB r ti r ti rr AA c i tt ])e()e(][)(ˆˆ[ω),(),( )ω(*)ω( ××××××××∇∇∇∇ 153
- 154. To obtain theHamiltonian for the electromagnetic field in the cavity it is necessary to express the field energy W in terms of canonical variable. From electromagnetic theory: where V is the volume of the cavity and the fields E and B are given by the expressions derived earlier. Because of the boundary conditions of the type exp(ikx x)=exp[ikx (x+L)], we get: 2017 MRT Therefore: If we write: ( ) ( ) = ≠ =∫ •± 0 00 e k krk for for V dV V i ti rr AtA k kk ω e)(),( − = ∫∫ +=•+•= VV dVdVW )( π8 1 )( π8 1 22 BEBBEE VdVVdV V i V i kk rkk kk rkk ′ •′−± −′ •′+± == ∫∫ δδ )()( ee and in our previous expression for E(r,t) and use the orthogonality condition êr(k)•ês(k)=δrs on the polarization vectors and the relation ωk =ω−k, we get: ∑∑∑∑∑∫ −+−−•−=• k k k k kkkkkekekkEE r s srsr r rr V tAtAtAtA c V tAtA c V dV )],(),(),(),()][(ˆ)(ˆ[ω),(),(ω 2 **2 2 *2 2 154
- 155. To compute thefield energy associated with B we employ the vector identity (A××××B)•(C××××D)=(A•C)(B•D)−(A•D)(B•C) to show that [k××××êr(k)]•[−k××××ês(k)]=δrs in which the orthogonality property êr(k)•k=0 has been used. We also have [k××××êr(k)]•[−k××××ês(k)]=−êr(k)•ês(−k). 2017 MRT Actually, W is dependent of time because of the exponential time dependence of Ar(k,t) as given previously. Hence: It is observed that the total energy is merely the sum of the energies in the individual modes as a consequence of the orthogonality conditions ∫V exp(±i(k+k′)•r)dV =δk′−kV and ∫V exp(±i(k−k′)•r)dV =δk′kV; furthermore the energy is shared equally by the electric and magnetic fields. ∑∑= k k kk r rr tAtA c V W ),(),(ω π2 *2 2 ˆ ˆ ˆ Therefore in computing the integral of B•B using the expression B(r,t) derived previously one finds two terms identical to the two terms on the right side of the ∫V E•EdV except for the sign of the second term. The field energy then becomes: ˆ ˆ ∑∑= k k kk r rr AA c V W )()(ω π2 *2 2 155
- 156. We now introducea new set of variables: which when substituted into our previous expression for W, gives: 2017 MRT )]()(ω[ π ω )()]()(ω[ π ω )( * kkkkkk k k k k rrrrrr PiQ V c APiQ V c A −=+= and ∑∑ += k k kk r rr QPW )](ω)([ 2 1 222 Upon inverting the Qr(k) and Pr(k) variables above, we get: )]()([ 2 ω π )()]()([ 2 1 π )( ** kkkkkk k rrrrrr AA c V iPAA c V Q −−=+= and Finally, it is noted that the Hamiltonian W=½ΣkΣr[Pr 2(k)+ωk 2Qr 2(k)] is precisely of the same form as that for an assembly of simple harmonic oscillators whose Hamiltonians are given by H=½(P2 +ω2Q2). Each mode of radiation field is therefore formally equivalent to a single harmonic oscillator when we let P2 =p2/m and Q2 =mq2 so that the Hamiltonian H=p2/2m+(m/2)ω2q2 became: )ω( 2 1 222 QPH += 156
- 157. Having identified theHamiltonian of each mode of a radiation field with that of a harmonic oscillator we may proceed with the quantization exactly as in the case of the harmonic oscillator. The transition to quantum mechanics is accomplished by interpreting Qr(k) and Pr(k) as operators that satisfy the commutation relations: 2017 MRT By analogy with the work done for the harmonic oscillator (i.e., a=[1/√(2hω)](ωQ+iP) and a† =[1/√(2hω)](ωQ−iP)) we define: which then must obey, the commutation rules: In term of these operators the Hamiltonian W=½Σkr[Pr 2(k)+ωk 2Qr 2(k)] becomes: 0])(,)([])(,)([])(,)([ =′=′=′ ′ kkkkkk kk srsrsrsr PPQQiPQ andδδh Quantization of the Radiation Field ])()(ω[ ω2 1 )(])()(ω[ ω2 1 )( † kkkkkk k k k k rrrrrr PiQaPiQa −=+= hh and 0)](),([)](),([)](),([ †† =′=′=′ ′ kkkkkk kk srsrsrsr aaaaaa andδδ ∑∑∑∑∑∑ += +== k k k k k kkkk r r r rr r r NaaHH 2 1 )(ω 2 1 )()(ω)( † hh in which Nr(k) is known as the number operator for the mode k and polarization r and is given by: )()()( † kkk rrr aaN = 157
- 158. The eigenvalues ofNr(k) are nr(k)=0,1,2,…, that is: where |nr(k)〉 represents an eigenstate of Nr(k). We see then, from the Hamiltonian H derived above, that the possible energies of the system which are the eigenvalues of H are: 2017 MRT Furthermore, the harmonic oscillator analogy permits us to write: consistent with a|n〉=√n|n−1〉, a†|n〉=√(n+1)|n+1〉 and a|0〉=0. )()()()( kkkk rrrr nnnN = ∑∑∑∑ +== k k k kk r r r r nEE 2 1 )(ω)( h 00)(1)(1)()()()()()()( † =++== kkkkkkkkk rrrrrrrrr annnannna and, 158
- 159. The quantity nr(k),also known as occupation numbers, are eigenvalues of the number operator Nr(k) and give the number of photons in the mode k and polarization r. The corresponding oscillator will have a set of levels with an energy difference hωk between adjacent levels (see Figure below which is the photon description of a radiation field). 2017 MRT The transition to quantum mechanics by means of the preceding formalism lends itself to the following interpretation: ar(k) and a† r(k) are annihilation and creation operators, respectively, for a photon with propagation vector k, and polarization vector êr(k), frequency ω, momentum hk, and energy hωk. 0 1 2 3 4 • • • nr (k) Photons • • • 1 • • • 20 5 6 hωk 159
- 160. A complete descriptionof the radiation field consists of an enumeration of the occupation numbers nr(k). Since each mode is independent of the product of all eigenstates, |nr(k)〉 is an eigenstate of the total field. A many-photon state is therefore described by: The notation is becoming quite cumbersome; we shall therefore make the replacement nri (ki) =ni so that the many-photon state is written as: 2017 MRT With the orthogonality requirement: for operators, we also replace the indices ri by the index i. The many-photon analog of ar(k)|nr(k)〉=√ nr(k)|nr(k)−1〉, a† r(k)|nr(k)〉=√[nr(k)+1]|nr(k)+1〉 and ar(k)|0〉=0 is: with: LLLL ),(,),(),()()()( 2121 2121 irrrirrr ii nnnnnn kkkkkk = ∑ += i ii nE 2 1 ωh ( ))(,,,, 21 irii i nnnnn k≡LL LLLLLL ii nnnnnnii nnnnnn ′′′=′′′ δδδ 2211 ,,,,,,,, 2121 as well as ai|n1,n1,…,0,…〉=0, and the analog of Nr(k)|nr(k) 〉=nr(k) |nr(k)〉 is: LLLL ,,,,,,,, 2121 iiii nnnnnnnN = LLLLLLLL ,1,,,1,,,,,1,,,,,,, 2121 † 2121 ++=−= iiiiiiii nnnnnnnannnnnnna & 160
- 161. When nr(k)=0, thereare no photons in the mode (k,r); nevertheless the energy in the mode, according to E=ΣkΣr Er(k) =ΣkΣr hωk[nr(k)+½], is ½hωk. This value, which is characteristic of the harmonic oscillator, is known as the zero point energy. 2017 MRT Since observables associated with non-commutating operators are subject to the uncertainty principle, an increase in precision in the number of photons means an increase in the uncertainty in the fields. For the radiation field as a whole with an infinite number of modes, the zero point energy becomes infinite. This is one of the particularities of the quantum-mechanical description of the radiation field. A formal explanation is based on the non-commutativity of the number operator Nr(k) with the annihilation and creation operators ar(k) and a† r(k) as a result of which ΣkΣrNr(k) does not commute with the fields E and B. When no photons are present, the fluctuations in the field strengths are responsible for the infinite zero point energy. Fortunately, a consistent description of physical processes in practically all cases is obtained by simply ignoring the infinite zero point energy of the radiation field. 161
- 162. The transition fromclassical to quantum mechanics seen in [Qr(k),Ps(k′)]=ihδkk′δrs and [Qr(k),Qs(k′)]=[Pr(k),Ps(k′)]=0 which implies that the classical vector potential A(r,t)=ΣkΣr êr(k){Ar(k) exp[i(k•r−ωkt)]+Ar * (k)exp[−i(k•r−ωkt)]} becomes a quantum- mechanical operator. 2017 MRT We now write the quantum-mechanical vector potential by substituting Ar(k) and Ar *(k) into A(r,t)=ΣkΣrêr(k){Ar(k) exp[i(k•r−ωkt)]+Ar *(k)exp[−i(k•r−ωkt)]} and we get: )( ω 2π )()( ω 2π )( † 2 * 2 kkkk kk rrrr a V c Aa V c A hh →→ and ∑∑ −•−−• += k rkrk k kk kkkerA r ti r ti rr aa V c t ]e)(e)()[(ˆ ω 2π ),( )ω(†)ω( 2 H h This transition may be accomplished by means of the transformations Qr(k)=√(V/π)(1/2c)[Ar(k)+Ar *(k)] and Pr(k)=−i√(V/π)(ωk/2c )[Ar(k)−Ar *(k)] which relate Ar(k) and Ar *(k) to Qr(k) and Pr(k); the latter in turn are related to the annihilation and creation operators ar(k) and ar †(k), via ar(k)=[1/√(2hωk)][ωk Qr(k)+iPr(k)] and ar †(k)=[1/√(2hωk)][ωk Qr(k) −iPr(k)]. Hence the required replacement is: which is spelled out in the Heisenberg representation. In the Schrödinger representation: ∑∑ •−• += k rkrk k kkkerA r i r i rr aa V c ]e)(e)()[(ˆ ω 2π )( † 2 h 162
- 163. The electric andmagnetic field operators may also be expressed in terms of ar(k) and ar †(k). Using our results for A(r) only, as well as Ar(k) and Ar *(k) just obtained, we get: Similarly, with this result for E(r) above, as well as Ar(k) and Ar *(k) again, we get: 2017 MRT ∑∑ •−• −= k rkrkk kkkerE r i r i rr aa V i ]e)(e)()[(ˆ ω2π )( †h ∑∑ •−• −= k rkrkk kkkekrB r i r i rr aa V i ]e)(e)()][(ˆˆ[ ω2π )( † ×××× h 163
- 164. The total Hamiltonianfor a radiation field interacting with an atomic system may be written as a sum of the three following terms: Here: 2017 MRT is the Hamiltonian for the free field. The Atomic Hamiltonian is: where V contains whatever terms are necessary to define the atomic state, e.g., Coulomb interaction with the nucleus, Coulomb repulsion among electrons, spin-orbit interaction external fields, etc. For HInteraction we refer to the Schrödinger equation and pick out all terms which contain the vector potential. nInteractioAtomicRadiation HHHH ++= ∑∑ += k k kk r rr aahH ]½)()([ω † Radiation V m p H i i + = ∑ 2 2 Atomic ψϕϕψϕ •−•−−•+ +=+′ pAAp ××××∇∇∇∇ΣΣΣΣ∇∇∇∇∇∇∇∇××××∇∇∇∇ΣΣΣΣ 2222 2 23 42 48822 1 )( cm e cm e cm p mc e c e m eE hhh 164
- 165. These terms are: However,the Coulomb gauge ∇∇∇∇•A=0 for the radiation field also leads to p•A=A•p; hence the Interaction Hamiltonian is: 2017 MRT AApAAp ××××∇∇∇∇ΣΣΣΣ •++•+• mc e cm e cm e cm e 2222 2 2 2 h 321 2 2 2 nInteractio 22 HHH mc e cm e cm e H ++= •++•= AAAp ××××∇∇∇∇ΣΣΣΣ h 165
- 166. The total HamiltonianH=HRadiation +HAtomic +HInteraction may now be written as: where: ∑∑∑∑ •−+•− •=•= k rk kk rk k kpkekpke r i rr r i rr a Vm e Ha Vm e H e)(])(ˆ[ ω 2π e)(])(ˆ[ ω 2π †)( 1 )( 1 hh and )( 1 )( 1 † 1 ]e)(e)(][)(ˆ[ ω 2π +− •−• += +•= ∑∑ HH aa Vm e H r i r i rr k rkrk k kkpke h With the use of the vector potential obtain A(r)=ΣkΣr êr (k){Ar (k)exp[i(k•r−ωkt)]+ Ar *(k)exp[−i(k•r−ωkt)]} earlier: in which H0 contains HRadiation and HAtomic and HInteraction is given by the expression derived above. We now assume that HInteraction can be regarded as a perturbation on H0 although, as in previous cases, the justification is not apparent until the calculations have been completed. We shall concentrate on the term, H1, of the perturbed Hamiltonian: nInteractio0 HHH += Ap•= cm e H1 which is most often the dominant term in electromagnetic interactions. 2017 MRT 166
- 167. For a singlemode characterized by (k,r) or what amounts to the same thing, for photons of momentum hk and polarization r, we have: 2017 MRT in which |ψa〉 represents the initial atomic state and |ψb〉 represents the final atomic state. In the same fashion, we have: a r i rb r ra r i rrrbrarb V n m e nan Vm e nHn ψψ ψψψψ ∑∑ ∑∑ • •− •= •−=− k rk k k rk k pke k kkpkekkk e])(ˆ[ ω )(2π )(;e)(])(ˆ[1)(; ω 2π )(;1)(; )( 1 h h a r i rb r ra r i rrrbrarb V n m e nan Vm e nHn ψψ ψψψψ ∑∑ ∑∑ •− •−+ • + = •+=+ k rk k k rk k pke k kkpkekkk e])(ˆ[ ω ]1)([2π )(;e)(])(ˆ[1)(; ω 2π )(;1)(; †)( 1 h h 167
- 168. Matrix elements ofthe type: maybesimplifiedif k•r<<1so that eik•r ≈1. Using the Atomic Hamiltonian HAtomic=Σi(pi 2/2m) +V and the commutation law [ri,p2]=2ihpi (ri =x, y,z), we have [r,HAtomic] =(ih/m)p. Thus: 2017 MRT For an atom, r is the position vector of an electron referred, most conveniently, to the nucleus; Ea and Eb are the eigenvalues for the Atomic Hamiltonian corresponding to the eigenstates |ψa〉 and |ψb〉, respectively; and ωab =ωk by energy conservation. The approximation eik•r ≈1 in the vector potential corresponds to the approximation in which all the terms in the multipole expansion of the field are neglected except for the electric dipole (E1) term. Therefore within the electric dipole approximation: and: a i bra i rb ψψψψ rkrk pkepke •• •≡• e)(ˆe])(ˆ[ ab abbaabababa i b mi miEE mi H i m ψψ ψψψψψψψψ r rrrp k rk ω ω)(],[e Atomic = =−==• hh abrabra i br im ψψψψψψ rkepkepke k rk •=•• • )(ˆω)(ˆe)(ˆ and abr r rarb abr r rarb V n ienHn V n ienHn ψψψψ ψψψψ rke k kk rke k kk • + =+ •=− + − )(ˆ ]1)([2π )(;1)(; )(ˆ )(2π )(;1)(; E1 )( 1 E1 )( 1 h h 168
- 169. The next higherapproximation is exp(ik•r)≈1+ik•r. To analyze the matrix elements containing the operator k•r (=r•k) it is convenient to write: where OA stands for the antisymmetric combination and OS for the symmetric combination. We consider the two terms separately. For OA, using the vector identity (A•C)(B•D)−(A•D)(B•C)≡(A××××B)•(C××××D): 2017 MRT L kkk µkekLkekLkekLkek prkekkprkekrpkekprrpke •−=•=•=•= ו=••−••=•−•= )](ˆˆ[ ω )](ˆˆ[ ω )](ˆˆ[ 2 ω )](ˆ[ )()](ˆ[)]()(ˆ[)]()(ˆ[)()(ˆ 2 1 2 1 2 1 2 1 A rrBrr rrrr e m i e m i c i i iiiO ×××××××××××××××× ×××× µ h SA 2 1 2 1 )()(ˆ)()(ˆ)(ˆ)]()(ˆ[ OO iiii rrrr +≡ •+•+•−•=••≡•• kprrpkekprrpkekrpkerkpke LL k k µkBµkekkk •=•−== −− )()](ˆˆ)[( ω2π )( ω 2π )( A )( 1 rrrr a V iOa Vm e H ×××× hh µB is the Bohr magneton and µµµµL (=µBL) the magnetic moment operator associated with the orbital angular momentum L. The vector k××××êr (k) lies in the direction of the magnetic field as is evidentfrom B(r)=iΣkΣr√(2πhωk/V )[k××××êr (k)][ar (k)exp(ik•r)−ar †(k)exp(−ik•r)]. The connection with the magnetic field can be made more explicit by writing the complete interaction asociated with OA. From our previous definition for H1 (−), we get: ˆ ˆ where Br (−)(k)is the term containingar(k) inB(r)=iΣkΣr√(...)[k××××êr(k)][ar(k)exp(ik•r)−...].ˆ 169
- 170. But in rectangularcomponents, this can be expressed as (omitting the proof): 2017 MRT For the M1 component of Ay (l = 2) we have: The result of this analysis is that OA or −Br (−)(k)•µµµµL or any of the equivalent forms of these operators are magnetic dipole (M1) operators. To determine the multipole character of the interaction −Br (−)(k)•µµµµL we may take the propagation vector k in the direction of the z-axis and the polarization vector êr(k) in the x-axis, k××××êr(k) is in the direction of the y-axis and: ˆ ˆ )()()()](ˆˆ[ 2 1 2 1 2 1 A zxyr pxpzkikikiO −=×=ו= prprkek ×××× )( )ˆˆˆ()ˆˆ()( 2 1 2 1 M1 )2( zx zyxzxx pxpzki pppxzki −= ++•−=•= kjieepA l )()( 2 1 M1 )2( zyy pypzki −=•= pA l which corresponds to the polarization vector êr(k) pointing in the direction of the y-axis since then k××××êr(k) is in the direction of the negative x-axis giving:ˆ )()()()](ˆˆ[ 2 1 2 1 2 1 A zyxr pypzkikikiO −=×−=ו= prprkek ×××× 170
- 171. 2017 MRT in which µµµµJis the total magnetic moment operator associated with both orbital and spin angular momenta. There is really no need to limit the magnetic moment operators to those which are associated with the orbital angular momentum. We may as well include the spin which will now take into amount the term proportional to ΣΣΣΣ•∇∇∇∇××××A in HInteraction. This amounts to adding 2S to L in OA =−i(mωk/e)[k××××êr(k)]•µµµµL. Hence the matrix elementsformagnetic (M1) transitions may now be written as: ˆ aBbr r abr r rarrbrarb V n i V n i nnnHn ψµψ ψψ ψψψψ )2()](ˆˆ[ )(ω2π )](ˆˆ[ )(2π )(;)(1)(;)(;1)(; )( M1 )( 1 SLkek k µkek k kkBµkkk k J J +•= •−= •−−=− −− ×××× ×××× h h aBbr r abr r rarrbrarb V n i V n i nnnHn ψµψ ψψ ψψψψ )2()](ˆˆ[ ]1)([ω2π )](ˆˆ[ ]1)([2π )(;)(1)(;)(;1)(; )( M1 )( 1 SLkek k µkek k kkBµkkk k J J +• + −= • + = •−+=+ ++ ×××× ×××× h h and: 171
- 172. For the symmetricoperator OS, we have: The replacement of p according to [r,HAtomic] = (ih/m)p gives: 2017 MRT and: which is identified as an operator as being the electric quadrupole (E2). krrkekrrke ••−=••−−= abr ba abrabab m EE m O ψψψψψψ )(ˆ 2 ω )(ˆ)( 2 S h kprrpke •+•= )()(ˆ2 1 S riO krrkekrrrrke ••=•+•−= ]),[)(ˆ 2 ]),[],([)(ˆ 2 AtomicAtomicAtomicS H m HH m O rr hh jirQ δ2 2 1−= rr which is an irreducible tensor of rank 2. We now have: )(2 1 S zx pxpzkiO += kke kkekke k k ˆ)(ˆ 2 ω )(ˆ 2 ω )(ˆ 2 ω 2 S ••−= ••−=••−= abr abrabr ba ab Q c m Q m Q m O ψψ ψψψψψψ The operator rr is a symmetric Cartesian tensor of rank 2. It is observed that êr(k)•〈ψb|r2δij |ψa〉•k=〈ψb|r2δij |ψa〉êr(k)•k=0 as a consequence of the transversality condition (i.e., ∇∇∇∇••••A=0 ⇒ êr (k)•k=0). Therefore rr may be replaced by: ˆ ˆ ˆ The multipole character of OS =(m/2h)êr(k)•[rr,HAtomic] may be investigated: 172
- 173. The matrix elementsfor electric quadrupole (E2) transitions may now be written as: 2017 MRT kke k kk k ˆ)(ˆ )(ω2π 2 )(;1)(; 3 E2 )( 1 ••−=− − abr r rarb Q V n c e nHn ψψψψ h and: kke k kk k ˆ)(ˆ ]1)([ω2π 2 )(;1)(; 3 E2 )( 1 •• + =+ + abr r rarb Q V n c e nHn ψψψψ h The discussion up to this point involved the interaction of a radiation field with a single electron. We shall now summarize the important expressions and put them in the form applicable to a multi-electron system. However, we shall continue to use a single mode characterized by (k,r). For a system containing N electrons let: where rj is the position vector of the j-th electron. For an atom the natural reference is the nucleus; more generally, as in molecules, rj is referred to the center of gravity of the positive charge. ∑= = N j j 1 rR 173
- 174. The interaction matrixelements are then the following: 2017 MRT kke k kk kke k kk k k ˆ)(ˆ ]1)([ω2π 2 )(;1)(; ˆ)(ˆ )(ω2π 2 )(;1)(; E2 3 E2 )( 1 3 E2 )( 1 •• + =+ ••−=− ∑ ∑ + − a j jbr r rarb a j jbr r rarb Q V n c e nHn Q V n c e nHn ψψψψ ψψψψ h h :)(QuadrupoleElectric a j j br r rarb a j j br r rarb V n inHn V n inHn ψψψψ ψψψψ ∑ ∑ • + =+ •−=− + − J J µkek k kk µkek k kk )](ˆˆ[ ]1)([2π )(;1)(; )](ˆˆ[ )(2π )(;1)(; M1 M1 )( 1 M1 )( 1 ×××× ×××× h h :)(DipoleMagnetic abr r rarb abr r rarb V n ienHn V n ienHn ψψψψ ψψψψ Rke k kk Rke k kk • + =+ •=− + − )(ˆ ]1)([2π )(;1)(; )(ˆ )(2π )(;1)(; E1 E1 )( 1 E1 )( 1 h h :)(DipoleElectric 174
- 175. In the descriptionof absoption and emission processes in atoms it is often permissible to replace the totality of atomic states by just two discrete states between which the transition occurs (see Figure – zero point energy has been omitted). 2017 MRT Such a simplification is possible if the energy separation between two states of an atom correspond to the photon energy hω whereas all other levels are spaced so that there are no energy differences close to hω. Assuming this to be the case we consider an atom in the state |ψa〉 interacting with a radiation field described by |nr (k)〉. The initial state of the entire system, i.e., atom plus field, is |ψA〉=|ψa;nr (k)〉. If absorption takes place, the atom makes a transition to the state |ψb〉 and there is one photon fewer in the field. Hence the final state of the system is |ψB〉=|ψb;nr (k)−1〉.* Thus: Transition Probabilities |ψb 〉 ABSORPTION EMISSION |ψa 〉 |ψb 〉 |ψa 〉 |ψa 〉 |ψb 〉 |ψa 〉 |ψb 〉 |ψA〉 = |ψa ; n〉 EA = Ea + nhω |ψB〉 = |ψb ; n – 1〉 EB = Eb + (n – 1)hω |ψB〉 = |ψb ; n + 1〉 EB = Eb + (n + 1)hω |ψA〉 = |ψa ; n〉 EA = Ea + nhω * In this notation reference to other atomic states or other photons is suppressed since they are not involved in the physical process. ( ) ( ) )ωω(ω ω]½)([1)(; ω]½)([)(; kk k k kk kk −=−−=− −+=−= ++== baabAB rbBrbB raAraA EEEE nEEn nEEn hh h h ψψ ψψ in which Ea and Eb are the energies of the initial and final atomic states, respectively,and: abba EE −=ωh 175
- 176. Now for abrief preamble on time-dependent perturbation theory. governs the evolution of a quantum system in time. H, the Hamiltonian of the system, may or may not itself be time-dependent. In a purely formal way, we saw that: 2017 MRT with: in which U(t,to) is known as an evolution operator. To obtain ψ(t) one may attempt to solve the time-dependent Schrödinger equation or one may seek the detailed form of the evolution operator since both methods give ψ(t) at an arbitrary time t once ψ(to) at an initial time to is known. A useful interpretation of U(t,to) may be obtained as follows: Suppose a system is known to be in the state ψa at an initial time to. At a later time t the system has evolved into the state U(t,to)ψa. The probability amplitude that U(t,to)ψa is a particular state ψb is given by the overlap integral 〈ψb|U(t,to)|ψa〉 which is the projection of ψb on U(t,to)ψa. 1),( oo =ttU 2 o ),( abba ttUW ψψ= ),( ),( tH t t i r r ΨΨΨΨ ΨΨΨΨ = ∂ ∂ h )(),()( oo tttUt ψψ = Hence the probability of finding a system in a state ψb at a time t when the system is known to have been in the state ψa at time t =to is: We know that the Schrödinger equation: 176
- 177. If it isstipulated that the Hamiltonian is independent of time, as will henceforth be assumed, a formal solution to the Schrödinger equation ih∂tΨΨΨΨ(r,t)=HΨΨΨΨ(r,t) is: in which the exponential operator is interpreted as: 2017 MRT Comparing ψ(t)=U(t,to)ψ(to) with ΨΨΨΨ(r,t)=exp[iHo(t−to)/h]ΨΨΨΨ(r,to), it is seen that an explicit form for the evolution operator is: Another formulation for the evolution operator is obtained by converting ih∂tU(t,to)= HU(t,to) and condition U(to,to)=1 into the integral equation: )( o o e),( ttH i ttU −− = h ),(e),( o )( o tt ttH i rr ΨΨΨΨΨΨΨΨ −− = h ∫ ′′−= t t tdttUH i ttU o ),(1),( oo h K hh h +− +−+= −− 2 o 2 2 o )( )( 1 !2 1 )( 1 1e o ttH i ttH i ttH i 177
- 178. And now fora preamble on the interaction representation… In which effects on the system due to Ho are the dominant ones while those due to VI (the perturbation potential) are relatively weaker and vary with time. 2017 MRT in terms of which we have the orthonormal set ϕk(r) which satisfies: ),()(),( ),( Io tVHtH t t i rr r ΨΨΨΨΨΨΨΨ ΨΨΨΨ +== ∂ ∂ h Io VHH += )()(o rr kkk EH ϕϕ = We shall seek solutions to the time-dependent Schrödinger equation: tE i k kk k tct h − ∑= e)()(),( rr ϕΨΨΨΨ with the decomposition: It is assumed that the time-independent Hamiltonian for the system can be written as the sum of two terms: 178
- 179. So, with: 2017 MRT If thislast equation is multiplied on the left by ϕk *(r) and integrated over r one obtains with the orthonormality on the ϕk(r): This is the differential equation whose solution provides the coefficients in the expansion ΨΨΨΨ(r,t)=Σk c(t)ϕk(r)exp(−iEk t/h). The quantity |cl(t)|2 is the probability of finding the system in the state ϕl at the time t. tE i k kk tE i k k k kk tctV t tc i hhh −− ∑∑ = ∂ ∂ e)()()(e)( )( I rr ϕϕ ∑ −− = ∂ ∂ k tEE i kkl l kl tctV t tc i )( I e)()( )( hh ϕϕ and: tE i k kk k tct h − ∑= e)()(),( rr ϕΨΨΨΨ in which case: tE i k kk tE i k k k k tE i k kkk k kk tctVtHtVH t tc EitcE t t i h hh hh − −− ∑ ∑∑ +=+ ∂ ∂ += ∂ ∂ e)()()(),(),()( e)( )( e)()( ),( IoIo rrr rr r ϕ ϕϕ ΨΨΨΨΨΨΨΨ ΨΨΨΨ 179
- 180. If cl (1) vanishesfor some reason or if a better approximation is called for, we use: 2017 MRT where: and so on… )2()1( lll ccc +≅ tdtd i tdtctV i tc n t t tEE i tEE i n t tEE i nnll knnl nl ′′ ′′′′ = ′′′′′′−= ∑∫ ∫ ∑∫ ′′ ′−′′− ′′− 0 0 )()( 2 0 )( )1( I )2( ee e)()()( hh h h h knnl tVtV ϕϕϕϕϕϕϕϕϕϕϕϕϕϕϕϕ )()( II ϕϕ Suppose only state m is populated when the time-dependent perturbation is turned on at t =0; then: mkkc δ=)0( ∫ ′′−= ′−t tEE i l td i tc kl 0 )( )1( e)( h h kl tV ϕϕϕϕϕϕϕϕ )(I We may the approximate cl by: From these last equations for cl (1) and cl (2) we can calculate any (order) of perturbation to a physical effect under consideration – even self-interaction effects! 180
- 181. When we aredealing with the emission and absorption of a photon by an atom, we may work with just cl (1). The time-dependent potential VI(t) can be written as: 2017 MRT where V′I is a time-independent operator. Hence: We can readily perform the time integration and obtain: where we have used: ti eVtV ω II )( m ′= Note that the transition probability per unit time is |cl (1)(t)|2/t, which is independent of t. )( sin π 1 lim 2 2 x x x δ α α α = ∞→ ∫ ′′−= ′−t tEE i kll tdV i tc kl 0 )ω( I )1( e)( hm h h ϕϕ ω)( π2 )( 2 I 2 )1( hm h klkll EEtVtc −′= δϕϕ ω)( π2 2 I hm h klkl EEV −′ δϕϕ 181
- 182. We now considerthe formal interaction representation which is particularly appropriate for certain formulation of time-dependent perturbation theory. 2017 MRT and an operator AI(t) in the same representation is given by: The time derivative of ψI(t) is: This is an equation of motion for the wave function in the interaction representation while the other equation of motion is for the operators AI(t): )(e)( o I tt tH i ψψ h= tH i tH i tAtA oo e)(e)(I hh − = t t tH i t t tH i tH i ∂ ∂ += ∂ ∂ )( e)(e )( oo o I ψ ψ ψ hh h and upon substituting ih∂ψ/∂t=Hψ =(Ho +V)ψ, we find: )()()(eee)(e )( II I oooo ttVtVtV t t i tH i tH i tH i tH i ψψψ ψ === ∂ ∂ − hhhhh ]),([ )( oI I HtA t tA i = ∂ ∂ h Let the time-independent Hamiltonian H in the Schrödinger representation be written as the sum of two parts Ho +V and a wave function ψI(t) in the interaction representation is defined by: 182
- 183. We now definean operator UI(t,to) by: with: Upon combining ψ(t)=U(t,to)ψ(to) with ψI(t)=exp(iHot/h)ψ(t) one finds: 2017 MRT from which it is concluded that: with the second equality based on U(t,to)=exp[−iH(t−to)/h]. The unitarity property of U(t,to) confers unitarity of UI(t,to) so that: )(),()( oIoII tttUt ψψ = ooooooo eeee),(e),( )( ooI tH i ttH i tH i tH i tH i ttUttU hhhhh −−−− == 1),( ooI =ttU )(e),(e)(),(e)(e)( oIoooI ooooo tttUtttUtt tH i tH i tH i tH i ψψψψ hhhh − === ),(),( o 1 Io † I ttUttU − = We may now also find the differential equation obeyed by UI(t,to) by substituting ψI(t)= UI(t,to)ψI(to) into ih∂ψI(t)/∂t=VI(t)ψI(t). Since ψI(to) is constant: ),()( ),( oII oI ttUtV t ttU i = ∂ ∂ h 183
- 184. We shall nowlet: and if we specialize to the case of a transition between the stationary states ϕa and ϕb, the transition probability is: The equality between the two forms in the above for wba arises as a result of UI(t,to)= exp(iHot/h)U(t,to)exp(−iHoto/h) which merely introduces a phase factor into the matrix elements when U(t,to) is replaced by UI(t,to). Since the probability is determined by the absolute square of the matrix elements, such phase factors are of no consequence. We shall use the form |〈ϕb|UI(t,to)|ϕa〉|2 to evaluate the probability per unit time: 2017 MRT Since the time dependence of 〈ϕb|UI(t,to)|ϕa〉 is contained entirely in the operator UI(t,to) which satisfies ih∂UI(t,to)/∂t=VI(t)UI(t,to), we have: bbbaaa EHEHVHH ϕϕϕϕ ==+= ooo and, 2 oI 2 o ),(),( ababba ttUttUW ϕϕϕϕ == ]),(),([),( * oIoI 2 oI abababba ttUttU td d ttU td d W ϕϕϕϕϕϕ == ]),(),()(Im[ 2 ]),(),()([ ]),()(),(),(),()([ * oIoII oIoII * oIIoI * oIoII abab abab ababababba ttUttUtV ttUttUtV i ttUtVttUttUttUtV i W ϕϕϕϕ ϕϕϕϕ ϕϕϕϕϕϕϕϕ h h h = −−= −= conjugatecomplex 184
- 185. We shall nowinsert(i.e.,using AI(t)=exp(iHot/h)A(t)exp(−iHot/h) and UI(t,to)=exp(iHot/h) ×exp[−iH(t–to)/h]exp(−iHoto/h)) VI(t)=exp(iHot/h)Vexp(−iHot/h) andUI(t,to)=UI(t,0)UI(0,to)= exp(iHot/h)exp(−iHt/h)UI(0,to) into Wba =(2/h)Im[〈ϕb|VI(t)UI(t,to)|ϕa〉〈ϕb|UI(t,to)|ϕa〉*] to obtain: Up to this point to was arbitrary; it will now be assumed that to →−∞. This will allow us to apply the perturbation V in adiabatically by which it is meant that V is multiplied by the convergence factor exp(−ε|t|/h) (ε >0). This is a convenient mathematical device and has the effect of causing the perturbation to vanish at very early and very late times but to remain unaffected at t=0. Therefore, with ψa=UI(0,−∞)ϕa an eigenfunction of H with eigenvalue Ea we get: 2017 MRT which is the reaction matrix. with: abba VR ψϕ= = −− * oIoI ),0(ee),0(eeIm 2 oo a tH i tH i ba tH i tH i bba tUtUVW ϕϕϕϕ hhhh h ]Im[ 2 ]Im[ 2 eeeeIm 2 * ** abba abab tE i ab tE i tE i ab tE i ba R VVW aaaa ψϕ ψϕψϕψϕψϕ h hh hhhh = = = −− 185
- 186. We shall nowreplace ψa in Wba =(2/h)Im[Rba〈ϕb|ψa〉*] by the Lippmann-Schwinger equation (stated here without proof): where we use the notation ψI(−∞)=ϕa and ψI(0)=ψa with the assumption that VI(−∞)=0 and Hoϕa =Eaϕa. By the way, this permits us to identifyUI(0,−∞)=1+limε →0[1/(Ea−H+iε)]V. We then get: 2017 MRT so that the final result is the Fermi Golden Rule: Now: )( π2 2 bababa EERW −= δ h a a aa V iHE ψ ε ϕψ ε ++ += → o0 1 lim ++ +== → * o0 ** 1 limIm 2 ]Im[ 2 a a bbaabbaabbaba V iHE RRRW ψ ε ϕϕϕψϕ εhh Assuming ϕa ≠ϕb, the first term in the brackets vanishes and: ++ = ++ = →→ εε ψϕ εε iEE R iEE V RW ba ba ba ab baba 2 0 * 0 limIm 2 limIm 2 hh )(π )( lim )( 1 Im 22022 ba bababa EE EEEEiEE −= +−+− = ++ → δ ε ε ε ε ε ε and 186
- 187. We shall describethe absorption of a photon by means of the time-dependent perturbation theory just developed where we showed that the probability per unit time for a transition from an arbitrary state |ψa〉 to a state |ψb〉, to first order, is given by: in which the potential V is the perturbing potential in the Schrödinger representation. 2017 MRT From the Electric Dipole (E1) obtained earlier: we therefore have, for absorption: in which the δ function: )( π2 2 baablm EEVW −= δψψ h )()(ˆ )(ωπ4 2 22 Absorption ABabr r EE V ne W −•= δψψ Rke kk )ωω( 1 )ω()( kk −=−−=− baabAB EEEE δδδ h h ensures the conservation of energy for the system as a whole (atom plus radiation field.) abr r rarb V n ienHn ψψψψ Rke k kk •=− − )(ˆ )(2π )(;1)(; E1 )( 1 h The assumption of a radiation field with a single frequency ωk (or single mode nr(k)) is clearly unrealistic since it is impossible to achieve infinitely sharp frequencies. 187
- 188. Physically it ismore meaningful to assume a distribution of modes within a narrow range of frequencies. For a radiation field in a cubical enclosure, according to dN= [V/(2π)3]k2dkdΩ, the number of modes per unit energy interval is: 2017 MRT We shall therefore replace the infinitely sharp density distribution δ(Eb −Ea −hωk) in WAbsorption by (1/h)dN/dωk above to obtain: Here α =e2/hc is the fine structure constant and quantities such as ωk and nr(k) must be understood as averages within the interval. For emission, the calculation goes through in exactly the same way but with the matrix elements: Ω= d c V d dN 2 33 ω )π2(ω 1 k k hh Ω•= d c n dW abr r 2 2 3 Absorption )(ˆ π2 )(ω ψψ α Rke kk abr r rarb V n ienHn ψψψψ Rke k kk • + =+ + )(ˆ ]1)([2π )(;1)(; E1 )( 1 h The final result, analogous to that in dWAbsorption above is: Ω• + = d c n dW abr r 2 2 3 Emission )(ˆ π2 ]1)([ω ψψ α Rke kk 188
- 189. The two equationsfor dWAbsorption and dWEmission are the probabilities per unit time for absorption and emission, respectively, of a photon with propagation vector k, polarization r, and frequency ωk contained within an element of solid angle dΩ. We may now sum over the two independent polarizations and integrate over the solid angle: 2017 MRT Therefore the total probability per unit time for absorption of a photon regardless of direction of propagation or polarization is: in which the subscripts referring to the propagation vector and the polarization are no longer needed, The corresponding expression for emission is: 3 π8 sin sin)(ˆ sinsin)(ˆ cossin)(ˆ 2 22 2 1 2 1 =Ω =• =• =• ∫ ∑= d ab r abr abab abab k k kk kk RRke RRke RRke θ θψψψψ ϕθψψψψ ϕθψψψψ 2 2 3 Absorption 3 ω4 ab c n W ψψ α R= 2 2 3 Emission 3 )1(ω4 ab c n W ψψ α R + = z y x ê1 θk kˆ 〈ψb|R|ψa〉 ê2 ϕk 189
- 190. A close connectionbetween absorption and emission is apparent from a comparison on the expressions for WAbsorption and WEmission. Nevertheless there is an important distinction inasmuch as the absorption probability is proportional to n which the emission probability is proportional to n+1 which then permits WEmission to be written as a sum of two terms: where: 2017 MRT WInduced is identical with WAbsorption, and is known as the induced (or stimulated) emission probability per unit time since it is proportional to n, the number of photons of frequency ω. On the other hand WEmission is independent of n; it is therefore a spontaneous emission probability per unit time. Thus, if n=0, there can be no absorption or induced emission, but since WSpontaneous is non-zero, spontaneous emission can occur. sSpontaneouInducedEmission WWW += 2 2 3 sSpontaneou 2 2 3 Induced 3 ω4 3 ω4 abab c W c n W ψψ α ψψ α RR == and 190
- 191. If W(b,a) representsthe transition probability per unit time from the state |αa;Ja,Ma〉 with degeneracy ga to the state with degeneracy gb where αa and αb represent the quantum numbers required to complete the specification of the states |ψa 〉 and |ψb〉, respectively, we shall have, in place of WAbsorption =(4αω3n/3c2)|〈ψb|R|ψa〉|2 and WEmission = [4αω3(n+1)/3c2)]|〈ψb|R|ψa〉|2, we have: 2017 MRT In these expressions the probabilities for all possible transitions |αa;Ja,Ma〉→|αb;Jb,Mb〉 have been added, but since the probability of an electron being in any one of the initial states |αa;Ja,Ma〉 is 1/ga, the sum of the probabilities must be multiplied by the factor. WInduced and WSpontaneous are correspondingly modified. Note that: where W(b,a) stands for either WAbsorption(b,a) or WEmission(b,a) and the same for W(a,b). ),(),( abW g g baW b a = ∑∑ ∑∑ + = = a b a b M M aaabbb a M M aaabbb a MJMJ c n g abW MJMJ c n g abW 2 2 3 Emission 2 2 3 Absorption ,;,; 3 )1(ω41 ),( ,;,; 3 ω41 ),( αα α αα α R R 191
- 192. It is convenientto work with quantities that do not contain the degeneracy ga, gb and are therefore symmetric in the initial and final states. Such a quantity is the line strength S defined by: In terms of the line strength: 2017 MRT ∑∑== a bM M aaabbb MJMJeabSbaS 22 ,;,;),(),( αα R ),( 3 ω4 ),(),( ),( 3 ω4 ),(),( 3 3 AbsorptionInduced 3 3 AbsorptionInduced baS gc n baWbaW baS gc n abWabW b a h h == == and: ),( 3 ω4 ),( ),( 3 ω4 ),( 3 3 Spontanous 3 3 Spontanous baS gc baW baS gc abW b a h h = = 192
- 193. The Einstein Acoefficient in dipole approximation is defined by: where WSpontaneous is the spontaneous emission probability per unit time. One may also define the spontaneous lifetime τ of a state |ψa 〉 by: 2017 MRT The Einstein B coefficient is related to the absorption (or induced emission) probability per unit time by the relation: where I(ω)dω is the energy per unit volume for photons in the interval ω to ω+dω. This quantity consists of the product of: 1) the number of modes in the interval dωdΩ (recall (1/h)dN/dωk earlier); 2) the number of independent polarizations (2); 3) the average number of photons (n) in dω; 4) the average energy of a photon (hω) – this product is then integrated over dΩ and divided by the volume V. Thus: and upon replacing WAbsorption =WInduced +BI(ω) by (4αω3n/3c2)|〈ψb|R|ψa〉|2, we get: )ω(InducedAbsorption IBWW == 32 3 3 2 π ω )ω( 1 ω2 )(2π ωω ω)ω( c n Id V n c dV dI h h =⇒Ω= ∫ Aτ 1= Einstein Coefficients 2 2 3 sSpontaneou 3 ω4 ab c WA ψψ α R=≡ A cn B ab 3 332 2 32 ω ωπ 3 ωπ4 hh == ψψ α R 193
- 194. When we takeaccount of possible degeneracies, the Einstein A coefficient is written as: 2017 MRT ),( 3 ω4 ,;,; 3 ω4 ),(),( 3 3 2 2 3 sSpontaneou baS gc MJMJ gc abWabA aM M aaabbb a a b h === ∑∑ αα α R ),( 3 π4 ),( ω π ),( 2 3 32 baS g abA c abB ahh == and the Einstein B coefficient is: 194 ),( 3 ω4 ),( 2 3 baS g baA bh = with: with: ),( 3 π4 ),( 2 2 baS g baB bh =
- 195. Let us nowsuppose that the population (atoms per cm3) in |ψa 〉 and |ψb〉 are Na and Nb, respectively. When the system is in equilibrium, the number of transitions per unit time |ψa 〉→|ψb〉 will be equal to the number of transitions per unit time |ψb〉→|ψa〉 or: where a refers to the lower state and b to the upper state (see Figure – where the radiative transitions between two levels are shown). 2017 MRT In terms of the Einstein coefficients: )],(),([),( StimulatedInducedAbsorption baWbaWNabWN ba += Planck’s Law |ψ a〉 |ψ b〉 WSponteneous(a,b) WInduced(a,b) WAbsorption(b,a) )],()ω(),([)ω(),( baAIbaBNIabBN ba += But: ),( ω π ),(),( ω π ),( 3 32 3 32 baA g gc abBbaA c baB a b hh == and 195
- 196. Also, the equilibriumpopulation satisfies the Boltzmann distribution*: where Ei is the separation in energy between the states – in this case |ψa〉 and |ψb〉: 2017 MRT This is known as Planck’s distribution law, where I(ω)dω is the energy per unit volume (under conditions of thermodynamic equilibrium) for photons in the interval ω to ω+dω. Tk E b a b a B i g g N N e= 1e 1 π ω )ω( ω32 3 − = TkB c I h h Note that Planck’s law is independent of degeneracies (i.e., ga or gb). * In chemistry, physics, and mathematics, the Boltzmann distribution is a certain distribution function (or probability measure) for the distribution of the states of a system. It underpins the concept of the canonical ensemble, providing its underlying distribution. A special case of the Boltzmann distribution, used for describing the velocities of particles of a gas, is the Maxwell–Boltzmann (M-B) distribution. So, the Boltzmann distribution for the fractional number of particles Ni /N occupying a set of states i possessing energy Ei is Ni /N = [gi exp(−Ei /kB T )]/Z(T) where kB is the Boltzmann constant, T is the temperature, gi is the degeneracy (i.e., the number of levels having energy Ei), N = ΣiNi is the total number of particles and Z(T) = Σigi exp [−Ei/kB T ] is the partition function which encodes the statistical properties of a system in thermodynamic equilibrium. Alternatively, for a single system at a well-defined temperature, it gives the probability that the system is in the specified state. The Boltzmann distribution applies only to particles at a high enough temperature and low enough density that quantum effects can be ignored, and the particles are obeying M-B statistics. When the energy is simply the kinetic energy of the particle Ei = ½mv2 then the distribution correctly gives the M-B distribution of gas molecule speeds, previously predicted by James C. Maxwell (1831-1879) in 1859. [Source: Wikipedia] To study this subject (e.g., canonical ensembles, partition functions, etc.) in its entirety see Feynman, R., Statistical Mechanics (Perseus Books). Inserting these relations into NaB(b,a)I(ω)=Nb[B(a,b)I(ω)+A(a,b)], we obtain: ωh=−⇒ abi EEE 196
- 197. We caution notto confuse I(ω) with I(ν) where ω=2πν. Since 2017 MRT the relation between I(ω) and I(ν) is: νν dIdI )(ω)ω( = π2 )( )ω( νI I = which differs from B=(π2c3/hω3)A by a factor of 2π. Therefore: and in place of B=(π2c3/hω3)A, the Einstein coefficient Bν referred to Uν is related to the A coefficient by: A h c B 3 3 π8 ν ν = It is pertinent to remark that in semiclassical theory the spontaneous part of the emission probability emerges only after one attempts to reconcile Planck’s radiation law, taken to be established experimentally, with thermodynamic requirements. On the other hand, the quantum mechanical treatment of the radiation field gives the spontaneous emission automatically. )ω()( ωInducedAbsorption IBIBWW === νν 1e 1π8 )( 3 3 − = Tk c h I ν ν ν h The final result, as a function of frequency, is: 197
- 198. The theory ofradiative processes developed up to this point is not entirely self- consistent. The reason is that the atomic states |ψa〉 and |ψb〉 between which transitions are assumed to occur are solutions of a time-independent Schrödinger equation and hence are stationary states. But it was also found that there exists a spontaneous emission process so that all states except the lowest one must eventually decay and therefore cannot be stationary. This feature must be incorporated by using time- dependent perturbation theory and eventually leads to the conclusion that the excited atomic state is broadened to a width Γ=h/τ as a result of the spontaneous emission process, resulting in a Lorentzian line shape L(ω) in the emission spectrum whose full width at half-maximum is Γ. This is called line broadening. A similar situation must exist in absorption; that is, the absorption line is broadened due to the finite lifetime of the excited state. 2017 MRT A Note on Line Broadening What has been described so far is broadening due to the radiation process itself and the line width associated with it is sometimes called the “natural” line width. There are a number of other processes that contribute to the broadening of a spectral line such as collisions between atoms which might have the effect of interrupting the wave train of the radiation emitted by an atom – which is a classical viewpoint.Quantummechanically, one would say that an excited atom cannot only relax to the ground state by spontaneous emission but may also release its excitation energy to another atom in the course of an impact. The lifetime is therefore shortened and may be completely dominated bythe time between collisions. This is known as pressure or collision broadening. In gases, the random motion of atoms can also give rise to Doppler effects that will broaden a line. 198
- 199. The Photoelectric Effect Theeffect whereby the absorption of a photon by an atom results in the removal of an electron from a bound state, leaving the atom in an ionized condition, is known as the photoelectric effect. We shall calculate the cross section for this process under certain simplifying assumptions such as the initial state |ψi 〉 being the basic Hydrogen one (i.e., a 1s state |ψ100 〉) and the final state |ψf 〉 being a free electron (i.e., with momentum hq). normalized in the volume V. Then hq is the momentum of the electron, that is: The initial state |ψi 〉 is assumed to be a 1s state of an hydrogenoïd atom (Z≠0): Hence |ψf 〉Free is a momentum eigenfunction with eigenvalue hq. 2017 MRT o e π 1 2/3 o s1100 arZ i a Z − == ψψ where ao is Bohr’s radius: rq• = i f V e 1 Free ψ f ii f VV ψψ qqpp rqrq hh === •• e 1 e 1 199 ( ) ( )MKSmorCGScm 11 2 e 2 o o 8 e 2 e 2 o 1029.5 επ4 1052.0 −− ×==×=== em a cmem a hhh α and the final state |ψf 〉 is that of a free electron:
- 200. For photon absorptionit is necessary to evaluate the matrix elements: o o eee)(ˆ π eee)(ˆ π 1 )(ˆe 2/3 o 2/3 o s1Free arZii r arZii rir i f Va Z Va Z −•• −••• • = • =• rkrq rkrqrk qke pkepke h ψψ Coordinate system used in the calculations. qkK −−−−= Defining: The relationship among the various vectors is shown in the Figure; it is noted that: in spherical coordinates. If the coordinate system is oriented so that the z-axis is along the direction of K and the polarization vector ê along the x-axis: we have: ∫∫∫ ′′== • ∞ −−•−•• π 00 2 sineeπ2eeeee ooo θθ drdrd iarZarZiarZii rKrKrkrq r 222 o 2 3 o 0 π 0 )( π4 sine π4 eeesin 2 sine oo KaZ aZ drKrr K Kr rK d arZarZiii + ===′′ ∫∫ ∞ −−••• rkrqrK andθθ θ ϕθ cos2)( cossin)(ˆ 2222 qk qr −+== =• qkqkK qke −−−− 2017 MRT Thus: ])(ˆ[ )( )(π8 e 222 o 2 2/3 o qkerk • + =• ri i f KaZV ZZa h ψψ z y x ê θ k ϕ q K e z y x r e− Ze+ o e π 1 2/3 o arZ i a Z − =ψ rq• = i f V e 1 ψ 1s 200
- 201. It will beassumed that the photon energy is large compared with the binding energy of the electron in the atom but small compared to the rest mass of the electron. The first assumption ensures that the final state is that of a free electron and the second avoids the necessity of a relativistic treatment. Fortunately both conditions are satisfied when: The first factor on the right is of the order of the binding energy I and the second factor is 2⋅137/Z; hence E is at least several times larger than I. With the assumption that E=I, the photons energy becomes comparable to thekineticenergyof the electron hck=mov2/2 from which itfollowsthat k/q=v/2c=Z/qao orqao=Z and Z2 +ao 2K2 =Z2 +ao 2(k2 +q2 −2qkcosθ) ≈q2ao 2(1−β cosθ) (with β =v/c). Therefore the matrix element is: where k=|k| is the photon wave number, ao the Bohr radius, and Z the atomic number of the atom. To see the justification of this criterion we first calculate the photon energy corresponding to kao ≈ Z: and the complete interaction matrix element is: 22 o 2 2/3 o )]cos1([ cossin)(π8 )(ˆe θβ ϕθ ψψ − =•• aqV qZZa ir i f h pkerk 2017 MRT Zak ≈o === 22 4 o 2 o 2 2 eZ cemZ a Zc kcE h h h h ir i f V n m e ψψ pkerk •• )(ˆe ω π2 o h 201
- 202. On inserting theseexpressions into the Fermi Golden Rule with the density of states: and the differential cross section is: the probability per unit time for photoelectric absorption is: When θ =π/2, ϕ =0 or π, the cross section is a maximum, that is, when the momentum of the electron is parallel to the polarization of the photon. The angular factor in the de- nominator causes the electron emission to have a slight forward tilt which increases as v/c increases. Ignoring the factor (1−βcosθ)4, the total cross section is (with 1/|k|=c/ω): 2017 MRT Ω= d qmV Ed Nd 2 o 3 )π2( h Ω − = d mkaq Z c e V n qkWn 4 o 5 o 5 2252 )cos1( cossin32 ),;,( θβ ϕθ ϕθ h h 4 22 5 oo 2 )cos1( cossin1 32 θβ ϕθ σ − = = Ω a Z cmc e c VW d d qk h h h 5 ooω3 π128 =Ω Ω = ∫ a Z m d d d q hασ σ 202
- 203. Higher Order ElectromagneticInteractions The process of absorption and emission (e.g., the photoelectric absorption Wn(k,q;θ,ϕ)) described earlier are one-photon processes since in every transition the field either loses or gains a photon while the atom undergoes a corresponding change of state to keep the total energy (atom plus field) constant. In the absence of an external magnetic field the Hamiltonian describing the interaction of an electron with a radiation field is: There are other important interactions between an atom and a radiation field which involve a change of more than one photon. In a general scattering event, for example, the field loses an incident photon characterized by kr (e.g.,aspart of the sums Σk and Σr seen earlier) and gains another photon – the scattered one with momentum k′ – characterized by k′s. With laser sources, numerous multiphoton processes have been observed although the present discussion will be restricted to several aspects of the two-photon interaction. where A is given by A(r)=ΣkΣr√(2πhc2/V ωk)êr(k)[ar(k)exp(ik•r)−ar †(k)exp(−ik•r)] and the H3 term is neglected. 321 2 2 2 nInteractio 22 HHH mc e cm e cm e H ++= •++•= AσAAp ××××∇∇∇∇ h 2017 MRT 203
- 204. As we’ve donebefore, we let: For H2, we have: in which Hkr (+)Hk′s (+) refers to the term containing a† kr a† k′s; Hkr (−)Hk′s (−) to the term with ar (k)as(k′), etc. where: 2017 MRT { } )()()()()()()()( )(†)(† )()(†† 22 †† 22 2 e)()(e)()( e)()(e)()( ωω )(ˆ)(ˆπ ]e)(e)(][e)(e)()][(ˆ)(ˆ[ ωω 1π + ′ −− ′ +− ′ −+ ′ + •′−•′−− •′+•′+− ′ •′−•′•−• ′ +++= ′+′+ ′+′ ′• = ′+′+′•= ∑∑ ∑∑ srsrsrsr i sr i sr r i sr i sr sr r i s i s i r i rsr HHHHHHHH aaaa aaaa mV e aaaa mV e H kkkkkkkk rkkrkk k rkkrkk kk k rkrkrkrk kk kkkk kkkk keke kkkkkeke h h ∑∑∑∑ •−+•− •=•= k rk kk rk k kpkekpke r i rr r i rr a Vm e Ha Vm e H e)(])(ˆ[ ω 2π e)(])(ˆ[ ω 2π †)( 1 )( 1 hh & )( 1 )( 1 † 1 ]e)(e)(][)(ˆ[ ω 2π +− •−• += +•= ∑∑ HH aa Vm e H r i r i rr k rkrk k kkpke h 204
- 205. Matrix elements ofar (k) and ar †(k) may be obtained from ar (k)|nr (k)〉=√ nr (k)|nr (k)−1〉 and ar †(k)|nr (k)〉=√[nr (k)+1]|nr (k)+1〉: which leads directly to: Since the photon modes are independent we also have: The only non-vanishing matrix elements of ar †(k) and ar (k) are those which involve a change of one photon. For all bilinear combinations of ar †(k) and ar (k) the change in the number of photons must be zero or two. )()()(1)(1)()()(1)( † kkkkkkkk rrrrrrrr nnannnan =−+=+ and )()()(),()()(1)(,1)( ]1)()[()(),()()(1)(,1)( )(]1)([)(),()()(1)(,1)( ]1)(][1)([)(),()()(1)(,1)( † † †† kkkkkkkk kkkkkkkk kkkkkkkk kkkkkkkk ′=′′−′− +′=′′+′− ′+=′′−′+ +′+=′′+′+ srsrsrsr srsrsrsr srsrsrsr srsrsrsr nnnnaann nnnnaann nnnnaann nnnnaann 0)](),([)](),([)](),([ ††† =′=′=′ kkkkkk srsrsr aaaaaa 2017 MRT 205
- 206. We consider atwo-level system (see Figure). )(),(;I kk ′= sri nnψ Also scattering has occurred, ns (k′) is increased by one photon and nr (k) is decreased by one photon so that the total system is now in the state |F 〉: with energy (N.B., not including the zero point energy): ssrri nnEE kk kk ′′++= ω)(ω)(I hh with energy: 2017 MRT 1)(,1)(;F +′−= kk srf nnψ ssrrf nnEE kk kk ′+′+−+= ω]1)([ω]1)([F hh The energy Ei relative to Ef is arbitrary and the scattered photon may have more of less energy compared with the incident photon. This represents the general case of Raman scattering and includes elastic scattering as a special case. Initially the atom is in the state |ψi 〉 and two photon modes with occupation numbers nr (k) and ns (k′) (i.e., simultaneously observable – through momentums k and k′ photon eigenvalues of the occupation numbers) are present. The total system is in the state |I 〉: |ψf〉 SCATTERING |ψi〉 |ψf〉 |ψi〉 | I 〉 = |ψi ; nr(k),ns(k′)〉 | F 〉 = |ψf ; nr(k)−1,ns(k′) +1〉 206
- 207. From 〈nr (k)+1,ns(k′)+1|ar †(k)as †(k′)|nr (k),ns (k′) 〉=√{[nr (k)+1][ns (k′)+1]}, 〈nr (k)+1,ns (k′)−1|ar †(k)as †(k′)|nr (k),ns (k′)〉=√{[nr (k)+ 1]ns (k′)}, and also 〈nr (k)−1,ns (k′)+1|ar(k)as †(k′)|nr (k),ns (k′)〉=√{nr (k)[ns (k′)+1]} and finally with 〈nr (k)−1,ns (k′)−1|ar(k)as(k′)|nr (k),ns (k′)〉=√[nr (k)ns (k′)], it follows that: i i fsr sr srsr srsr nn mV e HHHH HHHH ψψ rkk kk keke kk kkkk kkkk •′− ′ +−−+ −−++ ′• +′ =′=′ =′=′ )( 2 )()()()( )()()()( e)](ˆ)(ˆ[ ωω ]1)()[(π I)()(FI)()(F 0I)()(FI)()(F h The scattering process is regarded as a two-photon process in the sense that an inci- dent photon of specified energy, polarization, and propagation direction symbolized by the indices kr (i.e., a discrete sum for each index) is scattered so that the emerging pho- ton may have a different energy, polarization, and direction of propagation symbolized by the indices k′s. Hence, a kr photon has been lost and a k′s photon has been gained! Therefore: 2017 MRT i i fsr sr nn mV e H ψψ rkk kk keke kk •′− ′ ′• +′ = )( 2 2 e)](ˆ)(ˆ[ ωω ]1)()[(2π IF h Matrix elements of H1 will be of type ar(k)|nr(k)〉=√nr(k)|nr(k)−1〉 and ar †(k)|nr(k)〉= √[nr(k)+1]|nr(k)+1〉 in which where is a chance of one photon. Therefore H1 cannot contribute to scattering in the first order of perturbation theory but may contribute to higher order. The matrix elements of H2 are non-vanishing when there is a change of two photons; first order contributions from H2 are therefore expected. 207
- 208. The contribution ofH1 in second order may be obtained from the Fermi Golden Rule: with 〈ψk |R|ψm〉 to second-order given by: ∑ − += l lm mllk mkmk EE VV VR ψψψψ ψψψψ where | I 〉 and | F 〉 are given by | I 〉=|ψi ;nr(k),ns(k′)〉 and | F 〉=|ψf ;nr(k)−1,ns(k′)+1〉, respecti-vely and for the intermediate state | ψl 〉 there are two possibilities: Now let: FI == km ψψ and +′= ′−= = 1)(),(; )(,1)(; 2 1 2 1 kk kk srl srl l nnL nnL ψ ψ ψ 2017 MRT )( π2 2 mkmkmk EERW −= δψψ h 208
- 209. Hence there aretwo paths from | I 〉 to | F 〉: In the first path the transition | I 〉→| L1 〉 involves the loss (absorption) of a kr photon accompanied by an atomic transition |ψi〉→|ψl1 〉; the transition | L1 〉→| F〉 involves the gain (emission) of a k′s photon with an atomic transition |ψl1 〉→| ψf 〉. In the second path there is an emission of a k′s photon with an atomic transition |ψi〉→| ψl1 〉 in the step | L2 〉→| F〉. 2017 MRT FI FI 2 1 →→ →→ L L :2Path :1Path It is important to note that in each path the intermediate state differs from the initial and final states by just one photon. These steps may be symbolized by the Diagrams below showing the contribution from the p•A term in second order to photon scattering. | I 〉 | F 〉| L2 〉 | I 〉 | F 〉| L1 〉 k k′ kk′ t = t′′ k k′ k k′ t = t′ | I 〉 | I 〉 | F 〉 | F 〉 | L1 〉 | L2 〉 209
- 210. We saw earlierthat H1 (−) was associated with the absorption of a single photon and H1 (+) with the emission of a single photon. Therefore the matrix elements in the two paths are the following: 2017 MRT ir i lls i f sr srisrl srlsrf nn Vm e nnHnn nnHnnHLLH L ψψψψ ψψ ψψ ])(ˆ[e])(ˆ[e ωω ]1)()[(2π )(),(;)(,1)(; )(,1)(;1)(,1)(;IF FI 11 1 1 2 2 )( 1 )( 1 )( 111 )( 1 1 pkepke kk kkkk kkkk rkrk kk ••′ +′ = ′′−× ′−+′−= →→ ••′− ′ + +−+ h For is i llr i f sr srisrl srlsrf nn Vm e nnHnn nnHnnHLLH L ψψψψ ψψ ψψ ])(ˆ[e])(ˆ[e ωω ]1)()[(2π )(),(;1)(),(; 1)(),(;1)(,1)(;IF FI 22 2 2 2 2 )( 1 )( 1 )( 122 )( 1 2 pkepke kk kkkk kkkk rkrk kk •′• +′ = ′+′× +′+′−= →→ •′−• ′ + −+− h For 210
- 211. Both paths contributeto the second-order term in 〈ψk| R|ψm〉=〈ψk |V |ψm〉 +Σl[〈ψk|V |ψl〉〈ψl|V |ψm〉/(Em−El)]. On setting: the second-order term becomes: kk ′−−=−+−=− ωω 2211 II hh liLliL EEEEEEEE and in which the general summation index l indicates the sum over all intermediate states accessible to one-photon transitions. 2017 MRT −− •′• + +− ••′+′ ′ •′−• ••′− ′ ∑ k rkrk k rkrk kk pkepke pkepkekk ω ])(ˆ[e])(ˆ[e ω ])(ˆ[e])(ˆ[e ωω ]1)()[(2π 2 22 1 11 2 2 h h h li is i llr i f l li ir i lls i fsr EE EE nn Vm e ψψψψ ψψψψ 211
- 212. 2017 MRT The first-order contributiondue to the Hamiltonian H2 was given by: earlier and is to be added to: just derived so as to obtain the total matrix element 〈 F | HInteraction| I 〉 for the scattering process. We shall also adopt the dipole approximation whereby all exponentials are set to unity. Thus, and restoring | I 〉=|ψi;nr(k),ns(k′)〉 and | F 〉=|ψf ;nr(k)−1,ns(k′)+1〉, we get: −− •′• + +− ••′ + +′• +′ =′+′− ′ ′ ∑ kk kk pkepkepkepke keke kk kkkk ω )(ˆ)(ˆ ω )(ˆ)(ˆ1 )](ˆ)(ˆ[ ωω ]1)()[(2π )(),(;1)(,1)(; 2 nInteractio hh h li isllrf l li irllsf ifsr sr srisrf EEEEm nn mV e nnHnn ψψψψψψψψ δψψ −− •′• + +− ••′+′ ′ •′−• ••′− ′ ∑ k rkrk k rkrk kk pkepke pkepkekk ω ])(ˆ[e])(ˆ[e ω ])(ˆ[e])(ˆ[e ωω ]1)()[(2π 2 22 1 11 2 2 h h h li is i llr i f l li ir i lls i fsr EE EE nn Vm e ψψψψ ψψψψ i i fsr sr nn mV e H ψψ rkk kk keke kk •′− ′ ′• +′ = )( 2 2 e)](ˆ)(ˆ[ ωω ]1)()[(2π IF h 212
- 213. This last matrixelement for 〈 F |HInteraction| I 〉 may now be inserted into the Fermi formula Wkm =(2π/h)|〈ψk|R|ψm〉|2δ(Ek −Em) with the δ function replaced by a density of final states: The transition probability per unit time that an incident kr photon has been scattered into the element of solid angle dΩ as a k′s photon then becomes: This is the Kramers-Heisenberg dispersion formula (1925 – before QM; and Dirac, 1927). 2017 MRT Ω= d c V d dN 2 33 ω )π2(ω 1 k k hh 2 2 2 2 ω )(ˆ)(ˆ ω )(ˆ)(ˆ1 )](ˆ)(ˆ[]1)()[( ω ω −− •′• + +− ••′ + +′•+′Ω = ′ ′ ∑ kk k k pkepkepkepke kekekk hh li isllrf l li irllsf ifsrsr EEEEm nnd V c mc e W ψψψψψψψψ δ The intermediate states |ψl〉 which appear in theKramers-Heisenbergdispersionformu- la,aswellastheinitial state |ψi〉 and the final state |ψf 〉 arealleigenstates of theatom, i.e., solutions of the atomic Schrödinger equation. By virtue of the existence of matrix ele- ments between |ψi〉 and |ψl〉 and |ψl〉 and |ψf〉, the two states |ψi〉 and |ψf〉 can interact via two-photon processes. However, the transition |ψi〉→|ψl 〉 and |ψl 〉→| ψf 〉 are not observable – the states |ψl〉 are sometimes called “virtual” because of this feature. 213
- 214. The Kramers-Heisenberg dispersionformula may also be expressed as a differential scattering cross section: where: 2017 MRT 2 2 o ω )(ˆ)(ˆ ω )(ˆ)(ˆ1 )](ˆ)(ˆ[]1)([ ω ω )( −− •′• + +− ••′ + +′•+′= Ω = ′ = Ω ′ ′ ∑ kk k k pkepkepkepke kekek k k k hh li ilslfr l li ilrlfs ifsrs r EEEEm nr Vcn dW r s d d ψψψψψψψψ δ σ 2 cm-secscattered/photonsincidentofnumber sr-secscattered/photonsofnumber electrontheofradiusclassical== 2 2 o mc e r The formula above for the differential cross section contains the factor [ns(k′′′′)+1]; hence the cross section is a sum of two terms one of which is proportional to ns (k′′′′), the number of scattered photons. This term corresponds to stimulated Raman scattering; it contributes negligibly at ordinary intensities, but produces important effects at high intensities. 214
- 215. 2017 MRT ir i f r if rf ri V n m e HHR n n ψψ ψ ψ ])(ˆ[e ω )(π2 IFIF 1)(;F )(;I )( 11 )1( pke k k k rk k •=== −= = •− h Starting withthe Fermi Golden Rule Wkm =(2π/h)|〈ψk|R|ψm〉|2δ(Ek −Em) in which the general form of the matrix elements is 〈ψk |R|ψm〉≡Rkm = Rkm (1)+ Rkm (2) +…and it is possible to symbolize these expressions by means of diagrams which are helpful in writing the pertinent matrix elements associated with a particular process: Single photon emission: ir i f r if rf ri V n m e HHR n n ψψ ψ ψ ])(ˆ[e ω ]1)([π2 IFIF 1)(;F )(;I )( 11 )1( pke k k k rk k • + === −= = •−+ h k | I 〉 | F 〉 k | I 〉 | F 〉 Single photon absorption: 215
- 216. Processes involving twophotons (second order processes) are scattering, two-photon absorption and two-photon emission. Scattering has already been discussed earlier (the pertinent diagrams were shown 7 slides ago): 2017 MRT Two-photon absorption: ∑ ∑ ∑∑ ′ •′• ′ ••′ ′ −−−− +− •′•′ = +− ••′′ = +≡ − + − = −′=−′−= ′−=′= 2 2 22 1 1 11 2 21 1 2 1 ω ])(ˆ[e])(ˆ[e ωω )()(π2 ω ])(ˆ[e])(ˆ[e ωω )()(π2 IFIF 1)(),(;1)(,1)(;F )(,1)(;)(),(;I 2 2 4 2 2 3 43 I )( 122 )( 1 I )( 111 )( 1)2( 2 1 l li is i llr i fsr l li ir i lls i fsr L LL L if srlsrf srlsri EE nn Vm e M EE nn Vm e M MM EE HLLH EE HLLH R nnLnn nnLnn k rkrk kk k rkrk kk pkepkekk pkepkekk kkkk kkkk h h h h ψψψψ ψψψψ ψψ ψψ | I 〉 | F 〉| L2 〉 k′ k where | I 〉 | F 〉| L1 〉 k k′ 216
- 217. Two-photon emission: 2017 MRT ∑ ∑ ∑∑ ′ •′−•− ′ •−•′− ′ ++++ −− •′+′+ = −− ••′+′+ = +≡ − + − = +′=+′+= ′+=′= 2 2 22 11 11 2 21 1 2 1 ω ])(ˆ[e])(ˆ[e ωω ]1)(][1)([π2 ω ])(ˆ[e])(ˆ[e ωω ]1)(][1)([π2 IFIF 1)(),(;1)(,1)(;F )(,1)(;)(),(;I 2 2 6 2 2 5 65 I )( 122 )( 1 I )( 111 )( 1)2( 2 1 l li is i llr i fsr l li ir i lls i fsr L LL L if srlsrf srlsri EE nn Vm e M EE nn Vm e M MM EE HLLH EE HLLH R nnLnn nnLnn k rkrk kk k rkrk kk pkepkekk pkepkekk kkkk kkkk h h h h ψψψψ ψψψψ ψψ ψψ | I 〉 | F 〉| L2 〉 k′ k where | I 〉 | F 〉| L1 〉 k k′ 217
- 218. All the matrixelements considered thus far were of the operator H1 in HInteraction =H1 +H2; for H2 =Hr (+)(k)Hs (+)(k′) +Hr (−)(k)Hs (−)(k′) +Hr (+)(k)Hs (−)(k′)+Hr (−)(k)Hs (+)(k′) we have the following (note that in dipole approximation there is no contribution from the A2 term to two-photon absorption or emission; as shown previously the contribution to scattering occurs only in the elastic case): 2017 MRT Scattering: i i fsr sr srsrif srf sri nn Vm e HHHHHR nn nn ψψ ψ ψ rkk kk keke kk kkkk kk kk •′−− ′ +−−+ ′• +′ = ′=′== +′−= ′= )( 2 )()()()( 2 )1( e)](ˆ)(ˆ[ ωω ]1)()[(π2 I)()(F2I)()(F2IF 1)(,1)(;F )(),(;I h k′k | I 〉 | F 〉 218
- 219.
- 220. C. Harper, Introductionto Mathematical Physics, Prentice Hall, 1976. California State University, Haywood This is my favorite go-to reference for mathematical physics. Most of the complex variable and matrix definitions, &c. are succinctly spelled out and the wave packet discussions served as a primer followed by more in-depth discussion reference of Appendix A of Sakurai’s book below and also the solutions to the wave function (i.e. Radial, Azimutal and Angular) are very well presented and treated in this very readable 300 page volume. J.J. Sakurai, Modern Quantum Mechanics, Revised Edition, Addison-Wesley, 1994. University of California at Los Angeles Only half (Chapters 1-3 or about the first 200 pages) of this book makes for quite a thorough introduction to modern quantum mechanics. Most of the mathematical and angular momentum framework (i.e. orbital, spin and total as well as spherical harmonics discussed earlier) is based on Sakurai’s post-humanous presentation of the subject. S.S. Schweber, An Introduction to Relativistic Quantum Field Theory, Dover, 1989. Brandeis University Quite a voluminous 900 page tome written by a colleague of Hans Bethe. Most of the postulates and rotation invariance discussion, which was really the base or primer for the rest, is based on the first 50 pages of this book. The other 50 pages formed the basis of the relativistic material as well as the Klein-Gordon and Dirac equations. S. Weinberg, Lectures on Quantum Mechanics, Cambridge University Press, 2017. Josey Regental Chair in Science at the University of Texas at Austin Soon to become the seminal work on Quantum Mechanics, this ‘course’ from Weinberg fills-in all the gaps in learning and exposition required to fully understand the theory. Including the Historical Introduction, I would definitely suggest Chapter 2-4 and do the problems! Once you do, you will have truly mastered the theory to handle the next set of books from Weinberg – especially QFT I & II. S. Weinberg, The Quantum Theory of Fields, Volume I, Cambridge University Press, 1995. Josey Regental Chair in Science at the University of Texas at Austin Volume 1 (of 3) introduces quantum fields in which Chapter 1 serves as a historical introduction whereas the first 5 sections of Chapter 2 make for quite an elevated introduction to the relativistic quantum mechanics discussed here. Weinberg’s book was crucial in setting up the notation and the Lorentz and Poincaré transformations and bridging the gap for the relativistic one-particle and mass zero states using Wigner’s little group. Very high level reading! M. Weissbluth, Atoms and Molecules, Academic Press, 1978. Professor Emeritus of Applied Physics at Stanford Weissbluth’s book starts by knocking you off your chair with Angular Momentum, Rotations and Elements of Group Theory! The text is mathematical physics throughout but where more than one sentence occurs, the physics (or quantum chemistry) abounds. Chapter 15 starts Part III – One-electron Atoms with Dirac’s equation and in Chapter 16, it offers most of the explanation of the coupling terms generated from the Dirac equation. 2017 MRT References / Study Guide 220
- 221. Epilogue “Where did weget that [equation] from? Nowhere. It is not possible to derive it from anything you know. It came out of the mind of Schrödinger!” Richard Feynman ),(),(ˆ t t itH rr ΨΨΨΨΨΨΨΨ ∂ ∂ = h 2017 MRT 221
- 222. 8O (1s22s2p4) The formulafor the Oxygen atom is much more complicated but it can be represen- ted by the Bohr model of electrons orbiting a nucleus (8 protons and 8 neutrons). 2017 MRT 222
- 223. Ice forms asa Hydrogen bond between water molecules by a polar H bond: δ+-δ−. H bond H2O (1s[1 -1s22s2p[2] -1s1]) 2017 MRT 223
- 224. sp2 p 6C (1s22s2p2) Side viewTop view 2017 MRT 224 p and sp2 Valence Orbitals of Carbon.
- 225. When 6 Carbonatoms (6× 1s22s2p2) get together they become Benzene (C6H6). Benzene is a natural constituent of crude oil. C6H6 2017 MRT 225
- 226. Caffeine is acentral nervous system (CNS) stimulant (N.B., it is also a diuretic so drink lots – 2.5l – of water too!), having the effect of warding off drowsiness and restoring alertness. Beverages containing caffeine, such as coffee, tea, soft drinks and energy drinks,enjoypopularitygreat enough to make caffeine the world’s most widely consu- med psychoactive substance.In North America,90%of adults consume caffeinedaily. C8H10N4O2 2017 MRT 226
- 227. Adenosine is anucleoside comprised of adenine attached to a ribose (ribofuranose) moiety (i.e., each of two parts into which a thing is or can be divided) via a β-N9- glycosidic bond. Adenosine plays an important role in biochemical processes, such as energy transfer - as adenosine triphosphate (ATP) and adenosine diphosphate (ADP) - as well as in signal transduction as cyclic adenosine monophosphate, cAMP. It is also an inhibitory neurotransmitter, believed to play a role in promoting sleep and suppressing arousal, with levels increasing with each hour an organism is awake. C10H13N5O4 2017 MRT 227
- 228. Caffeine Adenosine Caffeine’s principalmode of action is as an antagonist of adenosine receptors in the brain. They are presented here side by side for comparison. 2017 MRT 228
- 229. The dietary formof Vitamin A (Retinol), is a yellow fat-soluble, antioxidant vitamin important in vision and bone growth. C20H30O 2017 MRT 229
- 230. Chlorophyll is anessential component of photosynthesis, which helps plants get energy from the light. Chlorophyll molecules are specifically arranged in and around pigment protein complexes called photosystems, which are embedded in the thylakoid membranes of chloroplasts. Mg 2017 MRT 230 C55H72O5N3Mg
- 231. C63H88CoN14O14P Vitamin B12 (Cyanocobalamin)is important for the normal functioning of the brain and nervous system and for the formation of blood. It is involved in the metabolism of every cell of the body. R = (CN, OH, CH3, Deoxyadenosyl) Corrin ring Rib Dimethylbenzimidazol Co 2017 MRT 231
- 232. Insulin is usedmedically in some forms of diabetes mellitus. Patients with type 1 diabetes mellitus depend on exogenous insulin (commonly injected subcutaneously) for their survival because of an absolute deficiency of the hormone; patients with type 2 diabetes mellitus have either relatively low insulin production or insulin resistance or both, and a non-trivial fraction of type 2 diabetics eventually require insulin administration when other medications become inadequate in controlling blood glucose levels. C257H383N65O77S6 2017 MRT 232 Nitrogen
- 233. The hemoglobin moleculein humans is an assembly of four globular protein subunits. Hemoglobin is synthesized in the mitochondria of the immature red blood cell throughout its early development from the proerythroblast to the reticulocyte in the bone marrow, when the nucleus has been lost. C2952H4664N812O832S8Fe4 Heme group 233 2017 MRT Fe
- 234. Red blood cell ββββchain αααα chain ββββ chain αααα chain Heme groupIron (Fe) Helical shape of the polypeptide molecule 234 2017 MRT
- 235.
- 236. 236 2017 MRT A bacteriophage isa virus that attacks bacteria. The phiX174 bacteriophage attacks the common human bacteria Escherichia coli, infecting the cell and forcing it to make new viruses. Do you think that viruses are living organisms? phiX174 is composed of a single circle of DNA surrounded by a shell of proteins. That's all. It can inject its DNA into a bacterial cell, then force the cell to create many new viruses. These viruses then burst out of the cell, and go on to hijack more bacteria. By itself, it is like an inert rock. But given the proper bacterial host, it is a powerful reproducing machine. This phage has a very small amount of DNA. It has 11 genes in 5386 bases (it is single stranded) in a circular topology. Several of them expressing similar function in two groups.
- 237.
- 238. 238 2017 MRT A cross-section ofa human cell. Nucleus Golgi apparatus Cilium Microvilli Chromatin Mitochondrion Centriole Lysosome Endoplasmic reticulum Nucleons Free ribosome Nuclear pores Peroxisome Ribosome
- 239. 239 2017 MRT Nucleus of theHuman Cell. Nucleolus Chromosomes Chromatin Nuclear pores Nuclear envelope
- 240. Human (X-) Chromosomes.A chromosome is a packaged and organized structure containing most of the DNA of a living organism. 240 2017 MRT
- 241. Deoxyribo-Nucleic acid, orDNA, is a nucleic acid molecule that contains the genetic instructions used in the development and functioning of all known living organisms. The main role of DNA is the long-term storage of information and it is often compared to a set of blueprints, since DNA contains the instructions needed to construct other components of cells, such as proteins and RNA molecules. The DNA segments that carry this genetic information are called genes, but other DNA sequences have structural purposes, or are involved in regulating the use of this genetic information. DNA is what composes the human chromosomes – just DNA! 241 2017 MRT
- 242. Only Adenine &Thymine and Guanine & Cytosine form base DNA structure pairs. Guanine Adenine Thymine Cytosine Adenine Thymine Guanine Cytosine 2017 MRT 242
- 243. Nucleotide formation inwhich the 4 bases form the polynucleotide chains of DNA. 243 2017 MRT
- 244. 244 2017 MRT Adenine is acomponent of ADP which is an Energy Reserve (c.f., previous Slide).
- 245.
- 246.
- 247. 247 2017 MRT Centromere Each DNA moleculehas been packaged into a mitotic chromosome that is 50000× shorter than its extended length. Entire mitotic chromosome Condensed section of chromosome Section of chromosome in an extended form 30 nm chromatin fiber of packaged chromosome ‘Beads on a string’ form of chromatin Short region of DNA double helix 1400 nm 700 nm 300 nm 30 nm 11 nm 2 nm
- 248. Guanine Cytosine Thymine Adenine Uracil Chromosome Chromosome strand Sugar and Phosphate units Basepairs Amino acid (three pairs of bases) A. DNA ladder splits. B. One strand contains code for mRNA. Uracil C. The two strands form into a spiral. D. mRNA strand is formed with uracil replacing Thymine. E. The strands of DNA rejoin. 248 2017 MRT DNA contains the unique genetic informationforeachindividual(e.g., cell reproduction).
- 249. Cell division isessential for an organism to grow, but when a cell divides it must replicate the DNA in its genome so that the two daughter cells have the same genetic information as their parent. 2017 MRT 249 Topoisomerase DNA primase RNA primer DNA ligase DNA Polymerase (Polαααα) Lagging strand Leading strand Okazaki fragment DNA Polymerase (Polδδδδ) Helicase Single strand, binding proteins
- 250. 23 pairs ofchromosomes estimated to contain 50000 to 100000 genes. 250 2017 MRT
- 251. 2017 MRT Facsimile of theHuman Genome. 251