This document discusses rational functions and their properties. It defines a rational function as a function of the form f(x) = p(x)/q(x) where p and q are polynomials. It then discusses how to find the domain, vertical asymptotes, horizontal asymptotes, and oblique asymptotes of rational functions. The key points are: 1) the domain excludes values where the denominator equals 0, 2) vertical asymptotes occur where the denominator equals 0, 3) the degree of the numerator and denominator determine if there is a horizontal or oblique asymptote. Comparing degrees is essential to finding asymptotes of rational functions.

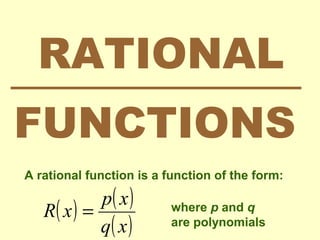
1. RATIONAL
FUNCTIONS
A rational function is a function of the form:
( ) ( )
( )xq
xp
xR = where p and q
are polynomials

2. ( ) ( )
( )xq
xp
xR =
What would the domain of a
rational function be?
We’d need to make sure the
denominator ≠ 0
( )
x
x
xR
+
=
3
5 2
Find the domain.{ }3: −≠ℜ∈ xx
( )
( )( )22
3
−+
−
=
xx
x
xH { }2,2: ≠−≠ℜ∈ xxx
( )
45
1
2
++
−
=
xx
x
xF
If you can’t see it in your
head, set the denominator = 0
and factor to find “illegal”
values.
( )( ) 014 =++ xx { }1,4: −≠−≠ℜ∈ xxx

3. The graph of looks like this:( ) 2
1
x
xf =
Since x ≠ 0, the graph approaches 0 but never crosses or
touches 0. A vertical line drawn at x = 0 is called a
vertical asymptote. It is a sketching aid to figure out the
graph of a rational function. There will be a vertical
asymptote at x values that make the denominator = 0
If you choose x values close to 0, the graph gets
close to the asymptote, but never touches it.

4. Let’s consider the graph ( )
x
xf
1
=
We recognize this function as the reciprocal
function from our “library” of functions.
Can you see the vertical asymptote?
Let’s see why the graph looks
like it does near 0 by putting in
some numbers close to 0.
10
10
1
1
10
1
==





f
100
100
1
1
100
1
==





f
10
10
1
1
10
1
−=
−
=





−f
100
100
1
1
100
1
−=
−
=





−f
The closer to 0 you get
for x (from positive
direction), the larger the
function value will be Try some negatives

5. Does the function have an x intercept?( )
x
xf
1
=
There is NOT a value that you can plug in for x that
would make the function = 0. The graph approaches
but never crosses the horizontal line y = 0. This is
called a horizontal asymptote.
A graph will NEVER cross a
vertical asymptote because the
x value is “illegal” (would make
the denominator 0)
x
1
0 ≠
A graph may cross a horizontal
asymptote near the middle of
the graph but will approach it
when you move to the far right
or left

6. Graph ( )
x
xQ
1
3+=
This is just the reciprocal function transformed. We can
trade the terms places to make it easier to see this.
3
1
+=
x
vertical translation,
moved up 3
( )
x
xf
1
=
( )
x
xQ
1
3+=
The vertical asymptote
remains the same because in
either function, x ≠ 0
The horizontal asymptote
will move up 3 like the graph
does.

7. Finding Asymptotes
VERTICALASYMPTOTES
There will be a vertical asymptote at any
“illegal” x value, so anywhere that would make
the denominator = 0
( )
43
52
2
2
−−
++
=
xx
xx
xR
Let’s set the bottom = 0
and factor and solve to
find where the vertical
asymptote(s) should be.
( )( ) 014 =+− xx
So there are vertical
asymptotes at x = 4
and x = -1.

8. If the degree of the numerator is
less than the degree of the
denominator, (remember degree
is the highest power on any x
term) the x axis is a horizontal
asymptote.
If the degree of the numerator is
less than the degree of the
denominator, the x axis is a
horizontal asymptote. This is
along the line y = 0.
We compare the degrees of the polynomial in the
numerator and the polynomial in the denominator to tell
us about horizontal asymptotes.
( )
43
52
2
+−
+
=
xx
x
xR
degree of bottom = 2
HORIZONTAL ASYMPTOTES
degree of top = 1
1
1 < 2

9. If the degree of the numerator is
equal to the degree of the
denominator, then there is a
horizontal asymptote at:
y = leading coefficient of top
leading coefficient of bottom
degree of bottom = 2
HORIZONTAL ASYMPTOTES
degree of top = 2
The leading coefficient
is the number in front of
the highest powered x
term.
horizontal asymptote at:
1
2=
( )
43
542
2
2
+−
++
=
xx
xx
xR
1
2
=y

10. ( )
43
532
2
23
+−
+−+
=
xx
xxx
xR
If the degree of the numerator is
greater than the degree of the
denominator, then there is not a
horizontal asymptote, but an
oblique one. The equation is
found by doing long division and
the quotient is the equation of
the oblique asymptote ignoring
the remainder.
degree of bottom = 2
OBLIQUE ASYMPTOTES
degree of top = 3
532 23
+−+ xxx432
−− xx
remaindera5 ++x
Oblique asymptote
at y = x + 5

11. SUMMARY OF HOW TO FIND ASYMPTOTES
Vertical Asymptotes are the values that are NOT in the
domain. To find them, set the denominator = 0 and solve.
To determine horizontal or oblique asymptotes, compare
the degrees of the numerator and denominator.
1. If the degree of the top < the bottom, horizontal
asymptote along the x axis (y = 0)
2. If the degree of the top = bottom, horizontal asymptote
at y = leading coefficient of top over leading
coefficient of bottom
3. If the degree of the top > the bottom, oblique
asymptote found by long division.

12. Problem 1
• In an inter-barangay basketball league, the
team from Barangay Culiat has won 12 out
of 25 games, a winning percentage of 48%.
How many games should they win in a row
to improve their win percentage to 60%?

13. From Problem No. 1
• We have seen that they need to win 8 games
consecutively to raise their percentage to
atleast 60%. What will be their winning
percentage if they win (a) 10 games in a
row (b) 15? 20? 30? 50? 100 games?

14. Why Should You Learn This?
• Rational functions are used to model and solve
many problems in the business world.
• Some examples of real-world scenarios are:
– Average speed over a distance (traffic
engineers)
– Concentration of a mixture (chemist)
– Average sales over time (sales manager)
– Average costs over time (CFO’s)

15. Domain
Find the domain of 2x
1f(x)
+
=
Denominator can’t equal 0
(it is undefined there)
2 0
2
x
x
+ ≠
≠ −
( ) ( )Domain , 2 2,−∞ − ∪ − ∞
Think: what numbers can I put in for x????

16. You Do: Domain
Find the domain of
2)1)(x(x
1-xf(x)
++
=
Denominator can’t equal 0
( ) ( )1 2 0
1, 2
x x
x
+ + ≠
≠ − −
( ) ( ) ( )Domain , 2 2, 1 1,−∞ − ∪ − − ∪ − ∞

17. You Do: Domain
Find the domain of =
+2
xf(x)
x 1
Denominator can’t equal 0
2
2
1 0
1
x
x
+ ≠
≠ −
( )Domain ,−∞ ∞

18. Vertical Asymptotes
At the value(s) for which the domain is undefined,
there will be one or more vertical asymptotes. List
the vertical asymptotes for the problems below.
2x
1f(x)
+
= 2x =−
2)1)(x(x
1-xf(x)
++
= 1, 2x x= − = −
=
+2
xf(x)
x 1
none

19. Vertical Asymptotes
The figure below shows the graph of 2x
1f(x)
+
=
The equation of the vertical asymptote is 2x=−

20. Vertical Asymptotes
• Set denominator = 0; solve for x
• Substitute x-values into numerator. The
values for which the numerator ≠ 0 are the
vertical asymptotes

21. Example
• What is the domain?
 x ≠ 2 so
 What is the vertical asymptote?
 x = 2 (Set denominator = 0, plug back into numerator,
if it ≠ 0, then it’s a vertical asymptote)
( ,2) (2, )−∞ ∪ ∞
2
2 3 1
( )
2
x x
f x
x
− −
=
−

22. You Do
 Domain: x2
+ x – 2 = 0
 (x + 2)(x - 1) = 0, so x ≠ -2, 1
 Vertical Asymptote: x2
+ x – 2 = 0
 (x + 2)(x - 1) = 0
 Neither makes the numerator = 0, so
 x = -2, x = 1
( , 2) ( 2,1) (1, )−∞ − ∪ − ∪ ∞
2
2
2 7 4
( )
2
x x
f x
x x
+ −
=
+ −

23. The graph of a rational function NEVER
crosses a vertical asymptote. Why?
• Look at the last example:
Since the domain is , and the
vertical asymptotes are x = 2, -1, that means
that if the function crosses the vertical
asymptote, then for some y-value, x would
have to equal 2 or -1, which would make
the denominator = 0!
( , 1) ( 1,2) (2, )−∞ − ∪ − ∪ ∞
2
2
2 7 4
( )
2
x x
f x
x x
+ −
=
+ −

24. Examples
f x
x
( ) =
+
4
12
f x
x
x
( ) =
+
2
3 12
What similarities do you see between problems?
The degree of the denominator is larger
than the degree of the numerator.
Horizontal
Asymptote
at y = 0
Horizontal
Asymptote
at y = 0

25. Examples
h x
x
x
( ) =
+
+
2 1
1 82x
15x
g(x) 2
2
−
+
=
What similarities do you see between problems?
The degree of the numerator is the same as
the degree or the denominator.
Horizontal
Asymptote
at y = 2
Horizontal
Asymptote
at
5
2
y =

26. Examples
13x
54x5x3x
f(x)
23
+
−+−
=
2x
9x
g(x)
2
+
−
=
What similarities do you see between problems?
The degree of the numerator is larger than
the degree of the denominator.
No
Horizontal
Asymptote
No
Horizontal
Asymptote

27. Asymptotes: Summary
1. The graph of f has vertical asymptotes at the
_________ of q(x).
2. The graph of f has at most one horizontal
asymptote, as follows:
a) If n < d, then the ____________ is a
horizontal asymptote.
b) If n = d, then the line ____________ is a
horizontal asymptote (leading coef. over
leading coef.)
c) If n > d, then the graph of f has ______
horizontal asymptote.
zeros
line y = 0
no
a
y
b
=

28. You Do
Find all vertical and horizontal asymptotes
of the following function
( )
2 1
1
x
f x
x
+
=
+
Vertical Asymptote: x = -1
Horizontal Asymptote: y = 2

29. You Do Again
Find all vertical and horizontal asymptotes
of the following function
( ) 2
4
1
f x
x
=
+
Vertical Asymptote: none
Horizontal Asymptote: y = 0

30. Oblique/Slant Asymptotes
The graph of a rational function has a
slant asymptote if the degree of the
numerator is exactly one more than the
degree of the denominator. Long division
is used to find slant asymptotes.
The only time you have an oblique
asymptote is when there is no horizontal
asymptote. You cannot have both.
When doing long division, we do not care
about the remainder.

31. Example
Find all asymptotes.
( )
2
2
1
x x
f x
x
− −
=
−
Vertical
x = 1
Horizontal
none
Slant
( )
2
2
1 2
-2
x
x x x
x x
− − −
− −
y = x

32. Example
• Find all asymptotes:
2
2
( )
1
x
f x
x
−
=
−
Vertical asymptote
at x = 1
n > d by exactly one, so
no horizontal
asymptote, but there is
an oblique asymptote.
( )
2
2
1
1 2
2
( 1)
1
-
x
x x
x x
x
x
+
− −
−
−
− −
−
y = x + 1