Partial Fraction Decompositions

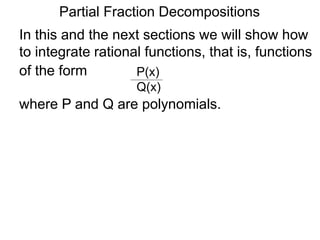
1. Partial Fraction Decompositions
In this and the next sections we will show how
to integrate rational functions, that is, functions
of the form P(x)
Q(x)
where P and Q are polynomials.

2. Partial Fraction Decompositions
We assume the rational functions are reduced.
In this and the next sections we will show how
to integrate rational functions, that is, functions
of the form P(x)
Q(x)
where P and Q are polynomials.

3. Partial Fraction Decompositions
We assume the rational functions are reduced.
Furthermore we assume that deg Q > deg P,
for if not, we can use long division to reduce
the problem to integrating such a function.
In this and the next sections we will show how
to integrate rational functions, that is, functions
of the form P(x)
Q(x)
where P and Q are polynomials.

4. Partial Fraction Decompositions
x3
x2 + x + 1
=
By long division

5. Partial Fraction Decompositions
Example:
x3
x2 + x + 1
=
1
x – 1 +
By long division
x2 + x + 1

6. Partial Fraction Decompositions
Example:
x3
x2 + x + 1
=
1
x – 1 +
By long division
So finding the integral of
x2 + x + 1
x3
x2 + x + 1
is reduced to
finding the integral of .
1
x2 + x + 1

7. Partial Fraction Decompositions
Example:
x3
x2 + x + 1
=
1
x – 1 +
By long division
So finding the integral of
x2 + x + 1
x3
x2 + x + 1
is reduced to
finding the integral of .
1
x2 + x + 1
To integrate P/Q, we break it down as the sum
of smaller rational formulas.

8. Partial Fraction Decompositions
Example:
x3
x2 + x + 1
=
1
x – 1 +
By long division
So finding the integral of
x2 + x + 1
x3
x2 + x + 1
is reduced to
finding the integral of .
1
x2 + x + 1
To integrate P/Q, we break it down as the sum
of smaller rational formulas.
This is called the partial fraction decomposition
of P/Q.

9. Partial Fraction Decompositions
Example:
x3
x2 + x + 1
=
1
x – 1 +
By long division
So finding the integral of
x2 + x + 1
x3
x2 + x + 1
is reduced to
finding the integral of .
1
x2 + x + 1
To integrate P/Q, we break it down as the sum
of smaller rational formulas.
This is called the partial fraction decomposition
of P/Q.
This decomposition is unique.

10. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.

11. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)

12. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2

13. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)

14. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)
= x2(x2 – 1)(x2 + 1)

15. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)
= x2(x2 – 1)(x2 + 1)
= x2(x – 1)(x + 1)(x2 + 1)

16. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)
= x2(x2 – 1)(x2 + 1)
= x2(x – 1)(x + 1)(x2 + 1)
b. x6 – 1

17. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)
= x2(x2 – 1)(x2 + 1)
= x2(x – 1)(x + 1)(x2 + 1)
b. x6 – 1 = (x3 – 1)(x3 + 1)

18. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)
= x2(x2 – 1)(x2 + 1)
= x2(x – 1)(x + 1)(x2 + 1)
b. x6 – 1 = (x3 – 1)(x3 + 1)
= (x – 1)(x2 + x + 1)(x + 1)(x2 – x + 1)

19. Partial Fraction Decompositions
Fundamental Theorem of Algebra:
Given any real (coefficient) polynomial Q(x),
it can be written as the product of
1st degree and irreducible 2nd degree factors.
Example:
Factor completely (over real numbers)
a. x6 – x2 = x2(x4 – 1)
= x2(x2 – 1)(x2 + 1)
= x2(x – 1)(x + 1)(x2 + 1)
b. x6 – 1 = (x3 – 1)(x3 + 1)
= (x – 1)(x2 + x + 1)(x + 1)(x2 – x + 1)
c. x2 – 2 = (x – 2)(x + 2)

20. Partial Fraction Decompositions
Reminder: To check if ax2 + bx + c is reducible,
check b2 – 4ac.

21. Partial Fraction Decompositions
Reminder: To check if ax2 + bx + c is reducible,
check b2 – 4ac.
If b2 – 4ac > 0, it is reducible.

22. Partial Fraction Decompositions
Reminder: To check if ax2 + bx + c is reducible,
check b2 – 4ac.
If b2 – 4ac > 0, it is reducible.
If b2 – 4ac < 0, it is irreducible.

23. Partial Fraction Decompositions
Reminder: To check if ax2 + bx + c is reducible,
check b2 – 4ac.
If b2 – 4ac > 0, it is reducible.
If b2 – 4ac < 0, it is irreducible.
Partial Fraction Decomposition Theorem:

24. Partial Fraction Decompositions
Reminder: To check if ax2 + bx + c is reducible,
check b2 – 4ac.
If b2 – 4ac > 0, it is reducible.
If b2 – 4ac < 0, it is irreducible.
Partial Fraction Decomposition Theorem:
Given P/Q where deg P < deg Q, then
P/Q = F1 + F2 + .. + Fn where
Fi = or(ax + b)k (ax2 + bx + c)k
(Ai and Bi are numbers)Aix + BiAi

25. Partial Fraction Decompositions
Reminder: To check if ax2 + bx + c is reducible,
check b2 – 4ac.
If b2 – 4ac > 0, it is reducible.
If b2 – 4ac < 0, it is irreducible.
Partial Fraction Decomposition Theorem:
Given P/Q where deg P < deg Q, then
P/Q = F1 + F2 + .. + Fn where
Fi = orAi
(ax + b)k
Aix + Bi
(ax2 + bx + c)k
and that (ax + b)k or (ax2 + bx + c)k are
factors in the factorization of Q(x).
(Ai and Bi are numbers)

26. Partial Fraction Decompositions
Example: a. For
P(x)
(x + 2)(x – 3)

27. Partial Fraction Decompositions
Example: a. For
P(x)
(x + 2)(x – 3)
has two linear factors (x + 2), (x – 3),
the denominator

28. Partial Fraction Decompositions
Example: a. For
P(x)
(x + 2)(x – 3)
has two linear factors (x + 2), (x – 3), therefore
P(x)
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
the denominator

29. Partial Fraction Decompositions
Example: a. For
P(x)
(x + 2)(x – 3)
has two linear factors (x + 2), (x – 3), therefore
P(x)
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
b. For P(x)
(x + 2)(x – 3)3
viewed having two factors (x + 2), (x – 3)3,
the denominator
, the denominator may be

30. Partial Fraction Decompositions
Example: a. For
P(x)
(x + 2)(x – 3)
has two linear factors (x + 2), (x – 3), therefore
P(x)
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
b. For P(x)
(x + 2)(x – 3)3
viewed having two factors (x + 2), (x – 3)3, hence
the denominators in the decomposition are
(x + 2), (x – 3), (x – 3)2, (x – 3)3 and
the denominator
, the denominator may be

31. Partial Fraction Decompositions
Example: a. For
P(x)
(x + 2)(x – 3)
has two linear factors (x + 2), (x – 3), therefore
P(x)
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
b. For P(x)
(x + 2)(x – 3)3
viewed having two factors (x + 2), (x – 3)3, hence
the denominators in the decomposition are
(x + 2), (x – 3), (x – 3)2, (x – 3)3 and
P(x)
(x + 2)(x – 3)3 = A
(x + 2)
+ B
(x – 3)
the denominator
, the denominator may be
+ C
(x – 3)2
+ D
(x – 3)3

32. Partial Fraction Decompositions
c. For
P(x)
(x + 2)(x2 + 3)
linear factor (x + 2) and a 2nd degree irreducible
factor (x2 + 3),
the denominator has one

33. Partial Fraction Decompositions
c. For
P(x)
(x + 2)(x2 + 3)
linear factor (x + 2) and a 2nd degree irreducible
factor (x2 + 3), hence
P(x)
(x + 2)(x2 + 3) =
A
(x + 2)
+
Bx + C
(x2 + 3)
the denominator has one

34. Partial Fraction Decompositions
c. For
P(x)
(x + 2)(x2 + 3)
linear factor (x + 2) and a 2nd degree irreducible
factor (x2 + 3), hence
P(x)
(x + 2)(x2 + 3) =
A
(x + 2)
+
Bx + C
(x2 + 3)
d. For P(x)
(x + 2)2(x2 + 3)2
viewed having two factors (x + 2)2, (x2 + 3)2,
the denominator has one
, the denominator may be

35. Partial Fraction Decompositions
c. For
P(x)
(x + 2)(x2 + 3)
linear factor (x + 2) and a 2nd degree irreducible
factor (x2 + 3), hence
P(x)
(x + 2)(x2 + 3) =
A
(x + 2)
+
Bx + C
(x2 + 3)
d. For P(x)
(x + 2)2(x2 + 3)2
viewed having two factors (x + 2)2, (x2 + 3)2, hence
the denominators in the decomposition are
(x + 2), (x + 2)2 , (x2 + 3), (x2 + 3)2, hence
the denominator has one
, the denominator may be

36. Partial Fraction Decompositions
c. For
P(x)
(x + 2)(x2 + 3)
linear factor (x + 2) and a 2nd degree irreducible
factor (x2 + 3), hence
P(x)
(x + 2)(x2 + 3) =
A
(x + 2)
+
Bx + C
(x2 + 3)
d. For P(x)
(x + 2)2(x2 + 3)2
viewed having two factors (x + 2)2, (x2 + 3)2, hence
the denominators in the decomposition are
(x + 2), (x + 2)2 , (x2 + 3), (x2 + 3)2, hence
= A
(x + 2)
+ B
(x + 2)2
the denominator has one
, the denominator may be
+ Cx + D
(x2 + 3)
+ Ex + F
(x2 + 3)2
P(x)
(x + 2)2(x2 + 3)2

37. Partial Fraction Decompositions
To find the exact decomposition, we use two
methods:

38. Partial Fraction Decompositions
To find the exact decomposition, we use two
methods:
1. by evaluation with the roots of the LCD

39. Partial Fraction Decompositions
To find the exact decomposition, we use two
methods:
1. by evaluation with the roots of the LCD
2. use the answers from 1, expand and match
coefficients.

40. Partial Fraction Decompositions
To find the exact decomposition, we use two
methods:
1. by evaluation with the roots of the LCD
2. use the answers from 1, expand and match
coefficients.
Example A. Find the partial fraction
decomposition of
2x +3
(x + 2)(x – 3)

41. Partial Fraction Decompositions
We know that
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
To find the exact decomposition, we use two
methods:
1. by evaluation with the roots of the LCD
2. use the answers from 1, expand and match
coefficients.
2x +3
Example A. Find the partial fraction
decomposition of
2x +3
(x + 2)(x – 3)

42. Partial Fraction Decompositions
We know that
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
To find the exact decomposition, we use two
methods:
1. by evaluation with the roots of the LCD
2. use the answers from 1, expand and match
coefficients.
2x +3
Clear the denominator, multiply it by (x + 2)(x – 3)
Example A. Find the partial fraction
decomposition of
2x +3
(x + 2)(x – 3)

43. Partial Fraction Decompositions
Example A. Find the partial fraction
decomposition of
We know that
(x + 2)(x – 3) =
A
(x + 2)
+
B
(x – 3 )
To find the exact decomposition, we use two
methods:
1. by evaluation with the roots of the LCD
2. use the answers from 1, expand and match
coefficients.
2x +3
(x + 2)(x – 3)
2x +3
Clear the denominator, multiply it by (x + 2)(x – 3)
We have 2x + 3 = A(x – 3) + B(x + 2)

44. Partial Fraction Decompositions
The factors (x – 3), (x + 2) have roots at x = 3, x = -2

45. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,

46. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,
We have 9 = 0 + 5B

47. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,
or B = 9/5
We have 9 = 0 + 5B

48. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,
Evaluate x = -2,
or B = 9/5
2x + 3 = A(x – 3) + B(x + 2)
We have 9 = 0 + 5B

49. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,
Evaluate x = -2,
or B = 9/5
2x + 3 = A(x – 3) + B(x + 2)
We have -1 = -5A + 0
We have 9 = 0 + 5B

50. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,
Evaluate x = -2,
or B = 9/5
2x + 3 = A(x – 3) + B(x + 2)
or A = 1/5
We have 9 = 0 + 5B
We have -1 = -5A + 0

51. Partial Fraction Decompositions
2x + 3 = A(x – 3) + B(x + 2)
The factors (x – 3), (x + 2) have roots at x = 3, x = -2
Evaluate at x = 3,
Evaluate x = -2,
or B = 9/5
2x + 3 = A(x – 3) + B(x + 2)
or A = 1/5
Hence
(x + 2)(x – 3) =
1/5
(x + 2)
+
9/5
(x – 3 ) .
2x +3
We have 9 = 0 + 5B
We have -1 = -5A + 0

52. Partial Fraction Decompositions
Example B. Find the partial fraction
decomposition of
1
(x – 2)(x – 3)3.

53. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
We know that
1
1
(x – 2)(x – 3)3.
Example B. Find the partial fraction
decomposition of

54. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
We know that
Clear the denominator, We have
1
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)
1
(x – 2)(x – 3)3.
Example B. Find the partial fraction
decomposition of

55. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
1
(x – 2)(x – 3)3.
We know that
Clear the denominator, We have
1
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)
Evaluate at x = 3,
Example B. Find the partial fraction
decomposition of

56. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
1
(x – 2)(x – 3)3.
We know that
Clear the denominator, We have
1
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)
Evaluate at x = 3, we have 1 = D.
Example B. Find the partial fraction
decomposition of

57. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
We know that
Clear the denominator, We have
1
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)
Evaluate at x = 3, we have 1 = D.
Evaluate at x = 2, we have -1= A.
1
(x – 2)(x – 3)3.
Example B. Find the partial fraction
decomposition of

58. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
We know that
Clear the denominator, We have
1
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)
Evaluate at x = 3, we have 1 = D.
Evaluate at x = 2, we have -1= A.
These are the only roots we can use to evaluate.
1
(x – 2)(x – 3)3.
Example B. Find the partial fraction
decomposition of

59. Partial Fraction Decompositions
(x – 2)(x – 3)3 = A
(x – 2)
+ B
(x – 3)
+ C
(x – 3)2
+ D
(x – 3)3
We know that
Clear the denominator, We have
1
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)
Evaluate at x = 3, we have 1 = D.
Evaluate at x = 2, we have -1= A.
These are the only roots we can use to evaluate.
For B and C, we use the “method of coefficient–matching”.
1
(x – 2)(x – 3)3.
Example B. Find the partial fraction
decomposition of

60. Partial Fraction Decompositions
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve

61. Partial Fraction Decompositions
Put A = -1 and D = 1 into the equation and expand.
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve

62. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
Put A = -1 and D = 1 into the equation and expand.
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve

63. Partial Fraction Decompositions
P - 2,23,33,113
Panels 64,66 and 71 have algebra errors
but the results are surprisingly not affected

64. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
Put A = -1 and D = 1 into the equation and expand.
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve

65. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
Put A = -1 and D = 1 into the equation and expand.
move all the explicit terms to one side
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

66. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
Put A = -1 and D = 1 into the equation and expand.
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

67. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

68. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

69. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
Hence B = 1,
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

70. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
Hence B = 1, put this back into the equation, we have
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

71. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
Hence B = 1, put this back into the equation, we have
x3 – 9x2 + 26x – 24 = 1 (x – 2)(x – 3)2+ C(x – 2)(x – 3)
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

72. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
Hence B = 1, put this back into the equation, we have
Match the constant terms from both sides
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = 1 (x – 2)(x – 3)2+ C(x – 2)(x – 3)
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

73. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
Hence B = 1, put this back into the equation, we have
Match the constant terms from both sides
…… – 24 = ….. – 18 + Cx2 – 5Cx + 6C
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = 1 (x – 2)(x – 3)2+ C(x – 2)(x – 3)
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

74. Partial Fraction Decompositions
1 = (-1)(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+(1)(x – 2)
match the highest degree term from both sides
Put A = -1 and D = 1 into the equation and expand.
x3 + …. = Bx3 + …..
Hence B = 1, put this back into the equation, we have
Match the constant terms from both sides
…… – 24 = ….. – 18 + Cx2 – 5Cx + 6C
We have -24 = -18 + 6C  -1 = C
1 = A(x – 3)3+ B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+D(x – 2)We’ve
x3 – 9x2 + 26x – 24 = 1 (x – 2)(x – 3)2+ C(x – 2)(x – 3)
x3 – 9x2 + 26x – 24 = B(x – 2)(x – 3)2+ C(x – 2)(x – 3)
move all the explicit terms to one side
1 = –x3 +9x2 – 27x + 27 + B(x – 2)(x – 3)2+ C(x – 2)(x – 3)+x – 2

75. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1

76. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1
(x + 2)(x2 + 1)
Example C. Find the decomposition of
1 – 2x

77. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1
We have
1 – 2x
(x + 2)(x2 + 1) =
A
(x + 2)
+
Bx + C
(x2 + 1)
(x + 2)(x2 + 1)
Example C. Find the decomposition of
1 – 2x

78. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1
We have
1 – 2x
(x + 2)(x2 + 1) =
A
(x + 2)
+
Bx + C
(x2 + 1)
Clear the denominator, we've
1 – 2x = A(x2 + 1) + (Bx + C)(x + 2)
(x + 2)(x2 + 1)
Example C. Find the decomposition of
1 – 2x

79. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1
We have
1 – 2x
(x + 2)(x2 + 1) =
A
(x + 2)
+
Bx + C
(x2 + 1)
Clear the denominator, we've
1 – 2x = A(x2 + 1) + (Bx + C)(x + 2)
Evaluate at x = -2,
(x + 2)(x2 + 1)
Example C. Find the decomposition of
1 – 2x

80. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1
We have
1 – 2x
(x + 2)(x2 + 1) =
A
(x + 2)
+
Bx + C
(x2 + 1)
Clear the denominator, we've
1 – 2x = A(x2 + 1) + (Bx + C)(x + 2)
Evaluate at x = -2, We have 5 = 5A or A = 1.
(x + 2)(x2 + 1)
Example C. Find the decomposition of
1 – 2x

81. Partial Fraction Decompositions
(x – 2)(x – 3)3 = -1
(x – 2)
+ 1
(x – 3)
+ -1
(x – 3)2
+ 1
(x – 3)3.
Therefore
1
(x + 2)(x2 + 1)
We have
1 – 2x
(x + 2)(x2 + 1) =
A
(x + 2)
+
Bx + C
(x2 + 1)
Example C. Find the decomposition of
Clear the denominator, we've
1 – 2x = A(x2 + 1) + (Bx + C)(x + 2)
Evaluate at x = -2, We have 5 = 5A or A = 1.
So we've 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x

82. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)

83. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C

84. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1

85. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms,

86. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.

87. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.
Hence B = -1

88. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.
Hence B = -1 and that 1 – 2x = -2x + Cx + 2C + 1

89. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.
Hence B = -1 and that 1 – 2x = -2x + Cx + 2C + 1
Compare the constant terms

90. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.
Hence B = -1 and that 1 – 2x = -2x + Cx + 2C + 1
Compare the constant terms
1 = 2C + 1

91. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.
Hence B = -1 and that 1 – 2x = -2x + Cx + 2C + 1
Compare the constant terms
1 = 2C + 1  C = 0.

92. Partial Fraction Decompositions
Expand 1 – 2x = x2 + 1 + (Bx + C)(x + 2)
1 – 2x = x2 + 1 + Bx2 + 2Bx + Cx + 2C
1 – 2x = Bx2 + x2 + 2Bx + Cx + 2C + 1
Compare the square terms, we've Bx2 + x2 = 0.
Hence B = -1 and that 1 – 2x = -2x + Cx + 2C + 1
Compare the constant terms
1 – 2x
(x + 2)(x2 + 1) =
1
(x + 2)
+
-x
(x2 + 1).
Therefore
1 = 2C + 1  C = 0.

93. Partial Fraction Decompositions
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
Example D. Find the decomposition of
1
x(1 – x)(1 + x)

94. Partial Fraction Decompositions
x(1 – x) (1 + x) =
A
x
+
B
(1 – x)
+
The decomposition has the form
1
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 + x)
Example D. Find the decomposition of
1
x(1 – x)(1 + x)

95. Partial Fraction Decompositions
x(1 – x) (1 + x) =
A
x
+
B
(1 – x)
+
The decomposition has the form
1
1 = A(1 – x) (1 + x) + Bx(1 + x) + Cx(1 – x)
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 + x)
Clearing the denominator, we have
Example D. Find the decomposition of
1
x(1 – x)(1 + x)

96. Partial Fraction Decompositions
x(1 – x) (1 + x) =
A
x
+
B
(1 – x)
+
The decomposition has the form
1
1 = A(1 – x) (1 + x) + Bx(1 + x) + Cx(1 – x)
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 + x)
Clearing the denominator, we have
Set x = 0, 1, –1, we obtain that
A = 1, B = 1/2, C = –1/2 respectively,
Example D. Find the decomposition of
1
x(1 – x)(1 + x)

97. Partial Fraction Decompositions
x(1 – x) (1 + x) =
A
x
+
B
(1 – x)
+
The decomposition has the form
1
1 = A(1 – x) (1 + x) + Bx(1 + x) + Cx(1 – x)
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 + x)
Clearing the denominator, we have
Set x = 0, 1, –1, we obtain that
A = 1, B = 1/2, C = –1/2 respectively, hence
x(1 – x) (1 + x)
=
1
x
+ 1/2
(1 – x)
–1 1/2
(1 + x)
Example D. Find the decomposition of
1
x(1 – x)(1 + x)

98. Partial Fraction Decompositions
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
Example E. Find the decomposition of
1
x(1 – x)2(1 + x)2

99. Partial Fraction Decompositions
x(1 – x)2(1 + x)2 =
A
x
+
B
(1 – x)
+
D
The decomposition has the form
1
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 – x)2 +
(1 + x)
+
E
(1 + x)2
Example E. Find the decomposition of
1
x(1 – x)2(1 + x)2

100. Partial Fraction Decompositions
x(1 – x)2(1 + x)2 =
A
x
+
B
(1 – x)
+
D
The decomposition has the form
1
1 = A(1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + Cx(1 + x)2
+ Dx(1 – x)2(1 + x) + Ex(1 – x)2
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 – x)2 +
(1 + x)
+
E
(1 + x)2
Clear the denominator, we have
Example E. Find the decomposition of
1
x(1 – x)2(1 + x)2

101. Partial Fraction Decompositions
x(1 – x)2(1 + x)2 =
A
x
+
B
(1 – x)
+
D
The decomposition has the form
1
1 = A(1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + Cx(1 + x)2
+ Dx(1 – x)2(1 + x) + Ex(1 – x)2
The fractional form 1
xK(1 – x)M(1 + x)M.
appears in the integration of the quotients of
powers of sine and cosine.
C
(1 – x)2 +
(1 + x)
+
E
(1 + x)2
Clear the denominator, we have
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Example E. Find the decomposition of
1
x(1 – x)2(1 + x)2

102. Partial Fraction Decompositions
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2

103. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2

104. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
= (1 – x2)2 + ¼ x[ (1 + x)2 – (1 – x)2]
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2

105. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
= (1 – x2)2 + ¼ x[ (1 + x)2 – (1 – x)2]
= 1 – 2x2 + x4 + ¼ x[(1 + x) – (1 – x)] [(1 + x) + (1 – x)]
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2

106. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
= (1 – x2)2 + ¼ x[ (1 + x)2 – (1 – x)2]
= 1 – 2x2 + x4 + ¼ x[(1 + x) – (1 – x)] [(1 + x) + (1 – x)]
= 1 – 2x2 + x4 + ¼ x[2x][2]
= 1 – x2 + x4
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2

107. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
= (1 – x2)2 + ¼ x[ (1 + x)2 – (1 – x)2]
= 1 – 2x2 + x4 + ¼ x[(1 + x) – (1 – x)] [(1 + x) + (1 – x)]
= 1 – 2x2 + x4 + ¼ x[2x][2]
= 1 – x2 + x4
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2
Hence,
1 = 1 – x2 + x4 + Bx(1 – x)(1 + x)2 + Dx(1 – x)2(1 + x)

108. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
= (1 – x2)2 + ¼ x[ (1 + x)2 – (1 – x)2]
= 1 – 2x2 + x4 + ¼ x[(1 + x) – (1 – x)] [(1 + x) + (1 – x)]
= 1 – 2x2 + x4 + ¼ x[2x][2]
= 1 – x2 + x4
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2
Hence,
1 = 1 – x2 + x4 + Bx(1 – x)(1 + x)2 + Dx(1 – x)2(1 + x) or that
x2 – x4 = Bx(1 – x)(1 + x)2 + Dx(1 – x)2(1 + x)

109. Partial Fraction Decompositions
(1 – x)2(1 + x)2 + ¼ x(1 + x)2 – ¼ x(1 – x)2
= (1 – x2)2 + ¼ x[ (1 + x)2 – (1 – x)2]
= 1 – 2x2 + x4 + ¼ x[(1 + x) – (1 – x)] [(1 + x) + (1 – x)]
= 1 – 2x2 + x4 + ¼ x[2x][2]
= 1 – x2 + x4
Expand the known parts by grouping them first:
Set x = 0, 1, –1, we obtain A = 1, C = 1/4, E = –1/4.
Hence
1 = (1 – x)2(1 + x)2 + Bx(1 – x)(1 + x)2 + ¼ x(1 + x)2
+ Dx(1 – x)2(1 + x) – ¼ x(1 – x)2
Hence,
1 = 1 – x2 + x4 + Bx(1 – x)(1 + x)2 + Dx(1 – x)2(1 + x) or that
x2 – x4 = Bx(1 – x)(1 + x)2 + Dx(1 – x)2(1 + x)
Compare the linear terms, we have 0 = Bx + Dx
so that D = –B

110. Partial Fraction Decompositions
Hence
x2 – x4 = Bx(1 – x)(1 + x)2 – Bx(1 – x)2(1 + x)

111. Partial Fraction Decompositions
Hence
x2 – x4 = Bx(1 – x)(1 + x)2 – Bx(1 – x)2(1 + x)
Compare the x4 terms, we have –x4 = –Bx4 – Bx4
so that B = 1/2, and D = –1/2.

112. Partial Fraction Decompositions
Hence
x2 – x4 = Bx(1 – x)(1 + x)2 – Bx(1 – x)2(1 + x)
Compare the x4 terms, we have –x4 = –Bx4 – Bx4
so that B = 1/2, and D = –1/2.
x(1 – x)2(1 + x)2 =
1
x +
1/2
(1 – x) +
1/2
Therefore
1 1/4
(1 – x)2 (1 + x) –
1/4
(1 + x)2–

113. Partial Fraction Decompositions
Hence
x2 – x4 = Bx(1 – x)(1 + x)2 – Bx(1 – x)2(1 + x)
Compare the x4 terms, we have –x4 = –Bx4 – Bx4
so that B = 1/2, and D = –1/2.
x(1 – x)2(1 + x)2 =
1
x +
1/2
(1 – x) +
1/2
Therefore
1 1/4
(1 – x)2 (1 + x) –
1/4
(1 + x)2–
In light of the last two examples, what do you think
is the decomposition of the rational forms:
1
x(1 – x)M(1 + x)M