This is a lecture is a series on combustion chemical kinetics for engineers. The course topics are selections from thermodynamics and kinetics especially geared to the interests of engineers involved in combusition

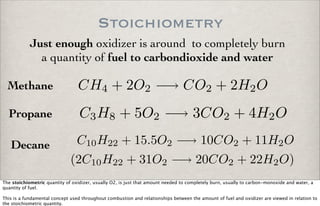
1. Stoichiometry
Just enough oxidizer is around to completely burn
a quantity of fuel to carbondioxide and water
CH4 + 2O2 −→ CO2 + 2H2O
C3H8 + 5O2 −→ 3CO2 + 4H2O
C10H22 + 15.5O2 −→ 10CO2 + 11H2O
(2C10H22 + 31O2 −→ 20CO2 + 22H2O)
Methane
Propane
Decane
The stoichiometric quantity of oxidizer, usually O2, is just that amount needed to completely burn, usually to carbon-monoxide and water, a
quantity of fuel.
This is a fundamental concept used throughout combustion and relationships between the amount of fuel and oxidizer are viewed in relation to
the stoichiometric quantity.

2. Stoichiometry
CH3OH + 1.5O2 −→ CO2 + 2H2O
Methanol
Methyl Butanoate
(simple ester used to model ester biofuels)
CH3CO2C3H7 + 6.5O2 −→ 5CO2 + 5H2O
(2CH3CO2C3H7 + 13O2 −→ 10CO2 + 10H2O
The same even with oxygenated fuels
The same holds for even oxygenated compounds. In this case the oxygen comes not only from O2, but also from the fuel itself. But,
nevertheless, in the stoichiometric mixture, the atom amounts balance.

3. Balancing Hydrocarbon Equations
CnHmOp + xO2 −→ yCO2 + zH2O
•All the carbons atoms go to CO2
•All the hydrogens go to H2O
•Count the number of oxygens on the right hand side
•Subtract the number of oxygens in the fuel.
Balancing a chemical equation uses the fundamental fact that during a chemical reaction the number of atoms on each side of the equation stay
the same.
In balancing a combustion equation to CO2 and water, we have a special case which simplifies determining how much fuel, oxidizer and
products are needed to balance the equation.
Essentially one recognizes that all the carbon of the fuel goes to CO2, all the hydrogens go to H2O. Secondly, all the oxygens on the right hand
side, the product side, come from the fuel and what is left over comes from pure O2.

4. 90 RON in air
Balance the equation for the complete combustion of 90 RON
mixture of heptane/isooctane in air:
•90 RON mixture
•10% heptane
•90% isooctane
•The oxidizer is air (approximately just nitrogen and oxygen):
•79% Nitrogen
•21% Oxygen
(.90 C8H18 + . 0.1 C7H16) + x(0.79 N2 + 0.21 O2)
−→ y CO2 + z H2O + 0.79x N2
A typical problem is to find the stoichiometric quantities needed of a mixtures of hydrocarbons in air. In this case, we are not dealing with
simple integer numbers for the quantities in the balanced equations.
But, nevertheless, the steps to compute the balanced equation are the same. The quantities x,y,z are just not integers.

5. 90 RON in Air
•How many Carbons?
•Left Hand Side
•Carbons from Heptane + Carbons from isooctane
•Each multiplied by percentage due to RON
•(0.10)(7) + (0.90)(8) = 7.9
•Right Hand Side
•This is the number of CO2 molecules
•7.9
To actually balance the equation of our example, we start with computing the number of carbons. On the left hand side, the source of carbons
is the
90 RON primary reference fuel, meaning a mixture of 10% heptane and 90% isooctane. This means that there is an e!ect number of carbons of
7.9 (notice it is between 7 and 8 carbons).
This has to balance with the right hand side. But the only carbons on this side is the one carbon of CO2, so that means there are 7.9 CO2
molecules produced.

6. 90 RON in Air
•How many hydrogens?
•Left hand side
•(0.1) hydrogens in heptane + (0.90) hydrogens in isooctane
•(0.10)(16) + (0.90)(18) = 17.8
•Right hand side
•hydrogens in water
•17.8/2 = 8.9 waters
How many hydrogens are there?
Using the same logic, 10% of heptane and 90% of isooctane, means that 10% of the 16 hydrogens come from heptane and 90% of the 18
hydrogens come from isooctane. This means there are e!ectively 17.8 hydrogens in the fuel (once again notice that it is something between 17
and 18 hydrogens).
All the hydrogens on the right hand side are in water. There are two hydrogens per water, so there are 8.9 waters.

7. RON 90 in AIR
•How many oxygens on the left hand side?
•All in the air (no oxygens in the fuel)
•Right hand side
•oxygens in carbonmonoxide + oxygens in water
•(7.9)(2) + (8.9)(1) = 24.7
•Left hand side
•oxygens in air = oxygens in right hand side
•x (2)(0.21) = 24.7
•solving: x=58.8
Now we have to balance the oxygens whose only source in the reactants is in air. Starting with the right hand side (since now the molecule
amounts are not fixed) we add up the oxygens in both carbonmonoxide and water. This gives a total of 24.7 oxygens.
This means on the left hand side, that the oxygens in air have to total 24.7. Since oxygen molecules are only 21% of the mixture and there are
two oxygens per oxygen molecule, x times 2 times 0.21 has to equal 24.7. This means that there are 58.8 portions of air.

8. 90 RON in Air
Final Balanced Equation
(.90 C8H18 + . 0.1 C7H16) + 58.8(0.79 N2 + 0.21 O2)
−→ 7.9 CO2 + 8.9 H2O + 46.5 N2
So the final balanced equation looks like this.

9. Fuel-Air Ratio by Weight
From the previous slide, the fuel air ratio is:
1 mole fuel per 58.8 moles air
However, usually, a fuel air mixture is done by weight.
x grams of fuel per y grams of air.
Another quantity is the percent, by weight, of fuel needed.
This is easily computed from the air-fuel ratio.
From the balanced example, we saw that there was 1 mole of 90 RON fuel to 58.8 moles of air.
Since in the laboratory, the number of moles cannot be directly measured, it is often more convenient to use units that are directly measurable,
such as weight and pressure.
More often than not, in engineering and combustion papers and tables, fuel and air quantities are given in kilograms and pressure. Fortunately,
this is a simple conversion of units.
Fuel to air ratio, by weight is also a useful measure, representing a measurable percentage.

10. Air Fuel Ratio
•Molecule Weight
•Heptane: 100.2 g/mol
•Isooctane: 114.23 g/mol
•90 RON Fuel
•(0.1)(heptane) + (0.90)(isooctane)
•(0.10)(100.2) + (0.90)(114.23) = 112.8 g/mol
•Air
•(0.79)(Nitrogen) + (0.21)(oxygen)
•(0.79)(28) + (0.21)(32.0) = 28.8 g/mol
To convert from moles to grams, of course we need the molecular weight of the components of the fuel, namely heptane and isooctane.
To compute the e!ective molecular weight of the 90 RON fuel, we take 10% of the heptane and 90% of the isooctane molecular weight, giving
112.8 g/mol.
The same principle is used to compute the e!ective molecular weight of air, 79% nitrogen and 21% oxygen, giving 28.8 g/mol.

11. Air-Fuel Ratio
•Air-Fuel Ratio
• (58.8 mol air)(28.8 g/mol) = 1693 g
•(1 mol fuel)(112.82 g/mol) = 112.8 g
•(1693 g)/(112.8 g) = 15
•Percentage fuel by Weight
•(1 g fuel)/15 g air) = 0.066
•Percent: 6.6%
Using the parameters of the last example, we have 58.8 moles of air, which means we have 1693 grams of air, with 1 mole of fuel, giving 112.8
grams of fuel. This yields an air to fuel ratio of 15.
Another quantity used is percentage weight of the fuel. This is simply the inverse of the air-fuel ratio multiplied by 100.

12. Examples
Fuel By Weight By Volume
Percent
(weight)
Gasoline 14.7:1 - 6.8
Natural Gas 17.2 9.7:1 5.8
Propane (LP) 15.5:1 23.9:1 6.45
Ethanol 9:1 - 11.1
Methanol 6.4:1 - 15.6
Hydrogen 34:1 2.39:1 2.9
Diesel 14.6:1 - 6.8
Here is a table from the literature of the di!erent typical ratios for common fuels, gasoline, natural gas, propane, ethanol,
methanol, hydrogen and diesel.

13. Ethalpy
dh = (
δh
δT
)P dT + (
δh
δP
)T dP
dh = (
δh
δT
)P dT
∆H = HS0
+
! S1
S0
CP dT
For a constant pressure reaction:
Integrating both sides from state 0 and state 1:
Under constant pressure, the total di!erential of h(T,P) the dP term is zero. If the result is integrated between state 1 and state 2, using the
expression for Cp, then the expression for the change in enthalpy between state 1 and state 2 is derived.

14. Heat of Formation
Definition:
Enthalpy (Heat) of Formation
The reference state of the elemental structures at
Room Temperature and Pressure
(298 Kelvin, 1 atm)
The following have zero enthalpy of formation:
H2, O2, C(graphite), N2
As a consequence of the first law of thermodynamics, we do not have to deal with absolute energy values such as the total energy of a
molecule, but relative energy values. In addition, since the first law says we only have to deal with the ‘before’ and ‘after’ states, we can pick
an arbitrarily convenient reference state and compare all values relative to it and even not worry about how we got there. In fact, whether the
actual process goes through this state is irrelevant.
Heat of formation uses the individual atoms as the base reference state at a standard temperature and pressure, 298 Kelvin and 1 atmosphere.
These individual atoms are defined to have a heat of formation of zero. In computing the enthalpy change between state 1 and state 2, we will
take a detour through this reference state.

15. Heat of Combustion
!"#$%&'%(&)*+,$-&.
(/0%1!2324567%%%%%%0%!0/%%1!0864937
1!:;04087
(!8%1!984:57%%%%%/0%1;7
!"#$%&'%<&=)#$-&.
&'%$>"%?"#@$#.$,
1984:57
!"#$%&'%<&=)#$-&.
&'%$>"%A=&B+@$,
1!:994;37
(1C=#D>-$"7%%%%%0%!0%%%%%%0%/0
Consequence of
the first law of thermodynamics
(a computable path is chosen)
Once again, as a consequence of the first law of thermodynamics, we can use heats of formation to compute the heat of combustion.
Essentially, we create a fictitious energy path. We start with the reactant molecules and break them apart into individual atoms. The energy
needed to do this is the heat of formation. We then recombine these atoms into the product molecules, and, once again the energy needed is
the heat of formation.
Obviously, the actual path in going from reactants to products does not follow this path, but using the first law of thermodynamics, we can use
are reference states for the computation.

16. Heating Value
•Higher Heating Value
•Thermodynamic heat of combustion
•Enthalpy change for the reaction with the same temperature
before and after combustion.
•Lower Heating Value
•Heat of Vaporization subtracted from higher heating value
•water component is in a vapor state after combustion
Heat released when a given amount of fuel is combusted.
A standard measurement used in industry for the heat content of a species, meaning how much heat is released when the species is
combusted. Two values are used.
The first is higher heating value. This is the thermodynamic heat of combustion, meaning the enthalpy change of combustion at a constant
temperature.
The second is the lower heating value. This is where the heat of vaporization is subtracted from the higher heating value.

17. Heating Value Relationship
hvapor,H2O(nH2O,out/nH2O,in)
Higher Heating Value =
Lower Heating Value +
The lower heating value is useful for boilers
where in the end the water is evaporators
The di!erence between the higher and the lower heating value is basically related to the heat of vaporization of water. It is for this reason that
the lower heating value is useful for boilers.

18. Lower Heating Value: Fuels
Small hydrocarbons:
46 to 50 MJ/kg
Higher hydrocarbons:
around 50 MJ/kg
Hydrogen:
120 MJ/kg
Carbon Monoxide
10.112 MJ/kg
Esters
30-40 MJ/kg
Alcohols
18-30 MJ/kh
This is a general comparison of the heating values of various groups of fuels. One notable fact is that the hydrocarbons have a much higher
heating value than the typical biofuels, such as alcohol or esters (which are derived from, for example, rapseed oil). It is also noteworthy that
hydrogen has the highest heating value.

19. Hydrocarbons
Methane
Ethane
Propane
Butane
Pentane
Hexane
Heptane
Octane
Nonane
Decane
Undecane
Dodecane
43 44 45 46 47 48 49 50
44.147
44.194
44.24
44.311
44.427
44.566
44.752
45.357
45.752
46.357
47.794
50.009
Lower Heating Value
These are the lower heating values of a series of hydrocarbon fuels, increasing in number of carbons. Methane has the highest
heating value among them.

20. Esters
Methoxymethane
Ethoxyethane
Propoxypropane
Butoxybutane
25 28 31 34 37 40
37.798
36.355
33.867
28.783
Lower Heating Value
Among the esters, those fuels similar to rapseed (or other) oils for biofuels, the larger esters have a higher heating value.

21. Ethanols
Methanol
Ethanol
n-propanol
Isopropanol
18 20 22 24 26 28 30 32
30.447
30.68
28.865
19.937
Lower Heating Value
Another class of biofuels are the ethanols. Other than methanol, these have a heating value of around 30 MJ/kg.

22. Temperature Dependence
But no heat release
Temperature Rise
But the temperature stays constant
Heat Lost to the Environment
Enthalpy
Temperature
Constant
Temperature
Process
Adiabatic Process
Reactants
Products
Heat Loss
And Temperature Gain
The enthalpy of a species (or set of species) is a function of temperature. For example, the total enthalpy of a set of reactants is a function of
temperature as is the total enthalpy of a set of products. This means that to find the gain or loss of energy to the system we need to know the
beginning and ending temperatures. It can also be seen in this figure that, for example, an adiabatic process, meaning that there is no change
in energy from reactants to products, there is an increase in temperature. The energy is not lost to the environment, but given to the products
in raising their temperature. We will see in the next slides that this is defined as the adiabatic flame temperature.

23. Adiabatic Flame temperature
Enthalpy
Temperature
Reactants Products
Constant Enthalpy (Adiabatic)
Adiabatic Flame Temperature
Adiabatic Flame Temperature is important to combustion because it represents the highest possible temperature a given combustion process
can achieve. It provides another tool to compare fuels.
The term adiabatic is that no heat is lost to the environment, i.e. the enthalpy of the system stays constant. The energy produced by the
reaction is put into raising the temperature of the products. Of course, no process is perfect and not all of the heat can be converted to
temperature, so this represents the highest theoretical limit. But it gives a good idea of the potential of a fuel.

24. Calculation
CH4 + 2(O2 + 3.76N2) −→ CO2 + H2O + 7.52N2
Complete Combustion of Methane
Hreact =
!
react
Nihi,298 = Hproducts =
!
prod
Njhj,T
Adiabatic Flame Temperature
The adiabatic flame temperature is solved for by setting the heat of combustion of the reactants equal to the heat of combustion of the
products. The term adiabatic means there is no loss of heat. This means that all the heat in the reactants goes into the products.
For example, let us look at the complete combustion of methane in air.

25. Enthalpies
Hreact,298 = hch4 + 2ho2 + hn2
Hprod,T (hco2,298 + CP,co2(T − 298))
(hh2o,298 + CP,h2o(T − 298))
(hn2,298 + CP,n2(T − 298))
=
(assume constant heat capacity)
match and solve for T
The temperature of the heat of combustion of the reactants is know, namely the standard temperature of 298, so the standard enthalpy of each
of reactants can be used. The enthalpy of the reactants is their sum.
However, the temperature of the products, i.e. the adiabatic flame temperature, is not known and has to be solved for. The main problem is
that the heat capacity is also a function of temperature. However, if we assume an average heat capacity, then the we have one linear equation
and one unknown, T.

26. Examples
hi,298 Cp,1200
CH4
CO2
H2O
N2
O2
Species
-74.831 ---
-393.546 56.21
-241.845 43.87
0 33.71
0 ----
The standard heats of formation, one atm and 298K, for both the reactants and products can be looked up. Since the heat capacity changes
with temperature, we make an assumption and choose the heat capacity at a temperature somewhere between 298 and the expected flame
temperature. In this case we choose 1200K. We these values the flame temperature can be solved for.

27. Examples
Fuel in air in oxygen
Methane 1957 2810
Ethane 1960
Propane 1980 2020
Butane 1970
Hydrogen 2045 2660
Acetylene 2400 3100
Here are some examples of the adiabatic flame temperature both in air and in pure oxygen for the stoichiometric full complete combustion.
The adiabatic flame temperature in air is always lower than that in pure oxygen because even though the stoichiometry between the fuel and
oxygen is the same in both cases, in air not only do the combustion products have to be heated up but also the inert nitrogen.

28. Equivalence Ratio
The adiabatic flame temperature peaks around equivalence ratio of 1.0. In one sense that is where the most e!cient stoichiometric combustion
occurs.
However, closer examination is that the peak is a bit to the rich side. The accepted explanation for this is disassoication e"ect. At higher
temperatures, the equilibrium, particularly CO2 is shifted toward its disassociation products, for example CO. This is an example of the fact
that incomplete combustion can always occur, we do not always have the ideal case.