COORDINATE GEOMETRY

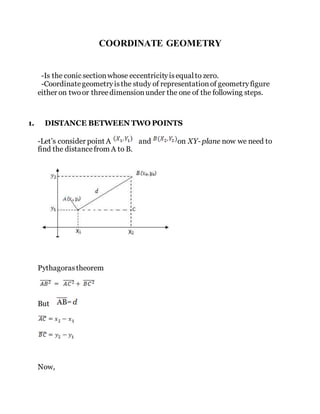
1. COORDINATE GEOMETRY
-Is the conic sectionwhose eccentricityisequalto zero.
-Coordinategeometryisthe study of representationof geometryfigure
either on twoor threedimensionunder the one of the following steps.
1. DISTANCE BETWEEN TWO POINTS
-Let’s consider point A and on XY- plane now we need to
find the distancefrom A to B.
Pythagorastheorem
But
Now,

2. For case of threeof dimension
MID – POINT
- - A midpoint ofline segment, is the point bisect a line segment.
OR
- Is the point which dividea certainlineinto two equalparts.
Proof,
-Consider point A(x1,y1), B(x2,y2) and R(x,y)
-Consider thefigurelie low.

3. From, similaritiesof ΔADRand ΔRCB.
but,
From equation

4. But
AD=X-X1
RC=X2-X
For X
For
From equation
But

5. Then,
EXAMPLE.
1. Find the distancebetweenA and B
2. Show that thepoint and are vertices of isosceles triangle.
3. Show that the point and are verticesof right angled
triangles.
4. Show that the point and (0, 1) areverticesof square.
Solution.
Given

6. Since
Then the point A (1,2), B (3,5), C (4,4) are verticesof isosceles triangle.
Hence shown.
TRI - SECTION
-Is the processof dividing a certainlineinto threesection or equalparts

7. Wherethe coordinateP intersectiondependson the two conditions.
i) When P is close to A
ii) When P is close to B

9. For P close to B.

11. RATIO THEOREM
- -Is the theorybased on a divisionof a lines segment either internally or
externally.
1. INTERNALLY DIVISION
Is a divisionof a lines segment internallyunder the given conditionof ratio.
-Let line AB being divided at R in ratioM: N.
Where
RatioM: N.
-Consider thefigurebelow.

12. From similaritiesof ΔADR and ΔRCB.

14. II. EXTERNALLY DIVISION
-Is the theorybased on a divisionof a lines segment externally under the
given ratio.
Let,

15. A (X1 ,Y1), B(X2,Y2) and R(X, Y) under rationR (X,Y).
-Consider thegraph below.

18. EXAMPLES
1. Find thecoordinateof point and divided internallyor
externallyin the ration
2. Find the point of in-sectionof a line a joining, point and if P
is closed of A.
3. Find the coordinateofin – sectionof a line AB at point P. If B is closer
to A given that A and B
SOLUTIONS

19. 1. For internallydivision

21. GRADIENT
- Is the rationexpressed as verticalchangeover horizontalchange.
OR
- Is the ratiobetweenchangein Y over changein X.
Mathematically
gradient denoted as
i.e
However thegradient canexplained byusing three different methods.
i) . GRADIENT FROM ANGLEOF INCLINATION
ii). GRADIENT FROM THE CURVE (calculusmethod)

22. iii). GRADIENT BETWEEN TWO POINTS.
- Consider thefigurebelow.
GRADIENT FROMANGLE OF INCLINATION
-Let θ be angleof inclination

23. GRADIENT FROMTHE CURVE
Thisis explained byusing calculusnotationidea where;
of a curveat a givenpoint.
However gradient canbeobtained directlyfrom the equationof a straight
line as coefficient of x from the equationinform of
y =mx + c
BEHAVIOUR OF GRADIENT BETWEEN TWO POINT
-Lets two pointsbe which canbe used to form theline
AB.

24. (i) If , and theline increasefrom left to right implypositive
slope.
(ii) If the line decreasefrom
right toleft imply slope.

25. (iii) If the line is horizontallywith zero gradient
(iv) If the line is verticalwith infinitygradient
COLLINEAR POINTS
- - Arepoint which lie on the same straight line

26. Where,
A, B, and C are collinear.
- - The conditionof collinear points have thesame slope/ gradient
Note:
If A ( ); B and C arecollinear thenthe area of
= 0.
Example 1
1. 1.Determinethe value of K such that following pointsarecollinear:-
a) and
b) and
2. Show that the points , and are collinear.
3. The straight line Cut the curve at P and Q.
Calculatethe length PQ.
4. If A and B are productsof OX and OY respectively. Show that xy=16.
If the area of is 8 unitssquare.

27. Solution:
A B , C
For collinear point
2. Give
; B C

28. Alternatively

29. Sincethe area of ΔABC is 0 unit hence the pointsare collinear.
3. Given

30. 4. Given

31. Since
Area of ΔOAB= 8 squareunits
Then,

32. 5. If and are thecollinear of midpoint of the line forming the points
and show that, x-y+1=0.
Solution
M.P = (x, y)
A and B

33. Required
EQUATION OF THE LINE
- Mostly depends on different format thoseunder one of the following
1. EQUATION OF A POINT AND SLOPE.
Let point be and slope be M.

34. -Let the two pointsbeing denoted as A and B
-Consider thefigurebelow
For the line only if

35. =
Multiplyby both side
=
3. EQUATION OF A SLOPE AND Y – INTERCEPT FORM
- -Consider theslope and – intercept,

36. 4. EQUATION OF 2 INTERCEPTSFORM
-Let consider X – intercept C, and Y intercept B

37. 5. FAMILY EQUATION
- Is the equationformed from intersectionoftwo lines passing through a
certainpoints.
E.g. equationfor intersectionofL1 and L2 passing through points(a,b) can
be obtained byusing theformula.

38. WhereK is constant
*
Important stepsof determining familyequation.
1. Solve for K by regarding equationsof twolines and the point passing
through.
2. Form familyequationby using the value of K without regarding the
point passing through.
Example.
1. Find the equationpassing through thepoint (2,3) from theintersection
to provided
Solution.
Point
But,
(

39. From
The equationis
ANGLE BETWEEN TWO LINES
-Let consider and whereQ is angle
madebetween and and is the angleof and is the angle of .
-Consider thefigurebelow

40. Our intentionstofind the value of which alwaysshould be acuteangle.
where

41. PARALLEL AND PERPENDICULAR LINES
(a) PARALLEL LINES
-Are the lines which never meet when they are produced
Meansthat is parallelto symbolically //
- -However conditionfor two or more lines to be parallel statethat they
posses the same gradient.

42. b) PERPENDICULAR LINES
- -Are the lines which intersect at right anglewhen they are produced.

43. Meansthat is perpendicular to
- -Symbolicallyis denoted as L1⊥L2
- -However theconditionfor two or more lines to be perpendicular states
that "Theproduct of their slopes should be equal to negativeone".
- -Let consider the figurebelow

44. NOTE:
1. The equationof the line parallel to the line = 0 passing
through a certainpoint isof the form of . Where – is
constant.
2. Theequationof the line perpendicular tothe line pass
through a certainpoint isof the form of when – is constant.
3. The calculationof K above done by substitutioncertainpoint passing
through.

45. THE EQUATION OF PERPENDICULAR BI SECTOR
- Let two point be A and B.
Where,
Line L is perpendicular bisector betweenpoint A and B.
Now our intentionisto find the equationof L.
IMPORTANT STEPS
1. Determinethemidpoint betweenpoint A and B.
2. SinceL and are ⊥ to each other then find slope of L.
for
3. Get equationof L as equationof perpendicular bisector of by using
and mid point of A and B.

46. THE COORDINATE OF THE FOOT OF PERPENDICULAR FROM
THE POINT
THE POINT TO THE LINE
- -Our intentionisto find the coordinateof thefoot (x,y) which act as the
point if intersectionof and .
- Let consider the figurebelow.
IMPORTANT STEPS
1. Get slope of formatted linei.e. and then use if to get slope of L2.
Since
2. From equationof by using and point provided from.
3. Get coordinateofthe food by solving the equation and
simultaneouslyas the way Y please.
EXAMPLE

47. 1. Find the acuteangle6. between lines
and
2. Find the acuteangle betweenthe lines represented by
3. find the equationof the line in which such that X – axisbisect the
angle betweenthe with line
4. find the equationof perpendicular bisector betweenA and B
5. Find the coordinateofthe foot perpendicular from of the line
6. Find theequationof the line parallel to the line 3x – 2y + 7 = 0 and
passing through thepoint
7. find the equationifthe line perpendiculartothe line
and passing through thepoint
8. Find the equationof perpendicular bisector ofAB. where A and B
are thepoint and respectively.
Solution
Given
Consider

48. From
Also
Recall
=
θ2tan-1 1
Therefore;

49. 2)
Solution
Given
Factorizecompletely
From

50. Recall
Given
Consider thefigurebelow

51. From
The slope is negativethenat x –axisy=0

52. 4) Solution
Given A B

53. From
Also
Midpoint =
M.p =
Then
The equationis
Solution
Given

54. From
But
For the equation

55. The coordinateofthe foot is
The perpendicular linefrom point A to the straightline
intersect theline at point B. if the perpendicular is
extended to C in such a way that AB = . Determineline
coordinateof C.
Solution

56. Given
Let
From
Then

57. The coordinateofis sincepoint B
Recall
Since
Then
For x
Compareoff

58. ∴
For y
The coordinateofC is

59. THE SHORTEST/PERPENDICULAR DISTANCE FROMTHE
POINT TO THE LINE
Introduction:
From the figureabove it be loved that PC is the only one posses
shortest distancerather thantheother as perpendiculartothe line
that is way, our intentionisto get shortest / perpendicular distance
from the point P to the line.
Our intentionis to find shortest distancefrom point to the
line .
From

60. Since
From the trigonometricidentity
Then

61. But
Also
Sincethepoint R is on the line

62. Hence;
Substitutethevalue of into 1 above
Then
Apply squareroot both sides
=

63. = +
Hence
At the point
Note:
The distancefrom the origintothe line ax +by + c = 0 is given by;
Example:
1. i) Find the perpendicular distancefrom point for the line
2. ii) Find the value of K if perpendicular distancefrom point for the
line is units.
3. iii) Find the shortest distancefrom the originto the line
4. If the shortest distancefrom the point to the line
is 3 units. Find the value fm.
Solution

64. Given: point
Line
Recall:
Theperpendicular distanceIs4 unit
Solution:
Given point
Required k

65. Then
THE EQUATION OF ANGLE BISECTOR BETWEEN TWO LINES
* Consider the figurebelow.

66. Where, PM and PN are perpendicular distancefrom point P. which are
always equal.
Since
Then
=
NOTE;

67. i) for the equationtake+ve
i ii) for the equationtake –ve
THE CONCURRENT LINES
These arethe lines which intersect at the samepoint.
Example:
- where and areconcurrent line.
- However thepoint of intersectionifconcurrent line normally
calculated under thefollowing steps.
1. Select two equationof straight linewhich relateto each other from the
those equationprovided.
2. Theget point of inter sectionof selected equationasusual. Pointsof
intersectionintothethird equationinsuch a waythat if the result of L.H.S
is equal R.H.S imply that theseline arecurrentslines.
Example;
i.Show that thelines
, and arecurrent lines

68. ii. Determinethevalue of M for which thelines
, – 3 = 0 and are
current.
iii. Find theequationof bisect of angle formed by the lines
represented by pair of the following.
a) and
b) and
Solution:
1)Given
By solving sincesimultaneousequation
)
=
For the first equationtaketheit be cones

69. Then for the equationtakecones from
=
=
The equationsof baseequationof the angle are
THE AREA OF TRIANGLE WITH THREE VERTICES
By geometricalmethod.
Consider the figurebelow.

70. Our intentionisto find the area of
Now,
Area of = area of trapezium ABED area of trapezium ACED
But area of trapezium
Also consider, Area of trapezium ABED
Area of trapezium DCEF
Area of trapezium
Then
But simplificationtheformula becomes
If ABC has A (x1, y1), B (x2, y2) and C (x3, y3) for immediatelycalculationof
area the following technique should be applied by regarding threevertices
of as A (x1, y1), B (x2, y2) and C (x3, y3)

71. Area =
TERMINOLOGIESOF TRIANGLE
is the line which dividesides of triangleat two equalpoints
-
If ABC has A (X1, Y1), B (X2, Y2) and C (X3, Y3) for immediately calculation of area the
following technique should be applied by regarding three vertices of as A (x1,y1),
B (x2, y2) and C (x3, y3)

72. Area =
TERMINOLOGIES OF TRIANGLE
1.MEDIAN
Median is the line which divide sides of triangle at two equal points
Where:
AQ, BR and CP are the median of
Also G is the centered of triangle
CENTROID FORMULA
Consider the figure below with verities A (x1, y1); B(x2, y2) and C (x3, y3)

73. Let line BM divide by point G in ration M: N = 2 : 1
Internally
The centroid formula is
=
2.
ALTITUDE OF TRIANGLE
Are the perpendicular drawn Y vertexes to the opposite side of triangles.
3. ORTHO CENTRE
Is the point of intersectionof altitude of triangle.
4. CIRCUM CENTRE
Is the point of intersection of perpendicular bisector of the side of triangle.

74. EXAMPLE.
1. find the area of triangle with vertices A (0,2), B (3,5) and C (-1, 9)
2. Find the centered of the where A (1,1); B (3,2) and C (5,4)
3. From triangle ABC, A (2,1) B (6, -9) and C (4,11) find the equation of altitude through A.

75. Area =
Area of = 12 Square units

76. Solution 2:
=
B(X2, Y2)=
C(X3, Y3)=
Recall
Solution :
Given
A , B C
Required the equation of latitude consider the figure below
Since
MAQ.MBC = -1
MAQ =
But
MBC =

77. MBC=
MBC =‾6
MBC=
Y = + 1
Y = - + 1
Y= +
THE LOCUS.
 Locus is a free point which moves on x – y plane under a given condition.
However the locus can be described by using various properties as vertical line (X = ), H
horizontal line (Y =B) straight line (Y = mx + C) on X – axis, (Y = C), on Y – axis
as well as equation of the circle x2 + y2 + 2gx + 2fy + C = 0 or x2 + y2 = r2
Note:
The point of locus is Locus equal distance means equal distance.
EXAMPLE.
1. Find the locus of P (x, y) which is equal distance from A (2, 3) and B (4, 7).
2. A point P moves so that it perpendicular distance from a line 3x + 5y + 4 = 0. Is proper final
to square of its distance from point Q (1, 2) if point (2,1) is
one possible position of P, prove that the equation of locus P is given by x2 + y2 – 8x – 14y -
3 = 0
3. Show that A (7, -2) and B (2, 6) are all equal distance from the line 3x -4y – 4 = 0.
4. A point Q moves such that its distance from point (5, 3) is equal to twice. Its distance from
the line X = 2. Find the equation of locus.
5. Find the locus of the point which moves so that the sum of the square of its distance from
point (-2, 0) and (2, 0) is 26 units.
Solution 1:
1. Given
A

78. B
Then consider
=
=
=
= =
Square both sides
+ = + ²
Expand
x² -4x + 4 +y² -6y +9 = x² - 8x + 16+ y² - 14y + 49
4x + 8y – 52 =0
The locus is straight line
2.
2. Given that 3x + 5y + 4 =0
P

79. Q
Point (2,-1) one possible position of P.
⊥ dPL
⊥dPl = IC ²
⊥dPl= --------------- (i)
Also
=
= + ²
⊥dPl = K
= K
But =
= K
= K
= 10K

80. =
⊥dDL=K
= K
=
=
6x + 10y + 8=
6x + 10y +8 = x² – 2x + 1 + y² – 4y + 4
² + y² – 8y- 14y -3=0
² + y² - 8y – 14y -3 =0
² + y² - 8y – 14y -3=0
Solution 4:
Q
A

81. L: =2
2QA = QL
Solution 5:
P
Points A & B (2, 0)

82. CIRCLE
Is the locus which sown on xy – plane so that it always constant distance i.e. radius from fixed
pilot i.e. Centre.
 Where the phiral of radius is radii.
GENERAL EQUATION OF CIRCLE
 consider the figure below on X – Y plane

83. Square both sides
r² =
Hence
r² =
The general formula for equation of the circle.
 By extending the above formula we get the general equation of circle.
= r²
² - 2ax + a² + y²- 2by + b² = r²
² + y² - 2ax – 2by + a² + b² - r² =0
² + y² + 2 y + a² + b² r² =0
-a =
-b =
Therefore
+ y² + 2 x + 2
Show as equation of circle
r

84. But ‾a = g, a= ‾g
‾b = f, b = ‾f
Also the coordinate of the center of the culve ( -g, -f)
1. To find the Coordinate of the Centre and radius of the circle make sure coeficient of X2 and y2 is
equal to 1.
2. Centre of the culve is (-1/2 coefficient x) (-1/2 coefficient + y)
3. If the circle posses through the origin, then c =0
4. If the Centre lie on X- axis f = 0
5. If the Centre lie on Y - axis g = 0
6. For the center at which the coordinate of the center lie at origin be (90). Their general equation
being in the form of
DETERMINATION OF CENTRE AND RADIUS OF THE CIRCLE.
Mostly done by using the two common methods:
1. By using general equation of circle.
2. By using completing the square method.
I. By using general equation of circle
Here normally the general equation applied in order to get the
value of G, F and C by comparing general equation of circle provided and equation of circle.
Where,
 coordinate of Centre (-g, -f)

85. II. By completing the square method
 is another method at which performed under the same rule of completing the
square method of a circle in order to express it in form of (x - a)2 + (y - b)2 = r2
Where,
i. Coordinate of the Centre taken under opposite sign of part of X and Y.
i.e. (x - 2)2 + (y + 3)2 = 9
C (a, b) = (2, -3)
ii. radius regarded as the square root of part of r2
r2 = 9
r2 = 3.
EXAMPLE.
1. Determine the coordinate of the Centre and radius of the following
(a) x2 + y2 – 4x – 6y – 12 = 0
(b) 4x2 + 4y2 – 20x – 4y + 16 = 0
2. Write down the standard equation of the circle with Centre at the origin and whose radius is 5
units.
3. Find the equation of circle passing through (2, 1) and Centre at (-3, -4).
EQUATION OF THE CIRCLE WITH TWO POINTS AS THE END OF DIAMETER.
 Let AB b diameter of the circle with coordinate A (x1,y1) and B (x2, y2) which give general
equation of (x – x1)(x – x2) + (y – y1)(y – y2) = 0.
Proof considers the figure below with two points as diameter.
Required equation of the circle let C be angle formed in the semi – circle.

86. Where < ABC = 90°
Since ACB = 90º
Then
Slope AB x Slope BC = -1
MAC x MBC = -1
But
MAC =
MBC =
+ =0
+ =0
EQUATION OF THE CIRCLE WITH THREE POINTS ON THE CIRCLE.
 Let consider the circle with three points on the circle such as A, B and C as shown below.

87. Generally the equation of the circle above calculated under the following methods.
I. By using general equation of the circle.
II. By using distance formula.
I. BY GENERAL EQUATION.
 Done by referring general equation of the circle x2 + y2 + 2gx + 2fy + c = 0
This form three equations in term of; f, g and c by substituting the point provided.
 Letters solves the equations three above in order to get the value of standard equation.
II. BY DISTANCE FORMULA
By using distance formula we formed two equations in term of A and B as C (a,b) under the
reference of distance formula above from the Centre to the point on the circle.
e.g.
Where, AO = BC and BO = OC.
By solving formed equation we can get value of C (a, b) together with radius we can
get the required equation.

88. EQUATION OF CIRCLE WITH TWO POINTS AND LINE PASSING AT THE
CENTRE.
 Let two point be A (x1, y1) and B (x2 ,y2) and line L1 Px + Qy + c = 0 passing at the Centre.
Now we need to find the equation of circle
Important steps
i. Since the line passing at the Centre means that the points of Centre satisfy the line.
By substituting the point in the line px + qy + c = 0.
ii. Form second equation by using distance formula in terms of a’ and b’
I.e. AC = CB
iii. By solving equations as can get the Centre
EXAMPLES.
1) Find the equation of the circle on the line forming the points (-1, 2) and (-3, 5) as the
diameter.
A circle is drown with points whose coordinate are A (OA) and B (P, Q) as the end of diameter,
the can lie cuts X – axis two points where coordinate are ( ) and (β, O), prove that + β = P
and P = Q.
Find the equation of the circle which passed through points A (-1, -5); B (6, 2) and C (0, 2).
Find the equation of the circle which is circumscribed about the triangle whose vertices are (-1, -
2); (1, 2) and (2, 3).
Find the equation of the circle whose Centre lies on the line y = 3x -7 and which pass through (1,
1) and (2, 1) and (2,3).
Find the equation of the circle passing through the origin and making intercepts (4,5) on the
axes coordinates.
Solutions
1. Given

89. Points B
Recall
= 0
=0
x² + 3x + x + 3 + y² – 5y – 2y + 10 =0
x² + 4x + 3 +y² – 7y + 10 =0
x² + y² + 4x – 7y + 13 =0
x² + y² + 4x – 7y + 13 = 0
From and (β,O)
Then x = β = X
(X – β) = 0
X - Xβ - X + β = 0
x² - (∝+β) x + β =0
x² - (∝+β)X + ∝β = 0
x - Px + a =0

90. Then
-
+ = P
=a
+ =a
A = a
Points A(‾1, ‾5) B(6, 2) and (0, 2)
By general equation
x2 + y2 + 2gx + 2fy + c =0
For A (‾1, 5)
26 – 2g – 10ƒ + c= 0
2g + 10 – c = 26 --------- (i)
+ + 2 g + 2
36 + 4 + 12g + 4f + c = 0
12g + 4f + c = ‾40
For c
4 + 4f + c =0
4f + c =‾4 ------------- (iii)

91. Hence
G=‾3, f = 2, c = ‾12
X² + y² + 2gx + 2fy + c =0
+ y² + 2 x + 2 y – 12 = 0
x² + y² -6x + 4y– 12 =0
Equation the circle is x² + y² – 6x + 4y – 12 =0
EQUATION OF CIRCLE WITH COORDINATE OF CENTRE AND EQUATION OF
TANGENT LINE.
Let consider Centre C (a, b) and tangent line px + qy -c = 0
Our intention is to find the equation of the circle.
IMPORTANT STEPS.
Determine the value of radius as shortest distance from Centre to the line.
By using value of radius and Centre we can get the equation.
TO THE CIRCLE.
Equation of tangent to the circle at the given point regarded in order to form of equations such
as.

92. Our intention is to find the equation of the tangent, let us find the slope.
By calculus method.
From
x² + y² = r²
But of a curve at
Apply both sides
Then
2x + 2y =0
2y = ‾2x

93. = M=
Then
=
a) By geometric method x² + y² = r²
Since
MCT ML = ‾1
But
MCT =
MCT =
ML =
ML =
MT =
But equation of tangent
Y = M(x -x1) + y1

94. But M =
=
PROBLEMS.
1. Find the equation of circle with Centre (3,-4) and tangent to line 3x + 4y + 3 = 0.
2. Find the equation of the circle whose Centre is 9 units to the right of Y – axis whose radius is
2 units and which touch the line 2x + y – 10 = 0.
3. Find the equation of tangent to the circle x2 + y2 = 18 at pint (7, -3).
4. Find the equation of tangent for the circle through point (2,-4) given that x2 + y2 – 2x – 4y +
1 = 0.
5. Show that the point (7, -5) lies on the circle x2 + y2 – 6x + 4y – 12 = 0. Find the equation of
tangent to the circle at the point.
Solution 1
C
From 3x + 4y + 3=0

95. From equation of circle
+ = r²
=
+ =
Centre 9 units to the right y-axis radius = 2
(i) 2x + y – 10=0

96. 2 x 5 = 8 + y
10 – 8 = y
y = 2
Then c(9,y)
But y =2
C(9,2)
From
= r²
3. Given
x² + y² =58
Point
Recall
xx1 + yy1 = r²
But (x1, y1) =
r² = 58
7x – 3y = 58
7x -3y =58
Solve equation 4
Given

97. x² + y² - 2x – 4y + 1 =0
By using calculation approach
5. Give:solve equation 5.
Point (7, -5)
x² + y² - 6x 4y – 12 =0
THE CONDITION OF CERTAIN LINE TO BE TANGENT TO THE CIRCLE.
In order y = Mx + c to be tangent to the circle x2 + y2 = r2, x2 + y2 + 2gx + 2fy + c = 0. always
can be checked by the following method:
By using value of radius as perpendicular ions hence from Centre to the line y = Mx + c by
solving y = Mx + C and x2 + y2 2gx + 2fy + c = 0. By substituting the value of Y into either of
the equation of circle hence if b2 = 4ac, then the line is length of the circle.
Examples.
1) Find the value of K if 12x + 5y + K = 0. is length to the circle x2 + y2 – 6x -10y + 9 = 0
2) Show that 5x + 12y – 4 = 0. Touches the circle x2 + y2 – 6x + 4y + 12 = 0.
Solution
From
X² + y² – 6x – 15y + 9=0
c(-g,-f)=(-1/2(x),-1/2(y))
=
Also r =
r =
r = 5 units

98. r = from Centre to tangent
r = at (x, y)
But r = 5
5 =
5=
5 x 13 = 61 + k
65 – 61 = k
K = 4
The value of k = 14
DISTANCE OF TANGENT FROM EXTERNAL POINT TO THE CIRCLE.
Consider the figure below.
By Pythagoras theorem
= +
=
But

99. CP =
²
Also
CT = radius
Then = g² + f² -c
From = ² -
² = + -
= x² + 2 x g + g + y² + 2fy + f² -g² - f² + c
= x²+ 2 x g + y² + 2fy + c
= x² + y² + 2xg + 2fy + c
=
EQUATION OF TANGENT TO THE CIRCLE FROM EXTERNAL POINT.
 Here the equation of tangent may be answered as Y = M (x1 – x1) + y1 where x, and y, are
the external point which may be at the origin but not at contact of tangent to the circle.
 So in order to get equation we need to find the slope ‘M’ e.g. let consider equation of tangent
from P(a, b) to the circle x2 + y2 = r2 or x2 + y2 + 2gx + 2fy + c = 0.
Y= M(x - x1) + y
Y= Mx - M1 + Y1
Mx– y - Mx1 + y1
From
r= d =

100. at
r= d = at
r =
at
Hence the equation of the line can be obtained by using slope M from above formula together
with point from.
GENERAL EQUATION OF TANGENT TO THE CURVE WITH THE GIVEN SLOPE.
Let Y = Mx + c be equation of tangent to the curve x2 + y2 = r2 or x2 + y2 + 2gx + c = 0. With
given slope M to solve for c.
From
y= Mx + c
x² + y² = r²
Solve the above equation
x² + y² = r²
But y = mx + c
x2(mx+C)2=r2
x² + m²x² + 2xmc + c²=r2
x²+m²x² + 2xmc + c² - r²=0
=0
a= m² + 1
b= 2mc
c= c2 – r2
Recall
b² =4ac
= 4
4m²c²= 4
4m²c²= 4m²c² - 4m²r² + 4c² 4r²
4m²c² = 4
m²c²= m²c² - m²r² + c² -r²
0= ‾m²r2 + c² - r²
c²= m²r² + r²
c² = r²
c=
c=
Recall
y = Mx + c

101. y =Mx ± r
y = Mx r
POSITION OF POINT WITH RESPECT TO CIRCLE
The done under the following
If then the point R lies inside the circle
If = r then the point P lies on the circle
If OR r then the point R we outside the circle
PROBLEMS.
1. Find the length of tangent from (1, 2) to the circle, x2 + y2 – 4x – 2y + 4 = 0.
2. Find the length of tangent from the origin to the circle x2 + y2 – 10x + 2y + 13 = 0.
3. Find the equation of tangent from (-1, 7) to the circle x2 + y2 = 5.
4. Find the equation of tangent to the circle x2 + y2 = 16. If the slope of tangent is 2.

102. 5. Examine whether the point (2, 3) lie at side/ inside the circle.
6. discuss the position of point (1, 2) and (6, 0) with respect to circle x2 + y2 – 4x – 2y – 11 = 0
Solution 1.
Given
(1, 2)
x² + y²- 4x – 2y +4 =0
c(-g, f) = (2, 1)
r=
r=
r=
r = 1units
Consider the figure below
By Pythagoras theorem
=2
Also
= Radius

103. PT = 1 Unit of length
Length of tangent = 1 unit
ORTHOGONAL CIRCLES
This circle said to be orthogonal only if their radii are perpendicular to each other.
Simply their radii meet at right angle.
Means that circle I and II are orthogonal
The condition for orthogonal circles mostly explained by concept of Pythagoras theorem r1
2 + r2
2 =
Where
C1C2 = distance at Centre
r1 and r2 are radii of the circles.
Alternatively
From
r1² + r2² = But r1 =
r1²= g1² + f1² - c ---------- (i)
Also
r2 =
= g2² + f2² - c2 -------- (ii)
Let C1 , (-g1 , -f1) and C2 (-g2, -f2)
Recall
=

104. = + ² ----- (iii)
From
r1
2 + r2
2 = 2²
When and 2 are constant of the circle
EXAMPLE
1. Find the value of K if circle x2 + y2 – 2y – 8 = 0 and x2 + y2 – 24x + Ky – 9 = 0 are orthogonal.
2. show that the following circle are orthogonal x2 + y2 – 6x -8y + 9 = 0
and x2 + y2 = 9.
3. Find the equation of circle which passes through the origin and cut orthogonally circle
x2 + y2 + 8y + 12 = 0 and x2 +y2 - 4x - 6y – 3 = 0.
4. find the equation of circle which cut the circle x2 + y2 – 2x – 4y + 2 = 0, x2 + y2 + 4x = 0.
5. find the equation of the circle through the point (2,0); (0, 2) and 2x2 + 2y2 + 5x–6y + 4 = 0
Solution 1
given
x² + y² - 2y – 8 =0
x² + y² - 24x + ky – 9 =0
Recall
2g1g2 + 2f1f2 =c1 + c2
Then
-g1 =0 = g1 =0
-f1 = 1 = f1 = ‾1
Also
-g2 = 12
g2 = ‾12
=
f2 =
But C1 = -8, and C2 = ‾9

105. C1 + C2 = 2g1g2 + 2f1f2
‾8 – 9 = 2 + 2
‾17 = ‾k
K = 17
The value of K = 17
Solution 2
= 9
From centres are
C1 and C2
Constants
C1 = 9, C2= ‾9
C1 = g1= ‾3, f1 = ‾4
C2 = g2 = 0, f2 = 0
Then
C1 + C1= 2g1g2+ 2f1f2
9 -9 = 2 + 2
0 = 0
Since LHS = RHS hence the circles are orthogonal
Solution 3
Passing through the origin
C = 0
x² + y² -8y + 12 = 0 and
x² + y² - 4x – 6y – 3 =0
Let x² + y² + 2gx + 2fy + c =0
2g1 = 2g
g1 =g
2f1 = 2f
f1 =f
c1 = c
But c = 0
For 2nd equation
2g2 = 0
g2 =0
2f2 = ‾8
f2 = ‾4
c2 = 12
Recall

106. c1 + c2 = 2g1g2 + 2f1f2
2g + 2f = 12 +0
‾8f = 12
f=
Also let 2nd equation be
2g2 = ‾4
g2 = ‾2
2f2 = ‾6
f2 = ‾3
c2 = ‾3
Recall
2g1g2 + 2f1f2 = c1 + c2
But
g1=g ,
f1 =f
2g + 2f = 0 +
‾4g + 6f = ‾3
From
4g + 6f = 3
But f =
4g = 3 + 9
=
g= 3
Value of g =3, f=
Recall
+ 2gx + 2fy + c =0
+ 6x – 3y =0
The equation is + 6x – 3y =0
Solution 4
Given
Points and
2x² + 2y² + 5x – 6y + 4 =0

107. Let
+ 2gx + 2fy + c = 0
Point =
4 + 0 + 4g + 0 + c =0
4 + 4g + c =0
4g + c = ‾4 -------- (i)
Also point (OR)
0 + 4 + 0 + 4f +C =0
4 + 4f + c =0
4f + c =‾4 -------------(ii)
Let
+ 2gx +2fy +c = 0
2g1 = 2g
g1=g
2f1 = f
f1 = f and c1 =c
The 2nd equation
2x² + 2y² + 5x – 6y + 4 =0
Recall
C1 + C2 = 2f1f2 + 2g1g2
C + 2= 2f + 2g
C + 2 = -3f +
- 3f – c = 2

108. g=
f=
c=
Then
+ 2gx + 2fy + c =0
Substitute the value of g, f and c
x² + y² + x + 2 y - =0
- - =0
7x² + 7y² - 8x – 8y -12 =0
The equation of the circle is 7x² + 7y² - 8x – 8y -12=0
INTERSECTION OF TWO CIRCLES.
Intersection of two circles may be forced into three cases.
1. Common tangent.
2. common chord
3. Line of separation.
1) COMMON TANGENT.
Is a line at which two circle intersection at a single point.
2) COMMON CHORD.
Common chord is the line at which two circles intersection at two distance point.
3) LINE OF SEPARATION.
Line of separation is the line between two circles which has no point of intersection

109. How to find common tangent, chord or line of separation?.
This is done by either fut. strait the equation of one circle from another circle. i.e. C1 – C2 or C2 – C1
The different between two equations of circle represent common tangent, chord or line of
separation.
Where,
The point of intersection of either common tangent or common chord obtained by solving them
simultaneously under the following.
1) If b2 = 4ac, the line is common tangent.
2) If b2 > 4ac, the line common chord.
3) If b2 < 4ac, the line is line of separation.
Note:
The other concept of intersection of circle explained as follow.
1) If the circle intersection externally.
CONCY CLIC CIRCLE.

110.  These are two circles which passes the same coordinate of Centre but varies is radius
Where
If four points are concyclic imply that by forming the equation of concyclic circle by using three
points the fourth point should be satisfy the equation.
EXAMPLE.
1. Show that the part of the line, 3y = x + 5 is a chord of a circle, x2 + y2 – 6x – 2y – 15 = 0.
2. Find the equation of common chord and the intersection point of the circles x2 + y2 + 6x – 3y + 4
= 0, 2x2 + 2y2 – 3x - 9y + 2 = 0.
3. Find the length of the chord of the circle x2 +y2 – 2x -4y – 5 = 0, whose mid-point (2, 3).
4. Show that the circle x2 + y2 – 4x + 6y – 10 = 0, and x2 + y2 – 10x + 6y + 14 = 0and passing
other.
5. Find the equation of the circle concentric with the circle x2 + y2 + 4x +6y + 11 = 0. And pass
through.(5, 4)
6. Find the equation of circle which passes through the Centre, x2+ y2 + 8x + 10 y – 7 = 0, and is
concentric with the circle 2x2 + 2y2 - 8x – 12y – 9 = 0.
7. Find the equation of the circle concentric with circle. 2x2 + 2y2 + 8x +10y – 39 = 0. And having
its area equal to 16
Solution
Given
x² + y² + 4x + 6y + 11=0
Point
=

111. R =
R =
R =
R=
(a, b) = (‾2, ‾3)
+ = r²
+ = 98