Algebra(04)_160619_01

© Art Traynor 2011
Algebra
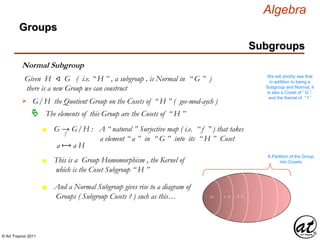
Normal Subgroup
Groups
Subgroups
Given H ⨞ G ( i.e. “ H ” , a subgroup , is Normal in “ G ” )
there is a new Group we can construct
G/H the Quotient Group on the Cosets of “ H ” ( gee-mod-aych )
The elements of this Group are the Cosets of “ H ”
G → G/H : A “ natural ” Surjective map ( i.e. “ f ” ) that takes
a element “ a ” in “ G ” into its “ H ” Coset
f
n
a ⟼ a H
This is a Group Homomorphism , the Kernel of
which is the Coset Subgroup “ H ”
n
And a Normal Subgroup gives rise to a diagram of
Groups ( Subgroup Cosets ? ) such as this…
nn
H a ·h b ·h
A Partition of the Group
into Cosets
We will shortly see that
in addition to being a
Subgroup and Normal, it
is also a Coset of “ G ”,
and the Kernel of “ f ”

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·h b ·h
A Partition of the Group
into Cosets
Suppose that we have an intermediate Subgroup ?
H ⨞ K ⊂ G “ K ” is a Subgroup of “ G ” that contains
the Normal Subgroup “ H ”
1
Then, in consequence :
“ H ” is Normal in “ K ”
Because to say that “ H ” is Normal in “ G ”
means that :
nn
∃ H H = g · H · g – 1 ∀ g ∈ G|:
This is true as well for any (?) Subgroup of “ G ”nn

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
H ⨞ K ⊂ G “ K ” is a Subgroup of “ G ” that
contains the Normal Subgroup “ H ”
1
We can construct a Quotient Group K/H
which can be regarded as a subset of G/H
G
“ K ”
K/H ⊂ G/H
… n – 1 ·H n ·H
A partition of the Group into
Coset Subgroups ( “ G/H ” ) ?
The “H” Cosets that contain Elements in “ K ”nn

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
H ⨞ K ⊂ G
K/H is in fact a Subgroup of G/H
( because “ K ” is a Subgroup of “ G ” )
G
“ K ”
K/H ⊂ G/H
… n – 1 ·H n ·H
1a
“ K ” is a Subgroup of “ G ” that
The cosets c·K are stable under multiplication just as
“ K ” itself is stable under multiplication
1b
The product of any two elements in “ K ” will remain in “ K ”nn

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
H ⨞ K ⊂ G
G
“ K ”
K/H ⊂ G/H
… n – 1 ·H n ·H
Akin to the “ H ” Cosets that contain Elements in “ K ”nn
If we take “ G ” partitioned into the distinct Cosets for
“ H ” , a distinct number of them will form the
Subgroup “ K ”
nn
“ K ” itself is a union of “ H ” Cosets { a ·H }nn
K = { ( ai ·H ) }∪i = 1
n
The Cosets corresponding to
a Subgroup of “ G ”
Lay, Section 2.5, (Pg. 39)

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
H ⨞ K ⊂ G
Conversely then, in consequence :
G
“ K ”
K/H ⊂ G/H
… n – 1 ·H n ·H
2 Any Subgroup of “ G ” containing “ H ” corresponds
to a Subgroup of “ G/H ” in this manner
There is thus a bijection between the Subgroups of “ G ”
containing a Normal Subgroup
and the Subgroups of the Quotient Group
nn

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
G
“ K ”
… n – 1 ·H n ·H
nn
Proof:
We will prove Professor Gross’s claim by demonstrating
the following :
G/H is a Quotient Subgroup of “ G ”o
“ H” is a Normal Subgroup of “ K ”o
There exists a Bijection Between “ S ” and “ K ”o
“ S ” and “ K ” share the same Order / Cardinalityo
“ S ” is the Set of all Subgroups of a Group “ G ”o
o
We utilize the “Architecture” of
Group Structure to discover
new patterns of Relation
Because every Normal
Subgroup corresponds to a
Group Homomorphism, the
Kernel of which is the
Normal Subgroup

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
G
“ K ”
… n – 1 ·H n ·H
nn
Proof:
a
Let “ G ” be a Group
Inexhaustive Inclusion
By Introduction, G.A.
Let G/H ⊂ G ( ∃ x ) ( x ∈ A ∧ x ∉ B )A Quotient Subgroup
Let S = { ( ai ·H ) }∪i = 1
n
Set of
Subgroups of “ G ”
Artin Section 2.2, ( Pg. 45 )
Proposition 2.4
Closure Property of Subgroups
( ∀ b ∈ ℤ ) ( H = b · ℤ ) ⊆ ℤ +
Let H ⨞ K ∀ K ∈ S
Definition 4.8
Normal Subgroup Structure Criteria
( N ⊆ G ) ( ∀ a ∈ N ∧ b ∈ G )
( b·a·b – 1 ∈ N )
b
c
d

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
G
“ K ”
… n – 1 ·H n ·H
nn
Proof:
Let S = { ( ai ·H ) }∪i = 1
n
Set of
Subgroups of “ G ”
Proposition 2.4
Closure Property of Subgroups
( ∀ b ∈ ℤ ) ( H = b · ℤ ) ⊆ ℤ +
Let H ⨞ K ∀ K ∈ S
Definition 4.8
Normal Subgroup Structure Criteria
( N ⊆ G ) ( ∀ a ∈ N ∧ b ∈ G )
( b·a·b – 1 ∈ N )
c
d
e ∴ ∃ f : S ⥴ K QED – By Implication

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
We will shortly see that in addition to
being a Subgroup and Normal, it is also
a Coset of “ G ”, and the Kernel of “ f ”
H ⨞ K ⊂ G
G
“ K ”
K/H ⊂ G/H
… n – 1 ·H n ·H
Example:
Let G = ℤ under addition and let “ p ” be a prime number
and consider the Subgroup H = p ·ℤ i.e. the multiples of “ p ”
If we have a Subgroup of ℤ which lies between ℤ and p ·ℤ ,
( i.e. there is a “arbitrary” K such that ℤ < k ·ℤ < p ·ℤ )
1
2

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
H ⨞ K ⊂ G
G
“ K ”
K/H ⊂ G/H
… n – 1 ·H n ·H
Example:
and consider the Subgroup H = p ·ℤ
i.e. the multiples of “ p ”If we have a Subgroup of ℤ which lies between ℤ and p ·ℤ ,
1
2
a S = { k ∀ k ℤ , ℤ < k ·ℤ < p ·ℤ }|:
Note that the quantification
( i.e. Instantiation ) assumption
is invalid for the field ℤ
( ∀ x ℤ ) Ф ( x ) ⊢ Ф ( k )
Ф ≔ k ℤ < k ·ℤ < p ·ℤ|:
Then either K = ℤ or K = p ·ℤ
I.e.: There is nothing “ properly “ that can
lie between these two Subgroups

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
Suppose that we have an intermediate Subgroup ? G
“ K ”
… n – 1 ·H n ·H
Example:
and consider the Subgroup H = p ·ℤ
i.e. the multiples of “ p ”If we have a Subgroup of ℤ which lies between ℤ and p ·ℤ ,
1
2
a S = { k ∀ k ℤ , ℤ < k ·ℤ < p ·ℤ }|:
( ∀ x ℤ ) Ф ( x ) ⊢ Ф ( k )
Ф ≔ k ℤ < k ·ℤ < p ·ℤ|:
Then either K = ℤ or K = p ·ℤ I.e.: There is nothing “ properly “ that can
i.e. : p ·ℤ ⊂ K ⊂ ℤ

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
i.e. the multiples of “ p ”
2
a S = { k ∀ k ℤ , ℤ < k ·ℤ < p ·ℤ }|:
( ∀ x ℤ ) Ф ( x ) ⊢ Ф ( k )
Ф ≔ k ℤ < k ·ℤ < p ·ℤ|:
Then either K = ℤ or K = p ·ℤ , ( p ·ℤ ⊂ K ⊂ ℤ )
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
Such a “ K ” gives a Subgroup of the Cyclic Group
( i.e. Quotient Group ) ℤ /p·ℤ
i.e.: either the zero Subgroup
or the entire Group H = ℤ /p·ℤ
Note that a Group of Prime Order
exhibits no non-trivial Subgroups
Because the Order of a Subgroup
( i.e. Cardinality ) divides the Order
of its Parent Group
1
Note that the multiples of ℤ ( i.e. p ·ℤ ) is not
the same as zee-mod-pee-zee ( i.e. ℤ / p ·ℤ )

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
Then either K = ℤ or K = p ·ℤ , ( p ·ℤ ⊂ K ⊂ ℤ ) I.e.: There is nothing “ properly “ that can
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
Such a “ K ” gives a Subgroup of the Cyclic Group
( i.e. Quotient Group ) ℤ /p·ℤ
i.e.: either the zero Subgroup
or the entire Group H = ℤ /p·ℤ
Note that a Group of Prime Order
exhibits no non-trivial Subgroups
Because the Order of a Subgroup
( i.e. Cardinality ) divides the Order
of its Parent Group
This “ H ” represents a Maximal Subgroup of “ H ”
i.e. H = ℤ /p·ℤ
Anything larger than “ H ” must
necessarily exhaust the entire Group
2
1

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
We will use the Quotient Theorem3
If the Subgroup of the Quotient Group ℤ /p·ℤ is zero
this means that “ K ” corresponds to “ p·ℤ ” because it is given
by the union of Cosets in that Subgroup…
And the only Coset in that Subgroup is “ H ” …

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
So we get p ·ℤ where p ·ℤ ⊂ K ⊂ ℤ p ·ℤ thus is said to “exhaust” K
K = { ai ·H }∪i = 1
n
p ·ℤ ·K· ⊂ ℤ → p ·ℤ = ai ·H ⊂ ℤ
If “H” is the only
Subgroup…
·

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
So we get p ·ℤ where p ·ℤ ⊂ K ⊂ ℤ p ·ℤ thus is said to “exhaust” K
K = { ai ·H }∪i = 1
n
p ·ℤ ·K· ⊂ ℤ → p ·ℤ ⊂ ℤ
If “H” is the only
Subgroup…
·

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
On the other hand, if the Subgroup “ K ” of this Cyclic Group ,
the Quotient Group ℤ /p·ℤ represents all of the Group …
Then the Subgroup H ⨞ K is the Union of all the
Cosets of “ H ” , namely ℤ
ℤ thus is said to “exhaust” K

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
the Quotient Group ℤ /p·ℤ , represents all of the Group …
K ≠ 0 ⊂ ℤ /p·ℤ
Recall that H ⨞ K thus
H ⨞ K ≠ 0 ⊂ ℤ /p·ℤ

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
H ⨞ K ≠ 0 ⊂ ℤ /p·ℤ
Then the Subgroup H ⨞ K is the Union of all the Cosets of “ H ”
H ⨞ K = { ai ·H }∪i = 1
n

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
H ⨞ K ≠ 0 ⊂ ℤ /p·ℤ
H ⨞ K = { ai ·H }∪i = 1
n
and the Union of all the Cosets is equal to ℤ …
{ ai ·H } = ℤ∪i = 1

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
H ⨞ K ≠ 0 ⊂ ℤ /p·ℤ
H ⨞ K = { ai ·H }∪i = 1
n
{ ai ·H } = ℤ∪i = 1
whereas p·ℤ = H …
thus p·ℤ ⨞ K …

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
H ⨞ K ≠ 0 ⊂ ℤ /p·ℤ
H ⨞ K = { ai ·H }∪i = 1
n
{ ai ·H } = ℤ∪i = 1whereas p·ℤ = H …
p·ℤ ⨞ K = { ai ·H }∪i = 1
n
n

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Proof:
H ⨞ K = { ai ·H }∪i = 1
n
{ ai ·H } = ℤ∪i = 1whereas p·ℤ = H …
p·ℤ ⨞ K = { ai ·H }∪i = 1
n
n
thus … p ·ℤ ⨞·K· ℤ → p ·ℤ ⊂ ℤ·

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Conclusion:
Any subgroup of G containing H maps to G/H
G → G/H : A “ natural ” Surjective map ( i.e. “ f ” ) that takes
any element in “ G ” into its “ H ” Coset
f
H ⊂ G
∴ ∀ H ∈ K , K → G/Hf

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Conclusion:
The inverse Image of “ f ” is simply the map “ f – 1 ”
∀ H ∈ K , K → G/Hf
∪i = 1
n
a subgroup of “ G/H ” in “ G ” , and G = { ai ·H } = K ,
the set of all elements mapping to the Subgroup

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Conclusion:
∀ H ∈ K , K → G/Hf
This gives us a subgroup “ K ” in “ G ” that corresponds Bijectively to the Subgroup “ G/H ”
i.e. : ∃ f : K ⊂ G ⥴ G/H

© Art Traynor 2011
Algebra
Normal Subgroup
Groups
Subgroups
H a ·H b ·H
“ K ”
… n – 1 ·H n ·H
Example:
2
i.e. : p ·ℤ ⊂ K ⊂ ℤ
Conclusion:
∀ H ∈ K , K → G/Hf
∃ f : K ⊂ G ⥴ G/H
And if we can say something about the Quotient Group , namely that it has no no-trivial
Subgroups , we then know that there’s nothing “ squeezed ” between ℤ and p ·ℤ

© Art Traynor 2011
Algebra
Abstract Vector Spaces
Definition
Usually treated in Linear Algebra over the Reals ℝ , or the Complex numbers ℂ
Canonically denoted “ V over ℝ ” or “ V over ℂ ”
An Abelian Group
Scalar Multiplication
1
2
Elements are denoted
by a lower case “ v ”
We can always add vectors
( as for the Integers ℤ – not a Vector Space )

With Operation ( LOC ) of “ + ”nn
i.e.: v + wnn
With Identity Element : 0
With Inverses : – v
v ⟼ c·v
By “ c ”  ℝ

© Art Traynor 2011
Algebra
Definition
An Abelian Group
1
2
With Operation ( LOC ) of “ + ” ; i.e.: v + w
v ⟼ c·v
By “ c ”  ℝ
A Vector Space is thus something “ a little more ”
( i.e. structurally enriched ) than an Abelian Group


© Art Traynor 2011
Algebra
Definition
An Abelian Group
1
2
With Operation ( LOC ) of “ + ” ; i.e.: v + w
v ⟼ c·v
By “ c ”  ℝ
Thus composed , ScaMul must obey some identities within a Vector Space
0 · v = 0v
1 · v = v
( a · b ) v = a ( b · v )
Etc …

© Art Traynor 2011
Algebra
Definition
Examples of Vector Spaces :
0 = { 0 } = 0v
1 zero vector
v = ℝ all real numbers ,
represented by the real number line
2
0 1 2– 1– 2
ℝ
– ∞ … …∞ +
3 v = ℝ n
v = ( ai , ai+1 , … , an – 1 , an ) ∀ ai ∊ ℝ
v + w = ( ai + bi , ai+1 + bi+1 , … , an – 1 + bn – 1 , an + bn )
v ⟼ c·vc·v = ( c·ai , c·ai+1 , … , c·an – 1 , c·an )
w = ( bj , aj+1 , … , am – 1 , am ) ∀ bj ∊ ℝ

© Art Traynor 2011
Algebra
Vector Addition
Vector Space LOC’s
v = ( ai , ai+1 , … , an – 1 , an ) ∀ ai ∊ ℝ
w = ( bj , aj+1 , … , am – 1 , am ) ∀ bj ∊ ℝ
x
y
O
( v + w )
vcorr
w
v
Vector Addition ( PVF )
a
a
b
b
( a + b )
( a + b )
v + w = ( ai + bi , ai+1 + bi+1 , … ,
an – 1 + bn – 1 , an + bn )
∀ n = m ( a square matrix )
3a
3b
Vector Scalar Multiplication
c·v = ( c·ai , c·ai+1 , … , c·an – 1 , c·an )
xO
2v
v
y Vector Scalar Multiplication ( PVF )
v
Vector Modulus
3c |v | = Σi = 0
n
ai
2
Vector Inner Product
3d v · w = Σi = 0
n
ai · bi
the length ( modulus ) of a vector
a Manifold…

© Art Traynor 2011
Algebra
Vector Spaces over a Field “ F ”
Vector Spaces Over a Field
Fields
Field :
Something that abstracts what the Reals ℝ , or the Complex numbers ℂ are
.Will preserve “characteristics” of ℝ and ℂ


Dimensionnn
Basisnn
Spannn
Matricesnn
Field Properties :
1 An Abelian Group under Addition
Additive Identity : Zero
Additive Inverses : – a ( negation / antipodes )

© Art Traynor 2011
Algebra
1 An Abelian Group under Addition
Additive Identity : Zero
Additive Inverses : – a ( negation / antipodes )
2 Features a Multiplicative LOC
F – { 0 } = F *
Forms an Abelian Group under multiplication “ x”
Multiplicative Identity : Unity or “ 1 ”
Inverse Definition : a –1 =
Inverses must be defined
for all non-zero elements
1
a
Fields
Notation: the set exclusion
operator would be a more
“ well formed ” means of
expressing this restriction…

© Art Traynor 2011
Algebra
An Additive Group
A Multiplicative Group
Two Groups are needed to form a Field
Counter Example
The Integers ℤnn
Consider the element “ 2 ” – its multiplicative inverse is 1
2
∉ ℤ ,
1
2 ∈ ℚ
1
2
which violates the Closure principle of GSC as this product
is not within the Group ℤ , i.e.
Fields

© Art Traynor 2011
Algebra
An Additive Group
A Multiplicative Group
Two Groups are needed to form a Field
Examples
The Real Numbers ℝ form a Fieldnn
The Complex Numbers ℂ form a Fieldnn
The Rational Numbers ℚ form a Fieldnn
a
b , b ≠ 0
a
b
= if a ·b′ = b ·a′a′
b′
Recall that ℤ is not a Field
Fields

© Art Traynor 2011
Algebra
Field Structure Criteria ( FSC )
Fields
Definition 2.3
A Field “ F ” is a Set
together with two Laws of Composition ( LOC’s )
to which the Distributive Property obtains
F ∗ F → F
+
a , b ⇝ a + b
Law of Composition (LOC)
is generically indicated
by the operator notation “ ∗ ”
F ∗ F → F
x
a , b ⇝ a · b
1. Closure under Addition
2. Closure under Multiplication
a. Additive Identity = 0
b. Additive Inverse = – a
a. Multiplicative Identity = 1
b. Multiplicative Inverse
a– 1 =
1
a
c. Satisfies Abelian Group
Structure Criteria ( AGSC )
c. F0 ↔ F – { 0 } satisfies
Associative & Commutative
Group properties
3. Distributive Property Obtains

© Art Traynor 2011
Algebra
Fields
Definition 2.3
F ∗ F → F
+
a , b ⇝ a + b
Which satisfies the following axioms :
Addition renders “ F ” into an Abelian Group F+
Identity Element is zero
Negation defines the Inverse Operation
a , b ↔ – a , – b

© Art Traynor 2011
Algebra
Fields
Definition 2.3
Which satisfies the following axioms :
Multiplication imparts “ F ” with Associative & Commutative properties
Identity Element is Unity , i.e. +1
F ∗ F → F
x
a , b ⇝ a · b
Exclusion of zero ,
i.e. F
x
= F0 ↔ F – { 0 }
admits Inverse Operation of Negative Unitary Exponentiation

a– 1 =
1
a
Group properties
a – 1 =
1
a

© Art Traynor 2011
Algebra
Fields
Definition 2.3
Closure Under Addition
Closure Under Multiplication
a– 1 =
1
a
Group properties
3. Distributive Property Obtains
Distributive Property Obtains
For all a , b , c in ℝ
∀ a , b , c ∊ F , ( a + b ) · c = a ·c + b · c
( a + b ) · c = a ·c + b · c
Note that by Artin’s reckoning this suggests
that the Field Distributive Property is
nevertheless chiral ( i.e. 1HS Distributive
does not imply LHSD = RHSD )

© Art Traynor 2011
Algebra
Subfields :
If closed under “ + , x , a – 1 = ”
1
a
ℚ is a Subfield of ℝ
ℝ is a Subfield of ℂ
ℚ ⊂ ℝ ⊂ ℂ
Fields
Subfields

© Art Traynor 2011
Algebra
How to Construct a Field
Best practice is to first seek the simplest example :
Fields
A Set , Vector Space , or Field populated with only a single Element
However we require two distinct elements for the respective identities:
Additionnn
Multiplicationnn
So any Field must contain a least two elements :
Additive Identitynn
Multiplicative Identitynn
Can it be done with only two ? … Yes !
Consider the simplest Field, Cardinality two , “ F ” = { 0 , 1 }
Mathematically identical ( or
akin ) to the sets of even and
odd numbers respectively…

© Art Traynor 2011
Algebra
Fields
MultiplicationTable
LOC
( x ) 0 1
0
1
0 0
0 1
Addition Table
LOC
( + ) 0 1
0
1
0 1
1 0
By dint of modular
arithmetic , we will
consider 1 + 1 = 0

© Art Traynor 2011
Algebra
Fields
MultiplicationTable
LOC
( x ) 0 1
0
1
0 0
0 1
Addition Table
LOC
( + ) 0 1
0
1
0 1
1 0
By dint of modular
consider 1 + 1 = 0
Under addition this forms
the Cyclic Group ℤ / 2 ·ℤ

© Art Traynor 2011
Algebra
Fields
MultiplicationTable
LOC
( x ) 0 1
0
1
0 0
0 1
Addition Table
LOC
( + ) 0 1
0
1
0 1
1 0
By dint of modular
consider 1 + 1 = 0
Under addition this forms
the Cyclic Group ℤ / 2 ·ℤ
Applying a Multiplicative LOC
to the Cyclic Group ℤ / 2 ·ℤ ,
we form a Field

© Art Traynor 2011
Algebra
More generally, if “ p ” is a prime number :
Fields
Then ℤ / p ·ℤ , with the Multiplicative LOC inherited from ℤ , is a Field
F = { ℤ / p ·ℤ , x }
a x b ≡ a · b ( mod p )nn
nn
However ℤ / n ·ℤ is not a Field if “ n ” is composite ! i.e. “ n ” is not Prime
a ≡ b ( mod n ) denotes “ a ”
is congruent to “ b ” , Modulo
“ n ” not Prime
To prove this we must show that :nn
if
then there is
an integer “ b ”
such that
a · b ≡ 1 ( mod p )
a ≢ 0 ( mod p )
And thus… b ≡ a –1 ( mod p )

© Art Traynor 2011
Algebra
Fields
However ℤ / n ·ℤ is not a Field if “ n ” is composite ! i.e. “ n ” is not Prime
To prove this we must show that :nn
if
then there is
an integer “ b ”
such that
a · b ≡ 1 ( mod p )
a ≢ 0 ( mod p )
And thus… b ≡ a –1 ( mod p )
Recall moreover that p ·ℤ ⊂ ℤ is a Maximal Subgroup !

© Art Traynor 2011
Algebra
Fields
Recall moreover that p ·ℤ ⊂ ℤ is a Maximal Subgroup!
if
then “a” cannot be
an element of p ·ℤ a ∉ p ·ℤ
a ≢ 0 ( mod p )
p ·ℤ + a ·ℤ = ℤ
i.e. if we have a non-
zero element “ mod p ”
means its not divisible by
“ p ” , so it cannot thus
be a member of “ p ·ℤ ”
and if we Adjoin “ a ” to
this Subgroup we necessarily
encompass the entire Group
As “ p ·ℤ + a ·ℤ ” is a
Subgroup of ℤ containing “ p
·ℤ ” but which nevertheless
is not equivalent to “ p ·ℤ ”
i.e. “ p ·ℤ + a ·ℤ ” is
necessarily something more
than “ p ·ℤ ” … something
nothing less than “ a ·ℤ ”therefore… p ·ℤ + a ·ℤ ≠ p ·ℤ
Anything bigger than “ p ·ℤ ” must give us all of ℤ
because “ p ·ℤ ” is a Maximal Subgroup !


© Art Traynor 2011
Algebra
Fields

This means that any Element in ℤ can be written as a Sum of two Multiplesnn
So since 1 ∈ ℤ we can write :nn
1 = m ·p + b ·a
1 ≡ b ·a ( mod p )
Identities…
This product must be
divisible by “ p ”
However the complex numbers ℂ are quite different as a Field …
ℤ / p ·ℤ is not a subfield of ℂnn
In any Field we must have the Element “ 1 ” i.e. “ Unity ”
( as it is the Multiplicative Identity ) → 1 ∈ F
o Field Structure Criteria ( FSC )

© Art Traynor 2011
Algebra
Fields

In any Field we must have the Element “ 1 ” i.e. “ Unity ”
( as it is the Multiplicative Identity ) → 1 ∈ F
o
Wiki : “ Field ( mathematics )
a– 1 =
1
a
Definition 2.3
Group properties
3. Exhibits Distributive Property
We can add “ 1 ” or “ Unity ” to itself any number of timeso
“ n ” times
1 + 1 + …+ 1 ∈ F 1 ≤ n
which is true of all distinct elements in Subfields of ℂ

© Art Traynor 2011
Algebra
Fields

Wiki : “ Field ( mathematics )
a– 1 =
1
a
Definition 2.3
Group properties
3. Exhibits Distributive Property
We can add “ 1 ” or “ Unity ” to itself any number of timeso
“ n ” times
1 + 1 + …+ 1 ∈ F 1 ≤ n
which is true of all distinct elements in Subfields of ℂ
However in ℤ / p ·ℤ the element “ 1 ” “ p ” times
is equal to zero !
o

© Art Traynor 2011
Algebra
Fields

“ p ” times
p + p + …+ p = 0 in ℤ / p ·ℤ
However in ℤ / p ·ℤ the element “ 1 ” “ p ” times
is equal to zero !
o
If we never get to zero, then this is
akin to the Complex Numbers ℂ …
marching off to infinity

© Art Traynor 2011
Algebra
Fields

Galois asked : What are the Finite Fields beyond ℤ / p ·ℤ ?nn
What is the Order ( Cardinality ) of “ F ” ?
i.e. : | F | for a Finite Field
i.e. : we have one for each prime “ p ”
The answer is “ pn ” , for a prime “ p ” for 1 ≤ n
Finite Fields are found for “ n ” on the interval 1 ≤ n ≤ 5o
A Finite Field of Order six cannot be constructed retaining all the
axioms necessary to satisfy FSC
o
For each “ p ” and “ n ” there is a unique such “ F ” up to Isomorphismo

© Art Traynor 2011
Algebra
Now we can define what constitutes a Vector Space over a Field
Fields
A Vector Space “ V ” over a field is :
1 A Set “ V ” of vectors
2 “ V ” is an Abelian Group under Addition
Identity is the zero vector 0v
3 There is an operation ( Scalar Product )
V x F → V
The Law of Composition (LOC)
here “ x ” is understood to be
arithmetic multiplication and not
Vector or cross multiplication
( v , c ) ⟼ c·v
> What operation the comma
separator indicates…
> If only the Scalar is drawn
from “ F ” or if the vector is
as well…
Unclear:
Must conform to certain properties :
Associative Property must obtain
0 · v = 0v I’m pretty sure Professor Gross
here was referring to the Zero
Vector…?
1 · v = v
Distributive Property must obtain
( a + b ) · v = a ·v + b · v
( a · b ) · v = a ·( b · v )
Not only Artin, but also Professor Gross here echoes the
notion that there is some Chirality to the Distributive
Property of a Vector Space over a Field (i.e. ( i.e. 1HS
Distributive does not imply LHSD = RHSD )
Definition 2.3

© Art Traynor 2011
Algebra
Examples of Vector Spaces over an Abstract Field “ F ”
Fields
1
2
3
4
{ 0v } The Zero Vector Space
V = F The Field itself
V = F
n
= { ( ai , ai+1 , … , an – 1 , an ) ai ∊ F }|:
V = F [ x ] = { all polynomials p ( x )
with coefficients in the Field “ F ” }
n-tuples of Elements in the Field
We can multiply in this
space because we can
multiply Polynomials

© Art Traynor 2011
Algebra
Vector Subspaces
W ⊂ V is a Vector Subspace if :
Vector Spaces
Subspaces
W ⊂ V is a Subgroup under Addition “ + ”
with Identity Element of Nullity ( i.e. 0 )
and stable ( i.e. Closed ) under Scalar Multiplication by “ F ”
Example:
V = F
2
W = F
2
= { ( a1 , a2 : a1 = c · a2 , c ∊ F }

© Art Traynor 2011
Algebra
Vector Subspaces
Next we examine the notion of a Homomorphism in a Vector Space
Vector Spaces
Subspaces
A linear map ( transformation )
T : V → W
T ·( v + w ) = T · v + T · w
A Group Homomorphism
GHSC
Group Homomorphism Structure Criteria
T ·( c · v ) = c · T · v
In “w” i.e. ( c · v ) ∊ V ( ‘Big’ V )
In “w” i.e. ( T · v ) ∊ W ( ‘Big’ W )
If the Group Homomorphism is Bijective moreover , then we deem it Isomorphic
Kernel ker ( T ) = { v T · v = 0 } is a Subspace of V ( ‘Big’ V )|:
because T ·( c · v ) = c · T · v = c · 0w = 0w
and Image img ( T ) = { T · v ∊ W } is a Subspace of W ( ‘Big’ W )
Once we have a Homomorphism that preserves Scalar Multiplication ,
both its Kernel and Image are Subspaces


© Art Traynor 2011
Algebra
Vector Subspaces
Vector Spaces
Subspaces
If we have a Subspace W ⊂ V , we can define V/W ( vee-mod-dubya )
V/W ( vee-mod-dubya ) is a Quotient Space
Quotient Spacenn
A Set of Cosets of W in V ( i.e. W ∊ V )
which is an Abelian Group
W ⊂ V has the Structure of a Vector Space over “ F ”
f : V → V / W , a linear transformation with Kernel “ W ”
and it features a natural map
We needn’t worry about the
normality of the Subgroup
“ V ” because the Quotient
Group V/W is Abelian

© Art Traynor 2011
Algebra
Vector Subspaces
Vector Spaces
Subspaces
All of Group Theory “ ports ” to Vector Space Theory
once we have imposed the added restrictions that :

1
2
The Subgroup is stable under multiplication
The Group Homomorphism commutes with Scalar Multiplication

© Art Traynor 2011
Algebra
Last time we defined a Field
Vector Spaces
Abstract Fields
Example:
( ℝ , ℤ / p ·ℤ )
A Vector Space over “ F ”
“ V ” over “ F ”
An Abelian Group together with
a Scalar Multiplication Law ( LOC ) from “ F ”
We defined a Linear Map … a Homomorphism
T : V → W Homomorphism of “ F ” Vector Spaces

Algebra(04)_160619_01

Recommended

Recommended

More Related Content

What's hot

What's hot (14)

Similar to Algebra(04)_160619_01

Similar to Algebra(04)_160619_01 (10)

More from Art Traynor

More from Art Traynor (20)

Algebra(04)_160619_01