Chapter 6 Section 3 Similar Figures
•Download as PPT, PDF•
1 like•1,824 views
Chapter 6, Section 3: Similar Figures and Scale Drawings
Report
Share
Report
Share
Recommended
Recommended
More Related Content
What's hot
What's hot (20)
Viewers also liked
Viewers also liked (20)
Chapter 6 Section 4 Fractions Decimals And Percents

Chapter 6 Section 4 Fractions Decimals And Percents
Similar to Chapter 6 Section 3 Similar Figures
Similar to Chapter 6 Section 3 Similar Figures (20)
More from Jessca Lundin
More from Jessca Lundin (16)
Recently uploaded
Recently uploaded (20)
Vishram Singh - Textbook of Anatomy Upper Limb and Thorax.. Volume 1 (1).pdf

Vishram Singh - Textbook of Anatomy Upper Limb and Thorax.. Volume 1 (1).pdf
Basic Civil Engineering first year Notes- Chapter 4 Building.pptx

Basic Civil Engineering first year Notes- Chapter 4 Building.pptx
Fostering Friendships - Enhancing Social Bonds in the Classroom

Fostering Friendships - Enhancing Social Bonds in the Classroom
Mixin Classes in Odoo 17 How to Extend Models Using Mixin Classes

Mixin Classes in Odoo 17 How to Extend Models Using Mixin Classes
Unit-V; Pricing (Pharma Marketing Management).pptx

Unit-V; Pricing (Pharma Marketing Management).pptx
UGC NET Paper 1 Mathematical Reasoning & Aptitude.pdf

UGC NET Paper 1 Mathematical Reasoning & Aptitude.pdf
This PowerPoint helps students to consider the concept of infinity.

This PowerPoint helps students to consider the concept of infinity.
Micro-Scholarship, What it is, How can it help me.pdf

Micro-Scholarship, What it is, How can it help me.pdf
Chapter 6 Section 3 Similar Figures
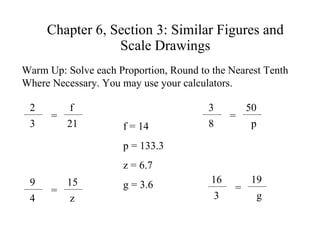
- 1. Chapter 6, Section 3: Similar Figures and Scale Drawings Warm Up: Solve each Proportion, Round to the Nearest Tenth Where Necessary. You may use your calculators. f = 14 p = 133.3 z = 6.7 g = 3.6 2 3 = f 21 3 8 = 50 p 9 4 = 15 z 16 3 = 19 g
- 3. These Figures Are Similar The symbol ~ means “ is similar to ”. To the right, ΔABC ~ ΔXYZ.
- 9. REMEMBER TO KEEP YOUR RATIOS INLINE!!! THIS compared to THAT. THIS AND THAT have to be in the same ORDER every TIME .
- 12. Guess Where This Is… This is the ratio for this Scale Representation!
